
NCERT Solutions for Vector Algebra Class 12 Maths Chapter 10 Solutions - FREE PDF Download
NCERT Solutions Class 12 Maths Chapter Vector Algebra can now be easily acquired by just visiting the main website of Vedantu. Here you can get complete solutions that are comprehensive and well-made to ease students’ doubts. Our Vector Class 12 Solutions can be referred to for understanding, practice, and revision of the concept.

- 5.1Exercise 10.1
Vectors Class 12 NCERT Solutions PDF has been created and designed by highly qualified and experienced teachers who have been involved in research work in a particular subject for many years. Solutions are available, especially for the convenience of students who want to explore more about a topic and to procure in-depth knowledge.
Glance on Class 12 Maths Chapter 10 - Vector Algebra
NCERT Solutions Class 12 Maths Chapter Vector Algebra explains vector operations, addition, subtraction, scalar multiplication, dot and cross products, and their applications.
Vectors are quantities with both magnitude (size) and direction. Examples include displacement, force, and velocity.
Scalars, on the other hand, only have magnitude (e.g., temperature, mass).
Vectors are represented using arrows, with the starting point (tail) and ending point (head) signifying direction.
The article Vector Algebra Class 12 NCERT Solutions contains chapter notes, important questions, exemplar solutions, exercises, and video links for Chapter 10 - Vector Algebra, which you can download as PDFs.
There are four exercises and one Miscellaneous Exercise (73 fully solved questions) in maths chapter 10 Vector Algebra Class 12 Solutions PDF.
Access Exercise wise NCERT Solutions for Chapter 10 Maths Class 12



Exercises Under NCERT Solutions for Class 12 Maths Chapter 10 Vector Algebra
Vectors Class 12 NCERT Solutions introduces students to the concept of vectors and their algebraic operations. It covers topics such as scalar and vector products, triple products, and their applications in geometry and physics.
The chapter is divided into four exercises and one miscellaneous exercise. Here is a brief summary of each exercise:
Exercise 10.1: This exercise has ten questions that ask students to find the direction cosines of a given vector and vice versa.
Exercise 10.2: This exercise has eleven questions that ask students to find the magnitude and direction of a given vector, as well as to perform vector operations such as addition, subtraction, and scalar multiplication.
Exercise 10.3: This exercise has seven questions that ask students to find the scalar and vector products of two given vectors.
Exercise 10.4: This exercise has six questions that ask students to find the scalar and vector triple products of given vectors.
Miscellaneous Exercise: This exercise has six questions that ask students to apply the concepts of vector algebra to solve problems in geometry and physics.
Overall, this chapter is essential for students who want to pursue higher education in Mathematics and Physics. It provides a solid foundation for students to understand the concepts of vectors and their applications in real-world problems.
Access NCERT Solutions for Class 12 Maths Chapter 10 – Vector Algebra
Exercise 10.1
1. Represent graphically a displacement of $40\;{\text{km}},{30^\circ }$ east of north.
Ans:
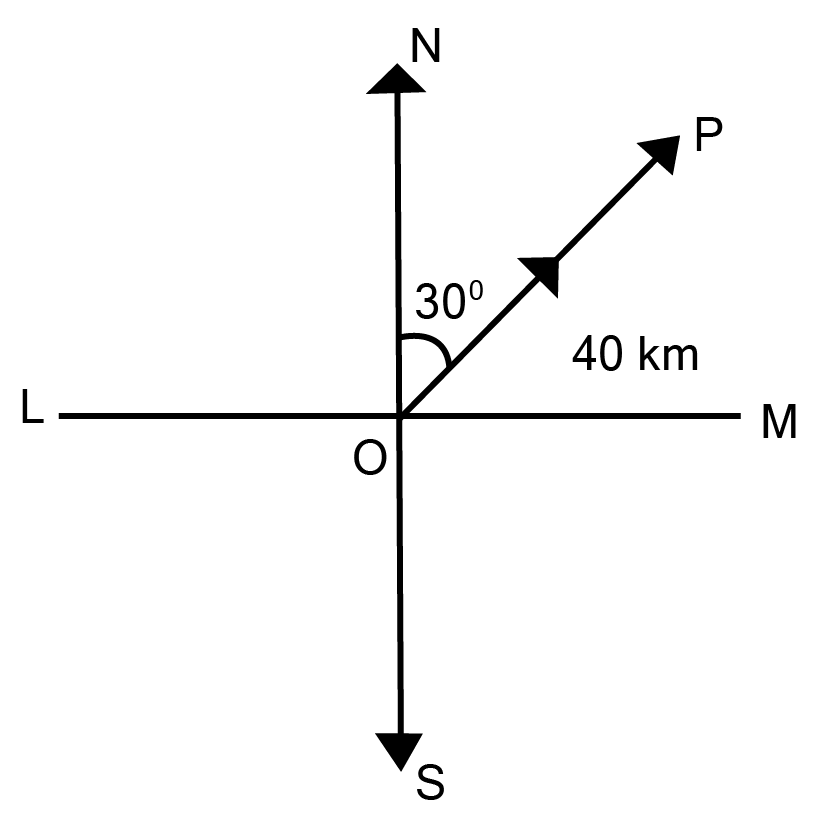
Here, the vector OP is representing the displacement of $40\;{\text{km}},{30^\circ }$ in East of North direction.
2. Classify the following measures as scalars and vectors.
i. $10\;{\text{kg}}$
Ans: $10\;{\text{kg}}$is a scalar quantity because it has only magnitude not direction.
ii. 2 meters north-west
Ans: 2 meters north-west is a vector quantity because it has both magnitude as well as direction.
iii. ${40^\circ }$
Ans: ${40^\circ }$ is a scalar quantity because it has only magnitude, not direction.
iv. $40$ watt
Ans: 40 watts is a scalar quantity because it has only magnitude, not direction.
v. ${10^{ - 19}}$coulomb
Ans: ${10^{ - 19}}$Coulomb is a scalar quantity because it has only magnitude not direction.
vi. $20\;{\text{m/}}{{\text{s}}^2}$
Ans: $20\;{\text{m/}}{{\text{s}}^2}$ is a vector quantity because it has both magnitude as well as direction.
3. Classify the following as scalar and vector quantities:-
i. Time period
Ans: Time period is a scalar quantity because it has only magnitude.
ii. distance
Ans: Distance is a scalar quantity because it has only magnitude.
iii. force
Ans: Force is a vector quantity because it has both magnitude as well as direction.
iv. velocity
Ans: Velocity is a vector quantity because it has both magnitude as well as direction.
v. work done
Ans: Work done is a scalar quantity because it has only magnitude.
4. In Figure, identify the following vectors.
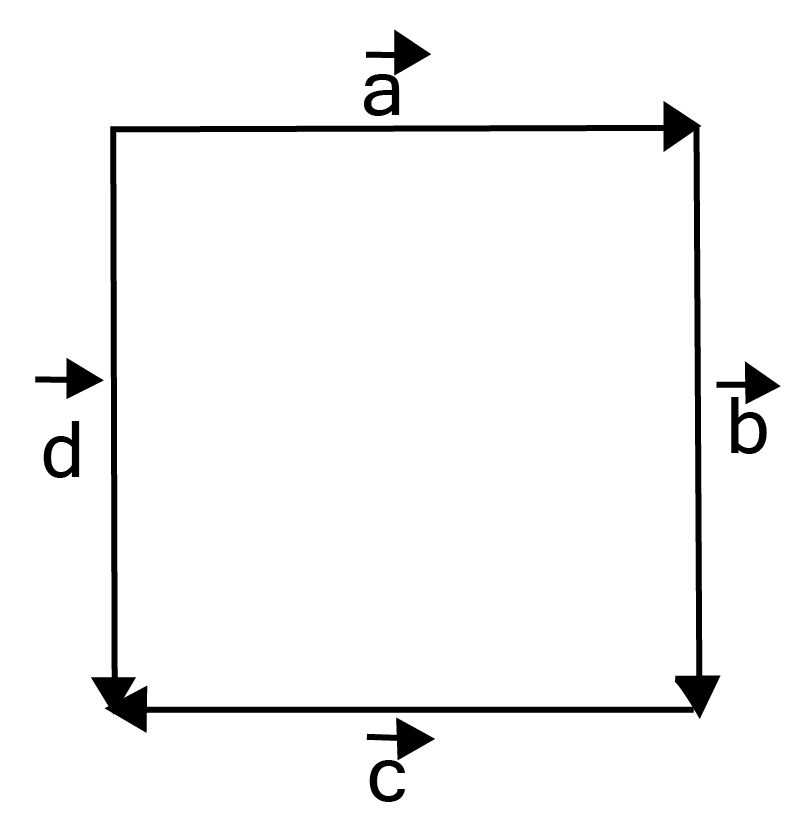
i. Co-initial
Ans: Vectors $\vec a$ and $\vec d$ are co-initial.
ii. Equal
Ans: Vectors $\vec b$ and $\vec d$ are equal.
iii. Collinear but not equal
Ans: Vectors $\vec a$ and $\vec c$ are collinear but not equal.
5. Answer the following as true or false.
i.$\vec a$ and $ - \vec a$ and are collinear.
Ans: True
ii. Two collinear vectors are always equal in magnitude.
Ans: False
iii. Two vectors having same magnitude are collinear.
Ans: False
iv. Two collinear vectors having the same magnitude are equal.
Ans: False
Exercise 10.2
1. Compute the magnitude of the following vectors:-
$\vec a = \hat i + \hat j + \hat k\;;\;\vec b = 2\hat i - 7\hat j - 3\hat k\;;\;\vec c = \dfrac{1}{{\sqrt 3 }}\hat i + \dfrac{1}{{\sqrt 3 }}\hat j - \dfrac{1}{{\sqrt 3 }}\hat k$
Ans: $|\vec a| = \sqrt {{{(1)}^2} + {{(1)}^2} + {{(1)}^2}} = \sqrt 3 $
$|\vec b| = \sqrt {{{(2)}^2} + {{( - 7)}^2} + {{( - 3)}^2}} $
$ = \sqrt {4 + 49 + 9} $
$ = \sqrt {62} $
$|\vec c| = \sqrt {{{\left( {\dfrac{1}{{\sqrt 3 }}} \right)}^2} + {{\left( {\dfrac{1}{{\sqrt 3 }}} \right)}^2} - {{\left( {\dfrac{1}{{\sqrt 3 }}} \right)}^2}} $
$ = \sqrt {\dfrac{1}{3} + \dfrac{1}{3} + \dfrac{1}{3}} = 1$
2. Write two different vectors having the same magnitude.
Ans: $\vec a = (\hat i - 2\hat j + 3\hat k)$ and $\vec b = (2\hat i + \hat j - 3\hat k)$.
$|\vec a| = \sqrt {{1^2} + {{( - 2)}^2} + {3^2}} = \sqrt {1 + 4 + 9} = \sqrt {14} $
$|\vec b| = \sqrt {{2^2} + {1^2} + {{( - 3)}^2}} = \sqrt {4 + 1 + 9} = \sqrt {14} $
But $\vec a \ne \vec b$
3. Write two different vectors having same direction.
Ans: $\vec p = (\hat i + \hat j + \hat k)$ and $\vec q = (2\hat i + 2\hat j + 2\hat k)$.
The Direction Cosines of $\vec p$ are $a = \dfrac{1}{{\sqrt {{1^2} + {1^2} + {1^2}} }} = \dfrac{1}{{\sqrt 3 }},b = \dfrac{1}{{\sqrt {{1^2} + {1^2} + {1^2}} }} = \dfrac{1}{{\sqrt 3 }},c = \dfrac{1}{{\sqrt {{1^2} + {1^2} + {1^2}} }} = \dfrac{1}{{\sqrt 3 }}$
The Direction Cosines of $\vec q$ are$a = \dfrac{2}{{\sqrt {{2^2} + {2^2} + {2^2}} }} = \dfrac{2}{{2\sqrt 3 }} = \dfrac{1}{{\sqrt 3 }},b = \dfrac{2}{{\sqrt {{2^2} + {2^2} + {2^2}} }} = \dfrac{2}{{2\sqrt 3 }} = \dfrac{1}{{\sqrt 3 }},c = \dfrac{2}{{\sqrt {{2^2} + {2^2} + {2^2}} }} = \dfrac{2}{{2\sqrt 3 }} = \dfrac{1}{{\sqrt 3 }}$
The Direction Cosines of $\vec p$ and $\vec q$ are equal but $\vec p \ne \vec q$.
4. Find the values of x and y so that the vectors $2\hat i + 3\hat j$ and $x\hat i + y\hat j$ are equal.
Ans: $2\hat i + 3\hat j = x\hat i + y\hat j \Rightarrow x = 2,y = 3$
5. Find the scalar and vector components of the vector with initial point $(2,1)$ and terminal point $( - 5,7)$.
Ans: Let the points be ${\text{P}}(2,1)$ and ${\text{Q}}( - 5,7)$
$\overrightarrow {PQ} = ( - 5 - 2)\hat i + (7 - 1)\hat j$
$ \Rightarrow \overrightarrow {PQ} = - 7\hat i + 6\hat j$
So, scalar components of required vector are $ - 7$ and 6 and the vector components are $ - 7\hat i$ and $6\hat j$.
6. Find the sum of the vectors $\vec a = \hat i - 2\hat j + \hat k\;,\;\vec b = - 2\hat i + 4\hat j + 5\hat k$ and $\vec c = \hat i - 6\hat j - 7\hat k$.
Ans: $\vec a + \vec b + \vec c = (1 - 2 + 1)\hat i + ( - 2 + 4 - 6)\hat j + (1 + 5 - 7)\hat k{\text{ }} = 0\hat i - 4\hat j - 1\hat k{\text{ }} = - \;4\hat j - \hat k{\text{ }}$
7. Find the unit vector in the direction of the vector $\vec a = \hat i + \hat j + 2\hat k$.
Ans: $|\vec a| = \sqrt {{1^2} + {1^2} + {2^2}} = \sqrt {1 + 1 + 4} = \sqrt 6 $
$\therefore \hat a = \dfrac{{\vec a}}{{|\vec a|}} = \dfrac{{\hat i + \hat j + 2\hat k}}{{\sqrt 6 }} = \dfrac{1}{{\sqrt 6 }}\hat i + \dfrac{1}{{\sqrt 6 }}\hat j + \dfrac{2}{{\sqrt 6 }}\hat k$
8. Find the unit vector in the direction of vector $\overrightarrow {PQ} $, where ${\text{P}}$ and ${\text{Q}}$ are the points $(1,2,3)$ and $(4,5,6)$, respectively.
Ans: $\overrightarrow {PQ} = (4 - 1)\hat i + (5 - 2)\hat j + (6 - 3)k = 3\hat i + 3\hat j + 3k$
$|\overrightarrow {PQ} | = \sqrt {{3^2} + {3^2} + {3^2}} = \sqrt {9 + 9 + 9} = \sqrt {27} = 3\sqrt 3 $
So, unit vector $ = \dfrac{{\overrightarrow {PQ} }}{{|\overrightarrow {PQ} |}} = \dfrac{{3\hat i + 3\hat j + 3\hat k}}{{3\sqrt 3 }} = \dfrac{1}{{\sqrt 3 }}\hat i + \dfrac{1}{{\sqrt 3 }}\hat j + \dfrac{1}{{\sqrt 3 }}\hat k$
9. For given vectors, $\vec a = 2\hat i - \hat j + 2\hat k$ and $\vec b = - \hat i + \hat j - \hat k$, find the unit vector in the direction of the vector $\vec a + \vec b$.
Ans: $\vec a + \vec b = (2 - 1)\hat i + ( - 1 + 1)\hat j + (2 - 1)\hat k = 1\hat i + 0\hat j + 1\hat k = \hat i + \hat k$
$|\vec a + \vec b| = \sqrt {{1^2} + {1^2}} = \sqrt 2 $
So, unit vector $ = \dfrac{{(\vec a + \vec b)}}{{|\vec a + \vec b|}} = \dfrac{{\hat i + \hat k}}{{\sqrt 2 }} = \dfrac{1}{{\sqrt 2 }}\hat i + \dfrac{1}{{\sqrt 2 }}\hat k$
10. Find a vector in the direction of vector $5\hat i - \hat j + 2\hat k$ which has magnitude 8 units.
Ans: $\vec a = 5\hat i - \hat j + 2\hat k$
$|\vec a| = \sqrt {{5^2} + {{( - 1)}^2} + {2^2}} = \sqrt {25 + 1 + 4} = \sqrt {30} $
$\therefore \hat a = \dfrac{{\vec a}}{{|\vec a|}} = \dfrac{{5\hat i - \hat j + 2\hat k}}{{\sqrt {30} }}$
So, a vector in direction of $5\hat i - \hat j + 2\hat k$ with magnitude 8 units is:$8\hat a = 8\left( {\dfrac{{5\hat i - \hat j + 2\hat k}}{{\sqrt {30} }}} \right) = \dfrac{{40}}{{\sqrt {30} }}\hat i - \dfrac{8}{{\sqrt {30} }}\hat j + \dfrac{{16}}{{\sqrt {30} }}\hat k$
11. Show that the vectors $2\hat i - 3\hat j + 4\hat k$ and $ - 4\hat i + 6\hat j - 8\hat k$ are collinear.
Ans: $\vec a = 2\hat i - 3\hat j + 4\hat k$ and $\vec b = - 4\hat i + 6\hat j - 8\hat k$
$\vec b = - 4\hat i + 6\hat j - 8\hat k = - 2(2\hat i - 3\hat j + 4\hat k) = - 2\vec a$
$\therefore \vec b = \lambda \vec a,\;\;\lambda = - 2$
So, the given vectors are collinear.
12. Find the direction cosines of the vector $\hat i + 2\hat j + 3\hat k$.
Ans: $\vec a = \hat i + 2\hat j + 3\hat k$.
$|\vec a| = \sqrt {{1^2} + {2^2} + {3^2}} = \sqrt {1 + 4 + 9} = \sqrt {14} $
So, the Direction cosines of $\vec a$ are $\left( {\dfrac{1}{{\sqrt {14} }},\dfrac{2}{{\sqrt {14} }},\dfrac{3}{{\sqrt {14} }}} \right)$
13. Find the direction cosines of the vector joining the points ${\text{A}}(1,2, - 3)$ and ${\text{B}}( - 1, - 2,1)$ directed from A to b.
Ans: $\overrightarrow {{\text{AB}}} = ( - 1 - 1)\hat i + ( - 2 - 2)\hat j + \{ 1 - ( - 3)\} \hat k$
$ \Rightarrow \overrightarrow {{\text{AB}}} = - 2\hat i - 4\hat j + 4\hat k$
$|\overrightarrow {{\text{AB}}} | = \sqrt {{{( - 2)}^2} + {{( - 4)}^2} + {4^2}} = \sqrt {4 + 16 + 16} = \sqrt {36} = 6$
So, the Direction cosines of $\overrightarrow {{\text{AB}}} $ are $\left( { - \dfrac{2}{6}, - \dfrac{4}{6},\dfrac{4}{6}} \right) = \left( { - \dfrac{1}{3}, - \dfrac{2}{3},\dfrac{2}{3}} \right)$
14. Show that the vector $\hat i + \hat j + \hat k$ is equally inclined to the axes OX, OY and OZ .
Ans: $\vec a = \hat i + \hat j + \hat k$
$|\vec a| = \sqrt {{1^2} + {1^2} + {1^2}} = \sqrt 3 $
So, the Direction Cosines of $\vec a$ are $\left( {\dfrac{1}{{\sqrt 3 }},\dfrac{1}{{\sqrt 3 }},\dfrac{1}{{\sqrt 3 }}} \right)$
$\cos \alpha = \dfrac{1}{{\sqrt 3 }},\cos \beta = \dfrac{1}{{\sqrt 3 }},\cos \gamma = \dfrac{1}{{\sqrt 3 }}$
So, the vector is equally inclined to OX, OY, and ${\text{OZ}}$.
15. Find the position vector of a point ${\text{R}}$ which divides the line joining two points P and Q whose position vectors are $\hat i + 2\hat j - \hat k$ and $ - \hat i + \hat j + \hat k$ respectively, in the ratio 2 : 1 ,
i. Internally
Ans: $\overrightarrow {{\text{OP}}} = \hat i + 2\hat j - \hat k$ and $\overrightarrow {{\text{OQ}}} = - \hat i + \hat j + \hat k$
The position vector of ${\text{R}}$ is $\overrightarrow {{\text{OR}}} = \dfrac{{2( - \hat i + \hat j + \hat k) + 1(\hat i + 2\hat j - \hat k)}}{{2 + 1}} = \dfrac{{( - 2\hat i + 2\hat j + 2\hat k) + (\hat i + 2\hat j - \hat k)}}{3}$
$ = \dfrac{{ - \;\hat i + 4\hat j + \hat k}}{3} = - \dfrac{1}{3}\hat i + \dfrac{4}{3}\hat j + \dfrac{1}{3}\hat k$
ii. Externally.
Ans: The position vector of $R$ is $\overrightarrow {{\text{OR}}} = \dfrac{{2( - \hat i + \hat j + \hat k) - 1(\hat i + 2\hat j - \hat k)}}{{2 - 1}} = ( - 2\hat i + 2\hat j + 2\hat k) - (\hat i + 2\hat j - \hat k)$
$ = - 3\hat i + 3\hat k$
16. Find the position vector of the mid point of the vector joining the points P(2,3,4) and Q(4,1,-2).
Ans: The position vector of $R$ is
$\overrightarrow {{\text{OR}}} = \dfrac{{(2\hat i + 3\hat j + 4\hat k) + (4\hat i + \hat j - 2\hat k)}}{2} = \dfrac{{(2 + 4)\hat i + (3 + 1)\hat j + (4 - 2)\hat k}}{2}$
$ = \dfrac{{6\hat i + 4\hat j + 2\hat k}}{2} = 3\hat i + 2\hat j + \hat k$
17. Show that the points ${\text{A}},{\text{B}}$ and ${\text{C}}$ with position vectors, $\vec a = 3\hat i - 4\hat j - 4\hat k\;,\;\vec b = 2\hat i - \hat j + \hat k$ and $\vec c = \hat i - 3\hat j - 5\hat k$, respectively form the vertices of a right angled triangle.
Ans:
$\overrightarrow {{\text{AB}}} = \vec b - \vec a = (2 - 3)\hat i + ( - 1 + 4)\hat j + (1 + 4)\hat k = - \hat i + 3\hat j + 5\hat k$
$\overrightarrow {{\text{BC}}} = \vec c - \vec b = (1 - 2)\hat i + ( - 3 + 1)\hat j + ( - 5 - 1)\hat k = - \hat i + 2\hat j - 6\hat k$
$\overrightarrow {{\text{CA}}} = \vec a - \vec c = (3 - 1)\hat i + ( - 4 + 3)\hat j + ( - 4 + 5)\hat k = 2\hat i - \hat j + \hat k$
$|\overrightarrow {{\text{AB}}} {|^2} = {( - 1)^2} + {3^2} + {5^2} = 1 + 9 + 25 = 35$
$|\overrightarrow {{\text{BC}}} {|^2} = {( - 1)^2} + {( - 2)^2} + {( - 6)^2} = 1 + 4 + 36 = 41$
$|\overrightarrow {CA} {|^2} = {2^2} + {( - 1)^2} + {1^2} = 4 + 1 + 1 = 6$
$|\overrightarrow {{\text{AB}}} {|^2} + |\overrightarrow {{\text{CA}}} {|^2} = 35 + 6 = 41 = |\overrightarrow {{\text{BC}}} {|^2}$
So, ABC is a right angled triangle.
18. In triangle ABC, which of the following is not true?
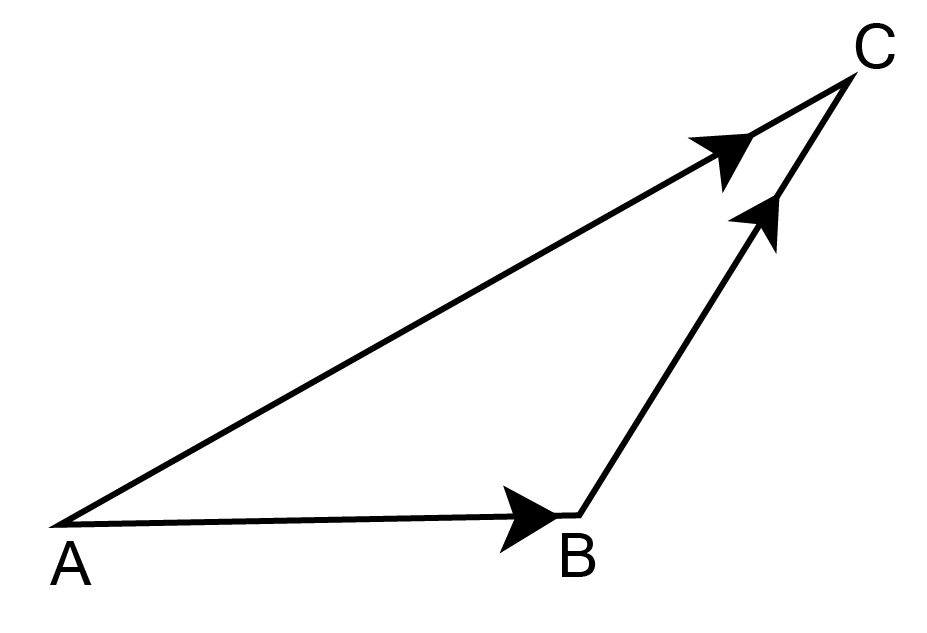
a. $\overrightarrow {{\text{AB}}} + \overrightarrow {{\text{BC}}} + \overrightarrow {{\text{CA}}} = \vec 0$
b. $\overrightarrow {{\text{AB}}} + \overrightarrow {{\text{BC}}} - \overrightarrow {{\text{AC}}} = \vec 0$
c. $\overrightarrow {{\text{AB}}} + \overrightarrow {{\text{BC}}} - \overrightarrow {{\text{CA}}} = \vec 0$
d. $\overrightarrow {{\text{AB}}} - \overrightarrow {{\text{CB}}} + \overrightarrow {{\text{CA}}} = \vec 0$
Ans:
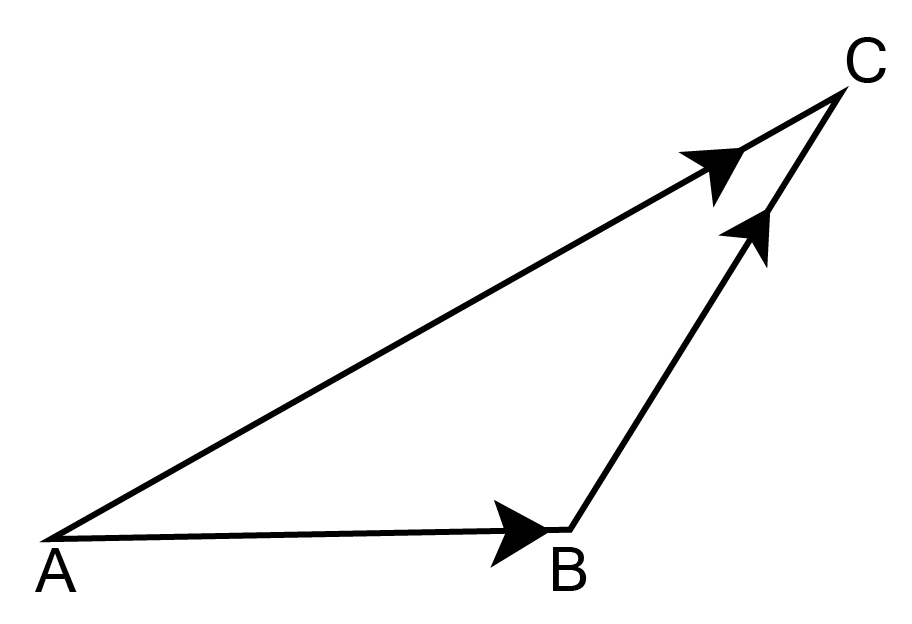
$\overrightarrow {{\text{AB}}} + \overrightarrow {{\text{BC}}} = \overrightarrow {{\text{AC}}} $
$ \Rightarrow \overrightarrow {{\text{AB}}} + \overrightarrow {{\text{BC}}} = - \overrightarrow {{\text{CA}}} $
$ \Rightarrow \overrightarrow {{\text{AB}}} + \overrightarrow {{\text{BC}}} + \overrightarrow {{\text{CA}}} = \vec 0$
$\overrightarrow {{\text{AB}}} + \overrightarrow {{\text{BC}}} = \overrightarrow {{\text{AC}}} $
$ \Rightarrow \overrightarrow {{\text{AB}}} + \overrightarrow {{\text{BC}}} - \overrightarrow {{\text{AC}}} = \vec 0$
$\overrightarrow {{\text{AB}}} + \overrightarrow {{\text{BC}}} + \overrightarrow {{\text{CA}}} = \vec 0$
$\overrightarrow {{\text{AB}}} - \overrightarrow {{\text{CB}}} + \overrightarrow {{\text{CA}}} = \vec 0$
If $\overrightarrow {{\text{AB}}} + \overrightarrow {{\text{BC}}} - \overrightarrow {{\text{CA}}} = \vec 0$
$\overrightarrow {{\text{AC}}} = \overrightarrow {{\text{CA}}} $
$ \Rightarrow \overrightarrow {{\text{AC}}} = - \overrightarrow {{\text{AC}}} $
$ \Rightarrow \overrightarrow {{\text{AC}}} + \overrightarrow {{\text{AC}}} = \vec 0$
$ \Rightarrow 2\overrightarrow {{\text{AC}}} = \vec 0$
$ \Rightarrow \overrightarrow {{\text{AC}}} = \vec 0$, which is not true.
So, the equation given in option ${\text{C}}$ is False.
Hence, the correct answer is ${\text{C}}$.
19. If $\vec a$ and $\vec b$ are two collinear vectors, then which of the following are incorrect?
a. $\vec b = \lambda \vec a$, for some scalar $\lambda $
b. $\vec a = \pm \vec b$
c. the respective components of $\vec a$ and $\vec b$ are proportional
d. both the vectors $\vec a$ and $\vec b$ have same direction, but different magnitudes
Ans: If $\vec a$ and $\vec b$ are collinear vectors, they are parallel. $\vec b = \lambda \vec a($ scalar $\lambda )$
If $\lambda = \pm 1$, then $\vec a = \pm \vec b$
If $\vec a = {a_1}\hat i + {a_2}\hat j + {a_3}\hat k$ and $\vec b = {b_1}\hat i + {b_2}\hat j + {b_3}\hat k$,
$\vec b = \lambda \vec a \Rightarrow {b_1}\hat i + {b_2}\hat j + {b_3}\hat k = \lambda \left( {a\hat i + {a_2}\hat j + {a_3}\hat k} \right)$
$ \Rightarrow {b_1}\hat i + {b_2}\hat j + {b_3}\hat k = \left( {\lambda {a_1}} \right)\hat i + \left( {\lambda {a_2}} \right)\hat j + \left( {\lambda {a_3}} \right)\hat k$
$ \Rightarrow {b_1} = \lambda {a_1},{b_2} = \lambda {a_2},{b_3} = \lambda {a_3}$
$ \Rightarrow \dfrac{{{b_1}}}{{{a_1}}} = \dfrac{{{b_2}}}{{{a_2}}} = \dfrac{{{b_3}}}{{{a_3}}} = \lambda $
Thus, respective components of $\vec a$ and $\vec b$ are proportional.
But, $\vec a$ and $\vec b$ may have different directions. So, option D is incorrect. The correct answer is ${\text{D}}$.
Exercise 10.3
1. Find the angle between two vectors $\vec a$ and $\vec b$ with magnitudes $\sqrt 3 $ and 2, respectively having $\vec a \cdot \vec b = \sqrt 6 $
Ans: $|\vec a| = \sqrt 3 \;,\;|\vec b| = 2\;,\;\vec a\;.\;\vec b = \sqrt 6 $
$\therefore \sqrt 6 = \sqrt 3 \times 2 \times \cos \theta $
$ \Rightarrow \cos \theta = \dfrac{{\sqrt 6 }}{{\sqrt 3 \times 2}}$
$ \Rightarrow \cos \theta = \dfrac{1}{{\sqrt 2 }}$
$ \Rightarrow \theta = \dfrac{\pi }{4}$
2. Find the angle between the vectors $i - 2\hat j + 3\hat k$ and $3\hat i - 2\hat j + \hat k$.
Ans: $|\vec a| = \sqrt {{1^2} + {{( - 2)}^2} + {3^2}} = \sqrt {1 + 4 + 9} = \sqrt {14} $
$|\vec b| = \sqrt {{3^2} + {{( - 2)}^2} + {1^2}} = \sqrt {9 + 4 + 1} = \sqrt {14} $
$\vec a\;.\;\vec b = (i - 2\hat j + 3\hat k)(3\hat i - 2\hat j + \hat k)$
$ = 1.3 + ( - 2)( - 2) + 3.1$
$ = 3 + 4 + 3$
$ = 10$
$\therefore 10 = \sqrt {14} \sqrt {14} \cos \theta $
$ \Rightarrow \cos \theta = \dfrac{{10}}{{14}}$
$ \Rightarrow \theta = {\cos ^{ - 1}}\left( {\dfrac{5}{7}} \right)$
3. Find the projection of the vector $\hat i - \hat j$ on the vector $i + \hat j$.
Ans: $\vec a = \hat i - \hat j$ and $\vec b = \hat i + \hat j$
Projection of $\vec a$ on $\vec b$ is $\dfrac{1}{{\mid \vec b|}}(\vec a \cdot \vec b) = \dfrac{1}{{\sqrt {1 + 1} }}\{ 1.1 + ( - 1)(1)\} = \dfrac{1}{{\sqrt 2 }}(1 - 1) = 0$
4. Find the projection of the vector $\hat i + 3\hat j + 7\hat k$ on the vector $7\hat i - \hat j + 8\hat k$.
Ans: $\vec a = \hat i + 3\hat j + 7\hat k$ and $\vec b = 7\hat i - \hat j + 8\hat k$
Projection of $\vec a$ on $\vec b$ is $\dfrac{1}{{|\vec b|}}(\vec a \cdot \vec b) = \dfrac{1}{{\sqrt {{7^2} + {{( - 1)}^2} + {8^2}} }}\{ 1(7) + 3( - 1) + 7(8)\} = \dfrac{{7 - 3 + 56}}{{\sqrt {49 + 1 + 64} }} = \dfrac{{60}}{{\sqrt {114} }}$
5. Show that each of the given three vectors is a unit vector, which are mutually perpendicular to each other. $\dfrac{1}{7}(2\hat i + 3\hat j + 6\hat k)\;\;,\;\;\dfrac{1}{7}(3\hat i - 6\hat j + 2\hat k)\;\;,\;\;\dfrac{1}{7}(6\hat i + 2\hat j - 3\hat k)$
Ans: $\vec a = \dfrac{1}{7}(2\hat i + 3\hat j + 6\hat k) = \dfrac{2}{7}\hat i + \dfrac{3}{7}\hat j + \dfrac{6}{7}\hat k$,
$\vec b = \dfrac{1}{7}(3\bar i - 6\hat j + 2\hat k) = \dfrac{3}{7}\hat i - \dfrac{6}{7}\bar j + \dfrac{2}{7}\hat k$
$\vec c = \dfrac{1}{7}(6\hat i + 2\hat j - 3\hat k) = \dfrac{6}{7}\hat i + \dfrac{2}{7}\hat j - \dfrac{3}{7}\hat k$
$|\vec a| = \sqrt {{{\left( {\dfrac{2}{7}} \right)}^2} + {{\left( {\dfrac{3}{7}} \right)}^2} + {{\left( {\dfrac{6}{7}} \right)}^2}} = \sqrt {\dfrac{4}{{49}} + \dfrac{9}{{49}} + \dfrac{{36}}{{49}}} = 1$
$|\vec b| = \sqrt {{{\left( {\dfrac{3}{7}} \right)}^2} + {{\left( { - \dfrac{6}{7}} \right)}^2} + {{\left( {\dfrac{2}{7}} \right)}^2}} = \sqrt {\dfrac{9}{{49}} + \dfrac{{36}}{{49}} + \dfrac{4}{{49}}} = 1$
$|\vec c| = \sqrt {{{\left( {\dfrac{6}{7}} \right)}^2} + {{\left( {\dfrac{2}{7}} \right)}^2} + {{\left( { - \dfrac{3}{7}} \right)}^2}} = \sqrt {\dfrac{{36}}{{49}} + \dfrac{4}{{49}} + \dfrac{9}{{49}}} = 1$
So, each of the vectors is a unit vector.
$\vec a\;.\;\vec b = \dfrac{2}{7} \times \dfrac{3}{7} + \dfrac{3}{7} \times \left( {\dfrac{{ - 6}}{7}} \right) + \dfrac{6}{7} \times \dfrac{2}{7} = \dfrac{6}{{49}} - \dfrac{{18}}{{49}} + \dfrac{{12}}{{49}} = 0$
$\vec b \cdot \vec c = \dfrac{3}{7} \times \dfrac{6}{7} + \left( {\dfrac{{ - 6}}{7}} \right) \times \dfrac{2}{7} + \dfrac{2}{7} \times \left( {\dfrac{{ - 3}}{7}} \right) = \dfrac{{18}}{{49}} - \dfrac{{12}}{{49}} - \dfrac{6}{{49}} = 0$
$\vec c\;.\;\vec a = \dfrac{6}{7} \times \dfrac{2}{7} + \dfrac{2}{7} \times \dfrac{3}{7} + \left( {\dfrac{{ - 3}}{7}} \right) \times \dfrac{6}{7} = \dfrac{{12}}{{49}} + \dfrac{6}{{49}} - \dfrac{{18}}{{49}} = 0$
So, given vectors are mutually perpendicular to each other.
6. Find $|\vec a|$ and $|\vec b|$, if $(\vec a + \vec b) \cdot (\vec a - \vec b) = 8$ and $|\overrightarrow a |\; = \;8|\vec b|$.
Ans: $(\vec a + \vec b) \cdot (\vec a - \vec b) = 8$
$ \Rightarrow \vec a\vec a - \vec a\;.\;\vec b + \vec b \cdot \vec a - \vec b\vec b = 8$
$ \Rightarrow |\vec a{|^2} - |\vec b{|^2} = 8$
$ \Rightarrow {(8|\vec b|)^2} - |\vec b{|^2} = 8$
$ \Rightarrow 64|\vec b{|^2} - |\vec b{|^2} = 8$
$ \Rightarrow 63|\vec b{|^2} = 8$
$ \Rightarrow |\vec b{|^2} = \dfrac{8}{{63}}$
$ \Rightarrow |\vec b| = \sqrt {\dfrac{8}{{63}}} $
$ \Rightarrow |\vec b| = \dfrac{{2\sqrt 2 }}{{3\sqrt 7 }}$
$|\vec a| = 8|\vec b| = \dfrac{{8 \times 2\sqrt 2 }}{{3\sqrt 7 }} = \dfrac{{16\sqrt 2 }}{{3\sqrt 7 }}$
7. Evaluate the product $(3\vec a - 5\vec b) \cdot (2\vec a + 7\vec b)$
Ans: $(3\vec a - 5\vec b) \cdot (2\vec a + 7\vec b)$
$ = 3\vec a \cdot 2\vec a + 3\vec a.7\vec b - 5\vec b \cdot 2\vec a - 5\vec b \cdot 7\vec b$
$ = 6\vec a\vec a + 21\vec a\vec b - 10\vec a\vec b - 35\vec b\vec b$
$ = 6|\vec a{|^2} + 11\vec a\vec b - 35|\vec b{|^2}$
8. Find the magnitude of two vectors $\vec a$ and $\vec b$, having the same magnitude and such that the angle between them is ${60^\circ }$ and their scalar product is $\dfrac{1}{2}$
Ans: Let $\theta $ be angle between $\vec a$ and $\vec b$.
$|\vec a| = |\vec b|,\vec a\;.\;\vec b = \dfrac{1}{2}$, and $\theta = {60^\circ }$
$\therefore \dfrac{1}{2} = |\vec a|\vec b\mid \cos {60^\circ }$
$ \Rightarrow \dfrac{1}{2} = |\vec a{|^2} \times \dfrac{1}{2}$
$ \Rightarrow |\vec a{|^2} = 1$
$ \Rightarrow |\vec a| = |\vec b| = 1$
9. Find $|\vec x|$, if for a unit vector $\vec a\;,\;(\vec x - \vec a) \cdot (\vec x + \vec a) = 12$
Ans: $(\vec x - \vec a) \cdot (\vec x + \vec a) = 12$
$ \Rightarrow \vec x \cdot \vec x + \vec x\vec a - \vec a\vec x - \vec a \cdot \vec a = 12$
$ \Rightarrow |\vec x{|^2} - |\vec a{|^2} = 12$
$ \Rightarrow |\vec x{|^2} - 1 = 12$
$ \Rightarrow |\vec x{|^2} = 13$
$\therefore |\vec x| = \sqrt {13} $
10. If $\vec a = 2\hat i + 2\hat j + 3\hat k,\vec b = - \hat i + 2\hat j + \hat k$ and $\vec c = 3\hat i + \hat j$ are such that $\vec a + \lambda \vec b$ is perpendicular to $\vec c$, then find the value of $\lambda $.
Ans: $\vec a + \lambda \vec b = (2\hat i + 2\hat j + 3\hat k) + \lambda ( - \hat i + 2\hat j + \dot k) = (2 - \lambda )\hat i + (2 + 2\lambda )\hat j + (3 + \lambda )\hat k$
$(\vec a + \lambda \vec b) \cdot \vec c = 0$
$ \Rightarrow [(2 + - )\hat i + (2 + 2\lambda )\hat j + (3 + \lambda )\hat k] \cdot (3\hat i + j) = 0$
$ \Rightarrow 3(2 - \lambda ) + (2 + 2\lambda ) + 0(3 + \lambda ) = 0$
$ \Rightarrow 6 - 3\lambda + 2 + 2\lambda = 0$
$ \Rightarrow - \lambda + 8 = 0$
$ \Rightarrow \lambda = 8$
11. Show that $|\vec a|\vec b + |\vec b|\vec a$ is perpendicular to $|\vec a|\vec b - |\vec b|\vec a$ , For any two nonzero vectors $\vec a$ and $\vec b$.
Ans: $(|\vec a|\vec b + |\vec b|\vec a) \cdot (|\vec a|\vec b - |\vec b|\vec a)$
$ = |\vec a{|^2}\vec b\vec b - |\vec a||\vec b|\vec b\vec a + |\overrightarrow b ||a|\vec a\vec b - |\vec b{|^2}\vec a \cdot \vec a$
$ = |\vec a{|^2}|\overrightarrow b {|^2} - |\overrightarrow b {|^2}|\overrightarrow a {|^2}\;\; = \;\;0$
12. If $\vec a \cdot \vec a = 0$ and $\vec a\vec b = 0$, then what can be concluded above the vector $\vec b$ ?
Ans: $\vec a \cdot \vec a = 0 \Rightarrow |\vec a{|^2} = 0 \Rightarrow |\vec a| = 0$
$\therefore \vec a$ is the zero vector. Thus, any vector $\vec b$ can satisfy $\vec a\;.\;\vec b = 0$.
13. If $\vec a,\vec b,\vec c$ are unit vectors such that $\vec a + \vec b + \vec c = 0$, find the value of $\vec a \cdot \vec b + \vec b \cdot \vec c + \vec c\;.\;\vec a$.
Ans: $|\vec a + \vec b + \vec c{|^2} = (\vec a + \vec b + \vec c) \cdot (\vec a + \vec b + \vec c) = |\vec a{|^2} + |\vec b{|^2} + |\vec c{|^2} + 2(\vec a\vec b + \vec b\vec c + \vec c \cdot \vec a)$
$ \Rightarrow 0 = 1 + 1 + 1 + 2(\vec a\vec b + \vec b \cdot \vec c + \vec c \cdot \vec a)$
$ \Rightarrow (\vec a\;.\;\vec b + \vec b \cdot \vec c + \vec c\;.\;\vec a) = \dfrac{{ - 3}}{2}$
14. If either vector $\vec a = \vec 0$ or $\vec b = \vec 0$, then $\vec a \cdot \vec b = 0.$ But the converse need not be true. Justify your answer with an example.
Ans: $\overrightarrow {\text{a}} = 2\widehat {\text{i}} + 4\widehat {\text{j}} + 3\widehat {\text{k}}$ and $\overrightarrow b = 3\widehat {\text{i}} + 3\widehat {\text{j}} - 6\widehat {\text{k}}$
$\vec a \cdot \vec b = 2.3 + 4.3 + 3( - 6) = 6 + 12 - 18 = 0$
$|{\text{a}}| = \sqrt {{2^2} + {4^2} + {3^2}} = \sqrt {29} $
$\therefore \vec a \ne \vec 0$
$|\vec b| = \sqrt {{3^2} + {3^2} + {{( - 6)}^2}} = 54$
$\therefore \vec b \ne \bar 0$
So, it is clear from the above example that the converse of the given statement need not be true.
15. If the vertices ${\text{A}},{\text{B}},{\text{C}}$ of a triangle ${\text{ABC}}$ are $(1,2,3),( - 1,0,0),(0,1,2)$, respectively, then find $\angle {\text{ABC}}$. $[\angle {\text{ABC}}$ is the angle between the vectors $\overrightarrow {{\text{BA}}} $ and $\overrightarrow {{\text{BC}}} $ ]
Ans: $\overrightarrow {{\text{BA}}} = \{ 1 - ( - 1)\hat i + (2 - 0)\hat j + (3 - 0)\hat k = 2\hat i + 2\hat j + 3\hat k$
$\overrightarrow {{\text{BC}}} = \{ 0 - ( - 1)\} \hat i + (1 - 0)\hat j + (2 - 0)\hat k = \hat i + \hat j + 2\hat k$
$\overrightarrow {{\text{BA}}} \cdot \overrightarrow {{\text{BC}}} = (2\hat i + 2\hat \jmath + 3\hat k) \cdot (\hat i + \hat \jmath + 2\hat k) = 2 \times 1 + 2 \times 1 + 3 \times 2 = 2 + 2 + 6 = 10$
$|\overrightarrow {{\text{BA}}} | = \sqrt {{2^2} + {2^2} + {3^2}} = \sqrt {4 + 4 + 9} = \sqrt {17} $
$|\overrightarrow {BC} | = \sqrt {1 + 1 + {2^2}} = \sqrt 6 $
$\therefore 10 = \sqrt {17} \times \sqrt 6 \cos (\angle {\text{ABC}})$
$ \Rightarrow \cos (\angle {\text{ABC}}) = \dfrac{{10}}{{\sqrt {17} \times \sqrt 6 }}$
$ \Rightarrow (\angle {\text{ABC}}) = {\cos ^{ - 1}}\left( {\dfrac{{10}}{{\sqrt {102} }}} \right)$
16. Show that the points ${\text{A}}(1,2,7),\;{\text{B}}(2,6,3)$ and ${\text{C}}(3,10, - 1)$ are collinear.
Ans: $\overrightarrow {{\text{AB}}} = (2 - 1)\hat i + (6 - 2)\hat j + (3 - 7)\hat k = \hat i + 4\hat j - 4\hat k$
$\overrightarrow {{\text{BC}}} = (3 - 2)\hat i + (10 - 6)\hat \jmath + ( - 1 - 3)\hat k = \hat i + 4\hat j - 4\hat k$
$\overrightarrow {{\text{AC}}} = (3 - 1)\hat i + (10 - 2)\hat j + ( - 1 - 7)\hat k = 2\hat i + 8\hat j - 8\hat k$
$|\overrightarrow {AB} | = \sqrt {{1^2} + {4^2} + {{( - 4)}^2}} = \sqrt {1 + 16 + 16} = \sqrt {33} $
$|\overrightarrow {BC} | = \sqrt {{1^2} + {4^2} + {{( - 4)}^2}} = \sqrt {1 + 16 + 16} = \sqrt {33} $
$|\overrightarrow {AC} | = \sqrt {{2^2} + {8^2} + {8^2}} = \sqrt {4 + 64 + 64} = 2\sqrt {33} $
$|\overrightarrow {{\text{AC}}} | = |\overrightarrow {{\text{AB}}} | + |\overrightarrow {{\text{BC}}} |$
Hence, the given points are collinear.
17. Show that the vectors $2\hat i - \hat j + \hat k\;,\;\hat i - 3\hat j - 5\hat k$ and $3\hat i - 4\hat j - 4\hat k$ form the vertices of a right angled triangle.
Ans: $\overrightarrow {{\text{OA}}} = 2\hat i - \hat j + \hat k\;,\;\overrightarrow {{\text{OB}}} = \hat i - 3\hat j - 5\hat k\;,\;\overrightarrow {{\text{OC}}} = 3\hat i - 4\hat j - 4\hat k$
$\overrightarrow {{\text{AB}}} = (1 - 2)\hat i + ( - 3 + 1)\hat j + ( - 5 - 1)\hat k = - \hat i - 2\hat j - 6 \hat k$
$\overrightarrow {{\text{BC}}} = (3 - 1)\hat i + ( - 4 + 3)\hat j + ( - 4 + 5)\hat k = 2\hat i - \hat j + \hat k$
$\overrightarrow {{\text{CA}}} = (2 - 3)\hat i + ( - 1 + 4)\hat j + (1 + 4)\hat k = - \hat i + 3\hat j + 5\hat k$
$|\overrightarrow {{\text{AB}}} | = \sqrt {{{( - 1)}^2} + {{( - 2)}^2} + {{( - 6)}^2}} = \sqrt {1 + 4 + 36} = \sqrt {41} $
$|\overrightarrow {BC} | = \sqrt {{2^2} + {{( - 1)}^2} + {1^2}} = \sqrt {4 + 1 + 1} = \sqrt 6 $
$|\overrightarrow {{\text{AC}}} | = \sqrt {{{( - 1)}^2} + {3^2} + {5^2}} = \sqrt {1 + 9 + 25} = \sqrt {35} $
$\therefore |\overrightarrow {BC} {|^2} + |\overrightarrow {AC} {|^2} = 6 + 35 = 41 = |\overrightarrow {AB} {|^2}$
Hence, $\Delta {\text{ABC}}$ is a right triangle.
18. If $\vec a$ is a nonzero vector of magnitude 'a' and $\lambda $ a nonzero scalar. then $\lambda \vec a$ is unit vector if
a. $\lambda = 1$
b. $\lambda = - 1$
c. $a = |\lambda |$
d. $a = \dfrac{1}{{|\lambda |}}$
Ans: $|\lambda \vec a| = 1$
$ \Rightarrow |\vec a| = \dfrac{1}{{|\lambda |}}$
$ \Rightarrow a = \dfrac{1}{{|\lambda |}}$
Exercise 10.4
1. Find $|\vec a \times \vec b|$, if $\vec a = \hat i - 7\hat j + 7\hat k$ and $\vec b = 3\hat i - 2\hat j + 2\hat k$
Ans: We have, $\vec a = \hat i - 7\hat j + 7\hat k$ and $\vec b = 3\hat i - 2\hat j + 2\hat k$
$\vec a \times \vec b = \left| {\begin{array}{*{20}{c}} {\hat i}&{\hat j}&{\hat k} \\ 1&{ - 7}&7 \\ 3&{ - 2}&2 \end{array}} \right|$
$ = \hat i( - 14 + 14) - \hat j(2 - 21) + \hat k( - 2 + 21) = 19\hat j + 19\hat k$
$\therefore |\vec a \times \vec b| = \sqrt {{{(19)}^2} + {{(19)}^2}} = \sqrt {2 \times {{(19)}^2}} = 19\sqrt 2 $
2. Find a unit vector perpendicular to each of the vector $\vec a + \vec b$ and $\vec a - \vec b$, where $\vec a = 3\hat i + 2\hat j + 2\hat k$ and $\vec b = \hat i + 2\hat j - 2\hat k$.
Ans: $\vec a = 3\hat i + 2\hat j + 2\hat k$ and $\vec b = \hat i + 2\hat j - 2\hat k$
$\vec a + \vec b = 4\hat i + 4\hat j\;,\;\vec a - \vec b = 2\hat i + 4\hat k$
$(\vec a + \vec b) \times (\vec a - \vec b) = \left| {\begin{array}{*{20}{c}} {\hat i}&{\hat j}&{\hat k} \\ 4&4&0 \\ 2&0&4 \end{array}} \right| = \hat i(16) - \hat j(16) + \hat k( - 8) = 16\hat i - 16\hat j - 8\hat k$
$|(\vec a + \vec b) \times (\vec a - \vec b)| = \sqrt {{{16}^2} + {{( - 16)}^2} + {{( - 8)}^2}} $
$ = \sqrt {{2^2} \times {8^2} + {2^2} \times {8^2} + {8^2}} $
$ = 8\sqrt {{2^2} + {2^2} + 1} = 8\sqrt 9 = 8 \times 3 = 24$
So, the unit vector is $ = \pm \dfrac{{(\vec a + \vec b) \times (\vec a - \vec b)}}{{|(\vec a + \vec b) \times (\vec a - \vec b)\mid }} = \pm \dfrac{{16\hat i - 16\hat j - 8\hat k}}{{24}}$
$ = \pm \dfrac{{2\hat i - 2\hat j - \hat k}}{3} = \pm \dfrac{2}{3}\hat i \mp \dfrac{2}{3}\hat j \mp \dfrac{1}{3}\hat k$
3. If a unit vector $\vec a$ makes an angle $\dfrac{\pi }{3}$ with $\hat i,\dfrac{\pi }{4}$ with $\hat j$ and an acute angle $\theta $ with $\hat k$, then find $\theta $ and hence, the components of $\vec a$
Ans: $\vec a = {a_1}\hat i + {a_2}\hat j + {a_3}\hat k$
$|\vec a|\; = 1$. $\cos \dfrac{\pi }{3} = \dfrac{{{a_1}}}{{|\vec a|}}$
$ \Rightarrow \dfrac{1}{2} = {a_1}$
$\cos \dfrac{\pi }{4} = \dfrac{{{a_2}}}{{|\vec a|}}$
$ \Rightarrow \dfrac{1}{{\sqrt 2 }} = {a_2}$
$\cos \theta = \dfrac{{{a_3}}}{{|\vec a|}}$
$ \Rightarrow {a_3} = \cos \theta $
$ \Rightarrow \sqrt {a_1^2 + a_2^2 + a_3^2} = 1$
$ \Rightarrow {\left( {\dfrac{1}{2}} \right)^2} + {\left( {\dfrac{1}{{\sqrt 2 }}} \right)^2} + {\cos ^2}\theta = 1$
$ \Rightarrow \dfrac{1}{4} + \dfrac{1}{2} + {\cos ^2}\theta = 1$
$ \Rightarrow \dfrac{3}{4} + {\cos ^2}\theta = 1$
$ \Rightarrow {\cos ^2}\theta = 1 - \dfrac{3}{4} = \dfrac{1}{4}$
$ \Rightarrow \cos \theta = \dfrac{1}{2} \Rightarrow \theta = \dfrac{\pi }{3}$
$\therefore {a_3} = \cos \dfrac{\pi }{3} = \dfrac{1}{2}$
So, $\theta = \dfrac{\pi }{3}$ and components of $\vec a$ are $\left( {\dfrac{1}{2},\dfrac{1}{{\sqrt 2 }},\dfrac{1}{2}} \right)$
4. Show that $(\vec a - \vec b) \times (\vec a + \vec b) = 2(\vec a \times \vec b)$
Ans: $(\vec a - \vec b) \times (\vec a + \vec b)$
$ = (a - b) \times \vec a + (a - b) \times b$
$ = a \times \vec a - \vec b \times \vec d + a \times \vec b - \vec b \times \vec b$
$ = 0 + \vec a \times \vec b + \vec a \times \vec b - 0$
$ = 2\;(\vec a \times \vec b)$
5. Find $\lambda $ and $\mu $ if $(2\hat i + 6\hat j + 27\hat k) \times (\hat i + \lambda \hat j + \mu \hat k) = \vec 0$
Ans: $(2\hat i + 6\hat j + 27\hat k) \times (\hat i + \lambda \hat j + \mu \hat k) = \vec 0$
$ \Rightarrow \left| {\begin{array}{*{20}{c}} {\hat i}&{\hat j}&{\hat k} \\ 2&6&{27} \\ 1&\lambda &\mu \end{array}} \right| = 0\hat i + 0\hat j + 0\hat k$
$ \Rightarrow \hat i(6\mu - 27\lambda ) - \hat j(2\mu - 27) + \hat k(2\lambda - 6) = 0\hat i + 0\hat j + 0\hat k$
$6\mu - 27\lambda = 0$
$2\mu - 27 = 0$
$2\lambda - 6 = 0$
$\lambda = 3$
$\mu = \dfrac{{27}}{2}$
6. Given that $\vec a\;.\;\vec b = 0$ and $\vec a \times \vec b = 0$. What can you conclude about $\vec a$ and $\vec b$ ?
Ans: $\vec a\;.\;\vec b = 0$
i. $|\vec a| = 0$ or $|\vec b| = 0$ or $\vec a \bot \vec b\quad ($ if $|\vec a| \ne 0$ and $|\vec b| \ne 0)$
$\vec a \times \vec b = 0$
ii. $|\overrightarrow a |\; = \;0\;{\text{or}}\;|\vec b|\; = \;0$ or (if $|\vec a| \ne 0$ and $|\vec b| \ne 0)$
But $\overrightarrow a $ and $\overrightarrow b $ cannot be parallel and perpendicular at same time.
So, $|\vec a| = 0$ or $|\vec b| = 0$
7. Let the vectors $\vec a,\vec b,\vec c$ given as ${a_1}\hat i + {a_2}\hat j + {a_3}\hat k\;,\;{b_1}\hat i + {b_2}\hat j + {b_3}\hat k\;,\;{c_1}\hat i + {c_2}\hat j + {c_3}\hat k$.
Then show that $ = \vec a \times (\vec b + \vec c) = \vec a \times \vec b + \vec a \times \vec c$
Ans: $(\vec b + \vec c) = \left( {{b_1} + {c_1}} \right)\hat i + \left( {{b_2} + {c_2}} \right)\hat j + \left( {{b_3} + {c_3}} \right)\hat k$
Now, $\vec a \times (\vec b + \vec c)\left| {\begin{array}{*{20}{c}} {\hat i}&{\hat j}&{\hat k} \\ {{a_1}}&{{a_2}}&{{a_3}} \\ {{b_1} + {c_1}}&{{b_2} + {c_2}}&{{b_3} + {c_3}} \end{array}} \right|$
$ = i\left[ {{a_2}\left( {{b_3} + {c_3}} \right) - {a_3}\left( {{b_2} + {c_2}} \right)} \right] - \hat j\left[ {{a_1}\left( {{b_3} + {c_3}} \right) - {a_3}\left( {{b_1} + {c_i}} \right)} \right] + \hat k\left[ {{a_1}\left( {{b_2} + {c_2}} \right) - {a_2}\left( {{b_1} + {c_1}} \right)} \right]$
$ = i\left[ {{a_2}{b_3} + {a_2}{c_3} - {a_3}{b_2} - {a_3}{c_2}} \right] + \hat j\left[ { - {a_1}{b_3} - {a_1}{c_3} + {a_3}{b_1} + {a_3}{c_1}} \right] + \hat k\left[ {{a_1}{b_2} + {a_1}{c_2} - {a_2}{b_1} - {a_2}{c_i}} \right]$
$\vec a \times \vec b = \left| {\begin{array}{*{20}{c}} {\hat i}&{\hat j}&{\hat k} \\ {{a_1}}&{{a_2}}&{{a_3}} \\ {{b_1}}&{{b_2}}&{{b_3}} \end{array}} \right|$
$ = i\left[ {{a_2}{b_3} - {a_3}{b_2}} \right] + \hat j\left[ {{b_1}{a_3} - a{b_3}} \right] + \hat k\left[ {{a_1}{b_2} - {a_2}h} \right]$
$\vec a \times \vec c = \left| {\begin{array}{*{20}{l}} {\hat i}&{\hat j}&{\hat k} \\ {{a_1}}&{{a_2}}&{{a_3}} \\ {{c_1}}&{{c_2}}&{{c_3}} \end{array}} \right|$
$ = \hat i\left[ {{a_2}{c_3} - {a_3}{c_2}} \right] + \hat j\left[ {{a_3}{c_1} - {a_1}{c_3}} \right] + \hat k\left[ {a{b_2} - {a_2}b} \right]$
$\left. {(\vec a \times \vec b) + (\vec a \times \vec c) = \hat i\left[ {{a_2}{b_3} + {a_2}{c_3} - {a_3}{b_2} - {a_3}{c_2}} \right] + \hat j{{[{b_1}{a_3} + {a_3}{c_1} - {a_1}{b_3} - {a_i}\} }_3}} \right]$
$ + \hat k\left[ {a,b, + {a_1}c, - a,{b_1} - a,{c_1}} \right]$
$\vec a \times (\vec b + \vec c) = \vec a \times \vec b + \vec a \times \vec c$
Hence, proved.
8. If either $\vec a = 0$ or $b = 0$, then $\vec a \times b = 0$.Is the converse true? Justify your answer with an example.
Ans: Let $\vec a = 2\hat i + 3\hat j + 4\hat k\;,\;\vec b = 4\hat i + 6\hat j + 8\hat k\;,\;\vec a \times \vec b = \vec 0$
$\vec a \times \vec b = \left| {\begin{array}{*{20}{l}} {\hat i}&{\hat j}&{\hat k} \\ 2&3&4 \\ 4&6&8 \end{array}} \right| = \hat i(24 - 24) - \hat j(16 - 16) + \hat k(12 - 12) = 0\hat i + 0\hat j + 0\hat k$
$|\vec a| = \sqrt {{2^2} + {3^2} + {4^2}} = \sqrt {29} $
$\therefore \vec a \ne \vec 0$
$|\vec b| = \sqrt {{4^2} + {6^2} + {8^2}} = \sqrt {116} $
$\therefore \vec b \ne \vec 0$
Hence, the converse of the statement need not be true.
9. Find the area of the triangle with vertices A $(1,1,2)$, B $(2,3,5)$ and $C(1,5,5)$.
Ans: $\overrightarrow {{\text{AB}}} = (2 - 1)\hat i + (3 - 1)\hat j + (5 - 2)\hat k = \hat i + 2\hat j + 3\hat k$
$\overrightarrow {{\text{BC}}} = (1 - 2)\hat i + (5 - 3)\hat j + (5 - 5)\hat k = - \hat i + 2\hat j$
Area $ = \dfrac{1}{2}|\overrightarrow {{\text{AB}}} \times \overrightarrow {{\text{BC}}} |$
$\overrightarrow {{\text{AB}}} \times \overrightarrow {{\text{BC}}} = \left| {\begin{array}{*{20}{l}} {\hat i}&{\hat j}&{\hat k} \\ 1&2&3 \\ { - 1}&2&0 \end{array}} \right| = \hat i( - 6) - \hat j(3) + \hat k(2 + 2) = - 6\hat i - 3\hat j + 4\hat k$
$|\overrightarrow {{\text{AB}}} \times \overrightarrow {{\text{BC}}} | = \sqrt {{{( - 6)}^2} + {{( - 3)}^2} + {4^2}} = \sqrt {36 + 9 + 16} = \sqrt {61} $
So, the area of $\Delta {\text{ABC}}$ is $\dfrac{{\sqrt {61} }}{2}$ sq units.
10. Find the area of the parallelogram whose adjacent sides are determined by the vector $\vec a = \hat i - \hat j + 3\hat k$ and $\overrightarrow {\text{b}} = 2\hat i - 7\hat j + \hat k$
Ans: $\vec a \times \overrightarrow {\text{b}} = \left| {\begin{array}{*{20}{c}} {\hat i}&{\hat j}&{\hat k} \\ 1&{ - 1}&3 \\ 2&{ - 7}&1 \end{array}} \right| = \hat i( - 1 + 21) - \hat j(1 - 6) + \hat k( - 7 + 2) = 20\hat i + 5\hat j - 5\hat k$
$\therefore |\vec a \times \vec b| = \sqrt {{{20}^2} + {5^2} + {5^2}} = \sqrt {400 + 25 + 25} = 15\sqrt 2 $
So, area of parallelogram is $15\sqrt 2 $ sq units
11. Let the vectors $\vec a$ and $\overrightarrow {\text{b}} $ be such that $|\vec a| = 3$ and $|\vec b| = \dfrac{{\sqrt 2 }}{3}$, then $\vec a \times \overrightarrow {\text{b}} $ is a unit vector, if the angle between $\vec a$ and $\vec b$is:
a. $\dfrac{\pi }{6}$
b. $\dfrac{\pi }{4}$
c. $\dfrac{\pi }{3}$
d. $\dfrac{\pi }{2}$
Ans:$|\vec a \times \vec b| = 1$
$ \Rightarrow ||a||\vec b|\sin \theta | = 1$
$ \Rightarrow |\vec a||\overrightarrow {\text{b}} ||\sin \theta | = 1$
$ \Rightarrow 3 \times \dfrac{{\sqrt 2 }}{3} \times \sin \theta = 1$
$ \Rightarrow \sin \theta = \dfrac{1}{{\sqrt 2 }}$
$ \Rightarrow \theta = \dfrac{\pi }{4}$
12. Area of a rectangle having vertices ${\text{A}},{\text{B}},{\text{C}}$, and ${\text{D}}$ with position vectors $ - \hat i + \dfrac{1}{2}\hat j + 4\hat k,\hat i + \dfrac{1}{2}\hat j + 4\hat k,\hat i - \dfrac{1}{2}\hat j + 4\hat k$ and $ - \hat i - \dfrac{1}{2}\hat j + 4\hat k$ respectively is
a. $\dfrac{1}{2}$
b. 1
c. 2
d. 4
Ans: $\overrightarrow {{\text{AB}}} = (1 + 1)\hat i + \left( {\dfrac{1}{2} - \dfrac{1}{2}} \right)\hat j + (4 - 4)\hat k = 2\hat i$
$\overrightarrow {{\text{BC}}} = (1 - 1)\hat i + \left( { - \dfrac{1}{2} - \dfrac{1}{2}} \right)\hat j + (4 - 4)\hat k = - \hat j$
$|\overrightarrow {{\text{AB}}} \times \overrightarrow {{\text{BC}}} | = \sqrt {{{( - 2)}^2}} = 2$
So, the area of the required rectangle is 2 square units.
Miscellaneous Exercise
1. Write down a unit vector in XY-plane, making an angle of ${30^\circ }$ with the positive direction of x axis.
Ans: Unit vector is $\vec r = \cos \theta \hat i + \sin \theta \hat j$, where $\theta $ is angle with positive ${\text{X}}$ axis. $\vec r = \cos {30^\circ }\hat i + \sin {30^\circ }\hat j = \dfrac{{\sqrt 3 }}{2}\hat i + \dfrac{1}{2}\hat j$
2. Find the scalar components and magnitude of the vector joining the points ${\text{P}}\left( {{x_1},{y_1},{z_1}} \right)$ and ${\text{Q}}\left( {{x_2},{y_2},{z_2}} \right)$
Ans: $\overrightarrow {{\text{PQ}}} = \left( {{x_2} - {x_1}} \right)\hat i + \left( {{y_2} - {y_1}} \right)\hat j + \left( {{z_2} - {z_1}} \right)\hat k$
$|\overrightarrow {{\text{PQ}}} |\; = \;\sqrt {{{\left( {{x_2} - {x_1}} \right)}^2} + {{\left( {{y_2} - {y_1}} \right)}^2} + {{\left( {{z_2} - {z_1}} \right)}^2}} $
3. A girl walks 4 km towards west, then she walks 3 km in a direction ${30^\circ }$ east of north and stops. Determine the girl's displacement from her initial point of departure.
Ans:
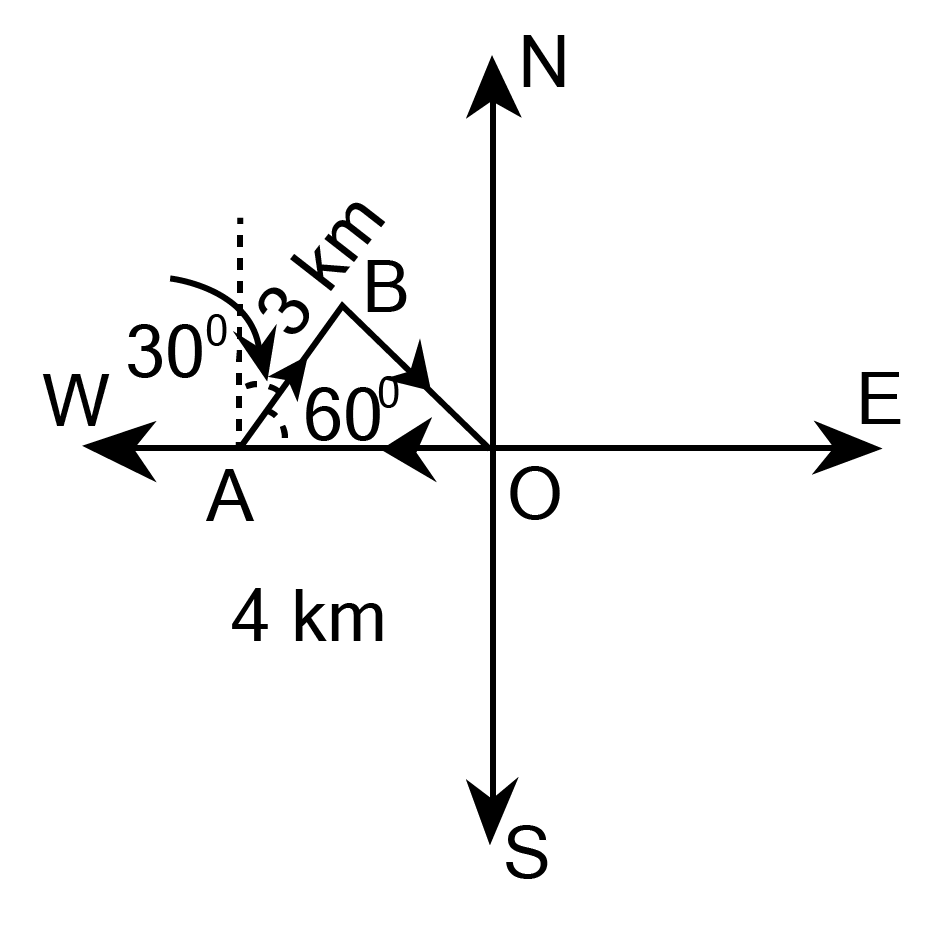
$\overrightarrow {{\text{OA}}} = - 4\hat i$
$\overrightarrow {{\text{AB}}} = \hat i|\overrightarrow {{\text{AB}}} |\cos {60^\circ } + \hat j|\overrightarrow {{\text{AB}}} |\sin {60^\circ }$
$ = \hat i3 \times \dfrac{1}{2} + \hat j3 \times \dfrac{{\sqrt 3 }}{2}$
$ = \dfrac{3}{2}\hat i + \dfrac{{3\sqrt 3 }}{2}\hat j$
$\overrightarrow {{\text{OB}}} = \overrightarrow {{\text{OA}}} + \overrightarrow {{\text{AB}}} $
$ = ( - 4\hat i) + \left( {\dfrac{3}{2}\hat i + \dfrac{{3\sqrt 3 }}{2}j} \right)$
$ = \left( { - 4 + \dfrac{3}{2}} \right)\hat i + \dfrac{{3\sqrt 3 }}{2}\hat j$
$ = \left( {\dfrac{{ - 8 + 3}}{9}} \right)\hat i + \dfrac{{3\sqrt 3 }}{9}\hat j$
$ = \dfrac{{ - 5}}{2}\vec i + \dfrac{{3\sqrt 3 }}{2}\hat j$
4. If $\overrightarrow a = \vec b + \vec c$, then is it true that $|\overrightarrow a | = |\vec b| + |\vec c|$ ? Justify your answer.
Ans: $\operatorname{In} \Delta {\text{ABC}},\overrightarrow {\;CB} = \vec a,\;\overrightarrow {CA} = b,\;\overrightarrow {AB} = \vec c$
$\vec a = \vec b + \vec c$, by triangle law of addition for vectors.
$|\vec a|\; < \;|\vec b| + |\vec c|$, by triangle inequality law of lengths.
Hence, it's not true that $|\vec a| = |\vec b| + |\vec c|$
5. Find the value of $x$ for which $x(\hat i + \hat j + \hat k)$ unit vector.
Ans: $|x(\hat i + \hat j + \hat k)| = 1$
$ \Rightarrow \sqrt {{x^2} + {x^2} + {x^2}} = 1$
$ \Rightarrow \sqrt {3{x^2}} = 1$
$ \Rightarrow \sqrt 3 x = 1$
$ \Rightarrow x = \pm \dfrac{1}{{\sqrt 3 }}$
6. Find a vector of magnitude 5 units, and parallel to the resultant of the vectors $\vec a = 2\hat i + 3\hat j - \hat k$ and $\vec b = \hat i - 2\hat j + \hat k$
Ans: $\vec c = \vec a + \vec b = (2 + 1)\hat i + (3 - 2)\hat j + ( - 1 + 1)\hat k = 3\hat i + \hat j$
$|\vec c| = \sqrt {{3^2} + {1^2}} = \sqrt {9 + 1} = \sqrt {10} $
$\therefore \hat c = \dfrac{{\vec c}}{{|\vec c|}} = \dfrac{{(3\hat i + \hat j)}}{{\sqrt {10} }}$
So, a vector of magnitude 5 and parallel to the resultant of $\vec a$ and $\vec b$ is $ \pm 5(\hat c) = \pm 5\left( {\dfrac{1}{{\sqrt {10} }}(3\hat i + \hat j)} \right) = \pm \dfrac{{3\sqrt {10} }}{2}\hat i \pm \dfrac{{\sqrt {10} }}{2}\hat j$
7. If $\vec a = \hat i + \hat j + \hat k\;,\;\vec b = 2\hat i - \hat j + 3\hat k$ and $\vec c = \hat i - 2\hat j + \hat k$, find a unit vector parallel to the vector $2\vec a - \vec b + 3\vec c$.
Ans: $2\vec a - \vec b + 3\vec c = 2(\hat i + \hat j + \hat k) - (2\hat i - \hat j + 3\hat k) + 3(\hat i - 2\hat j + \hat k)$
$ = 2\hat i + 2\hat j + 2\hat k - 2\hat i + j - 3\vec k + 3\hat i - 6\hat j + 3\hat k$
$ = 3\hat i - 3\hat j + 2\hat k$
$|2\vec a - \vec b + 3c| = \sqrt {{3^2} + {{( - 3)}^2} + {2^2}} = \sqrt {9 + 9 + 4} = \sqrt {22} $
Thus ,required unit vector is $\dfrac{{2\vec a - \vec b + 3\vec c}}{{|2\vec a - \vec b + 3\vec c|}} = \dfrac{{3\hat i - 3\hat j + 2\hat k}}{{\sqrt {22} }} = \dfrac{3}{{\sqrt {22} }}\hat i - \dfrac{3}{{\sqrt {22} }}\hat j + \dfrac{2}{{\sqrt {22} }}\hat k$
8. Show that the points ${\text{A}}(1, - 2, - 8),{\text{B}}(5,0, - 2)$ and ${\text{C}}(11,3,7)$ are collinear, and find the ratio in which B divides Ac.
Ans: $\overrightarrow {{\text{AB}}} = (5 - 1)\hat i + (0 + 2)\hat j + ( - 2 + 8)\hat k = 4\hat i + 2\hat j + 6\hat k$
$\overrightarrow {{\text{BC}}} = (11 - 5)\hat i + (3 - 0)\hat j + (7 + 2)\hat k = 6\hat i + 3\hat j + 9\hat k$
$\overrightarrow {AC} = (11 - 1)\hat i + (3 + 2)\hat j + (7 + 8)\hat k = 10\hat i + 5\hat j + 15\hat k$
$|\overrightarrow {{\text{AB}}} | = \sqrt {{4^2} + {2^2} + {6^2}} = \sqrt {16 + 4 + 36} = \sqrt {56} = 2\sqrt {14} $
$|\overrightarrow {{\text{BC}}} | = \sqrt {{6^2} + {3^2} + {9^2}} = \sqrt {36 + 9 + 81} = \sqrt {126} = 3\sqrt {14} $
$|\overrightarrow {AC} | = \sqrt {{{10}^2} + {5^2} + {{15}^2}} = \sqrt {100 + 25 + 225} = \sqrt {350} = 5\sqrt {14} $
$\therefore \;\;|\overrightarrow {{\text{AC}}} | = |\overrightarrow {{\text{AB}}} | + |\overrightarrow {{\text{BC}}} |$
So, the points are collinear.
Let B divide AC in ratio $\lambda :1$. $\overrightarrow {{\text{OB}}} = \dfrac{{\lambda \overrightarrow {{\text{OC}}} + \overrightarrow {{\text{OA}}} }}{{(\lambda + 1)}}$
$ \Rightarrow 5\hat i - 2\hat k = \dfrac{{\lambda (11\hat i + 3\hat j + 7\hat k) + (\hat i - 2\hat j - 8\hat k)}}{{\lambda + 1}}$
$ \Rightarrow (\lambda + 1)(5\hat i - 2\hat k) = 11\lambda \hat i + 3\lambda \hat j + 7\lambda \hat k + \hat i - 2\hat j - 8\hat k$
$ \Rightarrow 5(\lambda + 1)\hat i - 2(\lambda + 1)\hat k = (11\lambda + 1)\hat i + (3\lambda - 2)\hat j + (7\lambda - 8)\hat k$
$ \Rightarrow \lambda = \dfrac{2}{3}$
So, the required ratio is 2:3
9. Find the position vector of a point $R$ which divides the line joining two points $P$ and $Q$ whose position vectors are $(2\vec a + \vec b)$ and $(\vec a - 3\vec b)$ externally in the ratio 1: 2. Also, show that $P$ is the mid point of the line segment RQ.
Ans: $\overrightarrow {{\text{OP}}} = 2\vec a + \vec b,\overrightarrow {{\text{OQ}}} = \overrightarrow {\text{a}} - 3\overrightarrow {\text{b}} $
$\overrightarrow {OR} = \dfrac{{2(2\vec a + \vec b) - (\vec a - 3\vec b)}}{{2 - 1}} = \dfrac{{4\vec a + 2\vec b - \vec a - 3\vec b}}{1} = 3\vec a + 5\vec b$
So, the position vector of ${\text{R}}$ is $3\overrightarrow {\text{a}} + 5\overrightarrow {\text{b}} $
Position vector of midpoint of ${\text{RQ}} = \dfrac{{\overrightarrow {{\text{OQ}}} + \overrightarrow {{\text{OR}}} }}{2}$
$ = \dfrac{{(a\sqrt 6 ) + (3\vec a + 5\bar b)}}{2}$
$ = 2\vec a + \vec b$
$ = \overrightarrow {OP} $
Thus, ${\text{P}}$ is midpoint of line segment ${\text{RQ}}$
10. The two adjacent sides of a parallelogram are $2\hat i - 4\hat j + 5\hat k$ and $\hat i - 2\hat j - 3\hat k$. Find the unit vector parallel to its diagonal. Also, find its area.
Ans: Diagonal of a parallelogram is $\vec a + \vec b$
$\vec a + \vec b = (2 + 1)\hat i + ( - 4 - 2)\hat j + (5 - 3)\hat k = 3\hat i - 6\hat j + 2\hat k$
So, the unit vector parallel to diagonal is $\dfrac{{\vec a + \vec b}}{{|\vec a + \vec b|}} = \dfrac{{3\hat i - 6\hat j + 2\hat k}}{{\sqrt {{3^2} + {{( - 6)}^2} + {2^2}} }} = \dfrac{{3\hat i - 6\hat j + 2\hat k}}{{\sqrt {9 + 36 + 4} }} = \dfrac{{3\hat i - 6\hat j + 2\hat k}}{7} = \dfrac{3}{7}\hat i - \dfrac{6}{7}\hat j + \dfrac{2}{7}\hat k$
$\vec a \times \vec b = \left| {\begin{array}{*{20}{r}} {\hat i}&{\hat j}&k \\ 2&{ - 4}&3 \\ 1&{ - 2}&{ - 3} \end{array}} \right|$
$ = \hat i(12 + 10) - \hat j( - 6 - 5) + \bar k( - 4 + 4)$
$ = 22\hat i + 11\hat j$
$ = 11(2\hat i + \hat j)$
$\therefore |\vec a \times \vec b| = 11\sqrt {{2^2} + {1^2}} = 11\sqrt 5 $
So, area of parallelogram is $11\sqrt 5 $ sq units
11. Show that the direction cosines of a vector equally inclined to the axes OX, OY and OZ are $\dfrac{1}{{\sqrt 3 }}$ $\dfrac{1}{{\sqrt 3 }},\dfrac{1}{{\sqrt 3 }}$.
Ans: Let a vector be equally inclined to ${\text{OX}},{\text{OY}}$, and ${\text{OZ}}$ at an angle $\alpha $.
So, the Direction Cosines of the vector are $\cos \alpha ,\cos \alpha $and $\cos \alpha $.
${\cos ^2}\alpha + {\cos ^2}\alpha + {\cos ^2}\alpha = 1$
$ \Rightarrow 3{\cos ^2}\alpha = 1$
$ \Rightarrow \cos \alpha = \dfrac{1}{{\sqrt 3 }}$
So, the DCs of the vector are $\dfrac{1}{{\sqrt 3 }},\dfrac{1}{{\sqrt 3 }},\dfrac{1}{{\sqrt 3 }}$.
12. Let $\vec a = \hat i + 4\hat j + 2\hat k$ and $\vec b = 3\hat i - 2\hat j + 7\hat k$ and $\vec c = 2\hat i - \hat j + 4\hat k$. Find a vector $\vec d$ which is
perpendicular to both $\vec a$ and $\vec b$ and $\vec c \cdot \vec d = 15$
Ans: $\vec d = {d_1}\hat i + {d_2}\hat \jmath + {d_3}\hat k$
$\vec d\;.\;\vec a = 0 \Rightarrow {d_1} + 4{d_2} + 2{d_3} = 0$
$\vec d\;.\;\vec b = 0 \Rightarrow 3{d_1} - 2{d_2} + 7{d_3} = 0$
$\vec c \cdot \vec d = 15 \Rightarrow 2{d_1} - {d_2} + 4{d_3} = 15$
Solving these equations, we get ${d_1} = \dfrac{{160}}{3},{d_2} = - \dfrac{5}{3},{d_3} = - \dfrac{{70}}{3}$
$\therefore \vec d = \dfrac{{160}}{3}\hat i + \dfrac{5}{3}\widehat j + \dfrac{{70}}{3}\hat k = \dfrac{1}{3}(160\hat i + 5\hat j + 70\hat k)$
13. The scalar product of the vector $\hat i + \hat j + \hat k$ with a unit vector along the sum of vectors $2\hat i + 4\hat j - 5\hat k$ änd $\lambda \hat i + 2\hat j + 3\hat k$ is equal to one. Find the value of $\lambda $.
Ans: $(2\hat i + 4\hat j - 5\hat k) + (\lambda \hat i + 2\hat j + 3\hat k) = (2 + \lambda )\hat i + 6\hat j - 2\hat k$
So, unit vector along $(2\hat i + 4\hat j - 5\hat k) + (\lambda \hat i + 2\hat j + 3\hat k)$ is $\left( {\dfrac{{(2 + \lambda )\hat i + 6\hat j - 2\hat k}}{{\sqrt {{\lambda ^2} + 4\lambda + 44} }}} \right)$
$(\hat i + \hat j + \hat k) \cdot \left( {\dfrac{{(2 + \lambda )\hat i + 6\hat j - 2\hat k}}{{\sqrt {{\lambda ^2} + 4\lambda + 44} }}} \right) = 1$
$ \Rightarrow \dfrac{{(2 + \lambda ) + 6 - 2}}{{\sqrt {{\lambda ^2} + 4\lambda + 44} }} = 1$
$ \Rightarrow \sqrt {{\lambda ^2} + 4\lambda + 44} = \lambda + 6$
$ \Rightarrow {\lambda ^2} + 4\lambda + 44 = {(\lambda + 6)^2}$
$ \Rightarrow {\lambda ^2} + 4\lambda + 44 = {\lambda ^2} + 12\lambda + 36$
$ \Rightarrow 8\lambda = 8$
$ \Rightarrow \lambda = 1$
14. If $\vec a,\vec b,\vec c$ are mutually perpendicular vectors of equal magnitudes, show that the vector $\vec a + \vec b + \vec c$ is equally inclined to $\vec a\;,\;\vec b$ and $\vec c$.
Ans: $\vec a\;.\;\vec b = \vec b\;.\;\vec c = \vec c\;.\;\vec a = 0$
$|\vec a| = |\vec b| = |\vec c|$
Let $\vec a + \vec b + \vec c$ be inclined to $\vec a,\vec b,\vec c$ at angles ${\theta _1},{\theta _2},{\theta _3}$ respectively. $\cos {\theta _1} = \dfrac{{(\vec a + \vec b + \vec c) \cdot \vec a}}{{|\vec a + \vec b + \vec c||\vec a\mid }} = \dfrac{{\vec a \cdot \vec a + \vec b \cdot \vec a + \vec c \cdot \vec a}}{{|\vec a + \vec b + \vec c||\vec a\mid }} = \dfrac{{|\vec a{|^2}}}{{|\vec a + \vec b + \vec c||\vec a|}} = \dfrac{{|\vec a|}}{{|\vec a + \vec b + \vec c|}}$
$\cos {\theta _2} = \dfrac{{(\vec a + \vec b + \vec c)\vec b}}{{|\vec a + \vec b + \vec c||\vec b\mid }} = \dfrac{{\vec a\vec b + \vec b\vec b + \vec c\vec b}}{{|\vec a + \vec b + \vec c||\vec b\mid }} = \dfrac{{|\vec b{|^2}}}{{|\vec a + \vec b + \vec c||\vec b|}} = \dfrac{{|\vec b|}}{{|\vec a + \vec b + \vec c|}}$
$\operatorname{os} {\theta _3} = \dfrac{{(\vec a + \vec b + \vec c) \cdot \vec c}}{{|\vec a + \vec b + \vec c||\bar c|}} = \dfrac{{\vec a\vec c + \vec b\vec c + \vec c\vec c}}{{|\vec a + \vec b + \vec c||\vec c\mid }} = \dfrac{{|\vec c{|^2}}}{{|\vec a + \vec b + \vec c||\vec c|}} = \dfrac{{|\vec c|}}{{|\vec a + \vec b + \vec c|}}$
Since, $|\vec a| = |\vec b| = |\vec c|\; \Rightarrow \;\cos {\theta _1} = \cos {\theta _2} = \cos {\theta _3}$, So, ${\theta _1} = {\theta _2} = {\theta _3}$
15. Prove that, $(\vec a + \vec b) \cdot (\vec a + \vec b) = \;|\vec a{|^2} + |\vec b{|^2}$ if and only if $\vec a,\vec b$ are perpendicular, given $\vec a \ne \vec 0,\vec b \ne 0$
Ans: $(\vec a + \vec b) \cdot (\vec a + \vec b) = |\vec a{|^2} + |\vec b{|^2}$
$ \Rightarrow \vec a \cdot \vec a + \vec a\;.\;\vec b + \vec b \cdot \vec a + \vec b\;.\;\vec b = |\vec a{|^2} + |\vec b{|^2}$
$ \Rightarrow |\vec a{|^2} + 2\vec a\;.\;\vec b + |\vec b{|^2} = |a{|^2} + |\vec b{|^2}$
$ \Rightarrow 2\vec a\;.\;\vec b = 0$
$ \Rightarrow \vec a\;.\;\vec b = 0$
So $\vec a$ and $\vec b$ are perpendicular.
16. If $\theta $ is the angle between two vectors $\vec a$ and $\vec b$, then $\vec a\vec b \geqslant 0$ only when
a. $0 < \theta < \dfrac{\pi }{2}$
b. $0 \leqslant \theta \leqslant \dfrac{\pi }{2}$
c. $0 < \theta < \pi $
d. $0 \leqslant \theta \leqslant \pi $
Ans: $\therefore \vec a\vec b \geqslant 0$
$ \Rightarrow |\vec a||\vec b|\cos \theta \geqslant 0$
$ \Rightarrow \cos \theta \geqslant 0\quad \because [|\vec a| \geqslant 0$ and $|\vec b| \geqslant 0]$
$ \Rightarrow 0 \leqslant \theta \leqslant \dfrac{\pi }{2}$
$\vec a \cdot \vec b \geqslant 0$ if $0 \leqslant \theta \leqslant \dfrac{\pi }{2}$
So the right answer is B
17. Let $\vec a$ and $\vec b$ be two unit vectors and $\theta $ is the angle between them. Then $\vec a + \vec b$ is a unit vector if
a. $\theta = \dfrac{\pi }{4}$
b. $\theta = \dfrac{\pi }{3}$
c. $\theta = \dfrac{\pi }{2}$
d. $\theta = \dfrac{{2\pi }}{3}$
Ans: $|\vec a| = |\vec b| = 1$
$|\vec a + \vec b| = 1$
$ \Rightarrow (\vec a + \overrightarrow b )(\vec a + \overrightarrow b ) = 1$
$ \Rightarrow \vec a \cdot \vec a + \vec a\;.\;\vec b + \vec b\;.\;\vec a + \vec b\;.\;\vec b = 1$
$ \Rightarrow |\vec a{|^2} + 2\vec a\;.\;\vec b + |\overrightarrow b {|^2} = 1$
$ \Rightarrow {1^2} + 2|\vec a||\vec b|\cos \theta + {1^2} = 1$
$ \Rightarrow 1 + 2.1.1\cos \theta + 1 = 1$
$ \Rightarrow \cos \theta = - \dfrac{1}{2}$
$ \Rightarrow \theta = \dfrac{{2\pi }}{3}$
So, $\vec a + \vec b$ is unit vector if $\theta = \dfrac{{2\pi }}{3}$
The correct answer is D
18. The value of $\hat i.(\hat j \times \hat k) + \hat j \cdot (\hat i \times \hat k) + \hat k \cdot (\hat i \times \hat j)$ is
$\begin{array}{*{20}{l}} {{\text{ a. }}0}&{{\text{ b. }} - 1}&{{\text{ c. }}1}&{{\text{ d. }}3} \end{array}$
Ans:
$\hat i \cdot (\hat j \times \hat k) + \hat j \cdot (\hat i \times \hat k) + \hat k \cdot (\hat i \times \hat j)$
$ = \hat i\;.\;\hat i + \hat j \cdot ( - \hat j) + \hat k\;.\;\hat k$
$ = 1 - 1 + 1$
$ = 1$
The correct answer is C.
19. If $\theta $ is the angle between any two vectors $\vec a$ and $\vec b$, then $|\vec a\vec b| = |\vec a \times \vec b|$ when $\theta $ is equal to
a. 0
b. $\dfrac{\pi }{4}$
c. $\dfrac{\pi }{2}$
d. n
Ans: $|\vec a\vec b| = |\vec a \times \vec b|$
$ \Rightarrow |\vec a|\vec b|\cos \theta = |\vec a||\vec b\mid \sin \theta $
$ \Rightarrow \cos \theta = \sin \theta $
$ \Rightarrow \tan \theta = 1$
$ \Rightarrow \theta = \dfrac{\pi }{4}$
The correct answer is B
Overview of Deleted Syllabus for CBSE Class 12 Maths Vector Algebra
Chapter | Dropped Topics |
Vector Algebra | 10.7 - Scalar Triple Product |
10.7.1 - Coplanarity of Three Vectors |
Class 12 Maths Chapter 10: Exercises Breakdown
Exercise | Number of Questions |
Exercise 10.1 | 5 Questions & Solutions (5 Short Answers) |
Exercise 10.2 | 19 Questions & Solutions (5 Short Answers, 14 Long Answers) |
Exercise 10.3 | 18 Questions & Solutions (5 Short Answers, 13 Long Answers) |
Exercise 10.4 | 12 Questions & Solutions (4 Short Answers, 8 Long Answers) |
Miscellaneous Exercise | 19 Questions & Solutions |
Conclusion
Vector Class 12 NCERT Solutions provides a comprehensive understanding of the fundamental concepts and applications of vectors. This chapter covers various essential topics such as vector addition, subtraction, scalar multiplication, dot product, cross product, and their geometric interpretations. Through well-structured examples and step-by-step solutions, students gain a solid foundation in vector algebra, which is crucial for higher studies in mathematics, physics, and engineering. The exercises are designed to enhance problem-solving skills and analytical thinking. Typically 1 to 2 short answer questions and 1 to 2 long answer questions appear from Ch 10 Maths Class 12 in exams.
Other Study Material for CBSE Class 12 Maths Chapter 10
S.No. | Important Links for Chapter 10 Vector Algebra |
1 | |
2 | |
3 | |
4 | |
5 |
NCERT Solutions for Class 12 Maths | Chapter-wise List
Given below are the chapter-wise NCERT 12 Maths solutions PDF. Using these chapter-wise class 12th maths ncert solutions, you can get clear understanding of the concepts from all chapters.
S.No. | NCERT Solutions Class 12 Maths Chapter-wise List |
1 | |
2 | |
3 | |
4 | |
5 | |
6 | |
7 | |
8 | |
9 | |
10 | |
11 | |
12 |
Related Links for NCERT Class 12 Maths in Hindi
Explore these essential links for NCERT Class 12 Maths in Hindi, providing detailed solutions, explanations, and study resources to help students excel in their mathematics exams.
S.No. | Related NCERT Solutions for Class 12 Maths |
1 | |
2 |
Important Related Links for NCERT Class 12 Maths
S.No | Important Resources Links for Class 12 Maths |
1 | |
2 | |
3 | |
4 | |
5 | |
6 | |
7 | |
8 | |
9 | |
10 |
FAQs on NCERT Solutions for Class 12 Maths Chapter 10 Vector Algebra
1. What are the major topics of Class 12 Maths Chapter 10 as per the latest NCERT syllabus?
As per the latest syllabus of CBSE Class 12 Mathematics, following are some of the major topics covered in Chapter 10 Vector Algebra:
1. Introduction
2. Basic Concepts
Position Vector
Direction Cosines
3. Types of Vectors
Zero Vector
Unit Vector
Coinitial Vectors
Collinear Vectors
Equal Vectors
Negative of a Vector
4. Addition of Vectors
Properties of vector addition
5. Multiplication of a Vector by a Scalar
Components of a vector
Vector joining two points
Section formula
6. Product of Two Vectors
Scalar or dot product of two vectors
Projection of a vector on a line
Vector or cross product of two vectors
2. What is the difference between scalar and vector?
The main difference between a vector and a scalar quantity is that a vector quantity has a direction and a magnitude while a scalar has only magnitude. If a quantity has a direction associated with it, it is a vector quantity. However, the scalar quantity is not associated with the direction. Speed is a scalar quantity but velocity is a vector quantity as it specifies both a direction as well as a magnitude. In mathematical terms, a vector is an element of a vector space. Mathematically, we can demonstrate a vector as a directed line segment.
3. What are the important takeaways for a student from the 10th chapter of NCERT Class 12 Mathematics?
Students will understand how to differentiate between a scalar and vector quantity with the help of Class 12 Maths Chapter 10 Vector Algebra. The important things that a student will learn from this chapter are the magnitude and direction of a vector, direction cosines and direction ratios of a vector, types of vectors (equal, unit, zero, parallel and collinear vectors), position vector of a point, negative of a vector, components of a vector, etc. Students will also be able to perform addition of vectors, multiplication of a vector by a scalar and position vector of a point dividing a line segment in a given ratio. Students will get familiarized with the definition of Vectors, geometrical interpretation of vectors, properties and application of scalar (dot) product of vectors, vector (cross) product of vectors, the scalar triple product of vectors, etc.
4. Why should I refer to NCERT Solutions for Class 12 Maths Chapter 10 Vector Algebra?
Vector Algebra is an important branch of mathematics that deals with quantities having directions. The concepts of vector algebra are also useful in various topics of physics. Hence, students must pay attention to the chapter and solve all the problems given in the exercise. Students facing any doubt in the chapter can refer to NCERT Solutions for Class 12 Maths Chapter 10 Vector Algebra by Vedantu. These solutions include step-by-step answers to textbook questions. The expert in-house teachers at Vedantu provide the solutions. Hence, students can rely on them for exam preparation and revision. Students can perform better in exams with the help of NCERT Solutions as it will provide a better understanding of the chapter which is crucial for conceptual chapters like Vector Algebra.
5. What is vector algebra?
Vector algebra is one of the important topics of algebra. It deals with the algebraic expressions of vector quantities. Vector algebra is an algebra where important symbols, letters, numbers and variables denote vector algebraic expressions. In Mathematics, algebraic operations are performed on vector spaces and vectors. This branch of mathematics has hypotheses and rules based on the behaviour and properties of vectors. On Vedantu, you can understand the various concepts of the chapter with some solved examples.
6. What are the different types of vectors?
There are six types of vectors. These are-
Zero vector- This type of vector always has a zero magnitude.
Unit vector- A vector whose magnitude is always unity or one. It is denoted by a vector that is equal to the direction of the AB vector.
Coinitial vector- The vectors that have the same initial points.
Parallel or collinear vector- Two or more vectors are said to be collinear if they are parallel to the same line regardless of their directions and magnitudes.
Equal vector- The vectors that have the same direction and magnitude irrespective of the position of their initial points.
Negative of a vector- Vectors that have similar magnitude but have opposite directions.
7. What are the observations of the scalar product of two vectors?
The observations of the scalar product of two vectors are-
The dot product of two vectors is a real number.
If a and b vectors are non-zero vectors then, its dot product is zero only if a and b vectors are perpendicular to each other.
If the angle between a and b vectors is zero then, its dot product is equal to the magnitude of the individual product of the vectors.
If the angle between a and b vectors is 180 degrees then, its dot product is equal to the magnitude of the individual product of the vectors but having an opposite direction.
8. What are the observations of the cross product of two vectors?
The observations of the cross product of two vectors are as follows-
The cross product of two vectors is always a vector quantity.
If a and b vectors are not equal to zero vectors then, their cross product is equal to the product of the magnitude of individual vectors, sin angle between the two vectors and the normal vector.
If the angle between two vectors, a and b is 90 degrees then sin 90 is equal to 1. Therefore, in this case the vector product is equal to the product of the magnitude of individual vectors and the normal vector.
9. How can students receive decent marks in Chapter 10 of Class 12 Maths?
Chapter 10 Vector Algebra is one of the important chapters of Class 12 Maths. Students must learn this chapter very well as it is crucial from the board exam point of view. So, to get good marks in this chapter students have to follow the given tips.
Class 12 students have to take their classes seriously.
They should make notes for this chapter for doing a proper revision.
Students must practise the NCERT Solutions of Chapter 10 Vector Algebra to know the type of questions asked from this chapter in the exams.


























