NCERT Solutions for Maths Chapter 8 Application of Integrals Class 12 - FREE PDF Download
NCERT Solutions for CBSE Maths Chapter 8 Application of Integrals Class 12 are available in Vedantu. These NCERT Solutions are created as per the latest syllabus of NCERT Maths for Class 12. This PDF covers solutions for all questions that are covered in the CBSE Class 12 Maths textbook Chapter 8. All the solutions are explained in a step by step manner. Students can refer to these solutions to learn the important questions and prepare for their board exams. The NCERT Solutions for CBSE Chapter 8 Class 12 Maths are available in a PDF format and can be downloaded for free.
- 5.1Exercise 8.1
- 5.2Miscellaneous Exercise
Glance on Maths Chapter 8 Class 12 - Application of Integrals
Application of Integrals Class 12 NCERT Solutions deals with finding the area between curves, such as between a curve and the x-axis, or between two curves using definite integrals. It includes step-by-step solutions for different types of curves.
Problems in this Chapter focus on calculating the area enclosed by two given curves. Solutions illustrate the method of setting up integrals with appropriate limits and finding the difference between the two areas.
Comprehensive solutions to all exercise problems in the NCERT textbook are given. This includes detailed explanations, diagrams, and the rationale behind each step to ensure thorough understanding.
There is one exercise and a miscellaneous exercise (9 fully solved questions) in Class 12th maths chapter 8 Application of Integrals.
This article contains chapter notes, important questions, exemplar solutions, exercises, and video links for Chapter 8 - Application of Integrals, which you can download as PDFs.
Access Exercise Wise NCERT Solutions for Chapter 8 Maths Class 12
Exercise 8.1: This exercise teaches students how to find the area of a region bounded by curves using integration.
Miscellaneous Exercise: This exercise contains a collection of problems that require students to apply the concepts they have learned in the previous exercises to solve real-world problems.
Access NCERT Solutions for Class 12 Maths Chapter 8 – Application of Integrals
Exercise 8.1
1. Find the area of the region bounded by the ellipse \[\frac{{{\text{x}}^{\text{2}}}}{\text{16}}\text{+}\frac{{{\text{y}}^{\text{2}}}}{\text{9}}\text{=1}\].
Ans:
Area Bounded by Ellipse
Area of ellipse \[\text{=4 }\!\!\times\!\!\text{ }\] Area of \[\text{OAB}\]
Area of \[\text{OAB=}\int_{\text{0}}^{\text{4}}{\text{y}}\text{dx}\]
Substitute $y=3\sqrt{1-\frac{{{x}^{2}}}{16}}$
\[\text{=}\int_{\text{0}}^{\text{4}}{\text{3}}\sqrt{\text{1-}\frac{{{\text{x}}^{\text{2}}}}{\text{16}}}\text{dx}\]
simplifying,
\[\text{=}\frac{\text{3}}{\text{4}}\int_{\text{0}}^{\text{4}}{\sqrt{\text{16-}{{\text{x}}^{\text{2}}}}}\text{dx}\]
\[\text{=}\frac{\text{3}}{\text{4}}\left[ \frac{\text{x}}{\text{2}}\sqrt{\text{16-}{{\text{x}}^{\text{2}}}}\text{+}\frac{\text{16}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{x}}{\text{4}} \right]_{\text{0}}^{\text{4}}\]
Substituting the limits,
\[\text{=}\frac{\text{3}}{\text{4}}\left[ \text{2}\sqrt{\text{16-16}}\text{+8si}{{\text{n}}^{\text{-1}}}\text{(1)-0-8si}{{\text{n}}^{\text{-1}}}\text{(0)} \right]\]
\[\text{= }\frac{\text{3}}{\text{4}}\left[ \frac{\text{8 }\!\!\pi\!\!\text{ }}{\text{2}} \right]\]
\[\text{= }\frac{\text{3}}{\text{4}}\text{ }\!\![\!\!\text{ 4 }\!\!\pi\!\!\text{ }\!\!]\!\!\text{ }\]
\[\text{= 3 }\!\!\pi\!\!\text{ }\]
As a result, the ellipse's area is \[\text{= 4}\times \text{3 }\!\!\pi\!\!\text{ = 12 }\!\!\pi\!\!\text{ }\].
2. Find the area of the region bounded by the ellipse \[\frac{{{\text{x}}^{\text{2}}}}{4}\text{+}\frac{{{\text{y}}^{\text{2}}}}{\text{9}}\text{=1}\].
Ans:
Area Bounded by Ellipse
Area of ellipse \[\text{=4 }\!\!\times\!\!\text{ }\] Area of \[\text{OAB}\]
Area of \[\text{OAB=}\int_{\text{0}}^{2}{\text{y}}\text{dx}\]
Substitute $y=3\sqrt{1-\frac{{{x}^{2}}}{4}}$
\[\text{=}\int_{\text{0}}^{2}{\text{3}}\sqrt{\text{1-}\frac{{{\text{x}}^{\text{2}}}}{4}}\text{dx}\]
simplifying,
\[\text{=}\frac{\text{3}}{2}\int_{\text{0}}^{2}{\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}}\text{dx}\]
\[\text{=}\frac{\text{3}}{2}\left[ \frac{\text{x}}{\text{2}}\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}\text{+}\frac{4}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{x}}{2} \right]_{\text{0}}^{2}\]
Substituting the limits,
\[\text{=}\frac{\text{3}}{2}\left[ \frac{\text{2 }\!\!\pi\!\!\text{ }}{\text{2}} \right]\]
\[\text{=}\frac{\text{3 }\!\!\pi\!\!\text{ }}{2}\]
As a result, the ellipse's area is \[\text{4}\times \frac{\text{3 }\!\!\pi\!\!\text{ }}{2}\text{=6 }\!\!\pi\!\!\text{ }\].
3. Area lying in the first quadrant and bounded by the circle \[{{\text{x}}^{\text{2}}}\text{ + }{{\text{y}}^{\text{2}}}\text{ = 4}\] and the lines \[\text{x=0}\]
A. \[\text{ }\!\!\pi\!\!\text{ }\]
B. \[\frac{\text{ }\!\!\pi\!\!\text{ }}{2}\]
C. \[\frac{\text{ }\!\!\pi\!\!\text{ }}{3}\]
D. \[\frac{\text{ }\!\!\pi\!\!\text{ }}{4}\]
Ans:
Area Lying in the First Quadrant and Bounded by the Circle
\[\text{Area OAB=}\int_{0}^{\text{2}}{\text{y}}\text{dx}\]
substitute$y=\sqrt{4-{{x}^{2}}}$
\[\text{=}\int_{\text{0}}^{\text{2}}{\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}}\text{dx}\]1
\[\text{=}\left[ \frac{\text{x}}{\text{2}}\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}\text{+}\frac{\text{4}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{x}}{\text{2}} \right]_{\text{0}}^{\text{2}}\]
Substituting the limits,
\[\text{=2}\left( \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}} \right)\]
\[\text{= }\!\!\pi\!\!\text{ }\]
As a result, the required area is \[\text{ }\!\!\pi\!\!\text{ }\] option A.
4. Area of the region bounded by the curve \[{{\text{y}}^{\text{2}}}\text{ = 4x, y-axis}\] and the line \[\text{y=3}\] is
A. \[2\]
B. \[\frac{9}{4}\]
C. \[\frac{9}{3}\]
D. \[\frac{9}{2}\]
Ans:
\[\text{Area OAB=}\int_{0}^{3}{x}\text{dy}\]
substitute $x=\frac{{{y}^{2}}}{4}$
\[\text{=}\int_{\text{0}}^{3}{\frac{{{y}^{2}}}{4}}\text{dy}\]
\[\text{=}\frac{1}{4}\left[ \frac{{{y}^{3}}}{4} \right]_{\text{0}}^{3}\]
Substituting the limits,
\[\text{=}\frac{1}{\text{12}}\left( 27 \right)\]
\[\text{=}\frac{\text{9}}{\text{4}}\text{ units}\]
As a result, the correct response is \[\frac{\text{9}}{\text{4}}\text{ units}\] option B.
Miscellaneous Exercise
1. Find the area under the given curves and given lines:
(i) \[\text{y=}{{\text{x}}^{\text{2}}}\text{,x=1,x=2}\] and \[\text{x-axis}\]
Ans:
\[\text{AreaADCBA=}\int_{\text{1}}^{\text{2}}{\text{y}}\text{dx}\]
substitute $y={{x}^{2}}$
\[\text{=}\int_{\text{1}}^{\text{2}}{{{\text{x}}^{\text{2}}}}\text{dx}\]
Substituting the limits,
\[\text{=}\frac{\text{8}}{\text{3}}\text{-}\frac{\text{1}}{\text{3}}\]
\[\text{=}\frac{\text{7}}{\text{3}}\text{units}\]
(ii) \[\text{y=}{{\text{x}}^{4}}\text{,x=1,x=5}\] and \[\text{x-axis}\]
Ans:
\[\text{AreaofADCBA=}\int_{\text{1}}^{\text{5}}{{{\text{x}}^{\text{4}}}}\text{dx}\]
Integrating using the power rule,
\[\text{=}\left[ \frac{{{\text{x}}^{\text{5}}}}{\text{5}} \right]_{\text{1}}^{\text{5}}\]
Substituting the limits,
\[\text{=}\frac{{{\text{(5)}}^{\text{5}}}}{\text{5}}\text{-}\frac{\text{1}}{\text{5}}\]
Simplifying,
\[\text{=(5}{{\text{)}}^{\text{4}}}\text{-}\frac{\text{1}}{\text{5}}\]
\[\text{=625-}\frac{\text{1}}{\text{5}}\]
\[\text{=624}\text{.8 units}\]
2. Sketch the graph of \[\text{y=}\left| \text{x+3} \right|\] and evaluate \[\int_{-6}^{0}{\left| \text{x+3} \right|}\text{dx}\].
Ans:
\[\left( \text{x+3} \right)\le 0\] for \[\text{-6}\le \text{x}\le \text{-3}\]
\[\left( \text{x+3} \right)\ge \text{0}\] for \[\text{-3}\le \text{x}\le 0\]
Therefore,
\[\int_{\text{-6}}^{\text{0}}{\text{ }\!\!|\!\!\text{ }}\text{(x+3) }\!\!|\!\!\text{ dx=-}\int_{\text{-6}}^{\text{-3}}{\text{(x+3)}}\text{dx+}\int_{\text{-3}}^{\text{0}}{\text{(x+3)}}\text{dx}\]
Integrating using the power rule
\[\text{= -}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+3x} \right]_{\text{-6}}^{\text{-3}}\text{+}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+3x} \right]_{\text{-3}}^{\text{0}}\]
Substituting the limits,
\[\text{=-}\left[ \left( \frac{{{\text{(-3)}}^{\text{2}}}}{\text{2}}\text{+3(-3)} \right)\text{-}\left( \frac{{{\text{(-6)}}^{\text{2}}}}{\text{2}}\text{+3(-6)} \right) \right]\text{+}\left[ \text{0-}\left( \frac{{{\text{(-3)}}^{\text{2}}}}{\text{2}}\text{+3(-3)} \right) \right]\]
Simplifying,
\[\text{= -}\left[ \text{-}\frac{\text{9}}{\text{2}} \right]\text{-}\left[ \text{-}\frac{\text{9}}{\text{2}} \right]\]
\[\text{=9}\]
3. Find the area bounded by the curve \[\text{y=sinx}\] between \[\text{x=0}\] and \[\text{x=2 }\!\!\pi\!\!\text{ }\].
Ans:
Therefore, \[\text{area = Area OABO+ Area BCDB}\]
Area Bounded by Curve y=sinx
\[\text{=}\int_{\text{0}}^{\text{ }\!\!\pi\!\!\text{ }}{\text{sin}}\text{xdx+}\left| \int_{\text{ }\!\!\pi\!\!\text{ }}^{\text{2 }\!\!\pi\!\!\text{ }}{\text{sin}}\text{xdx} \right|\]
\[\text{= }\!\![\!\!\text{ -cosx }\!\!]\!\!\text{ }_{\text{0}}^{\text{ }\!\!\pi\!\!\text{ }}\text{+}\left| \text{ }\!\![\!\!\text{ -cosx }\!\!]\!\!\text{ }_{\text{ }\!\!\pi\!\!\text{ }}^{\text{2 }\!\!\pi\!\!\text{ }} \right|\]
Substituting the limits,
\[\text{= }\!\![\!\!\text{ -cos }\!\!\pi\!\!\text{ +cos0 }\!\!]\!\!\text{ + }\!\!|\!\!\text{ -cos2 }\!\!\pi\!\!\text{ +cos }\!\!\pi\!\!\text{ }\!\!|\!\!\text{ }\]
Simplifying,
\[\text{=1+1+ }\!\!|\!\!\text{ (-1-1) }\!\!|\!\!\text{ }\]
\[\text{=2+ }\!\!|\!\!\text{ -2 }\!\!|\!\!\text{ }\]
\[\text{=2+2}\]
\[\text{=4 units}\]
4. Area bounded by the curve \[\text{y=}{{\text{x}}^{3}}\], the \[\text{x-axis}\] and the ordinates \[\text{x = -2}\] and \[\text{x = 1}\] is
A. \[\text{-9}\]
B. \[\text{-}\frac{\text{15}}{\text{4}}\]
C. \[\frac{\text{15}}{\text{4}}\]
D. \[\frac{\text{17}}{\text{4}}\]
Ans:
As shown in the diagram, the required area is:
\[\text{Required area =}\int_{\text{-2}}^{\text{1}}{\text{y}}\text{dx}\]
\[\text{=}\int_{\text{-2}}^{\text{1}}{{{\text{x}}^{\text{3}}}}\text{dx}\]
Integrating using the power rule
\[\text{=}\left[ \frac{{{\text{x}}^{\text{4}}}}{\text{4}} \right]_{\text{-2}}^{\text{1}}\]
Substituting the limits,
\[\text{=}\left[ \frac{\text{1}}{\text{4}}\text{-}\frac{{{\text{(-2)}}^{\text{4}}}}{\text{4}} \right]\]
Simplifying,
\[\text{=}\left( \frac{\text{1}}{\text{4}}\text{-4} \right)\]
\[\text{= -}\frac{\text{15}}{\text{4}}\text{ units}\]
So, the correct answer is \[\text{ -}\frac{\text{15}}{\text{4}}\text{ units}\] option B.
5. The area bounded by the curve \[\text{y=x}\left| \text{x} \right|\text{,x-axis}\] and the ordinate \[\text{x = 1}\] and \[\text{x = -1}\] is given by (Hint \[\text{y = }{{\text{x}}^{2}}\] if \[x>0\] and \[\text{y = -}{{\text{x}}^{2}}\] if \[x<0\])
A. \[0\]
B. \[\frac{\text{1}}{\text{3}}\]
C. \[\frac{2}{\text{3}}\]
D. \[\frac{4}{\text{3}}\]
Ans:
\[\text{Required area=}\int_{\text{-1}}^{\text{1}}{\text{y}}\text{dx}\]
\[\text{=}\int_{\text{-1}}^{\text{1}}{\text{x}}\text{ }\!\!|\!\!\text{ x }\!\!|\!\!\text{ dx}\]
\[\text{= -}\int_{\text{-1}}^{\text{0}}{{{\text{x}}^{\text{2}}}}\text{dx+}\int_{\text{0}}^{\text{1}}{{{\text{x}}^{\text{2}}}}\text{dx}\]
Integrating using the power rule
\[\text{= -}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{-1}}^{\text{0}}\text{+}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{0}}^{\text{1}}\]
Substituting the limits,
\[\text{= -}\left( \text{-}\frac{\text{1}}{\text{3}} \right)\text{+}\frac{\text{1}}{\text{3}}\]
\[\text{=}\frac{\text{2}}{\text{3}}\text{units}\]
So, the correct answer is \[\frac{\text{2}}{\text{3}}\text{units}\] option C.
Overview of Deleted Syllabus for CBSE Class 12 Maths Application of Integrals
Class 12 Maths Chapter 8: Exercises Breakdown
Conclusion
Chapter 8, "Application of Integrals," is a crucial part of the Class 12 Maths curriculum. This chapter primarily focuses on using integrals to find the area under simple curves, including lines, circles, parabolas, and ellipses. Students also learn to find the area under the simple curve, which is a vital skill for various mathematical and engineering applications. Over the past few years, Chapter 8 has consistently featured in the exams with an average of 4-6 questions. These questions often include a mix of straightforward calculations and application-based problems that test the depth of understanding of integral applications.
Other Study Material for CBSE Class 12 Maths Chapter 8
Chapter-Specific NCERT Solutions for Class 12 Maths
Given below are the chapter-wise NCERT Solutions for Class 12 Maths. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.
FAQs on NCERT Solutions for Class 12 Maths Chapter 8 Application of Integrals
1. Which are the important questions in the application of integrals?
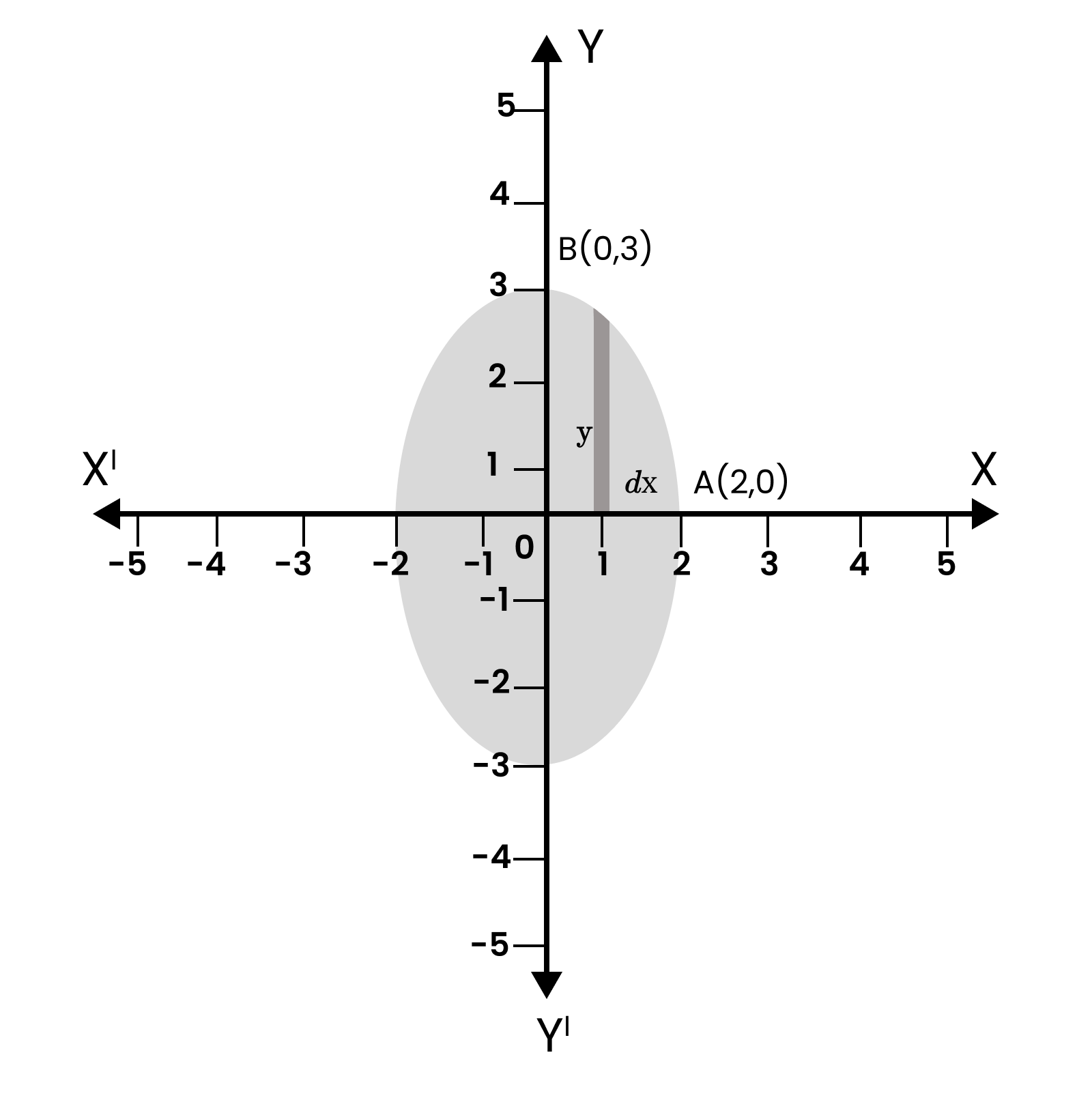
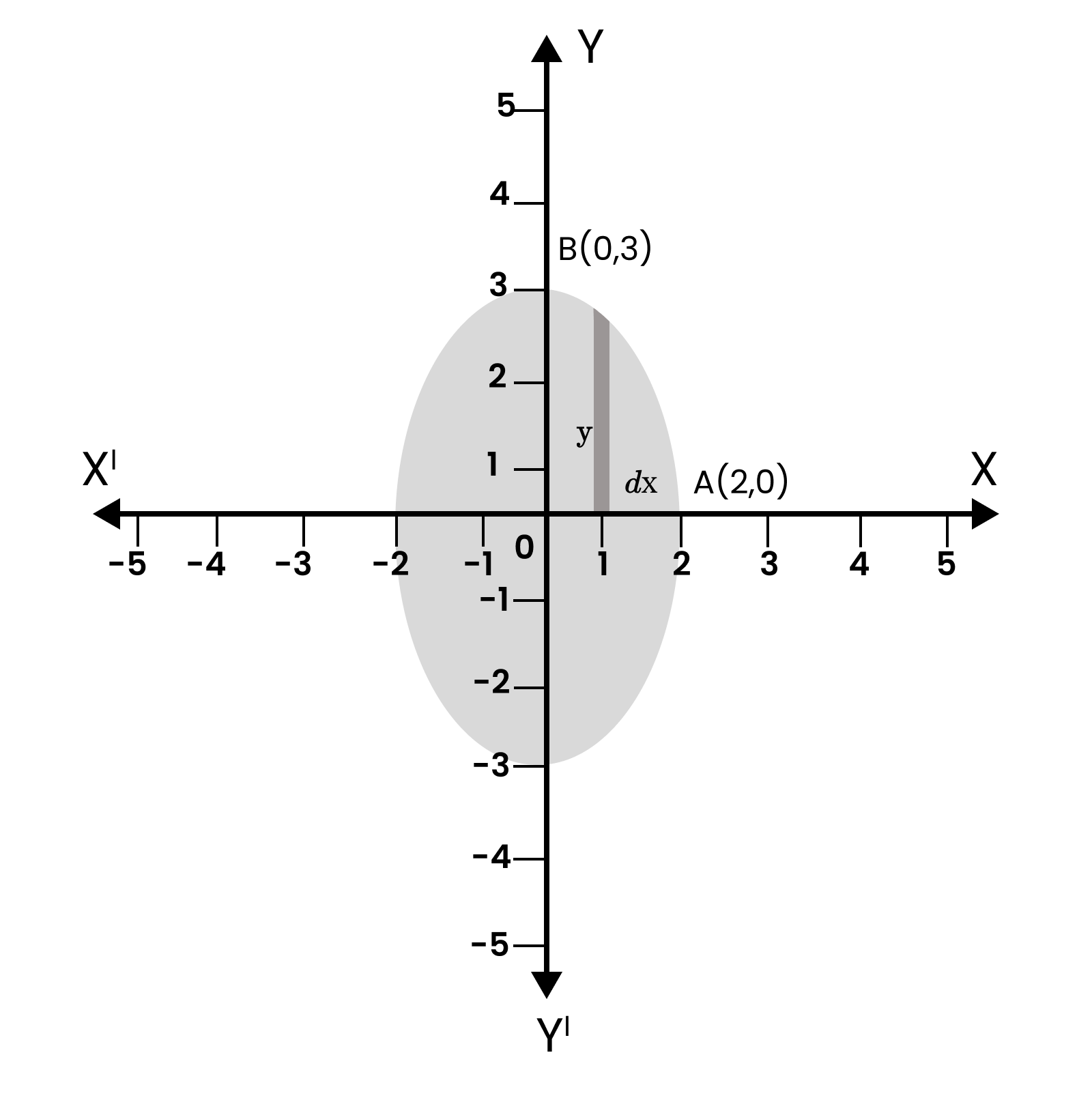
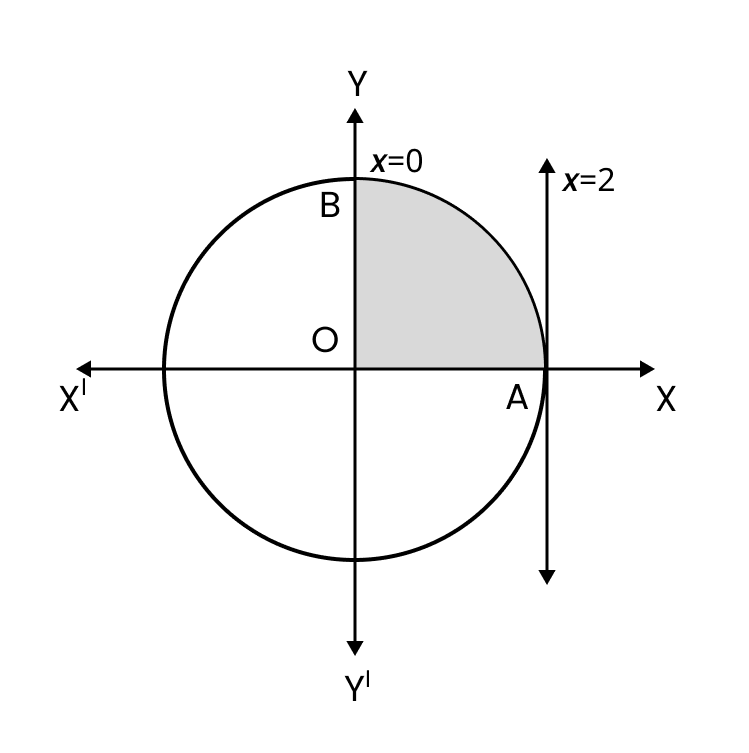
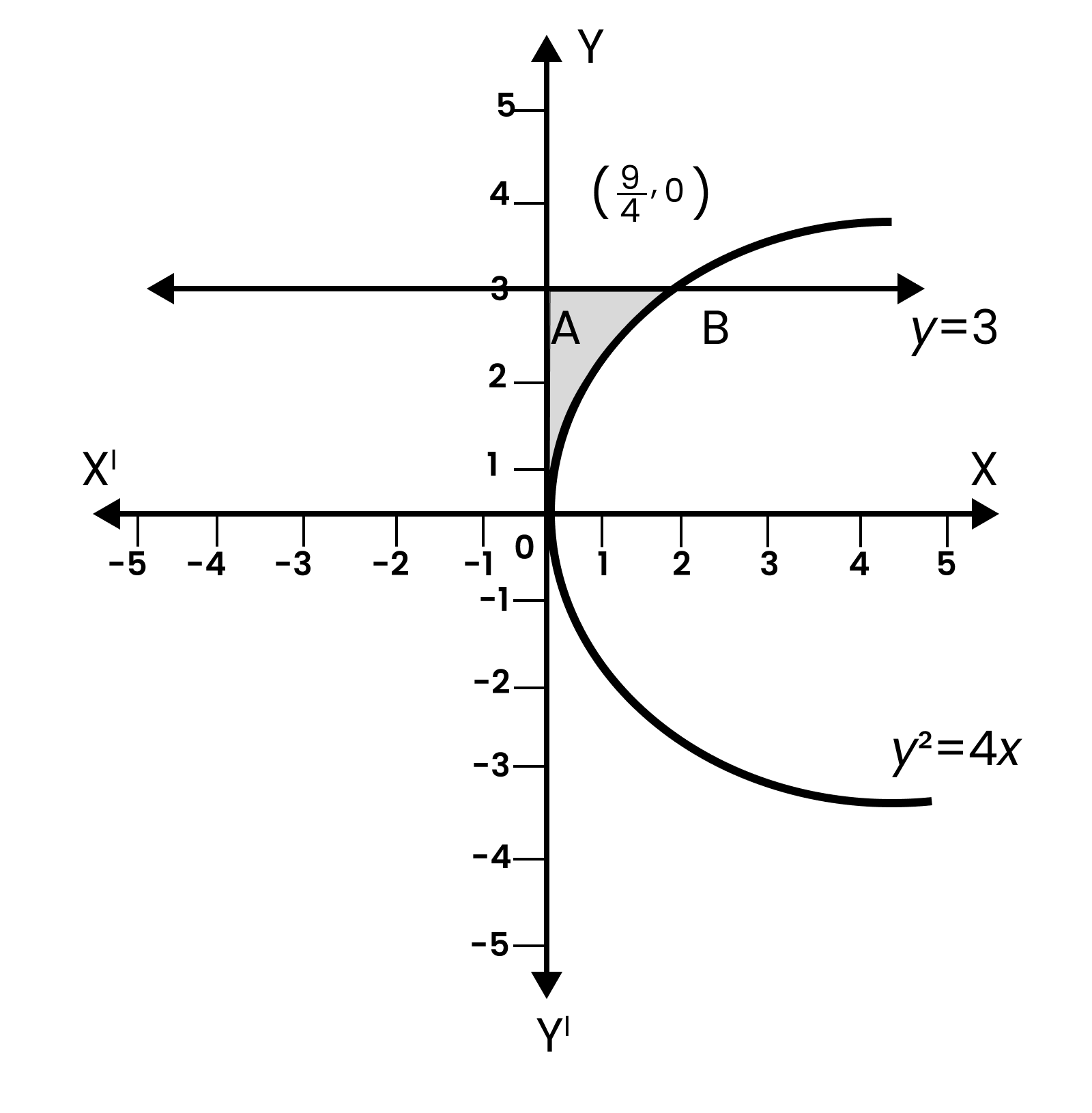
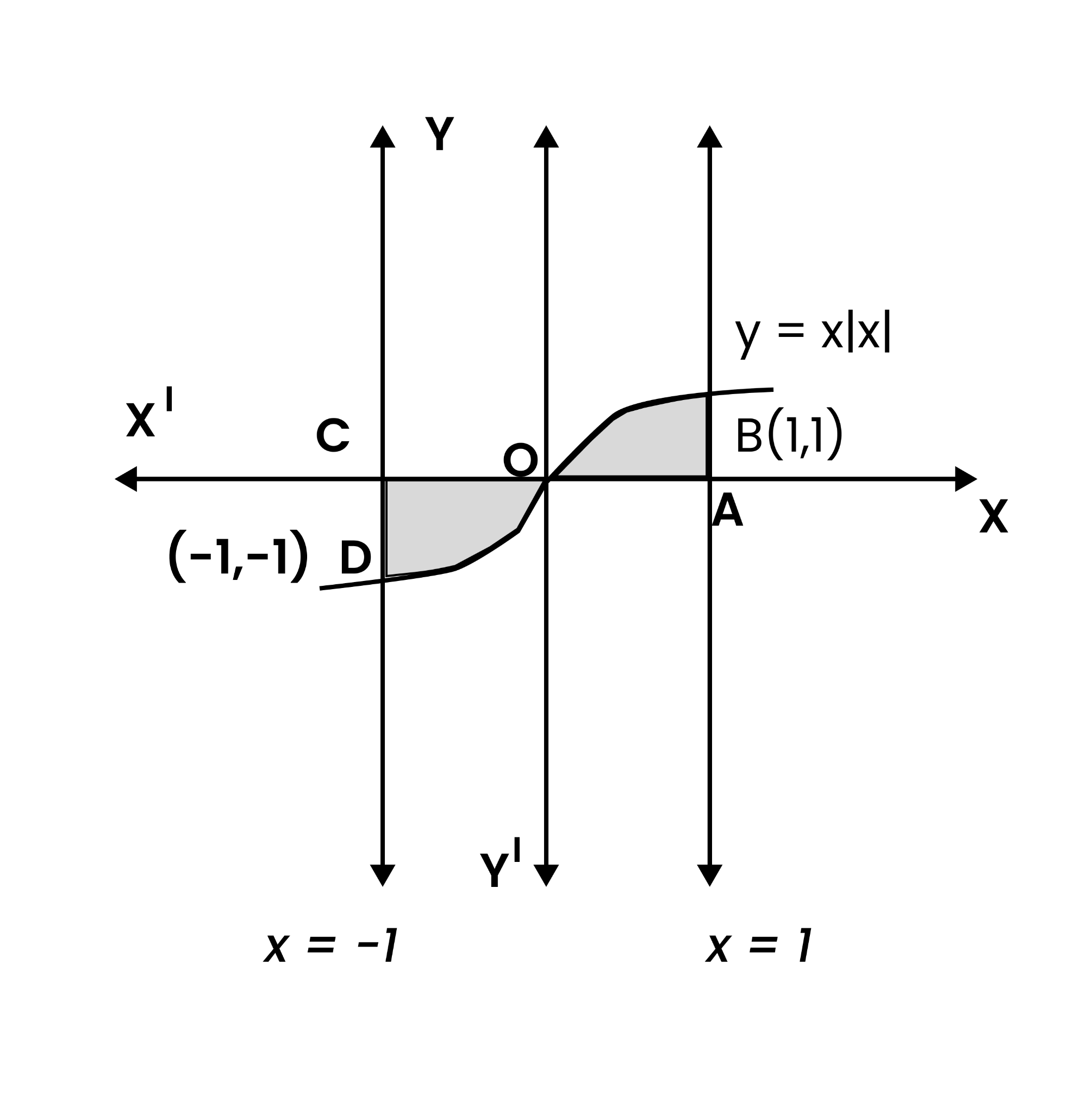
Finding the area enclosed by a single curve (y = f(x)) and the x or y-axis within a specific interval.
Integrals Class 12 NCERT Solutions PDF Download is available for students.
2. What is the application of integral in real life?
Class 12 Maths Integration NCERT Solutions have a surprising number of real-world applications! Here are some of the common ones:
Shape Design and Construction
Calculating Work Done
Fluid Mechanics:
Motion and Kinematics
Economics and Business
3. What is the objective of the application of integrals?
Calculate Areas and Volumes
Find Work Done by Variable Forces
Analyze Motion and Change
Model and Analyze Rates of Change
4. How many exercises are in the application of integrals?
The exact number of exercises in applications of integrals class 12 (Chapter 8) can vary depending on the specific textbook or curriculum you're following. There isn't a universally fixed number. But in the NCERT Text Book, there is one exercise and a miscellaneous exercise, both exercises cover the topic - Area under a simple curve.
Exercise 8.1: Consists of 4 questions
Miscellaneous Exercise
5. What are the three types of integrals?
There are more than three fundamental types of integrals, but here's a breakdown of two commonly encountered categories in introductory calculus:
Definite Integrals: These integrals represent the definite accumulation of a quantity over a specific interval. They are denoted by a lower and upper bound with an integral sign: ∫ mobilya f(x) dx (where a and b are the bounds and f(x) is the integrand). Definite integrals are used to calculate areas, volumes, work done by a force, and other quantities that have a clear starting and ending point.
Indefinite Integrals: These integrals represent the general antiderivative of a function. They are denoted without bounds and result in a family of functions that differ by a constant (C): ∫ f(x) dx = F(x) + C (where F(x) is the antiderivative). Indefinite integrals are useful for finding functions whose derivative is a known function and are often used as intermediate steps when solving definite integral problems.
Here are some additional types of integrals you might encounter in more advanced calculus:
Improper Integrals: These are definite integrals where the integrand approaches positive or negative infinity, or oscillates infinitely, at one or both bounds. Special techniques are needed to evaluate them and determine if they converge to a finite value.
Multiple Integrals: These integrals extend the concept of integration to higher dimensions. They are used to calculate volumes of 3D shapes, surface integrals (representing mass or flow over a surface), and line integrals (representing work done along a path).
Contour Integrals: These integrals are used in complex analysis to integrate functions along curves in the complex plane. They have applications in electromagnetism, fluid mechanics, and other areas.
6. Why do we use integrals in work?
We use integrals to calculate work done when the force isn't constant throughout the process. Here are some uses of Application of Integrals class 12.
Work and Force: In simple terms, work (W) is the product of force (F) and distance (d): W = F * d. This formula works well when the force applied is constant.
Variable Force: However, in many real-world scenarios, the force acting on an object changes as the object moves. Imagine lifting a weight with a spring attached. The force required increases as the spring gets compressed.
Infinitely Small Segments: Integrals come in to handle this variable force. We can imagine dividing the total distance into infinitely small segments (like infinitesimal slices of bread in a loaf). Over each tiny segment, we can assume the force is somewhat constant.
Summing Up Work: Then, we can calculate the work done by the force within each segment (considering the average force over that segment). Finally, we sum the work done in all these infinitesimal segments using an integral. This summation process gives us the total work done for the entire variable force scenario.
By using integrals, we can account for the continuous change in force and obtain an accurate measure of the total work done. This is essential in various fields like:
Engineering: Calculating the work done by a machine lifting an object, a car engine compressing air, or a fluid exerting pressure on a turbine.
Physics: Understanding the work done by gravity on a falling object, a spring contracting, or an electric field moving a charged particle.
Economics: Analyzing the work done (energy expended) in production processes with variable costs or effort.