NCERT Solutions for Maths Class 9 Chapter 9 Circles Exercise 9.3 - FREE PDF Download
NCERT Solutions for Class 9 Maths Chapter 9 Circles Ex 9.3
FAQs on NCERT Solutions for Class 9 Maths Chapter 9 Circles Ex 9.3
1. What are the theorems about angles in a circle in NCERT Class 9 Maths Chapter 9 Exercise 9.3 Solutions?
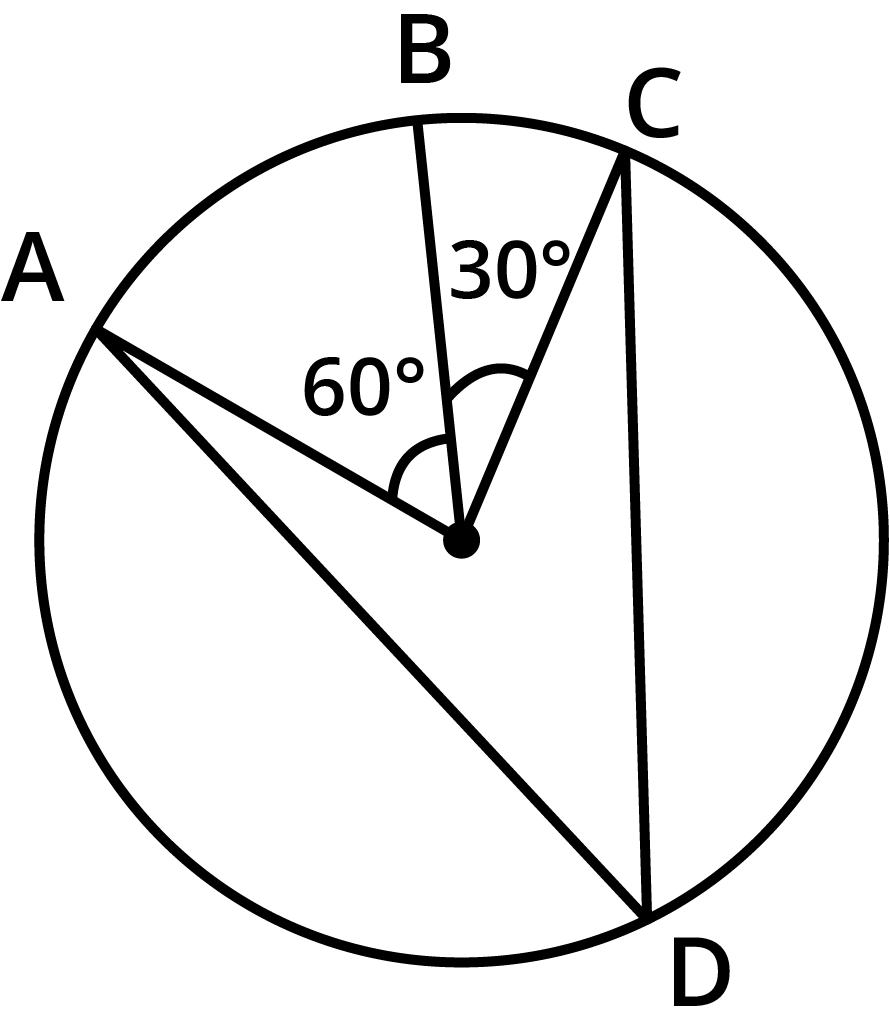
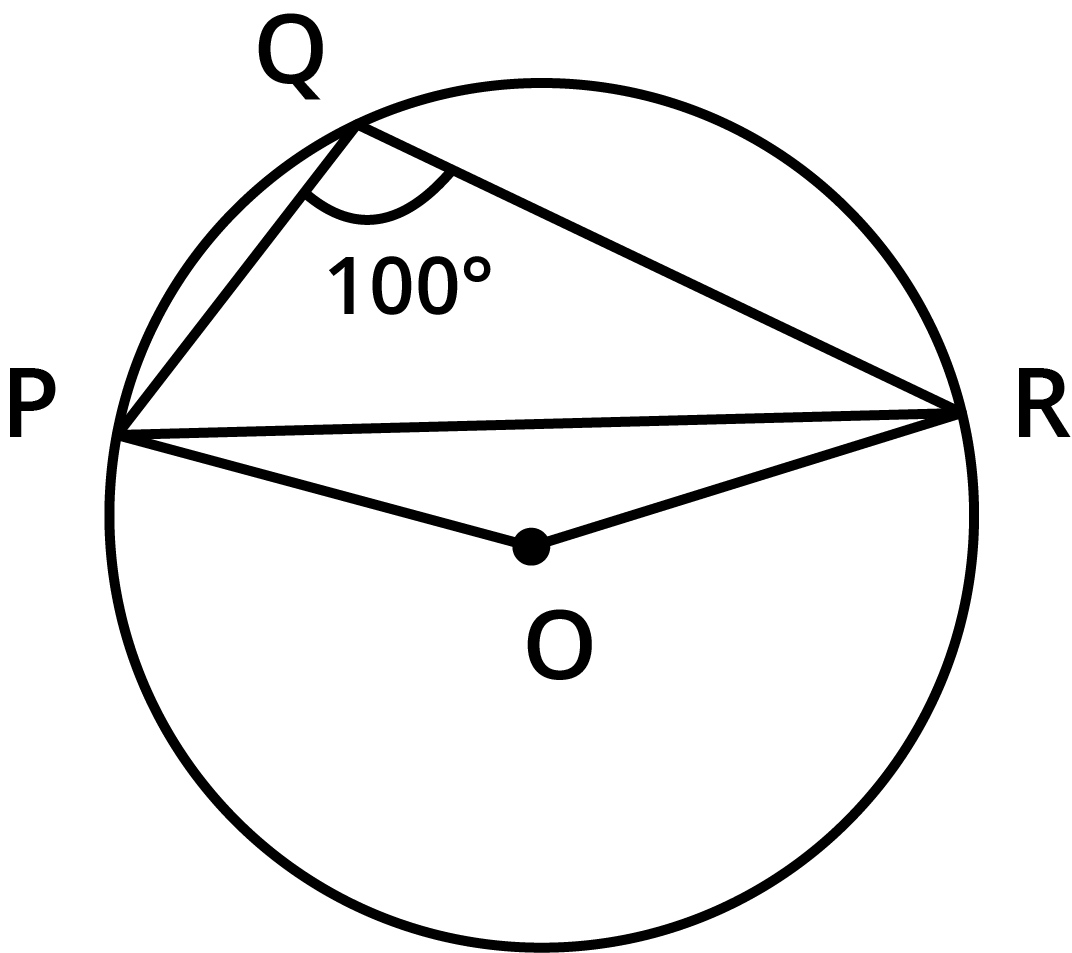
Theorem 1: The angle subtended by an arc at the centre is twice the angle subtended at any other remaining part of the circle.
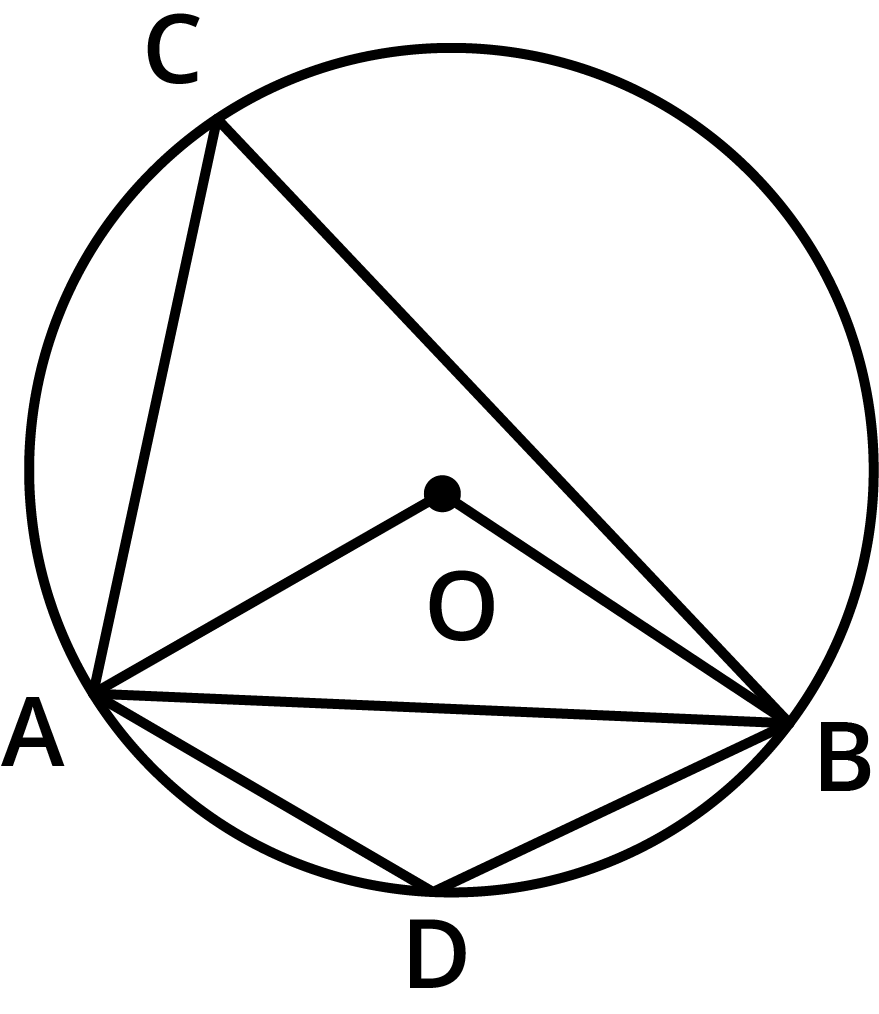
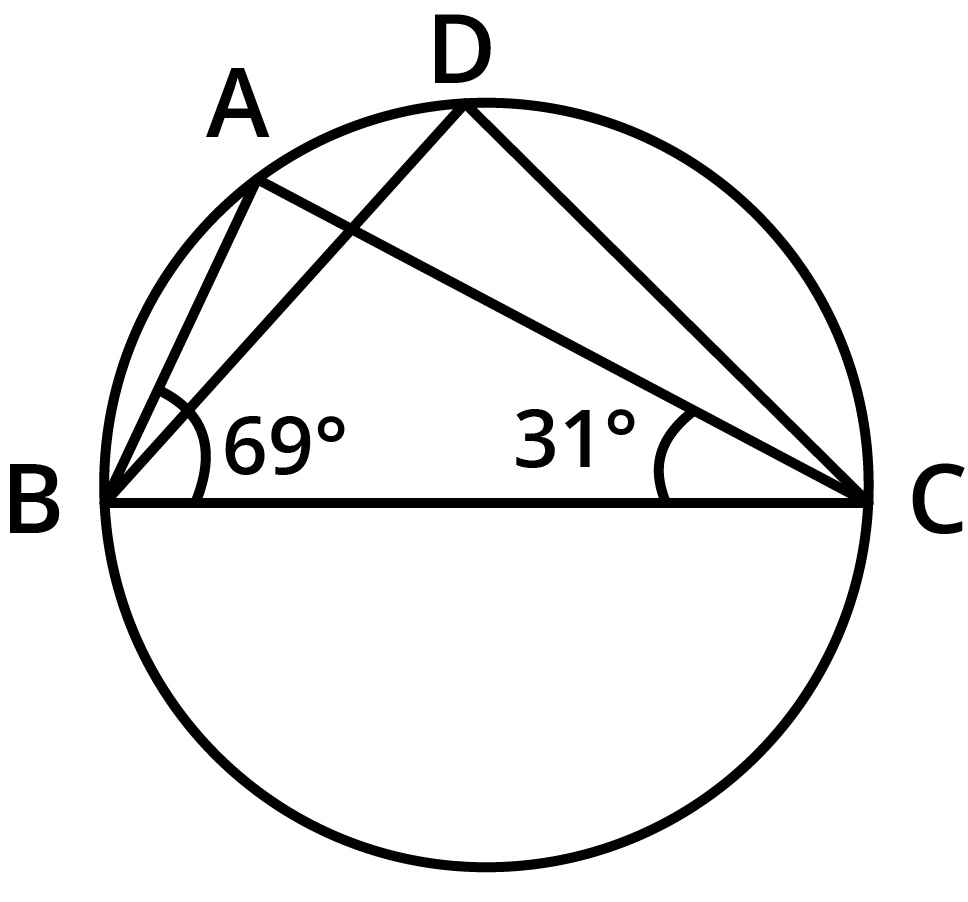
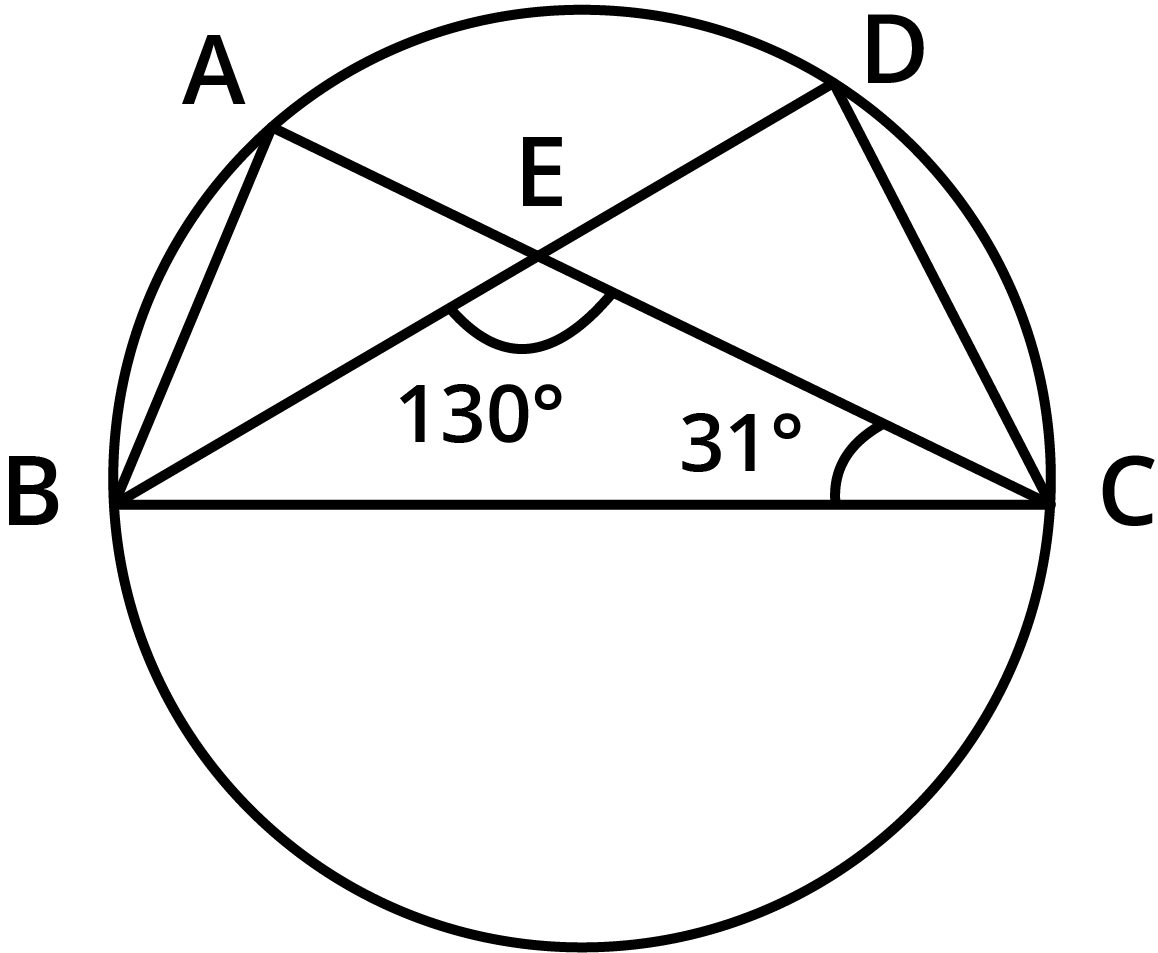
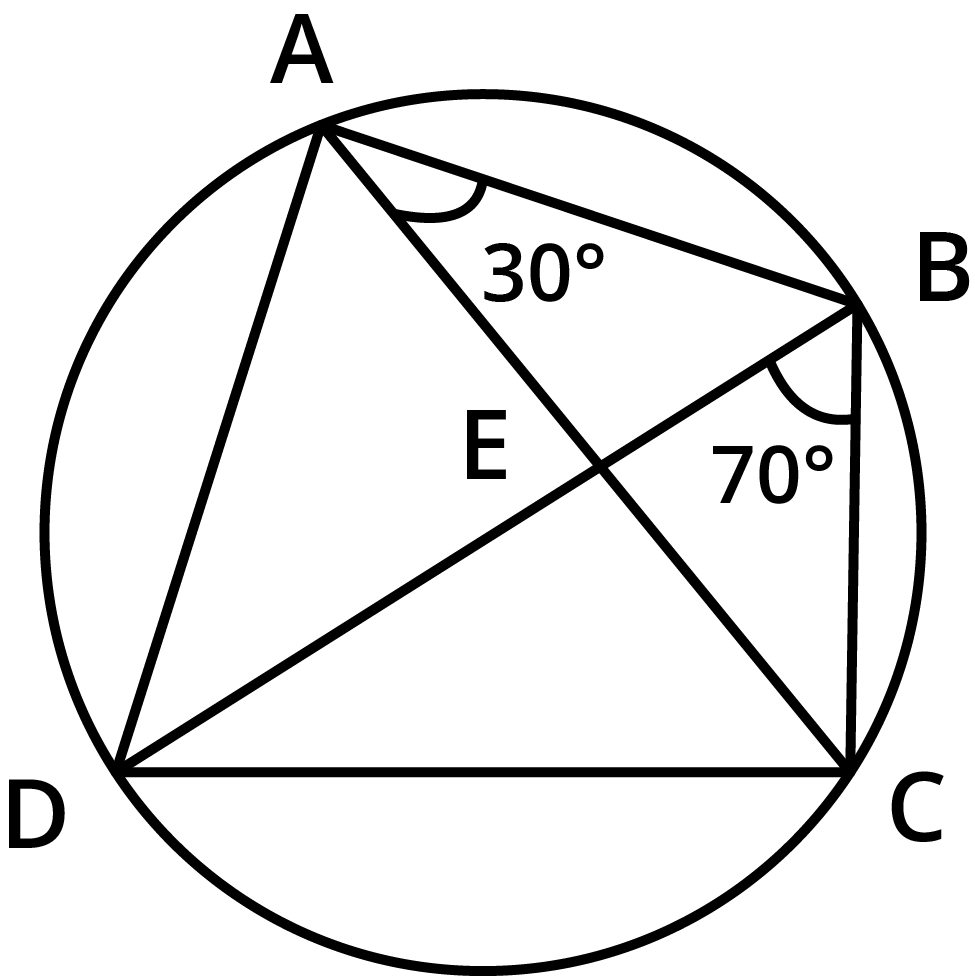
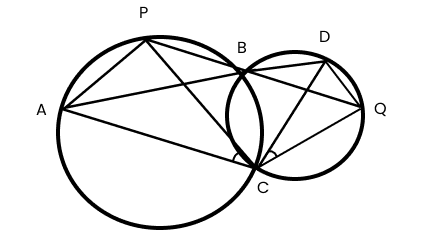
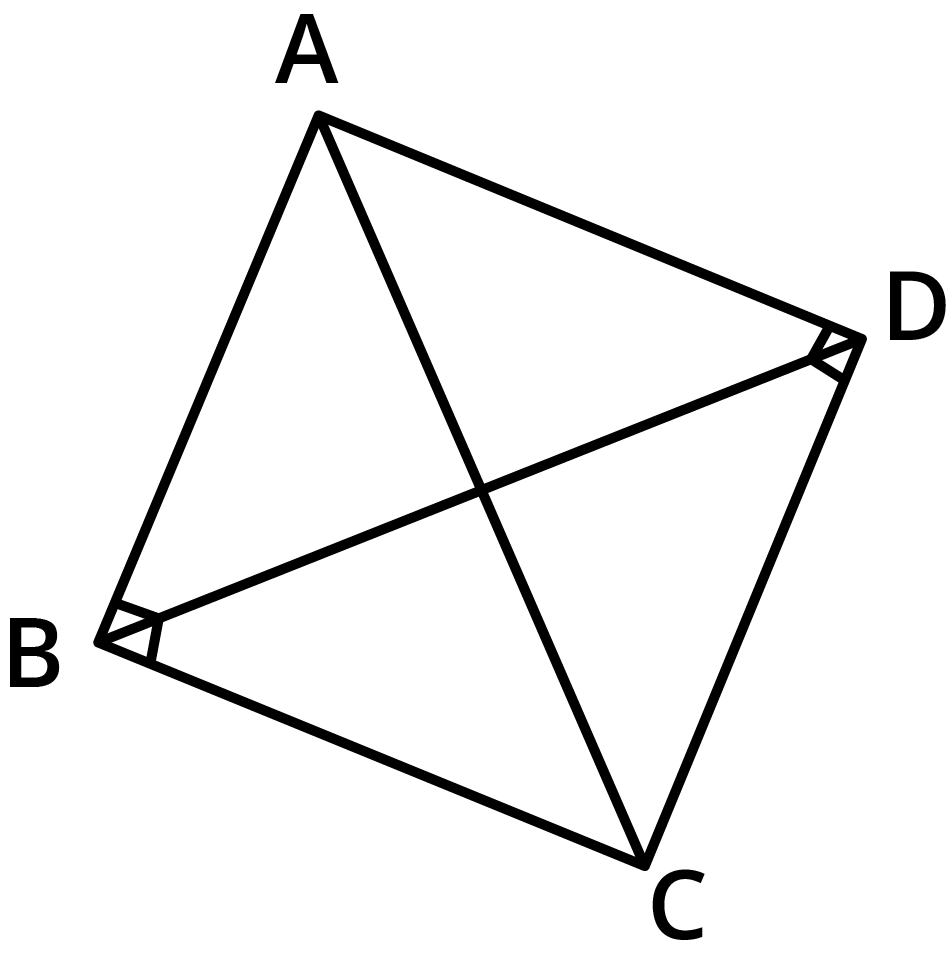
Theorem 2: Angles lying in the same segment of a circle are equal.
2. What is a cyclic quadrilateral in NCERT Class 9 Maths Chapter 9 Exercise 9.3 Solutions?
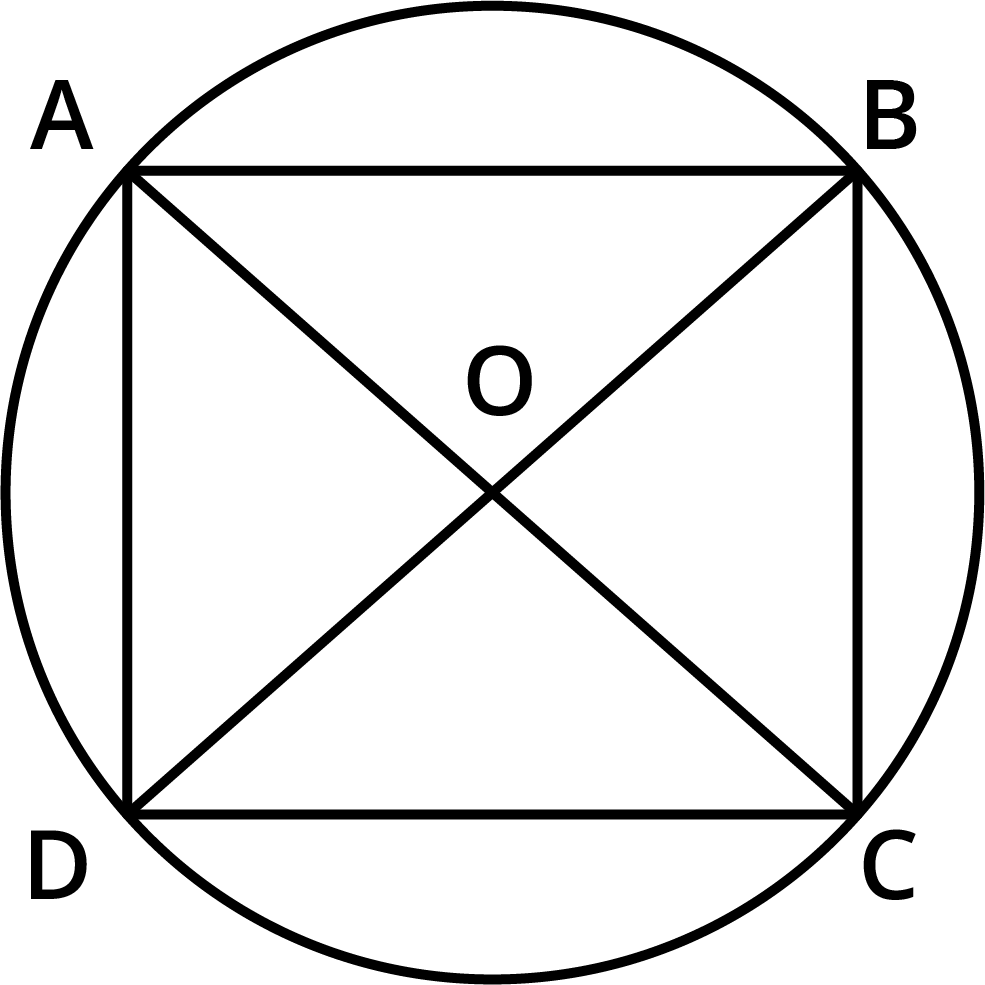
A cyclic quadrilateral is defined as a quadrilateral all of whose vertices lie on the same circle.
3. Give the theorems about cyclic quadrilaterals in Class 9 Maths Chapter 9 Circles Exercise 9.3.
Theorem 1: The sum of the opposite angles in a quadrilateral is 180°.
Theorem 2 (Converse): A quadrilateral is said to be cyclic if the sum of opposite angles in a quadrilateral is 180°.
4. Where can I get easy solutions to the sums in Class 9 Maths Chapter 9 Circles Exercise 9.3?
Refer to the NCERT Solutions of Class 9 Maths Chapter 9 Circles Exercise 9.3 for easy solutions to the sums covered in the exercise. Download the solutions PDF for free from the Vedantu mobile app or website. Here, you can get the easiest step-wise explanation for each sum given in Exercise 9.3. The subject matter experts at Vedantu have prepared these solutions PDF to make your learning experience easy.
5. How many sums are there in Class 9 Maths Chapter 9 Circles Exercise 9.3?
There are 12 sums in Exercise 9.3 of Class 9 Chapter 9. A few sums have sub-parts and the sums vary from one another. Every sum needs you to devise the theorems and concepts of circles. The concepts of cyclic quadrilaterals and theorems are covered in this exercise of the chapter on Circles. The sums are application-based and one sum may require more than one theorem or concept to be applied.
6. Are the NCERT Solutions for Class 9th Maths Chapter 9 Exercise 9.3 available for free?
You can download the NCERT Solutions of Class 9th Maths Chapter 9 Exercise 9.3 from the Vedantu website as well as a mobile app for absolutely free of cost. You can refer to these study resources online as well as offline by signing up on Vedantu. There is no doubt that Vedantu is the best learning platform for students. Apart from providing quality study material, it is helping students to gain knowledge for free.
7. Is Class 9th Maths Chapter 9 Exercise 9.3 important for exams?
Each exercise is important from the viewpoint of the examination. Try to practice each exercise thoroughly. All the sums given in the examples and exercises are to be solved and practised regularly to develop a good grasp of the concepts. So, Exercise 9.3 is important for the Class 9 Maths examination. Every question from this exercise is of utmost importance. You can refer to the NCERT Solutions of Class 9th Maths Chapter 9 Exercise 9.3 on the Vedantu website in case of any doubt.
8. What are the important topics in Class 9th Maths Chapter 9 Exercise 9.3?
To practice the exercise, it is important to analyze the important topics covered in it. A few of the important topics covered in Class 9th Maths NCERT Solutions Chapter 9 Exercise 9.3 are given below.
Introduction to Circles
Angles that are subtended by an arc of a circle
Angles that are subtended by a chord of a circle
Cyclic quadrilaterals
Theorems used in the chapter.
9. What topics are covered in Class 9th Maths NCERT Solutions Chapter 9 Exercise 9.3?
Class 9th Maths NCERT Solutions Chapter 9 Exercise 9.3 covers the angle subtended by an arc of a circle and the properties of cyclic quadrilaterals. These topics include understanding how angles are formed within circles and the relationship between angles in cyclic quadrilaterals.
10. Why is Class 9th Maths Chapter 9 Circles Exercise 9.3 important for students?
Class 9th Maths Chapter 9 Circles Exercise 9.3 is important because it helps students grasp essential geometric concepts related to circles. Understanding these properties is crucial for solving various geometry problems and is frequently tested in exams.
11. How do the NCERT solutions help in solving Class 9th Maths Chapter 9 Circles Exercise 9.3?
The NCERT solutions provide step-by-step explanations for each problem in Exercise 9.3. These detailed solutions help students understand the methods and concepts, making it easier to solve similar problems on their own.
12. Where can I find the NCERT Solutions for Class 9th Maths Chapter 9 Circles Exercise 9.3?
The NCERT Solutions for Exercise 9.3 can be downloaded from Vedantu's website. They are available in PDF format, making it easy for students to access and use them for their studies and exam preparations.
13. What is the circle of theorem 9.3 Class 9?
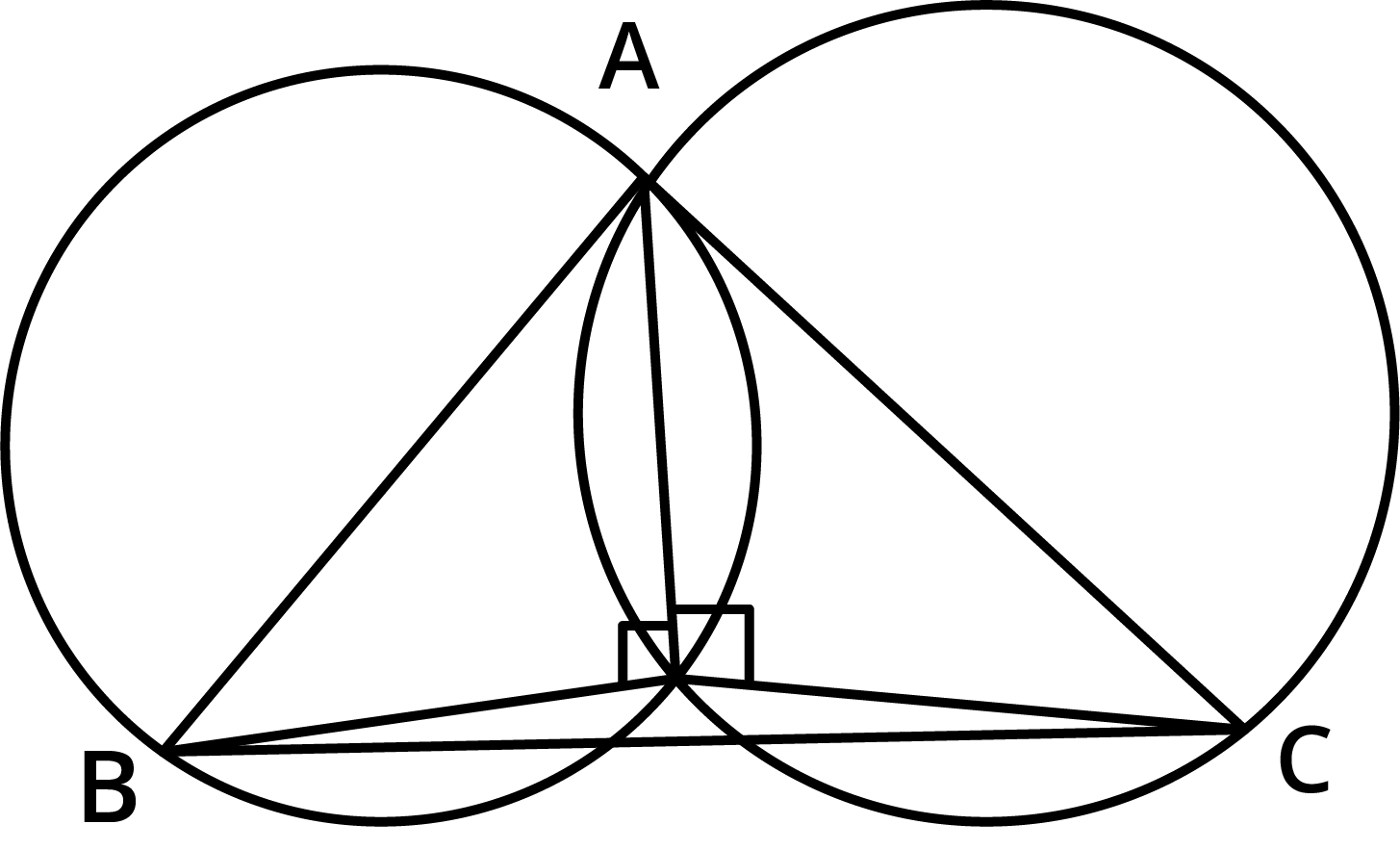
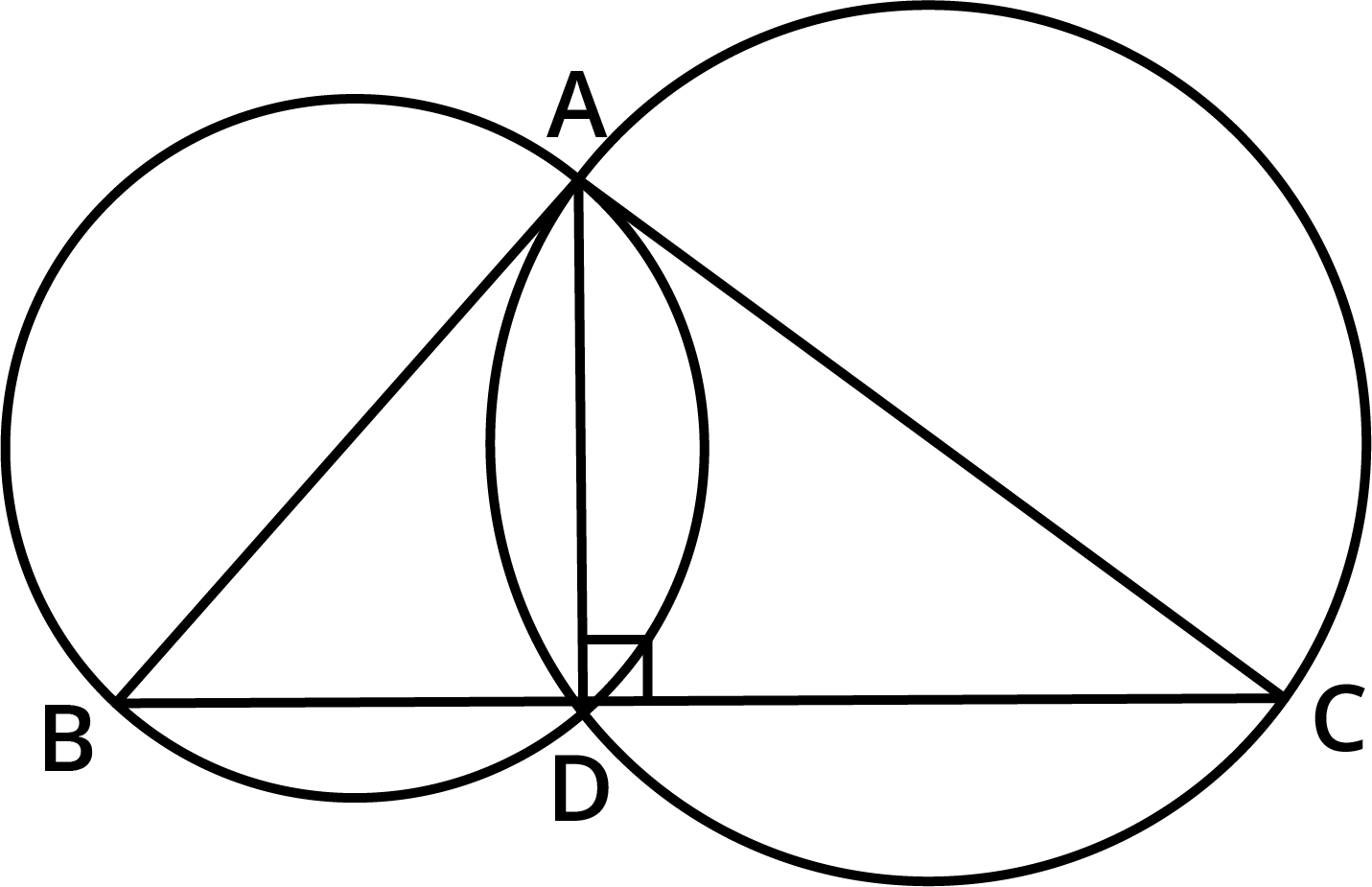
The circle in Theorem 9.3 refers to the shape where all points on the edge are the same distance from a central point. The theorem explains how a perpendicular line from the centre to a chord will divide the chord into two equal parts.














 Watch Video
Watch Video