NCERT Solutions for Class 12 Maths Chapter 6 - Application of Derivatives Exercise 6.2

1. Show, that the function given by $f\left( x \right)=3x+17$ is strictly increasing on $R$.

Ans: Let the two numbers in $R$be ${{x}_{1}}$ and ${{x}_{2}}$.

$ {{x}_{1}}<{{x}_{_{2}}}\Rightarrow 3{{x}_{1}}<3{{x}_{2}} $ 

$ \Rightarrow 3{{x}_{1}}+17<3{{x}_{2}}+17 $ 

$ =f\left( {{x}_{1}} \right)<f\left( {{x}_{2}} \right)$ 

Thus, the function is strictly increasing on $R.$ 

2. Show that the function given $f\left( x \right)={{e}^{2x}}$ is strictly increasing on $R$.

Ans: Let the two numbers in $R$be ${{x}_{1}}$ and ${{x}_{2}}$.

Then, we have: 

$ {{x}_{1}}<{{x}_{2}}\Rightarrow 2{{x}_{1}}<2{{x}_{2}} $ 

$ \Rightarrow {{e}^{2{{x}_{1}}}}<{{e}^{2{{x}_{2}}}} $ 

$ \Rightarrow f\left( {{x}_{1}} \right)<f\left( {{x}_{2}} \right)$ 

Hence, $f$ is strictly increasing on $R.$ 

3. Show that the function given by $f\left( x \right)=\sin x$ is

A) Strictly increasing in $\left( 0,\frac{\pi }{2} \right)$ 

B) Strictly decreasing in $\left( \frac{\pi }{2},\pi  \right)$ 

C) Neither increasing nor decreasing in $\left( 0,n \right)$ 

Ans: The given function is $f\left( x \right)=\sin x$.

$\therefore f'\left( x \right)=\cos x$ 

A) Since for each $x\in \left( 0,\frac{\pi }{2} \right)$, $\cos x>0$, we have $f'\left( x \right)>0$.

Hence, $f$ is strictly increasing in $\left( 0,\frac{\pi }{2} \right)$.

B) Since for each $x\in \left( \frac{\pi }{2},\pi  \right),\cos x<0,$ we have $f'\left( x \right)<0$.

Hence, $f$ is strictly increasing in $\left( \frac{\pi }{2},\pi  \right)$ 

C) It is clear from the results obtained in (A) and (B) that $f$ is neither increasing nor decreasing in $\left( 0,n \right)$.

4. Find the intervals in which the function $f$ given by $f\left( x \right)=2{{x}^{2}}-3x$ is

(A) Strictly increasing

(B) Strictly decreasing

Ans: The function provided is $f\left( x \right)=2{{x}^{2}}-3x$.

  $ f'\left( x \right)=4x-3 $ 

  $ \therefore f'\left( x \right)=0 $ 

  $ \Rightarrow x=\frac{3}{4} $ 

Now, the point $\frac{3}{4}$ divides the real line into two disjoint intervals i.e.,  

$\left( -\infty ,\frac{3}{4} \right)$  and $\left( \frac{3}{4},\infty  \right)$ .

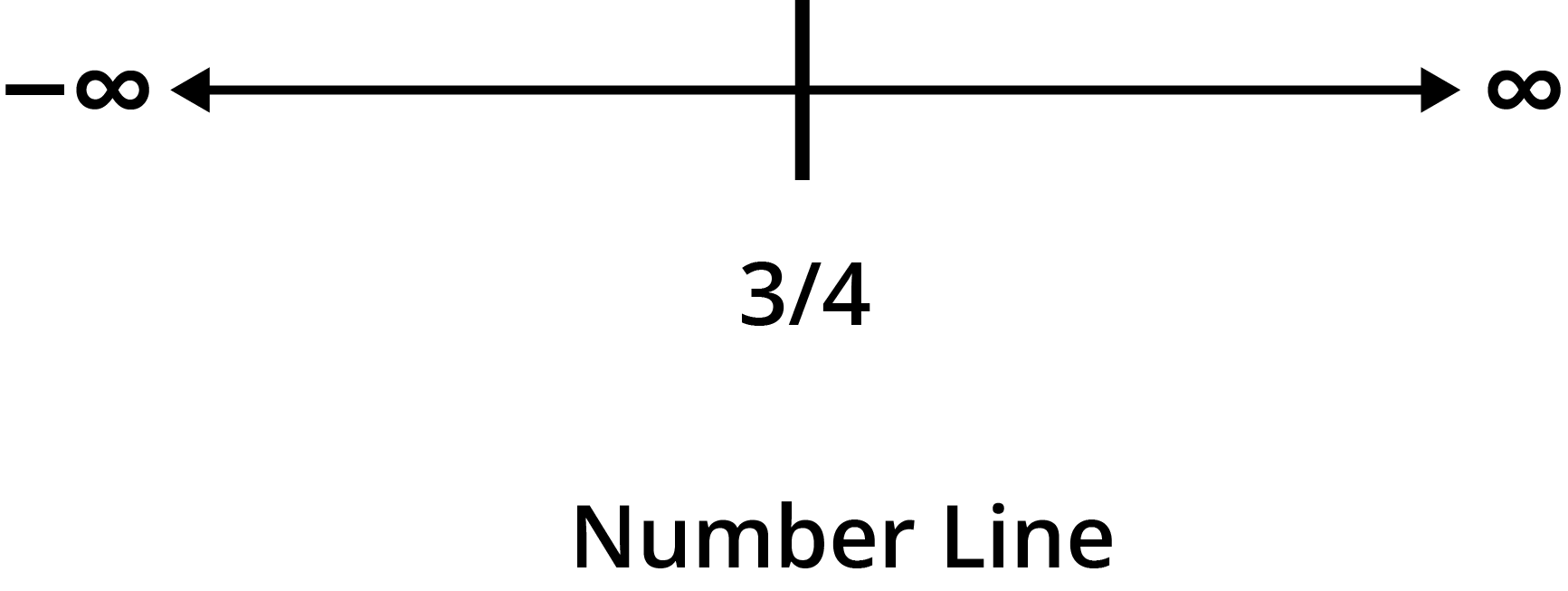
In interval, $\left( -\infty ,\frac{3}{4} \right),f'\left( x \right)=4x-3<0$ 

Hence, the given function $\left( f \right)$ is strictly decreasing in interval $\left( -\infty ,\frac{3}{4} \right)$

In interval, $\left( \frac{3}{4},\infty  \right),f'\left( x \right)=4x-3>0$ 

Hence, the given function $\left( f \right)$ is strictly decreasing in interval $\left( \frac{3}{4},\infty  \right)$

5. Find the intervals in which the function $f$ is given as $f\left( x \right)=2{{x}^{2}}-3{{x}^{2}}-36x+7$ 

(A) strictly increasing

(B) strictly decreasing

 Ans: The given function is

 $ f\left( x \right)=2{{x}^{3}}-3{{x}^{2}}-36x+7 $ 

 $ f'\left( x \right)=6{{x}^{2}}-6x-36 $ 

 $ =6\left( {{x}^{2}}-x-6 \right) $ 

 $ =6\left( x+2 \right)\left( x-3 \right) $ 

 $ \therefore f'\left( x \right)=0 $ 

 $ \Rightarrow x=-2,3 $ 

The points $x=-2,3$ divide the real line into three disjoints intervals 

i.e., $\left( -\infty ,-2 \right),\left( -2,3 \right),$and $\left( 3,\infty  \right)$.

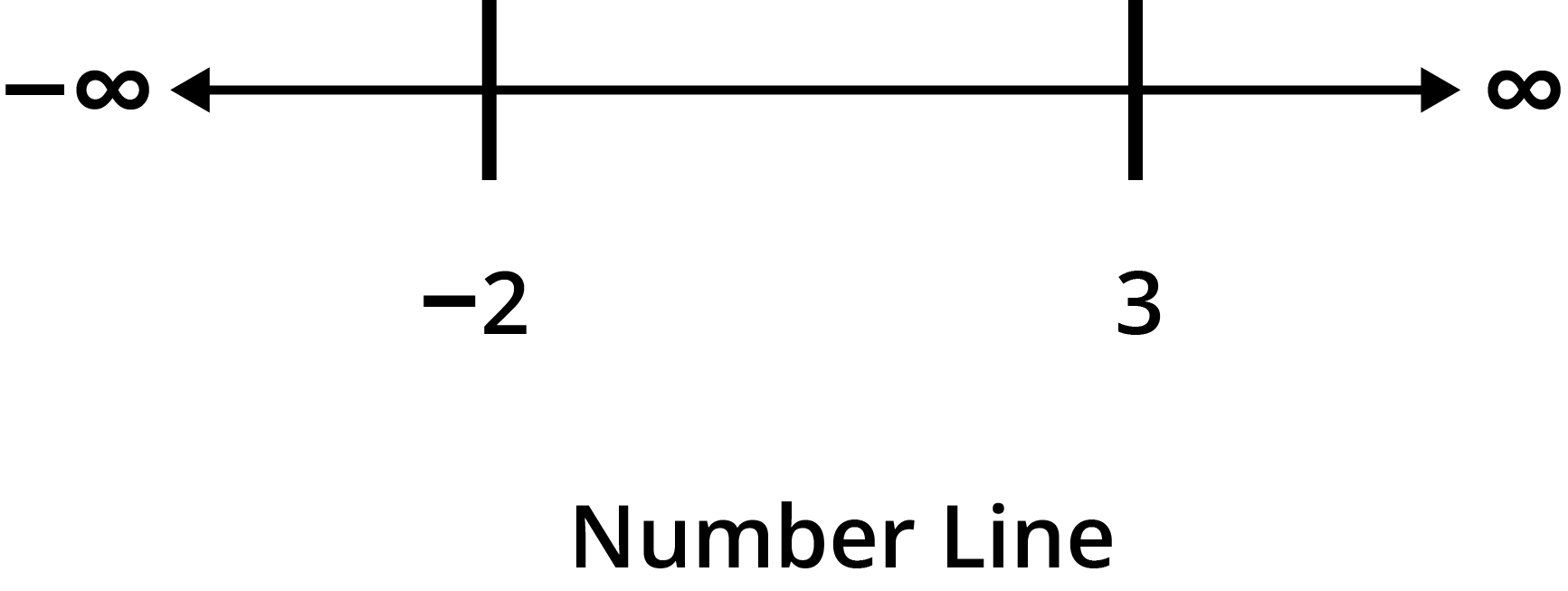
In intervals $\left( -\infty ,-2 \right)$ and $\left( 3,\infty  \right)$, ${f}'\left( x \right)$ is positive while in the interval $\left( -2,3 \right),f'\left( x \right)$ is negative.

Hence, the given function $\left( f \right)$is strictly increasing in intervals $\left( -\infty ,-2 \right)\cup \left( 3,\infty  \right)$ while the function $\left( f \right)$ is strictly decreasing in interval $\left( -2,3 \right)$.

6. Find the intervals in which the following functions are strictly 

increasing or decreasing:

 $ \left( a \right){{x}^{2}}+2x-5 $ 

 $ \left( b \right)10-6x-2{{x}^{2}} $ 

 $ \left( c \right)-2{{x}^{3}}-9{{x}^{2}}-12x+1 $ 

 $ \left( d \right)6-9x-{{x}^{2}} $ 

 $ \left( e \right){{\left( x+1 \right)}^{3}}{{\left( x-3 \right)}^{3}} $ 

Ans:  

a) Given that

$ f\left( x \right)={{x}^{2}}+2x-5 $ 

$ \therefore f'\left( x \right)=2x+2 $ 

Now, 

 $ f'\left( x \right)=0 $ 

 $ \Rightarrow x=-1 $

The real line is divided into two disjoint intervals $\left( -\infty ,-1 \right)and\left( -1,\infty  \right)$ by the point $x=-1$.

In interval $\left( -\infty ,-1 \right),$ 

$\therefore f'\left( x \right)=2x+2<0$ 

Therefore, $f$ is strictly decreasing in the interval $\left( -\infty ,-1 \right)$.

Thus $f$ is strictly decreasing for $x<-1$ 

In interval $\left( -1,\infty  \right),$ 

$\therefore f'\left( x \right)=2x+2>0$ 

Therefore, $f$ is strictly decreasing in interval $\left( -1,\infty  \right)$ 

Thus $f$ is strictly increasing for $x>-1$.

b) Given that,

$ f\left( x \right)=10-6x-2{{x}^{2}} $ 

$ \therefore f'\left( x \right)=-6-4x $ 

Now,

$ f'\left( x \right)=0 $ 

$ \Rightarrow x=\frac{-3}{2} $ 

The real line is divided into two disjoint intervals  $\left( -\infty ,-\frac{3}{2} \right)and\left( -\frac{3}{2},\infty  \right)$ by the point $x=-\frac{3}{2}$.

In interval $\left( -\infty ,-\frac{3}{2} \right)$ i.e., when $x<-\frac{3}{2},f'\left( x \right)=-6-4x<0.$ 

$\therefore f$ is strictly increasing for $x<-\frac{3}{2}$ 

In interval $\left( -\frac{3}{2},\infty  \right)$ i.e., when $x>\frac{3}{2},f'\left( x \right)=-6-4x<0$.

$\therefore f$ is strictly increasing for $x<-\frac{3}{2}$ 

c) Given that,

 $ f\left( x \right)=-2{{x}^{3}}-9{{x}^{2}}-12x+1 $ 

 $ \therefore f'\left( x \right)=-6{{x}^{2}}-18x-12 $ 

 $ =-6\left( {{x}^{2}}+3x+2 \right) $ 

 $ =-6\left( x+1 \right)\left( x+2 \right) $ 

Now,

 $ f'\left( x \right)=0 $ 

 $ \Rightarrow x=-1 $ 

and $x=-2$ 

The real line is divided into three disjoint interval $\left( -\infty ,-2 \right),\left( -2,-1 \right)and\left( -1,\infty  \right)$ by the points $x=-1,-2$  when $x<-2$ and $x>-1$,

$f'\left( x \right)=-6\left( x+1 \right)\left( x+2 \right)<0$ 

$\therefore f$ is strictly increasing for $x<-2<x>-1$ 

Now, in interval $\left( -2,-1 \right)$ i.e., when $-2<x<-1$,

$f'\left( x \right)=-6\left( x+1 \right)\left( x+2 \right)>0$ 

$\therefore f$ is strictly increasing for $-2<x<-1$

d) Given that,

$ f\left( x \right)=6-9x-{{x}^{2}} $ 

$ \therefore f'\left( x \right)=-9-2x $ 

Now, $f'\left( x \right)=0$ gives $x=-\frac{9}{2}$ 

The real line is divided into two disjoint intervals $\left( -\infty ,-\frac{9}{2} \right)and\left( -\frac{9}{2},\infty  \right)$ by the point $x=-\frac{9}{2}$.

In interval $\left( -\infty ,-\frac{9}{2} \right)$ i.e., for $x<\frac{9}{2},$ 

$\therefore f$  is strictly increasing for $x<\frac{9}{2}$

In interval $\left( \frac{9}{2},\infty  \right)$ i.e., for $x<-\frac{9}{2},f'\left( x \right)=-9-2x>0$ 

$\therefore f$  is strictly increasing for $x<-\frac{9}{2}$

In interval $\left( -\frac{9}{2},\infty  \right)$ i.e., for $x>-\frac{9}{2},f'\left( x \right)=-9-2x<0$ 

$\therefore f$  is strictly increasing for $x>-\frac{9}{2}$

e) Given that,

$ f\left( x \right)={{\left( x+1 \right)}^{3}}{{\left( x-3 \right)}^{3}} $ 

$ f'\left( x \right)=3x{{\left( x+1 \right)}^{2}}{{\left( x-3 \right)}^{3}}+3{{\left( x-3 \right)}^{2}}{{\left( x+1 \right)}^{3}} $ 

$ =3{{\left( x+1 \right)}^{2}}{{\left( x-3 \right)}^{2}}\left[ x-3+x+1 \right] $ 

$ =6{{\left( x+1 \right)}^{2}}{{\left( x-3 \right)}^{2}}\left( x-1 \right) $ 

Now, 

$ f'\left( x \right)=0 $ 

$ \Rightarrow x=-1,3,1 $  

The points $x=-1,1,3$ divided the real line into four disjoint intervals. i.e., $\left( -\infty ,-1 \right),\left( -1,1 \right),\left( 1,3 \right)and\left( 3,\infty  \right)$ 

In intervals $\left( -\infty ,-1 \right)and\left( -1,1 \right),f'\left( x \right)=6{{\left( x+1 \right)}^{2}}{{\left( x-3 \right)}^{2}}\left( x-1 \right)<0$ 

$\therefore f$ is strictly decreasing in interval $\left( -\infty ,-1 \right)and\left( -1,1 \right)$ 

In intervals $\left( 1,3 \right)and\left( 3,\infty  \right),f'\left( x \right)=6{{\left( x+1 \right)}^{2}}{{\left( x-3 \right)}^{2}}\left( x-1 \right)>0$ 

$\therefore f$ is strictly decreasing in the interval $\left( 1,3 \right)and\left( 3,\infty  \right)$.

7. Show that $y=\log \left( 1+x \right)-\frac{2x}{2+x},x>-1,$ is an increasing function of 

$x$ throughout its domain.

Ans: We are given that,

$ y=\log \left( 1+x \right)-\frac{2x}{2+x} $ 

$ \therefore \frac{dy}{dx}=\frac{1}{1+x}-\frac{\left( 2+x \right)\left( 2 \right)-2x\left( 1 \right)}{{{\left( 2+x \right)}^{2}}} $ 

 $ =\frac{{{x}^{2}}}{{{\left( 2+x \right)}^{2}}} $ 

Now,

$ \frac{dy}{dx}=0 $ 

 $ \Rightarrow \frac{{{x}^{2}}}{{{\left( 2+x \right)}^{2}}}=0 $ 

 $ \Rightarrow {{x}^{2}}=0 $ 

$ \Rightarrow x=0 $  

Since $x>-1$ point $x=0$ divides the domain $\left( -1,\infty  \right)$ in two disjoint 

intervals i.e., $-1<x<0$ and $x>0.$ 

 When $-1<x<0$we have:

  $ x<0\Rightarrow {{x}^{2}}>0 $ 

 $ x>-1\Rightarrow \left( 2+x \right)>0 $ 

 $ \Rightarrow {{\left( 2+x \right)}^{2}}>0 $ 

 \[\therefore y=\frac{{{x}^{2}}}{{{\left( 2+x \right)}^{2}}}>0\]

Also, when $x>0$ 

 $ x<0\Rightarrow {{x}^{2}}>0, $ 

 $ {{\left( 2+x \right)}^{2}}>0 $ 

 $ \therefore y=\frac{{{x}^{2}}}{{{\left( 2+x \right)}^{2}}}>0 $ 

 Hence, the function $f$ is increasing throughout this domain.

8. Find the values of $x$ for which $y={{\left[ x\left( x-2 \right) \right]}^{2}}$ is an increasing function.

Ans: We have,         

  $ y={{\left[ x\left( x-2 \right) \right]}^{2}} $ 

 $ ={{\left[ {{x}^{2}}-2x \right]}^{2}} $ 

 $ \therefore \frac{dy}{dx}=2\left( {{x}^{2}}-2x \right)\left( 2x-2 \right) $ 

 $ =4x\left( x-2 \right)\left( x-1 \right) $ 

 $ \therefore \frac{dy}{dx}=0 $ 

 $ \Rightarrow x=0,1,2 $ 

The points $x=0,1,2$ divide the real line into four disjoint intervals i.e., 

$\left( -\infty ,0 \right),\left( 0,1 \right),\left( 1,2 \right)and\left( 2,\infty  \right).$ 

In intervals $\left( -\infty ,0 \right)and\left( 1,2 \right),\frac{dy}{dx}<0$ 

$\therefore y$ is strictly decreasing in intervals $\left( -\infty ,0 \right)and\left( 1,2 \right)$ 

However, in intervals $\left( 0,1 \right)and\left( 2,\infty  \right)$, $\frac{dy}{dx}>0$ 

$\therefore y$ is strictly decreasing in intervals $\left( 0,1 \right)and\left( 2,\infty  \right)$

$\therefore y$ is strictly decreasing in intervals $0<x<1$  and $x>2$.

9. Prove that $y=\frac{4\sin \theta }{\left( 2+\cos \theta  \right)}-\theta $ is an increasing function of $\theta $ in $\left[ 0,\frac{\pi }{2} \right]$.

Ans: We have,

 $ y=\frac{4\sin \theta }{\left( 2+\cos \theta  \right)}-\theta  $ 

 $ \therefore \frac{dy}{d\theta }=\frac{\left( 2+\cos \theta  \right)\left( 4\cos \theta  \right)-4\sin \theta \left( -\sin \theta  \right)}{{{\left( 2+\cos \theta  \right)}^{2}}}-1 $ 

 $ =\frac{8\cos \theta +4{{\cos }^{2}}\theta +4{{\sin }^{2}}\theta }{{{\left( 2+\cos \theta  \right)}^{2}}}-1 $ 

 $ =\frac{8\cos \theta +4}{{{\left( 2+\cos \theta  \right)}^{2}}}-1 $ 

Now,           

$ \frac{dy}{d\theta }=0 $ 

$ \Rightarrow \frac{8\cos \theta +4}{{{\left( 2+\cos \theta  \right)}^{2}}}=1 $ 

$ \Rightarrow 8\cos \theta +4=4+{{\cos }^{2}}\theta +4\cos \theta  $ 

$ \Rightarrow {{\cos }^{2}}\theta -4\cos \theta =0 $ 

$ \Rightarrow \cos \theta =0,4 $ 

Since, 

$ \cos \theta \ne 4, $ 

$ \cos \theta =0 $ 

$ \Rightarrow \theta =\frac{\pi }{2} $ 

Now, $\frac{dy}{d\theta }=\frac{\cos \left( 4-\cos \theta  \right)}{{{\left( 2+\cos  \right)}^{2}}}$ 

In the interval $\left[ 0,\frac{\pi }{2} \right]$, we have $\cos \theta >0$,

Also, $4>\cos \theta \Rightarrow 4-\cos \theta >0$ 

$\therefore \cos \theta \left( 4-\cos \theta  \right)>0$ and also ${{\left( 2+\cos \theta  \right)}^{2}}>0$ 

$ \Rightarrow \frac{\cos \theta \left( 4-\cos \theta  \right)}{{{\left( 2+\cos \theta  \right)}^{2}}}>0 $ 

$ \Rightarrow \frac{dy}{dx}>0 $  

Therefore $y$ is strictly increasing in the interval $\left( 0,\frac{\pi }{2} \right)$ 

Hence, $y$ is increasing in the interval $\left( 0,\frac{\pi }{2} \right)$.

10. Prove that the logarithmic function is strictly increasing on $\left( 0,\infty  \right)$.

Ans: The given function is $f\left( x \right)\log x$.

\[f'\left( x \right)=\frac{1}{x}\] 

It is clear that for $x>0,f'\left( x \right)=\frac{1}{x}>0$ 

Hence, $f\left( x \right)\log x$ is strictly increasing in the interval $\left( 0,\infty  \right)$.

11. Prove that the function $f$ is given by $f\left( x \right)={{x}^{2}}-x+1$ is neither strictly increasing nor strictly decreasing on $\left( -1,1 \right)$.

Ans: The given function is $f\left( x \right)={{x}^{2}}-x+1$ 

$\therefore f'\left( x \right)=2x-1$ 

Now,

$ f'\left( x \right)=0 $ 

 $ \Rightarrow x=\frac{1}{2}. $ 

The point $\frac{1}{2}$ divides the interval $\left( -1,1 \right)$ into two disjoint intervals i.e.,    

$\left( -1,\frac{1}{2} \right)and\left( \frac{1}{2},1 \right).$ 

Now, in the interval $\left( -1,\frac{1}{2} \right),f'\left( x \right)=2x-1<0$ 

Therefore, $f$ is strictly decreasing in the interval $\left( -1,\frac{1}{2} \right)$,

However, in the interval $\left( \frac{1}{2},1 \right),f'\left( x \right)=2x-1>0$     

Therefore, $f$ is strictly decreasing in the interval $\left( \frac{1}{2},1 \right)$,

Hence, $f$ is neither strictly increasing nor decreasing in interval 

$\left( -1,1 \right)$.

12. Which of the following functions are strictly decreasing on $\left( 0,\frac{\pi }{2} \right)$?

(A) \[\mathbf{cosx}\] (B) $\cos 2x$  (C) $\cos 3x$  (D) $\tan x$ 

 Ans. 

A) Let ${{f}_{1}}\left( x \right)=\cos x$ 

$\therefore {{f}_{1}}^{\prime }\left( x \right)=-\sin x$ 

In interval, $\left( 0,\frac{\pi }{2} \right),{{f}_{1}}^{\prime }\left( x \right)=-\sin x<0$ 

$\therefore {{f}_{1}}\left( x \right)=\cos x$ is strictly decreasing in the interval $\left( 0,\frac{\pi }{2} \right)$.

B) Let ${{f}_{2}}\left( x \right)=\cos 2x$ 

$\therefore {{f}_{2}}^{\prime }\left( x \right)=-2\sin 2x$ 

Now,

$ 0<x<\frac{\pi }{2}\Rightarrow 0<2x<\pi  $ 

$ \Rightarrow \sin 2x>0\Rightarrow -2\sin 2x<0 $ 

$\therefore {{f}_{2}}^{\prime }\left( x \right)=-2\sin 2x<0$  on $\left( 0,\frac{\pi }{2} \right)$ 

$\therefore {{f}_{2}}\left( x \right)=\cos 2x$ is strictly decreasing in interval $\left( 0,\frac{\pi }{2} \right)$$$ 

C) Let ${{f}_{3}}\left( x \right)=\cos 3x$ 

$\therefore {{f}_{3}}^{\prime }\left( x \right)=-3\sin 3x$ 

Now,

  $ {{f}_{3}}^{\prime }\left( x \right)=0 $ 

 $ \Rightarrow \sin 3x=0 $ 

 $ \Rightarrow 3x=\pi ,x\in \left( 0,\frac{\pi }{2} \right) $ 

 $ \Rightarrow x=\frac{\pi }{3} $ 

The point $x=\frac{\pi }{3}$  divides the interval $\left( 0,\frac{\pi }{2} \right)$  into two disjoint intervals i.e., $\left( 0,\frac{\pi }{3} \right)$  and $\left( \frac{\pi }{3},\frac{\pi }{2} \right)$.

Now, in the interval $\left( 0,\frac{\pi }{3} \right)$, ${{f}_{3}}\left( x \right)=-3\sin 3x<0\left[ 0<x<\frac{\pi }{3}\Rightarrow 0<3x<\pi  \right]$ 

$\therefore {{f}_{3}}$ is strictly decreasing in interval x$\left( 0,\frac{\pi }{3} \right)$ 

However, in the interval $\left( \frac{\pi }{3},\frac{\pi }{2} \right)$, ${{f}_{3}}\left( x \right)=-3\sin 3x>0\left[ \frac{\pi }{3}<x<\frac{\pi }{2}\Rightarrow \pi <3x<\frac{3\pi }{2} \right]$ 

$\therefore {{f}_{3}}$ is strictly increasing in the interval $\left( \frac{\pi }{3},\frac{\pi }{2} \right)$ 

Hence, ${{f}_{3}}$ is neither increasing nor decreasing in interval $\left( 0,\frac{\pi }{2} \right)$

D) Let ${{f}_{4}}\left( x \right)=\tan x$ 

$\therefore {{f}_{4}}^{\prime }\left( x \right)={{\sec }^{2}}x$ 

In interval $\left( 0,\frac{\pi }{2} \right)$,${{f}_{4}}^{\prime }\left( x \right)={{\sec }^{2}}x>0$ 

\[∴f_4\] is strictly increasing in the interval $\left( 0,\frac{\pi }{2} \right)$.

Therefore, functions $\cos x$and $\cos 2x$  are strictly decreasing in $\left( 0,\frac{\pi }{2} \right)$.

Hence, the correct options are A and B.

13. On which of the following intervals is the function $f$ is given by 

$f\left( x \right)={{x}^{100}}+\sin x-1$ strictly decreasing?

A. $\left( 0,1 \right)$ 

B. $\left( \frac{\pi }{2},\pi  \right)$ 

C. $\left( 0,\frac{\pi }{2} \right)$ 

D. None of these

Ans: We have,

$ f\left( x \right)={{x}^{100}}+\sin x-1 $ 

$ \therefore {f}'\left( x \right)=100{{x}^{90}}+\cos x $ 

In interval $\left( 0,1 \right),\cos x>0$ and $100{{x}^{90}}>0$ 

$\therefore f'\left( x \right)>0$ 

Thus, the function $f$ is strictly increasing in the interval $\left( 0,1 \right)$.

In interval $\left( \frac{\pi }{2},\pi  \right),\cos x<0$  and \[\text{100}{{\text{x}}^{90}}>0\].

Also, $100{{x}^{90}}>\cos x$ 

$\therefore f'\left( x \right)>0in\left( \frac{\pi }{2},\pi  \right)$ 

Thus, the function $f$ is strictly increasing in the interval $\left( \frac{\pi }{2},\pi  \right)$.

In the interval $\left( 0,\frac{\pi }{2} \right)$, $\cos x<0$ and $100{{x}^{90}}>0$ 

$ \therefore 100{{x}^{90}}+\cos x>0 $ 

$ \Rightarrow f'\left( x \right)>0on\left( 0,\frac{\pi }{2} \right) $ 

$\therefore f$ is strictly increasing in the interval $\left( 0,\frac{\pi }{2} \right)$

Hence, the function $f$ is strictly decreasing in none of the intervals. The correct option is D.

14. Find the least value of $a$ such that the function $f$ given 

$f\left( x \right)={{x}^{2}}+ax+1$ is strictly increasing on $\left( 1,2 \right)$.

Ans: We have,

$ f\left( x \right)={{x}^{2}}+ax+1 $ 

$ \therefore {f}'\left( x \right)=2x+a $ 

Now, the function $f$ will be increasing in $\left( 1,2 \right)$ if ${f}'\left( x \right)>0$ in $\left( 1,2 \right)$.

  $ \Rightarrow 2x+a>0 $ 

 $ \Rightarrow 2x>-a $ 

 $ \Rightarrow x>\frac{-a}{2} $ 

 As a result, we must determine the smallest value of $a$ such that 

 $x>\frac{-a}{2},x\in \left( 1,2 \right)$ 

 $\Rightarrow x>\frac{-a}{2}\left( 1<x<2 \right)$ 

 Thus, the least value is given by

  $ \frac{-a}{2}=1 $ 

 $ \Rightarrow a=-2 $ 

Hence, the required value of $a$ is $-2$.

15. Let I be any interval disjoint from $\left( -1,1 \right)$, prove that the function $f$ given by $f\left( x \right)=x+\frac{1}{x}$ is strictly increasing on I.

Ans: We have,

$ f\left( x \right)=x+\frac{1}{x} $ 

 $ \therefore {f}'\left( x \right)=1-\frac{1}{{{x}^{2}}} $ 

Now, 

$ f\left( x \right)=0 $ 

$ \Rightarrow x=\pm 1 $ 

The real line is divided into three disjoint intervals i.e., 

$\left( -\infty ,-1 \right),\left( -1,1 \right),\left( 1,\infty  \right)$ by the points $x=1,-1$.

In the interval $\left( -1,1 \right)$ we observe,

$ -1<x<1 $ 

$ \Rightarrow {{x}^{2}}<1 $ 

$ \Rightarrow 1<\frac{1}{{{x}^{2}}},x\ne 0 $ 

$ \therefore f'\left( x \right)=1-\frac{1}{{{x}^{2}}}<0on\left( -1,1 \right)\sim \left\{ 0 \right\}. $ 

$\therefore f$ is strictly decreasing on $\left( -1,1 \right)\sim \left\{ 0 \right\}$.

In the interval, $\left( -\infty ,-1 \right)and\left( 1,\infty  \right),$ it is observed that:

$ x<-1 $ 

$ \Rightarrow {{x}^{2}}>1 $ 

$ \Rightarrow 1>\frac{1}{{{x}^{2}}} $ 

$ \Rightarrow 1-\frac{1}{{{x}^{2}}}>0 $ 

$\therefore f'\left( x \right)=1-\frac{1}{{{x}^{2}}}>0$  on $\left( -\infty ,-1 \right)and\left( 1,\infty  \right).$

$\therefore f$ is strictly increasing on $\left( -\infty ,-1 \right)and\left( 1,\infty  \right).$

Hence, the function $f$is strictly increasing in the interval I disjoint  

from $\left( -1,1 \right)$.

Hence, the given result is proved.

16. Prove that the function $f$ given by $f\left( x \right)=\log \sin x$ is strictly increasing on $\left( 0,\frac{\pi }{2} \right)$ and strictly decreasing on $\left( \frac{\pi }{2},\pi  \right)$.

Ans: We have,

$ f\left( x \right)=\log \sin x $ 

 $ \therefore f'\left( x \right)=\frac{1}{\sin x}\cos x=\cot x $ 

In interval, $\left( 0,\frac{\pi }{2} \right),f'\left( x \right)=\cot x>0$ 

$\therefore f$ is strictly increasing in $\left( 0,\frac{\pi }{2} \right)$ 

In interval, $\left( \frac{\pi }{2},\pi  \right),f'\left( x \right)=\cot x<0$ 

$\therefore f$ is strictly increasing in $\left( \frac{\pi }{2},\pi  \right)$.

17. Prove that the function $f$ is given by $f\left( x \right)=\log \cos x$ is strictly decreasing on $\left( 0,\frac{\pi }{2} \right)$ and strictly increasing on $\left( \frac{\pi }{2},\pi  \right)$.

Ans: We have,

$ f\left( x \right)=\log \cos x $ 

$ \therefore f'\left( x \right)=\frac{1}{\cos x}\left( -\sin x \right)=-\tan x $ 

In interval $\left( 0,\frac{\pi }{2} \right)$,

$ \tan x>0\Rightarrow -\tan x<0 $ 

$ \therefore f'\left( x \right)<0on\left( 0,\frac{\pi }{2} \right) $ 

$\therefore f$ is strictly decreasing on $\left( 0,\frac{\pi }{2} \right)$ 

In interval $\left( \frac{\pi }{2},\pi  \right)$,

$ \tan x<0\Rightarrow -\tan x>0 $ 

$ \therefore f'\left( x \right)>0on\left( \frac{\pi }{2},\pi  \right) $ 

$\therefore f$ is strictly decreasing on $\left( \frac{\pi }{2},\pi  \right)$. 

18. Prove that the function given by $f\left( x \right)={{x}^{3}}-3{{x}^{2}}+3x=100$ is increasing in R.

Ans: We have,

$ f\left( x \right)={{x}^{3}}-3{{x}^{2}}+3x=100 $ 

$ f'\left( x \right)=3{{x}^{2}}-6x+3 $ 

$ =3\left( {{x}^{2}}-2x+1 \right) $ 

$ =3{{\left( x-1 \right)}^{2}} $ 

For any $x\in R$ 

${{\left( x-1 \right)}^{2}}>0$ 

Thus, $f'\left( x \right)$ is always positive in R.

Hence, the given function $f$ is increasing in R.

19. The interval in which $y={{x}^{2}}{{e}^{-x}}$ is increasing is

A) $\left( -\infty ,\infty  \right)$ 

B) $\left( -2,0 \right)$ 

C) $\left( 2,\infty  \right)$ 

D) $\left( 0,2 \right)$ 

Ans: We have,        

$ y={{x}^{2}}{{e}^{-x}} $ 

 $ \therefore \frac{dy}{dx}=2x{{e}^{-x}}-{{x}^{2}}{{e}^{-x}}=x{{e}^{-x}}\left( 2-x \right) $ 

Now,

$ \frac{dy}{dx}=0 $ 

 $ \Rightarrow x=0,2 $ 

 The points $x=0,2$ divided the real line into the three disjoint intervals 

 i.e., $\left( -\infty ,0 \right),\left( 0,2 \right),\left( 2,\infty  \right).$ 

 In intervals $\left( -\infty ,0 \right)and\left( 2,\infty  \right),f'\left( x \right)<0$ as ${{e}^{-x}}$ is always positive.

 $\therefore f$ is decreasing on $\left( -\infty ,0 \right)and\left( 2,\infty  \right)$.

 In interval $\left( 0,2 \right),f'\left( x \right)>0$ 

 $\therefore f$is strictly increasing on $\left( 0,2 \right)$.

Hence $f$ is strictly increasing in the interval $\left( 0,2 \right)$

Thus, D is the correct option.

Conclusion

In conclusion, Class 12 Maths Exercise 6.2 serves as a valuable practice ground for wielding derivatives to analyze the monotonic behavior of functions. By working through these problems, Students will solidify their understanding of how a function's rate of change (represented by the derivative) dictates its increasing or decreasing nature across different intervals. Ex 6.2 Class 12 Maths NCERT Solutions equips students to move beyond simply calculating derivatives and leverage them to gain deeper insights into the characteristics of functions - a crucial skill for higher mathematics and various scientific applications.

Class 12 Maths Chapter 6: Exercises Breakdown

CBSE Class 12 Maths Chapter 6 Other Study Materials

NCERT Solutions for Class 12 Maths | Chapter-wise List

Given below are the chapter-wise NCERT 12 Maths solutions PDF. Using these chapter-wise class 12th maths ncert solutions, you can get clear understanding of the concepts from all chapters.

Related Links for NCERT Class 12 Maths in Hindi

Explore these essential links for NCERT Class 12 Maths in Hindi, providing detailed solutions, explanations, and study resources to help students excel in their mathematics exams.

Important Related Links for NCERT Class 12 Maths