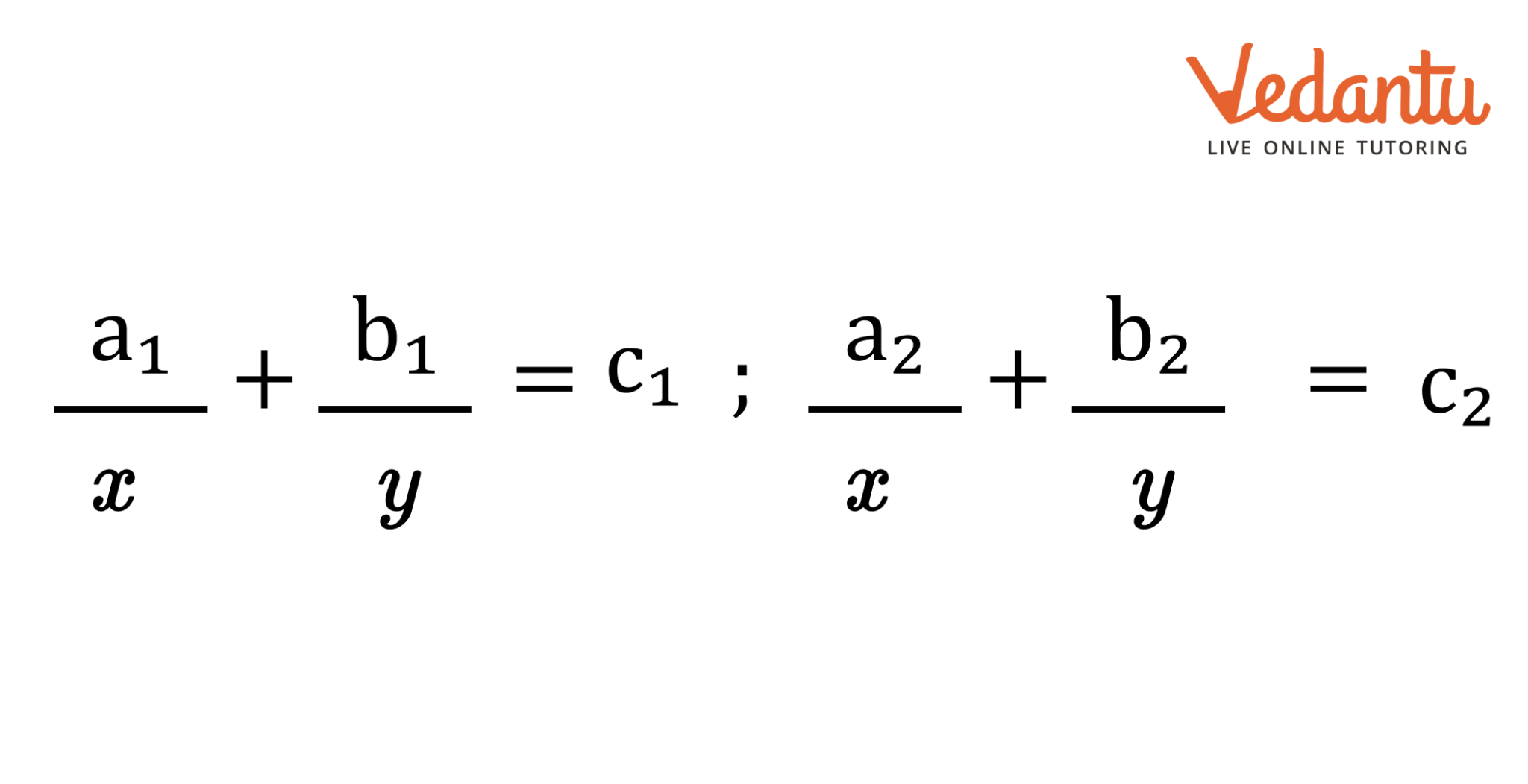NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables - Free PDF
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations In Two Variables



FAQs on NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations In Two Variables
1. Where can I get the Best NCERT Maths Class 10 Chapter 3 Solutions Online?
You can get the best NCERT Solutions for class 10 chapter 3 on Vedantu for free download in the form of a PDF. Subject experts prepare the solutions to let students get maximum benefits of it. Also, every answer is explained with detailed step-wise explanations so students can refer to them to clear their doubts. All the material is prepared by our subject-matter experts keeping in mind the new syllabus.
2. How Many Solutions can you Find for a Linear Equation in Two Variables?
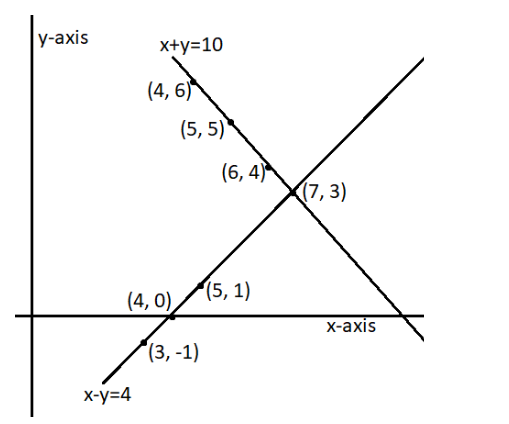
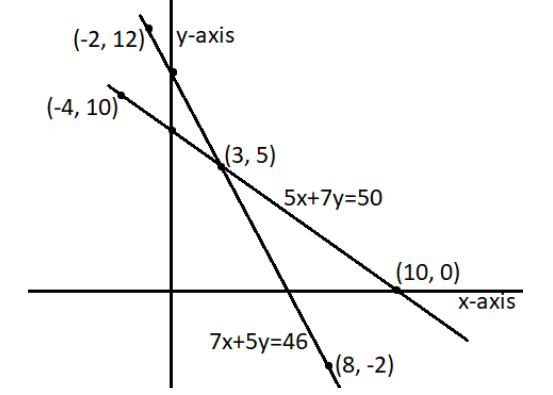
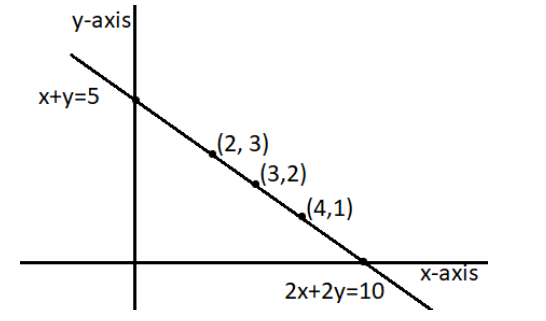
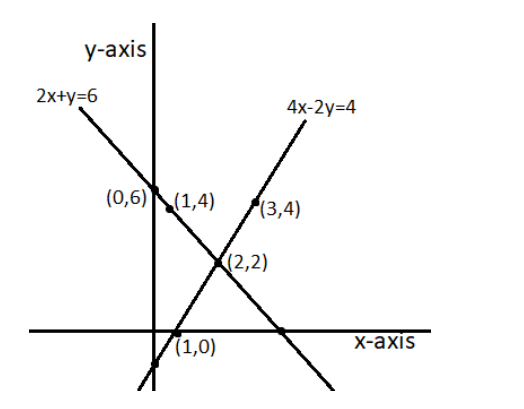
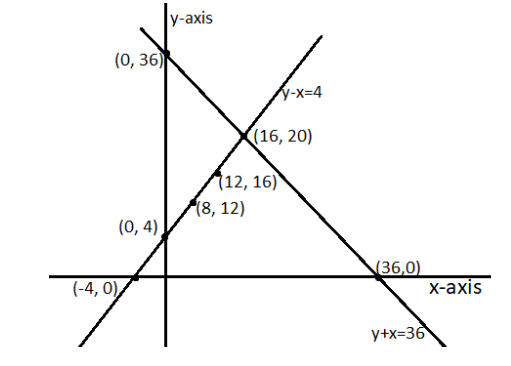
A linear equation in two variables, it can be represented in the form of a line on the graph. A line is endless, and hence it can have infinitely many solutions. Each solution of the linear equation in two variables will be uniquely identified and plotted on the graph. Each point on the line will be the solution to the linear equation defining that line. Thus there is no end to the solutions of a linear equation in two variables. Suppose if we have two different lines of linear equation, then those either intersect, coincide, or are parallel to each other.
3. How many exercises are there in Class 10 Maths Chapter 3?
NCERT Class 10 Maths Chapter 3 is Pair of Linear Equations in Two Variables. This chapter has a total of seven exercises. Vedantu offers solutions for all the exercises. The NCERT Solutions for Class 10 Maths Chapter 3 have been created by subject matter experts. You need to download them in PDF format so that you can study them anytime and anywhere.
4. What is a Linear Equation in Two variables Class 10?
A Linear equation in Two variables is written in the form of ax + by + c=0. In this, a, b, and c are real numbers where c is a constant and a and b are the coefficients of x and y, respectively. It is important that a and b should not be zero.
Examples of Linear equations in Two variables are x+ 2y = 14, 10x - 8y = 7, etc. Linear equations in Two variables have two values as a solution, one for x and the other for y. Vedantu has explained these concepts in detail and provided the solutions to exercises from NCERT textbooks.
5. What are the most important questions of 10th Maths Chapter 3?
Here are some important questions of Class 10th Maths Chapter 3 - Pair of Linear Equations in Two Variables:
Linear Equations
Forms of Linear Equations
The Standard Form of Linear Equations
Solving Linear Equations with One Variable
Solving Linear Equations with Two Variables
You can find the solutions to all the exercises of 10th Maths Chapter 3 in Vedantu’s NCERT Solutions for Class 10 Maths. All the questions present there are accurately answered, and the concepts are properly explained.
6. How can I download the Class 10 Maths Chapter 3 solutions?
Class 10 Maths Chapter 3, A Linear Equation in Two variables, is one of the scoring chapters. If you want to get the solutions for this chapter, you can visit the official website of Vedantu. We offer the NCERT Solutions for Class 10 Maths in PDF format so that you can download them for offline use. It will allow you to study them anywhere and at any time. You can also download the solutions from the Vedantu App. All you have to do is download the app from Google play store, follow the instructions to sign in, and download the study material. The best part is that all these solutions are available for free.
7. How do you solve Linear Equations in Class 10?
Following are the different methods of solving Linear Equations:
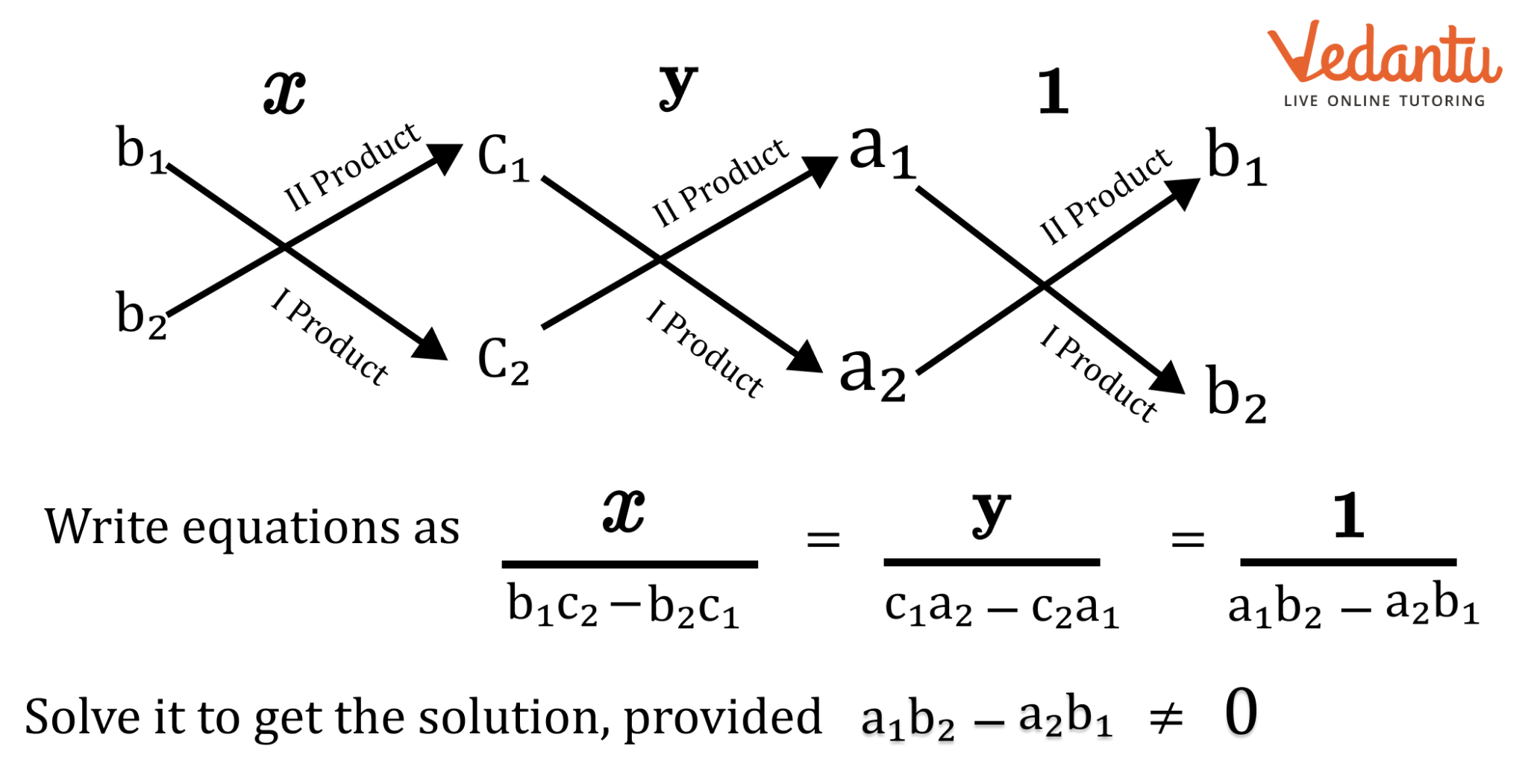
Cross multiplication method
Substitution method
Elimination method
Graphical method
8. What are the topics covered in class 10 chapter 3 maths?
The topics covered in class 10 chapter 3 maths from Pair Of Linear Equations In Two Variables are:
Basics of linear equations: This lays the foundation, defining linear equations and introducing the concept of solving them in pairs.
Graphical method: You'll learn how to visualize the solutions of a pair of equations by graphing them.
Algebraic methods: Here, you'll explore different techniques to solve linear equations algebraically, including:
Elimination method
Substitution method
Cross-multiplication method
Applications: You'll learn how to use these methods to solve real-world problems that can be modeled by a pair of linear equations.
9. What is the formula of the substitution method?
The substitution method is a methodical approach to solving a system of two linear equations. Nevertheless, it lacks a single formula. This is how the process is broken down:
Express one variable in terms of the other: In one of the equations, rearrange the equation to isolate (solve for) one variable (x or y) in terms of the other variable.
Take the expression you just got for that variable and plug it into the other equation. This replaces that variable entirely with the expression you obtained.
Solve for the remaining variable: Now you have a single equation with just one variable remaining. Solve this equation like you would normally solve any linear equation.
Solve for the first variable: Once you find the value of the remaining variable, plug it back into the equation you isolated it from in step 1. Now you can solve for the first variable's value.
Substitute both your solutions (the values of x and y) back into the original equations. If both sides evaluate to the same value, then your solution is correct.
10. What is the name of Chapter 3 Maths Class 10?
Pair of Linear Equations in Two Variables is the title of Maths Class 10 Chapter 3. For this chapter, most curricula use this standard name. It appropriately conveys the material, which is on comprehending and resolving equation systems with two unknowns denoted by variables.
11. What is another name for the substitution method?
In the context of solving linear equations, the substitution method is the most commonly used word, but it lacks a commonly accepted replacement name.
It may be referred to as the replacement method in some sources. This highlights the fact that an expression comprising the other variable is being used in place of one variable.
But in mathematics teaching, "substitution method" is the accepted and commonly used phrase.
12. What is the main goal of the substitution method?
Converting a system of two linear equations with two variables into a single equation with one variable is the primary objective of the substitution method.
Finding an Isolated Variable: To begin, examine both equations and attempt to find an isolated variable (x or y) in relation to the other variable in one of the equations.This could require simple algebraic operations.
Replacing the Expression: After a variable has been identified, you take that expression in its entirety and replace it in the other equation.This just substitutes the expression you got in step 1 for one variable.
Finding the Single Variable: At this point, there is just one variable left in your equation.To determine the value of that variable, solve this equation just like you would any other single-variable problem.
Identifying the Additional Variable: You re-insert the value of one variable into the original equations (where the substitution was not done).You may now solve for the other variable as a result.
13. Does Maths Class 10 Chapter 3 NCERT Solutions require knowledge of all three approaches in order to solve pairs of linear equations in two variables?
Yes, mastering all three techniques is required in order to solve two linear equations in two variables found in NCERT Solutions for Class 10 Maths Chapter 3. These subjects will be covered in further education and might even come up in their Class 10 final exams. BYJU'S does a fantastic job of explaining these ideas. Therefore, the primary goal of these solutions developed by BYJU'S experts is to impart information on the foundational ideas of mathematics, which helps students grasp each idea with clarity.























 Watch Video
Watch Video