NCERT Solutions for Class 6 Chapter 8 Maths - FREE PDF Download
Vedantu provides NCERT Solutions for Class 6 Maths Chapter 8, "Playing with Constructions," making it easy for students to learn this important topic engagingly. This chapter focuses on understanding geometric constructions, including using a compass and straightedge, along with practical examples that demonstrate these concepts. Students can follow along with clear explanations and visual aids, making maths fun and easy to understand.
 Table of Content
Table of ContentThese solutions align with the CBSE Class 6 Maths Syllabus, ensuring that students cover the essential material needed for their grades. With step-by-step guidance, Class 6 Maths NCERT Solutions helps students practise and understand the concepts of constructions effectively. Download the FREE PDF to help students use geometric constructions in real-life situations and improve their math skills.
Glance on Class 6 Maths Chapter 8 - Playing with Constructions
The chapter introduces the concept of geometric constructions, explaining the use of a compass and straightedge in detail.
It covers how to create basic shapes like circles, triangles, and squares, providing clear methods for performing each construction step.
Students learn to apply constructions in various contexts, enhancing their problem-solving and spatial skills.
The chapter includes real-life examples to show how geometric constructions are used in everyday activities, such as drawing and building.
Various practice problems are provided to help students apply what they have learned and reinforce their understanding of constructions effectively.
Access Exercise Wise NCERT Solutions for Chapter 8 Maths Class 6
Exercises Under NCERT Solutions for Class 6 Maths Chapter 8 Playing with Constructions
NCERT Solutions for Chapter 8 Class 6 Maths, "Playing with Constructions," covers the following exercises:
Exercise 8.1: Artwork - This exercise introduces geometric constructions through art, where students learn to create shapes and designs using a compass and straightedge. It encourages creativity while applying mathematical concepts.
Exercise 8.2: Squares and Rectangles - Here, students learn how to construct squares and rectangles accurately. The exercise emphasizes understanding the properties of these shapes and the importance of precise measurements.
Exercise 8.3: Constructing Squares and Rectangles - This exercise provides step-by-step instructions for constructing squares and rectangles, reinforcing the techniques learned in previous exercises.
Exercise 8.4: An Exploration in Rectangles - Students explore the properties of rectangles through constructions, helping them understand the relationship between length and width.
Exercise 8.5: Exploring Diagonals of Rectangles and Squares - This exercise focuses on constructing and understanding the diagonals of squares and rectangles, enhancing students' spatial reasoning.
Exercise 8.6: Points Equidistant from Two Given Points - In this exercise, students learn how to find points that are equidistant from two given points, applying their understanding of constructions in a practical context.
Access NCERT Solutions for Class 6 Maths Chapter 8 Playing with Constructions
Exercise 8.1
1. A Person
Draw a figure in the given form to depict “A person”. How will you draw this?
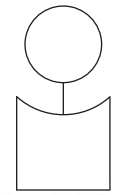
This figure has two components.
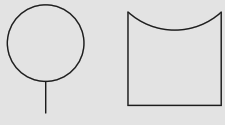
You might have figured out a way of drawing the first part. See this for drawing the second part.
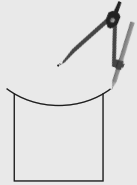
The challenge here is to find out where to place the tip of the compass and the radius to be taken for drawing this curve. You can fix a radius in the compass and try placing the tip of the compass in different locations to see which point works for getting the curve. Use your estimate to determine where to keep the tip.
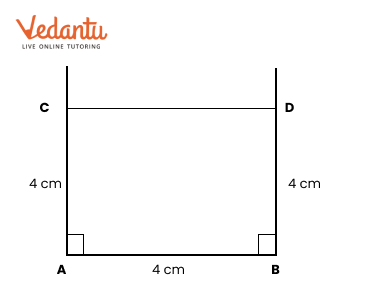
Ans: Step 1. We start with the base of the diagram. We take a line AB of length 4 cm as the base. (Fig. 1)
Step 2. At A and B, draw perpendicular lines using a protractor. (Fig. 2)

Step 3. Using a ruler, mark points C and D such that AC = 4 cm and BD = 4 cm. Join C and D by a line. (Fig. 3)
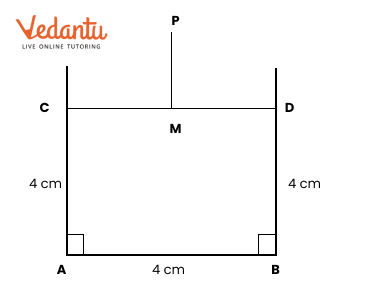
Step 4. Using a ruler, take point M on the CD such that CM = 2 cm. M is the midpoint of CD. Using a protractor, draw a perpendicular to CD at M. Take a point P on this perpendicular such that PM = 2 cm. Distance PM can also be slightly less than or greater than 2 cm. (Fig. 4)
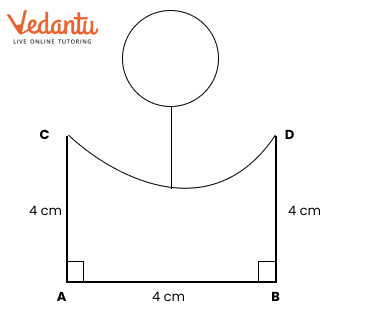
Step 5. Join PD. With the centre at P, draw an arc from D to C of a radius equal to PD. With the centre at P, draw a circle of radius 1.5 cm. Extend PM to touch the arc. (Fig. 5)

Step 6. Erase the extra lines in Fig. 5 as in Fig. 6.
Step 7. Fig. 6 represents the required depiction of the given “A person”.
Construct this.
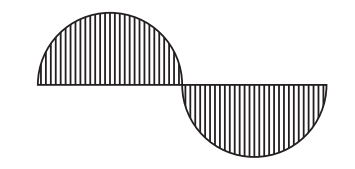
As the length of the central line is not specified, we can take it to be of any length. Let us take AB to be the central line such that the length of AB is 8 cm. We write this as
AB = 8 cm. Here, the first wave is drawn as a half circle.

1. What radius should be taken in the compass to get this half circle? What should be the length of AX?
Ans: We have AB = 8 cm.
Since the “Wavy Wave” has two equal half circles, we have AX = XB.
∴ X is the mid-point of AB.
∴ AX = $\frac{8}{2}= 4 cm$
∴ The length of AX is 4 cm.
Let M be the mid-point of AX.
∴ AM = MX = $\frac{8}{2}= 4 cm$
The centre of the half circle is M.
∴ Radius of half circle = AM = 2 cm
∴ The radius of the half circle is 2 cm.
2. Take a central line of a different length and try to draw the wave on it.
Ans: Step 1. We start with the central line of different lengths, say, 10 cm. (Figure 1)
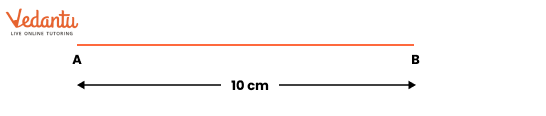
Step 2. Since
10 ÷ 2 = 5, using a ruler, take point C on AB such that AC = 5 cm. C is the mid-point of AB.
As 5 ÷ 2 = 2.5, using a ruler, take points D on AC and E on CB such that AD = 2.5 cm and CE = 2.5 cm.
D is the mid-point of AC and E is the mid-point of CB. (Figure 2)

Step 3. With the centre at D, draw a half circle above the central line AB and of radius 2.5 cm. With the centre at E, draw a half circle below the central line AB and of radius 2.5 cm. (Figure 3)

Step 4. Draw vertical lines in the half circles above and below the line AB. (Figure 4)

Step 5. The figure represents the required depiction of the given “Wavy Wave” with the central line of length 10 cm.
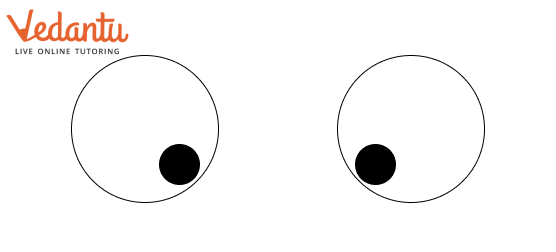
3. Eyes
How do you draw these eyes with a compass?
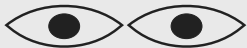
Draw a figure in the given form to depict “Eyes”.
Ans: Step 1. Take a line AB of length 8.5 cm (4 cm + 0.5 cm + 4 cm) as a base. Take points C and D on AB such that AC = 4 cm and AD = 4.5 cm (4 cm + 0.5 cm). (Fig. 1)

Step 2. Take points E and F on AB such that AE = 2 cm and FB = 2 cm. E is the mid-point of AC and F is the mid-point of DB. (Fig. 2)

Step 3. Using a protractor, draw perpendiculars at E and F. (Fig. 3)
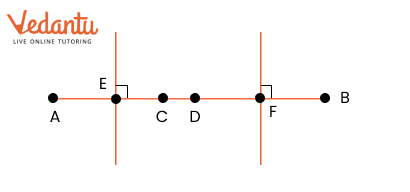
Step 4. Using a ruler, take points G, H, I, and J such that EG, EH, FI, and FJ are all equal to 1.5 cm. Equal distances can also be slightly less than or greater than 1.5 cm (Fig. 4)

Step 5. With the centre at G, draw an arc from A to C of a radius equal to AG. Similarly, with the centre at H, I, and J draw arcs of radius equal to AG. Erase the extra lines as shown in Fig. 5.
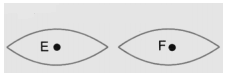
Step 6. At points E and F, draw two black dots of big size. (Fig. 6)

Step. 7. Figure represents the required depiction of “Eyes”.
Exercise 8.2
1. Draw the rectangle and four squares configuration (shown in Fig. 8.3) on a dot paper. What did you do to recreate this figure so that the four squares are placed symmetrically around the rectangle? Discuss with your classmates.
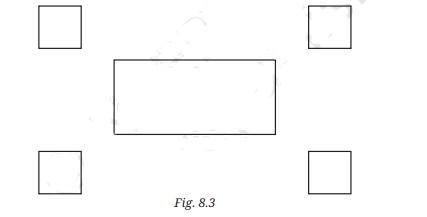
Ans:
Step 1. Take a square dot paper and mark a dot on it at A. Start from A move 10 dots to the right and mark the tenth dot at B.
Step 2. Start from B and move 6 dots above B and mark the 6th dot as C. Start from A and move 6 dots above A and mark the 6th dot as D. Join AB, BC, CD, and DA.
Step 3. Take points E, F, G, and H on the dot paper as shown in the figure.
Step 4. Take points I, J, K, and L at a distance of 4 dots from E, F, G, and H respectively. Join IE, FJ, GK, and LH.
Step 5. On LH and GK, construct squares above the rectangle.
Step 6. On IE and FJ, construct squares below the rectangle.

Step 7. The figure is the required configuration of one rectangle and four squares on a square dot paper.
2. Identify if there are any squares in this collection. Use measurements if needed.
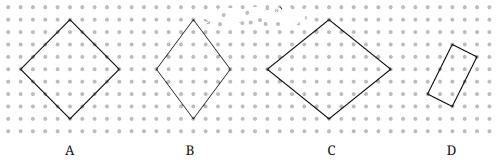
Think: Is it possible to reason out if the sides are equal or not, and if the angles are right or not without using any measuring instruments in the above figure? Can we do this by only looking at the position of corners in the dot grid?
Ans:
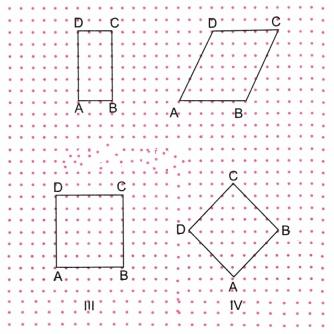
Fig. I: In this figure, AB and BC are not equal. So, ABCD cannot be a square.
Fig. II: In this figure, ∠BAD is not equal to 90°. So, ABCD cannot be a square.
Fig. III: In this figure, counting dots between sides, we find that AB, BC, CD, and DA are all equal sides. Also, the position of the dots on the sides shows, that each angle of ABCD is 90°.
∴ ABCD is a square.
Fig. IV: In this figure, counting dots between sides, we find that AB, BC, CD, and DA are all equal sides. Also, using a protractor, we find that each angle of ABCD is 90°.
∴ ABCD is a square.
3. Draw at least 3 rotated squares and rectangles on a dot grid. Draw them such that their corners are on the dots. Verify if the squares and rectangles that you have drawn satisfy their respective properties.
Ans: We draw two rotated squares and two rotated rectangles on a dot grid such that the comers of squares and rectangles are on dots.

We have drawn two rotated squares (II and III) and two rotated rectangles (I and IV). These squares and rectangles are drawn keeping in view the number of dots between sides and also the position of sides.
Using a protractor, we find that all angles of figures I to IV are 90°.
Using a ruler, we find that the opposite sides of Figures I and IV are equal and all sides of Figures II and III are equal.
∴ By definition, figures I and IV are rectangles, and figures II and III are squares.
Exercise 8.3
1. Draw a rectangle with sides of length 4 cm and 6 cm. After drawing, check if it satisfies both the rectangle properties.
Ans: We shall draw a rectangle of the form shown in Fig. 1.

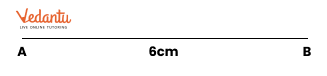
Step 1. Using a ruler, draw a line AB equal to 6 cm. (Fig. 2)
Step 2. Using a protractor, draw perpendicular lines at A and B (Fig. 3)

Step 3. Using a ruler, mark point P on the perpendicular line at A such that AP = 4 cm. Using a ruler, mark point Q on the perpendicular line at B such that BQ = 4 cm. (Fig. 4).

Step 4. Join P and Q using a ruler. Erase the lines above P and Q. (Fig. 5).
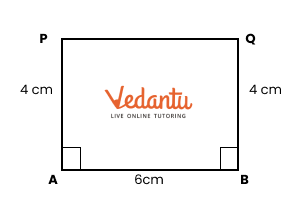
Step 5. Using a ruler, verify that PQ is of length 6 cm.
Using a protractor, verify that ∠P and ∠Q are 90° each.
Step 6. We have:
(i) AB = PQ = 6 cm and AP = BQ = 4 cm
(ii) ∠A = ∠B = ∠Q = ∠P = 90°.
Step 7. ABQP in Fig. 5 is the required rectangle of sides 4 cm and 6 cm.
2. Draw a rectangle of sides 2 cm and 10 cm. After drawing, check if it satisfies both the rectangle properties.
Ans: We shall draw a rectangle of the form shown in Fig. 1.

Step 1. Using a ruler, draw a line AB equal to 10 cm. (Fig. 2).
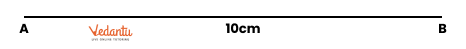
Step 2. Using a protractor, draw perpendicular lines at A and B (Fig. 3).
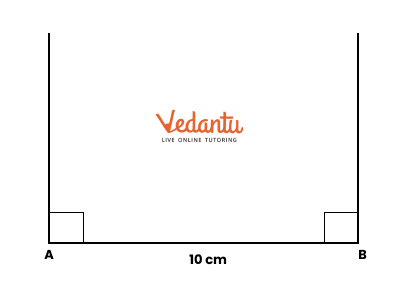
Step 3. Using a ruler, mark point P on the perpendicular at A such that AP = 2 cm. Using a ruler, mark point Q on the perpendicular at B such that BQ = 2 cm. (Fig. 4)
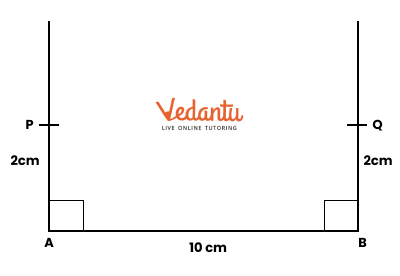
Step 4. Join P and Q using a ruler. Erase the lines above P and Q. (Fig. 5)

Step 5. Using a ruler, verify that PQ is of length 10 cm.
Using a protractor, verify that ∠P and ∠Q are 90° each.
Step 6. We have:
(i) AB = PQ = 10 cm and AP = BQ = 2 cm
(ii) ∠A = ∠B = ∠P = ∠Q = 90°.
Step 7. ABQP in Fig. 5 is the required rectangle of sides 2 cm and 10 cm.
3. Is it possible to construct a 4-sided figure in which—
• all the angles are equal to 90º but
• opposite sides are not equal?
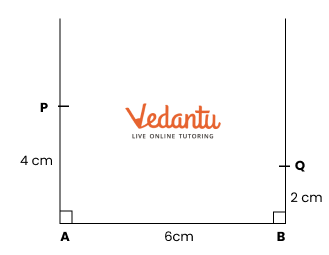
Ans: Step 1. Using a ruler, draw a line AB equal to 6 cm, say. (Fig. 1).

Step 2. Using a protractor, draw perpendicular lines at A and B (Fig. 2).

Step 3. Using a ruler, mark point P on the perpendicular at A such that AP = 4 cm.
Using a ruler, mark point Q on the perpendicular at B such that BQ = 2 cm, which is not equal to AP. (Fig. 3).
Step 4. In Fig. 3, the opposite sides of AP and BQ are not equal. Join P and Q using a ruler. Erase the lines above P and Q (Fig. 4).
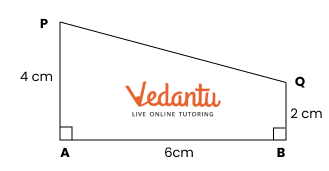
Step 5. Using a protractor, we find that neither ∠P nor ∠Q is 90°.
Step 6. We conclude that it is not possible to construct a 4-sided figure in which all angles are 90° and opposite sides are not equal.
Exercise 8.4
1. Breaking Rectangles
Construct a rectangle that can be divided into 3 identical squares.

Ans: We shall draw a rectangle of the form shown in Fig. 1.

Step 1. Let us keep the vertical side of the rectangle to 3 cm. Since the rectangle is to be divided into three identical squares, the length of the rectangle must be 3 cm + 3 cm + 3 cm = 9 cm.
Step 2. Using a ruler, draw a line AB equal to 9 cm. (Fig. 2).

Step 3. Using a ruler, find points P and Q on AB such that AP = 3 cm and PQ = 3 cm. Here, QB is also 3 cm. (Fig. 3).
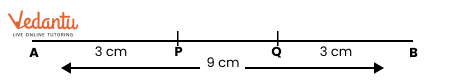
Step 4. Using a protractor, draw perpendicular lines at A, P, Q, and B. (Fig. 4).

Step 5. Using a ruler, mark points A’, P’, Q’, and B’ on perpendiculars at A, P, Q, and B respectively such that AA’ = PP’ = QQ’ BR’ = 3 cm. (Fig. 5).
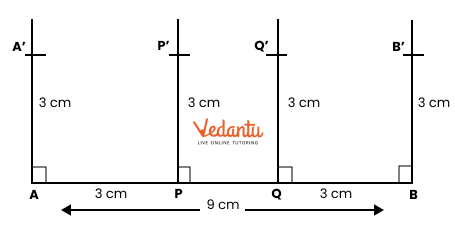
Step 6. Join A’ and P’, P’ and Q’, and Q’ and B’ using a ruler. Erase the lines above A’, P’, Q’, and B’. (Fig. 6).

Step 7. ABB’A’ is the required rectangle which is divided into 3 identical squares APP’A’, PQQ’P’, and QBB’Q’.
2. Give the lengths of the sides of a rectangle that cannot be divided into: (Page 201)
(i) Two identical squares
(ii) Three identical squares.
Ans: (i) Let the smaller side of a rectangle be x cm. If the larger side of the rectangle is 2x cm (x cm + x cm), then this rectangle can be divided into two identical squares of side x cm. (Fig. 1)
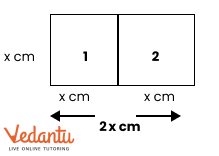
Let us consider a rectangle of sides 4 cm and 6 cm. Here, 6 is not equal to 8 (4 + 4), so, it cannot be divided into two identical squares as shown in Fig. 2
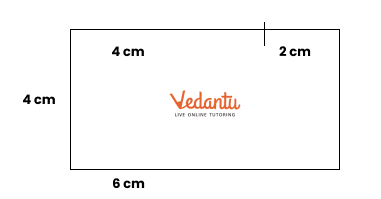
(ii) Let the smaller side of a rectangle be x cm. If the larger side of the rectangle is 3x cm (x cm + x cm + x cm), then this rectangle can be divided into three identical squares of side x cm. (Fig. 3)

Let us consider a rectangle of sides 3 cm and 8 cm. Here, 8 is not equal to 9 (3 + 3 + 3), so, it cannot be divided into three identical squares as shown in Fig. 4.

Figure it Out:
1. A Square within a Rectangle
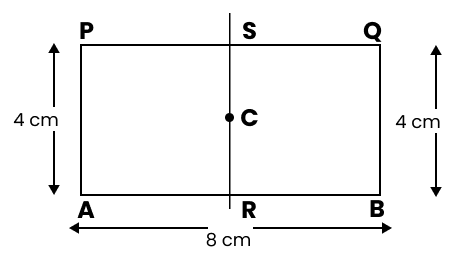
Construct a rectangle of sides 8 cm and 4 cm. How will you construct a square inside, as shown in the figure, such that the centre of the square is the same as the centre of the rectangle?
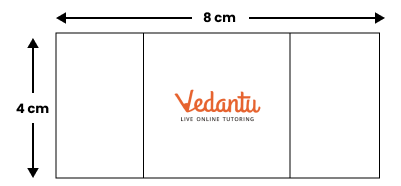
Hint: Draw a rough figure. What will be the sidelength of the square? What will be the distance between the corners of the square and the outer rectangle?
Ans: The centre of a rectangle (or square) is the point of intersection of its diagonals.
Step 1. Using a ruler, draw a line AB equal to 8 cm. Using a protractor, draw perpendicular lines at A and B. Using a ruler, mark point P on the perpendicular line at A such that AP = 4 cm. Using a ruler, mark point Q on the perpendicular line at B such that BQ = 4 cm. Join P and Q using a ruler. Erase the lines above P and Q. (Fig. 1)

Step 2. Draw diagonals AQ and BP, using a ruler. Let the diagonals intersect at C. This point is the centre of the rectangle ABQP and of the required square. (Fig. 2)
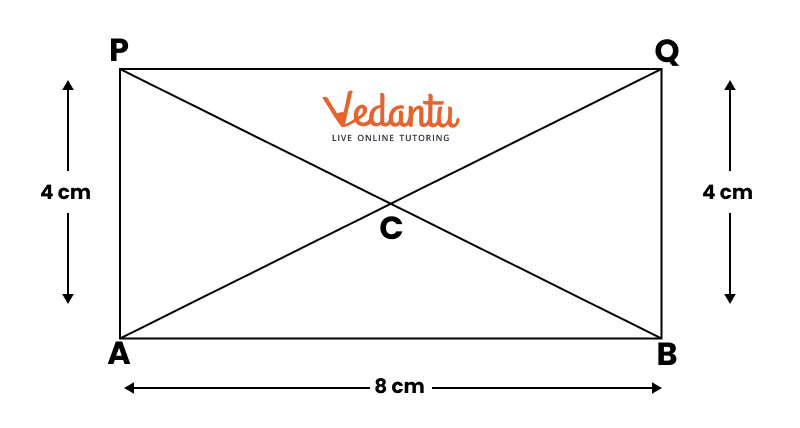
Step 3. Erase diagonals AQ and BP. Using a protractor, draw a perpendicular line on AB and pass through the centre C. Let this perpendicular meet AB at R and PQ at S. (Fig. 3).
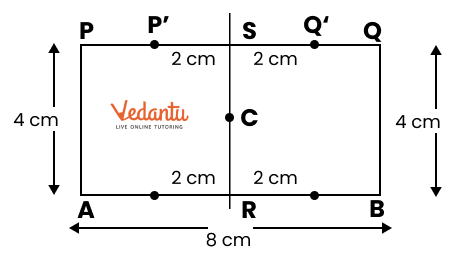
Step 4. Since AP = 4 cm, each side of the square must be 4 cm. Using a ruler, mark points A’ and B’ on AB such that A’R = 2 cm and RB’ = 2 cm. Thus, A’B’ = A’R + RB’ = 2 cm + 2 cm = 4 cm.
Similarly, using a ruler, mark points P’ and Q’ on PQ such that P’S = 2 cm and SQ’ = 2 cm. Thus, P’Q’ = P’S + SQ’ = 2 cm + 2 cm = 4 cm. (Fig. 4).
Step 5. Using a ruler, join A’ and P’ and also B’ and Q’. Erase the line RS. (Fig. 5).
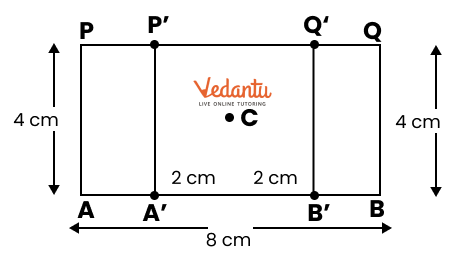
Step 6. In Fig. 5, A’B’Q’P’ is the required square with centre C, which is also the centre of the given rectangle.
2. Falling Squares
Construct the ‘Falling Squares” figure shown below:

Make sure that the squares are aligned the way they are shown. Now, try this.
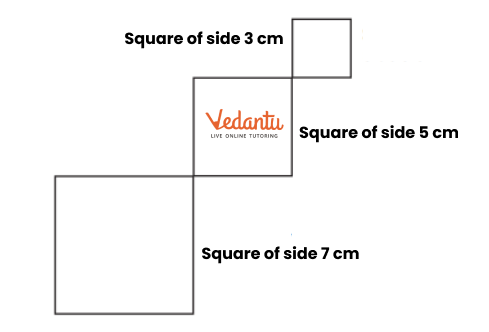
Ans: In the given figure, there are three falling squares and the side of each square is 4 cm.
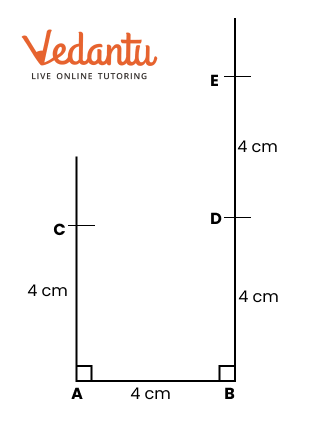
Step 1. Using a ruler, draw a line AB equal to 4 cm. Using a protractor, draw perpendicular lines at A and B.
Using a ruler, mark point C on a perpendicular line at A such that AC = 4 cm.
Using a ruler, mark points D and E on a perpendicular line at B such that BD = 4 cm and DE = 4 cm. (Fig. 1).
Step 2. Join C and D. Produce CD to F such that DF = 4 cm. Using a protractor, draw a perpendicular line at F. Using a ruler, mark points G and H on a perpendicular line at F such that FG = 4 cm and GH = 4 cm. (Fig. 2).

Step 3. Join E and G. Produce EG to I such that GE = 4 cm. Using a protractor, draw a perpendicular line at I. Using a ruler, mark point J on the perpendicular line at I such that IJ = 4 cm. Join H and J. Erase extra lines in the figure. (Fig. 3).
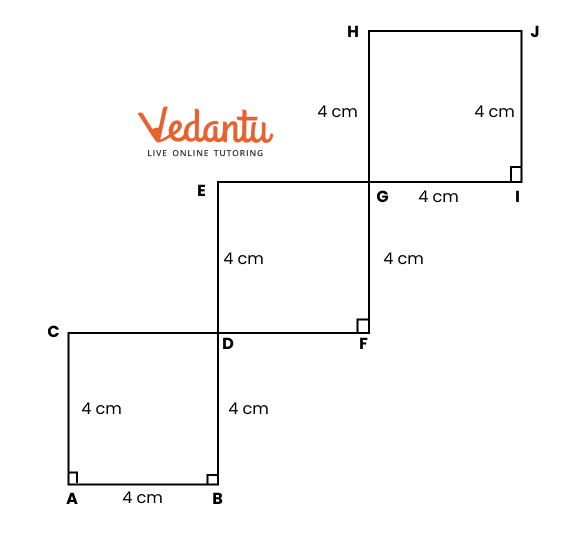
Step 4. Fig. 3 is the required figure of three “falling squares” each of side 4 cm.
3. Shadings
Construct the figure given below. Choose the measurement of your choice. Note that the larger 4-sided figure is square and so tire the smaller ones.

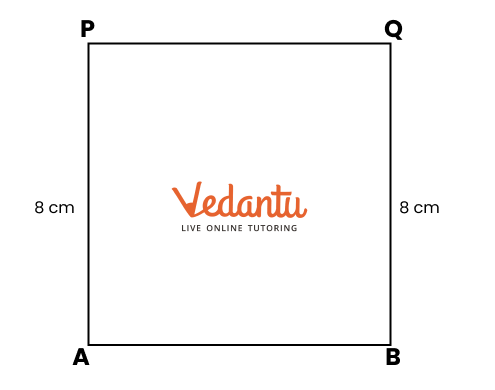
Ans: Step 1. Using a ruler, draw a line AB equal to 8 cm. Because, 8 ÷ 4 = 2, we shall draw smaller squares of side 2 cm. Using a protractor, draw perpendicular lines at A and B. Using a ruler, mark point P on the perpendicular line at A such that AP = 8 cm. Using a ruler, mark point Q on the perpendicular line at B such that BQ = 8 cm. Join P and Q using a ruler. Erase the lines above P and Q (Fig. 1).
Step 2. On the lines AB, BQ, QP, and PA, mark points at distances of 2 cm, using a ruler. Draw horizontal lines and vertical lines to get 16 squares. (Fig. 2)
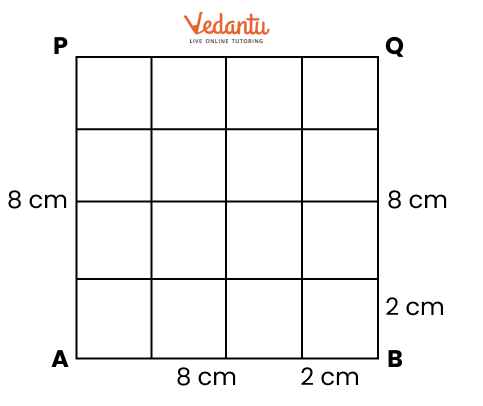
Step 3. From comer A, erase the inner sides of four squares to get a square of side 4 cm with one comer at A. Draw parallel diagonals of the remaining 12 small squares of side 2 cm each. (Fig. 3)
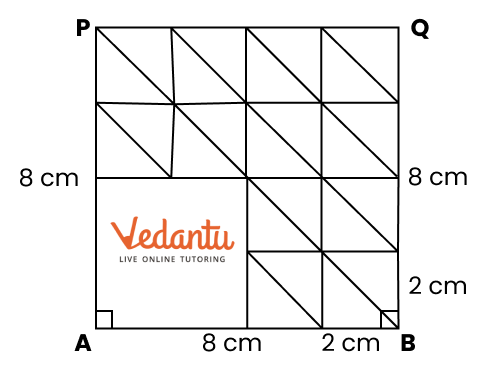
Step 4. In the 12 small squares, draw horizontal lines in the portion above the diagonals. (Fig. 4)
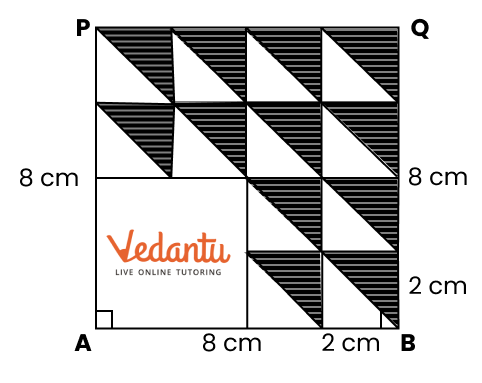
Step 5. Fig. 4 is the required figure having 12 small squares in a square.
4. Square with a Hole

Observe that the circular hole is the same as the centre of the square.
Construct a “Square with a Hole” as shown in the given figure. The centre of the hole is the same as the center of the square.
Hint: Think where the centre of the circle should be.
Ans: The centre of a square is the point of intersection of its diagonals. This centre is also the centre of the hole in the figure.
Step 1. Using a ruler, draw a line AB equal to 5 cm, say. Using a protractor, draw perpendicular lines at A and B. Using a ruler, mark point P on the perpendicular line at A such that AP = 5 cm. Using a ruler, mark point Q on the perpendicular line at B such that BQ = 5 cm. Join P and Q using a ruler. Erase the lines above P and Q (Fig. 1).
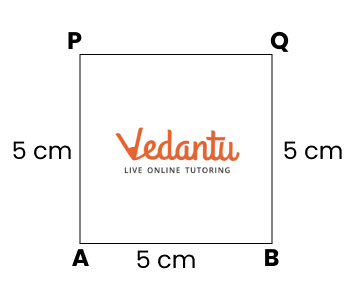
Step 2. Draw diagonals AQ and BP using a ruler. Let the diagonals intersect at C. This point is the centre of the square ABQP. Erase the diagonals AQ and BP. (Fig. 2).
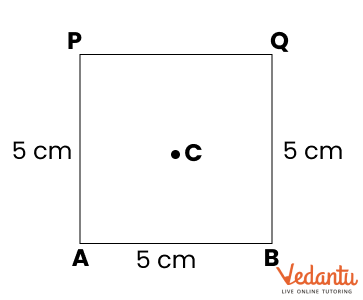
Step 3. With centre at C and a radius of 1.5 cm, say, draw a circle using a compass. (Fig. 3)
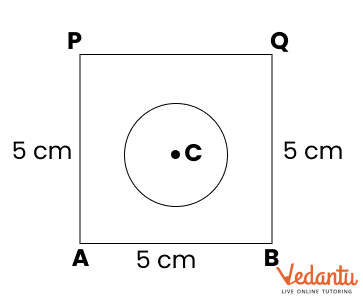
Step 4. Fig. 3 is the required “Square with a Hole”.
5. Square with more Holes
Construct a “Square with Four Holes” as shown in the given figure.

Ans: In the figure, the centre of a circle is the same as that of the corresponding square.
Step 1. Using a ruler, draw a line AB equal to 8 cm, say. Using a protractor, draw perpendicular lines at A and B. Using a ruler, mark point P on the perpendicular line at A such that AP = 8 cm. Using a ruler, mark point Q on the perpendicular line at B such that BQ = 8 cm. Join P and Q using a ruler. Erase the lines above P and Q. (Fig. 1)

Step 2. Using a ruler, find points C, D, E, and F such that AC = 4 cm, BD = 4 cm, QE = 4 cm, and PF = 4 cm. Join C and E and also F and D. (Fig. 2)
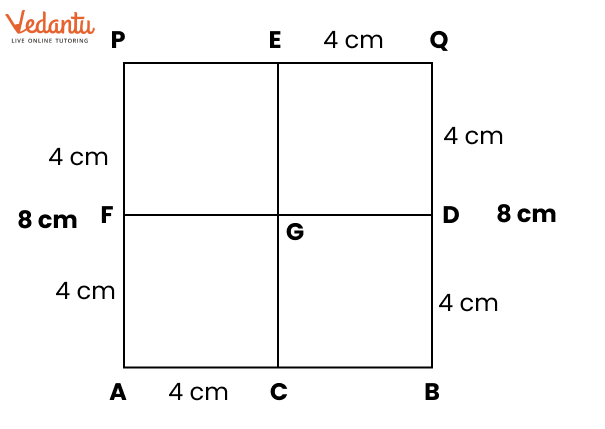
Step 3. Let G be the intersection of lines FD and CE. Find the centres of squares ACGF, CBDG, DQEG, and GEPF by joining their respective diagonals. (Fig. 3)

Step 4. Erase the extra lines used for finding the centres of the smaller circles. With centre at centres of small squares, draw four circles of radius 1.3 cm, say. (Fig. 4)
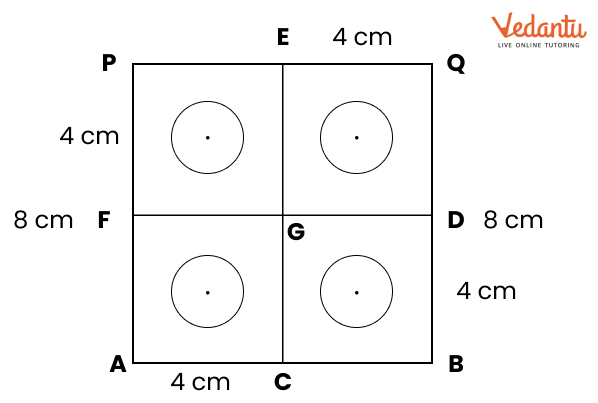
Step 5. Fig. 4 is the required “‘Square with Four Holes”.
6. Square with Curves
This is a square with 8 cm sidelengths.

Construct a “Square with Curves ”, taking a square of side 8 cm as shown in the figure.
Hint: Think where the tip of the compass can be placed to get all 4 arcs to bulge uniformly from each of the sides. Try it out!
Ans: In the given figure, the centres of the four arcs are outside the square.
Step 1. Using a ruler, draw a line AB equal to 8 cm. Using a protractor, draw perpendicular lines at A and B. Using a ruler, mark point P on the perpendicular line at A such that AP = 8 cm. Using a ruler, mark point Q on the perpendicular line at B such that BQ = 8 cm. Join P and Q using a ruler. Erase the lines above P and Q. (Fig. 1)

Step 2. Using a ruler, mark points C, D, E, and F such that AC = 4 cm, BD = 4 cm, QE = 4 cm, and PF = 4 cm. Join C and E and also D and F. Extend these lines outside the square. (Fig. 2)
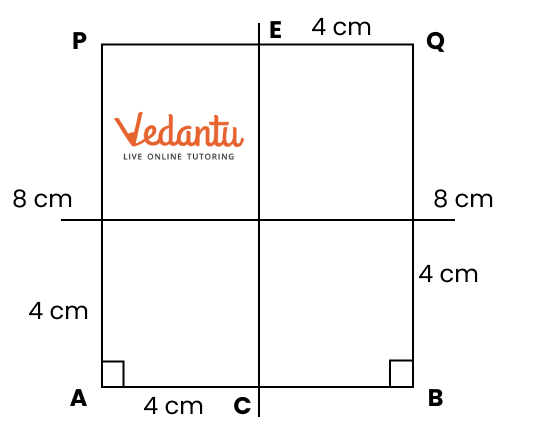
Step 3. Extend DF and take points G and H on it so that DG and FH are equal to 4 cm. Extend CE and take points I and J on it so that Cl and EJ are equal to 4 cm. The distance 4 cm can be taken slightly less than or greater than 4 cm. Join B and G. (Fig. 3)
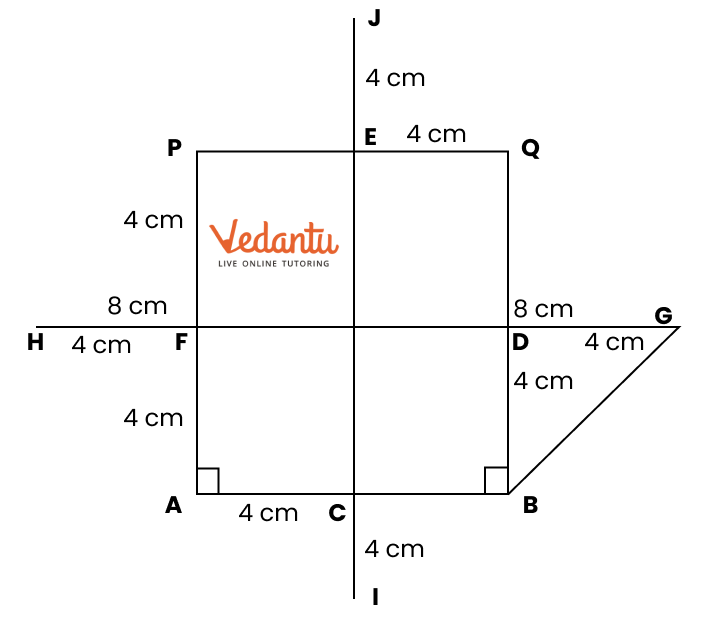
Step 4. With centres at G, H, I, and J and a radius equal to BG, draw four arcs inside the square as shown in the given figure. Erase the extra lines. (Fig. 4).

Step 5. Fig. 4 is the required “Square with Curves” with the square of side 8 cm.
Exercise 8.5
1. Construct a rectangle in which one of the diagonals divides the opposite angles into 50° and 40°.
Ans: We shall draw a rectangle of the form shown in Fig. 1.
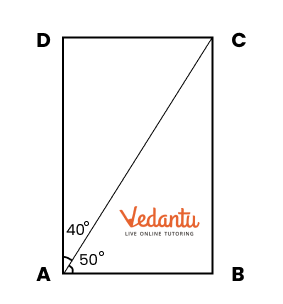
Step 1. Using a ruler, draw a line AB equal to 4 cm, say. (Fig. 2.)

Step 2. Using a protractor, mark dots C and D at angles 50° and 90° (50° + 40°), keeping the central point of the protractor at A. (Fig. 3)
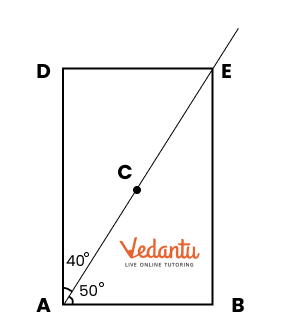
Step 3. Using a protractor, draw a perpendicular line to AB at B and let it intersect the extended line AC at E. (Fig 3)
Step 4. Using a protractor, draw a perpendicular line to BE at E and let it intersect the extended line AD at F. (Fig 4)
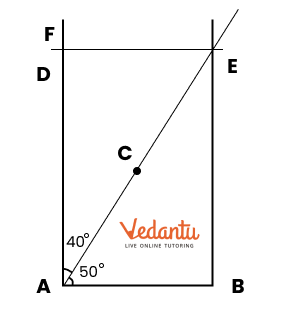
Step 5. Erase the extra lines in Fig. 4. (Fig. 5)
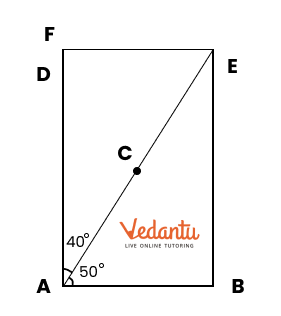
Step 6. Fig. 5 is the required rectangle in which one of the diagonals divides the opposite angles into 50° and 40°.
2. Construct a rectangle in which one of the diagonals divides the opposite angles into 45° and 45°. What do you observe about the sides?
Ans: Do it yourself.
3. Construct a rectangle one of whose sides is 4 cm and the diagonal is of length 8 cm.
Ans: We shall draw a rectangle of the form shown in Fig. 1.
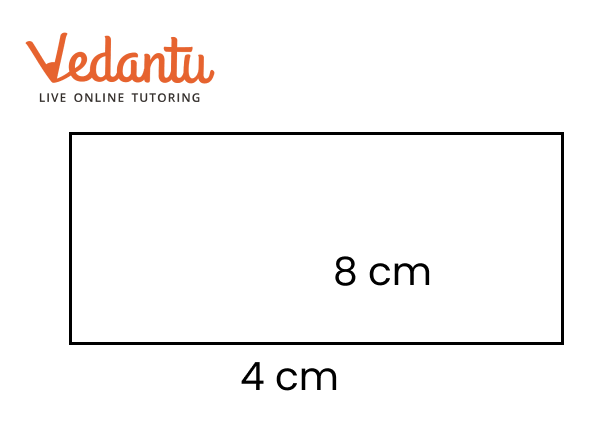
Step 1. Using a ruler, draw a line AB equal to 4 cm. (Fig. 2)
Step 2. Using a protractor, draw a perpendicular line to AB at A and B (Fig. 3)
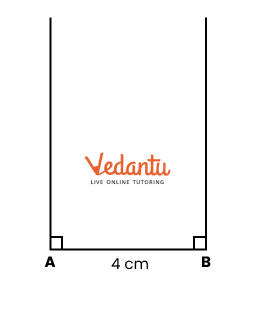
Step 3. With the centre at A and a radius equal to 8 cm, draw an arc to intersect the perpendicular at B. Similarly, draw an arc of radius 8 cm with a centre at B to intersect perpendicular at A. (Fig. 4)
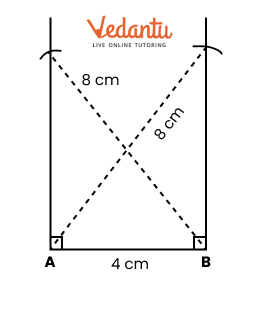
Step 4. Join the points of intersection of arcs by PQ. (Fig. 5)
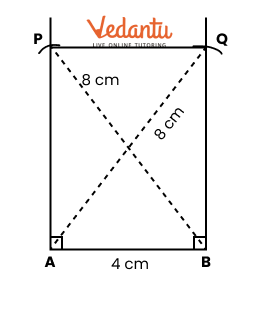
Step 5. Erase the extra lines in Fig. 5 (Fig. 6)
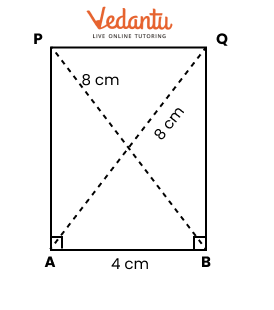
Step 6. Fig. 6 is the required rectangle with one side equal to 4 cm and diagonals of length 8 cm.
4. Construct a rectangle one of whose sides is 3 cm and the diagonal is of length 7 cm.
Ans:
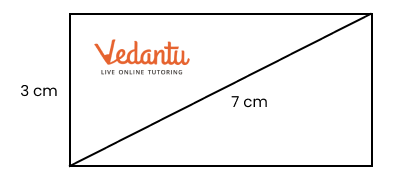
Exercise 8.6
1. Construct a bigger house in which all the sides are of length 7 cm.
Ans:

Step 1: Using a ruler, draw a line segment DE measuring 7 cm.

Step 2: Utilise a protractor to create perpendicular lines at points D and E on line DE. Mark point B on the perpendicular line at D such that BD is 7 cm long. Similarly, mark point C on the perpendicular at E, ensuring that CE is also 7 cm.
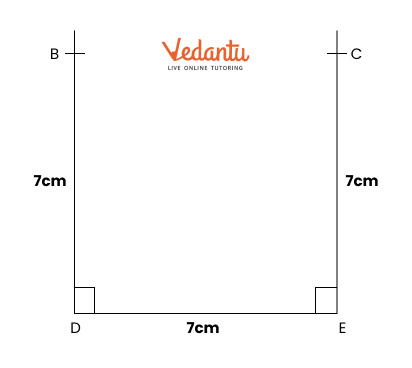
Step 3: Calculate 7 cm – 1 cm = 6 cm, and then divide by 2 to get 3 cm. Use a ruler to mark points P and Q on line DE such that DP is 3 cm and QE is 3 cm. From points P and Q, draw perpendicular lines to DE, each measuring 2 cm in length.

Step 4: Connect points R and S. With a compass set at points B and C, draw arcs with a radius of 7 cm to find their intersection point, labelled A. Then, connect points A to B and A to C. Using the compass centred at A, draw an arc from B to C. Finally, erase any unnecessary lines.

Step 5: The result is a completed drawing of the house, with all lines forming the outline measuring 7 cm.
2. Try to recreate ‘A Person’, ‘Wavy Wave’, and ‘Eyes’ from the section ‘Artworkʼ, using ideas involved in the ‘House’ construction.
Ans: A Person

Wavy Wave

Eyes
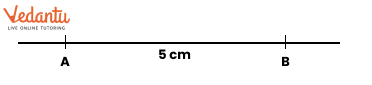
3. Is there a 4-sided figure in which all the sides are equal in length but is not a square? If such a figure exists, can you construct it?
Ans: Yes, a four-sided figure in which all sides are equal in length but is not a square does exist. This figure is called a rhombus.
Step 1: Draw a straight line and mark points A and B on it, ensuring that the distance AB measures 5 cm.
Step 2: Using a protractor, create points C and D such that the angles to the right of A and B each measure 60°.
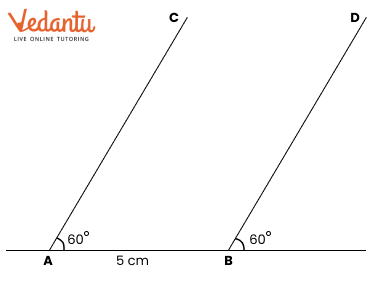
Step 3: With a ruler, identify point P on line AC so that AC is 5 cm long, and point Q on line BD so that BD is also 5 cm. Connect points P and Q.

Step 4: Measure the distance PQ with a ruler; it should equal 5 cm. Therefore, in the figure ABQP, all sides are equal to 5 cm. Additionally, ∠A measures 60°, which is not 90°.
Hence, ABQP is not a square.
Step 5: This demonstrates that there exist four-sided figures with all sides equal in length that are not squares.
Benefits of NCERT Solutions for Class 6 Maths Chapter 8 Playing with Constructions
Provides easy-to-follow explanations about geometric constructions, helping students understand concepts like using a compass and straightedge.
Offers clear steps and methods for constructing shapes, making it simpler for students to learn and apply these techniques effectively.
Helps students learn the basics of geometric constructions, which is essential for understanding more advanced topics in geometry.
Includes various practice problems that improve student’s ability to create accurate shapes and designs, enhancing their problem-solving skills.
The FREE PDF download allows students to study and practice at their own pace, making learning more convenient and flexible.
Important Study Material Links for Class 6 Maths Chapter 8 - Playing with Constructions
S. No | Study Material Links for Chapter 8 |
1. | |
2. | |
3. | Class 6 Playing with Constructions Important Formulas |
4. | Class 6 Playing with Constructions NCERT Exemplar Solution |
5. | Class 6 Playing with Constructions RD Sharma Solutions |
6. | Class 6 Playing with Constructions RS Aggarwal Solutions |
Conclusion
NCERT Solutions for Class 6 Maths Chapter 8, "Playing with Constructions," helps students understand how to create and work with geometric shapes using a compass and straightedge. The clear explanations make it easier to learn these important concepts and their applications in real life. Practising the exercises improves student’s skills in constructing shapes accurately, which is essential for more advanced geometry topics. With the solutions available as a FREE PDF download, students can study and review at their own pace, making learning both convenient and effective.
Chapter-wise NCERT Solutions Class 6 Maths
The chapter-wise NCERT Solutions for Class 6 Maths are given below. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.
S. No | NCERT Solutions Class 6 Chapter-wise Maths PDF | |
1. | ||
2. | ||
3. | ||
4. | ||
5. | ||
6. | ||
7. | ||
8. | ||
9. | ||
Related Important Links for Maths Class 6
Along with this, students can also download additional study materials provided by Vedantu for Maths Class 6.
S. No | Important Links for Class 6 Maths |
1. | |
2. | |
3. | |
4. | |
5. |
FAQs on NCERT Solutions for Class 6 Maths Chapter 8: Playing with Constructions
1. What topics are covered in Chapter 8?
Chapter 8 focuses on geometric constructions using a compass and straightedge. It includes creating shapes like squares, rectangles, and circles. Students also learn to explore the properties of these shapes through construction.
2. How do NCERT Solutions help with this chapter?
The NCERT Solutions provide clear steps and examples for each exercise in Chapter 8. These solutions help students understand how to perform geometric constructions accurately and effectively.
3. Can I download the solutions for Chapter 8?
Yes, the NCERT Solutions for Class 6 Maths Chapter 8 are available for free download as a PDF. This allows you to study and practice constructions at your own pace.
4. What types of problems are included in Chapter 8?
Chapter 8 includes problems related to constructing different shapes, exploring their properties, and finding points equidistant from two given points. Students practice these constructions step-by-step.
5. How do the solutions support learning in Chapter 8?
The solutions offer step-by-step guidance on solving construction problems. They help students visualise and understand the construction process, making it easier to apply these concepts.
6. Are the solutions for Chapter 8 useful for homework?
Yes, the NCERT Solutions for Chapter 8 are very helpful for homework. They provide detailed explanations and answers that make it easier for students to complete their assignments correctly.
7. What should I do if I find a construction problem difficult?
If a problem is difficult, refer to the NCERT Solutions for Class 6 Maths Chapter 8. The solutions include clear explanations that can help clarify the concepts and guide you through the construction process.
8. How can I practise the problems from Chapter 8?
Use the free PDF of NCERT Solutions for Class 6 Maths Chapter 8 to practise problems. Following the solutions allows you to work through different types of construction tasks and improve your skills.
9. How do I know if I’ve solved a construction problem correctly?
Check your answers against the NCERT Solutions for Chapter 8. The solutions provide correct answers and methods, allowing you to confirm if your answers and steps are accurate.
10. What concepts are reinforced through Chapter 8?
Chapter 8 reinforces concepts such as geometric constructions, properties of shapes, and using a compass and straightedge. It helps students understand how to create accurate shapes and their relationships.





















