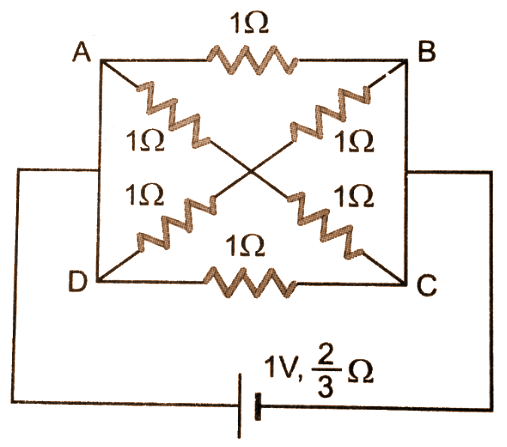
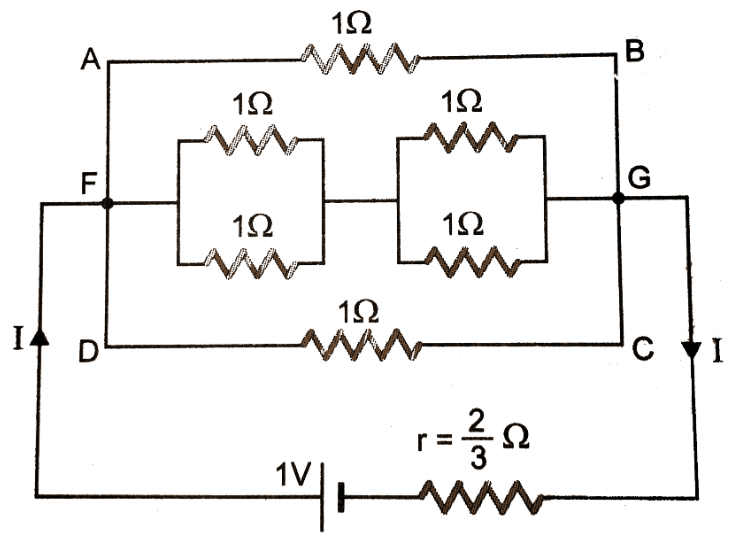
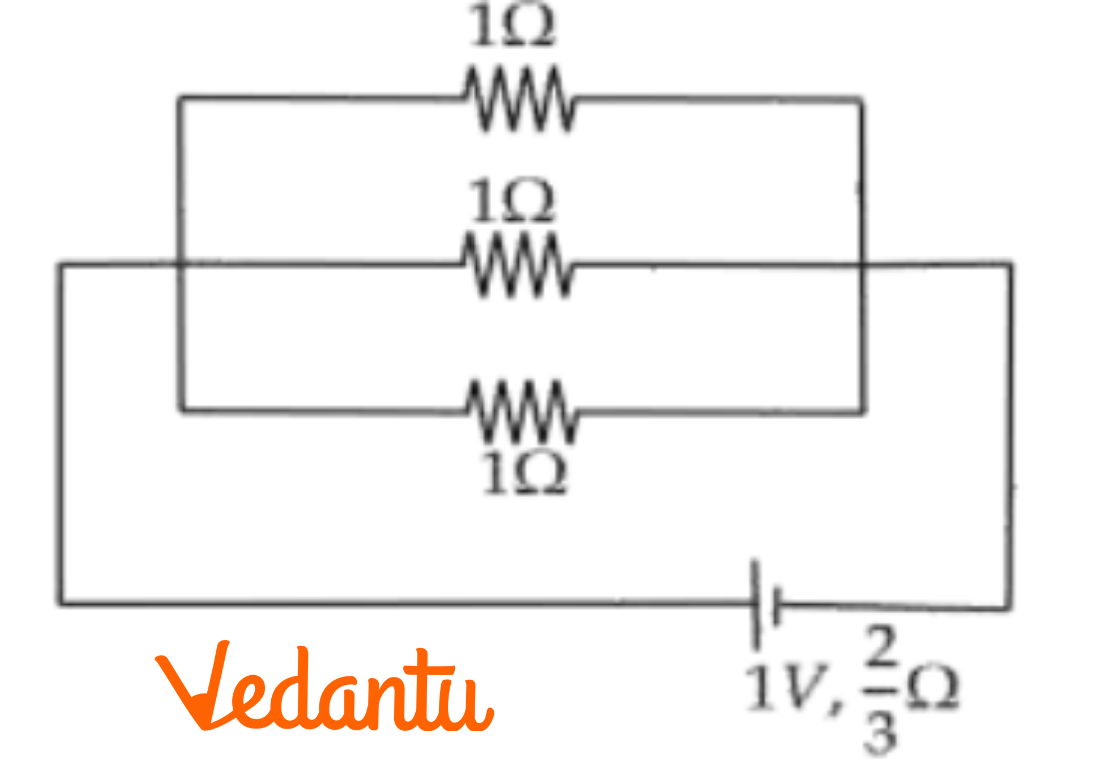
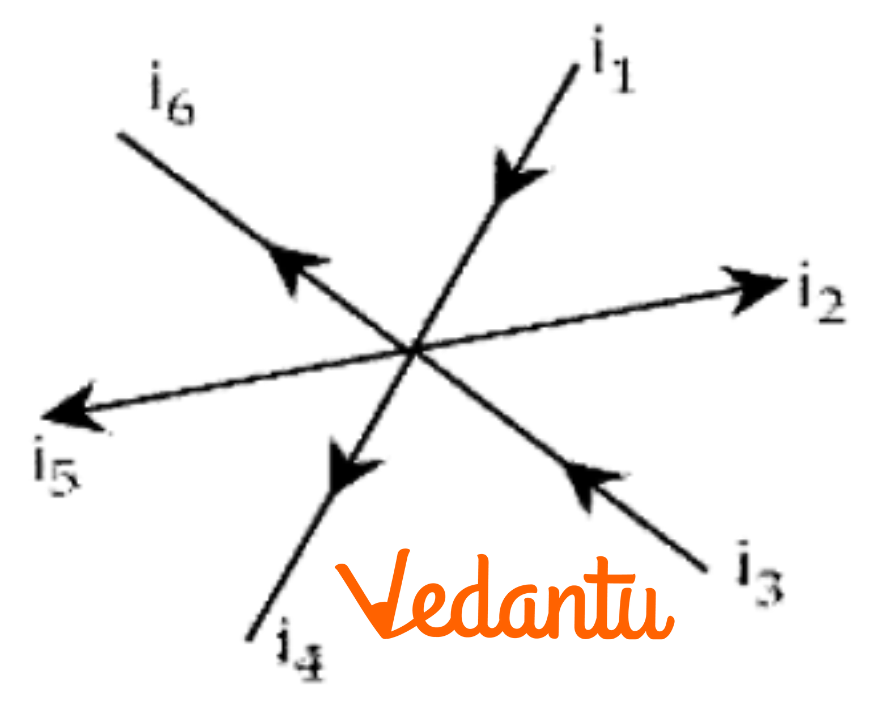
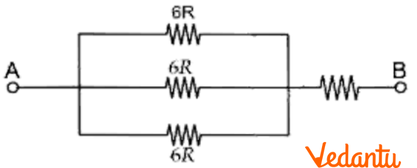
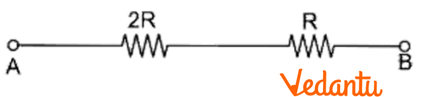
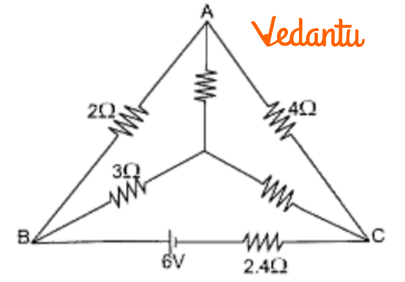
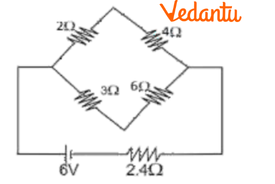
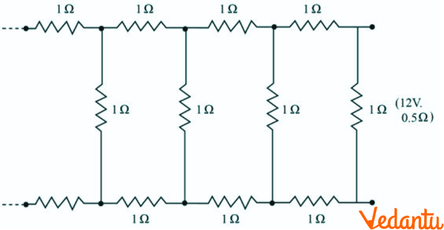
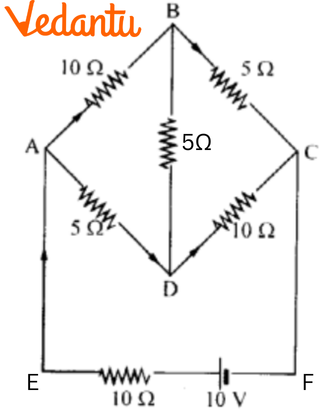
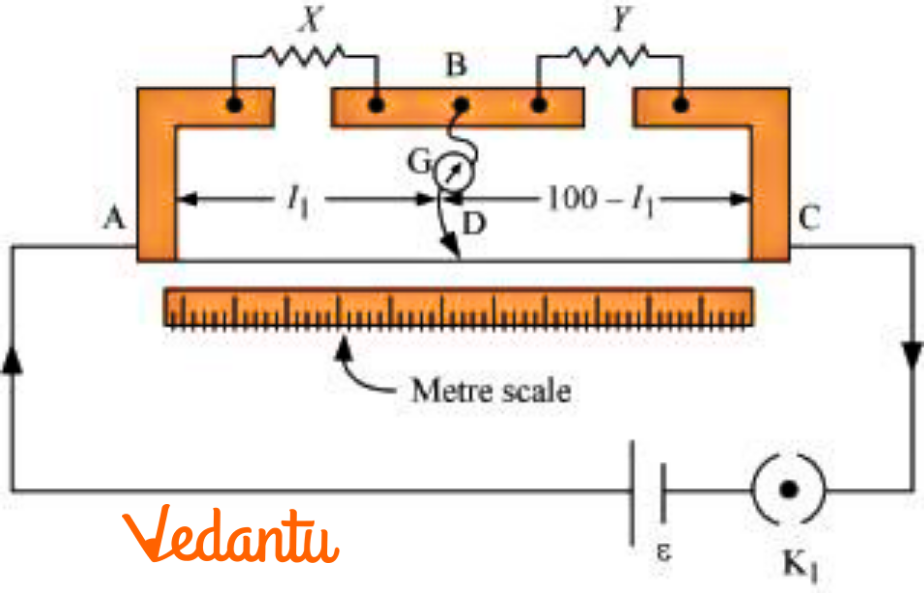
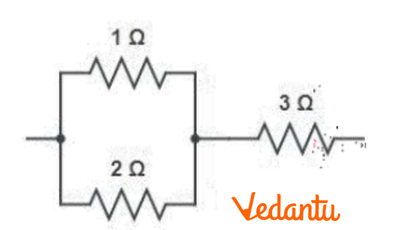
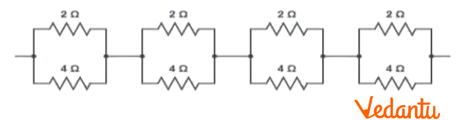
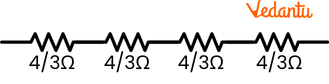
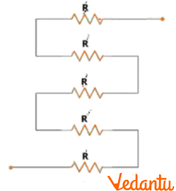
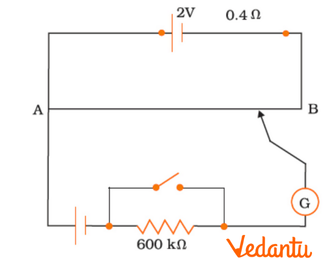
Download Important Questions for Class 12 Current Electricity FREE PDF









FAQs on Current Electricity Class 12 Important Questions: CBSE Physics Chapter 3
1. What is the potential difference and how is current and potential difference related to each other?
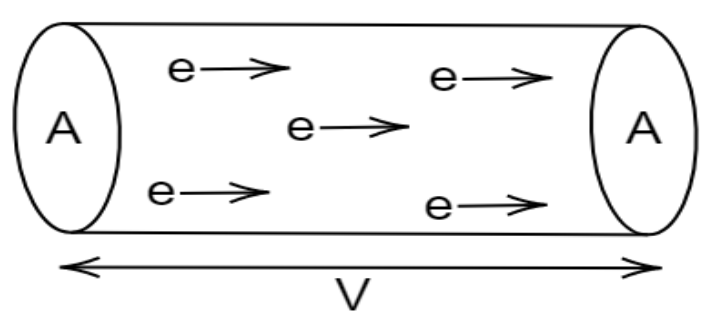
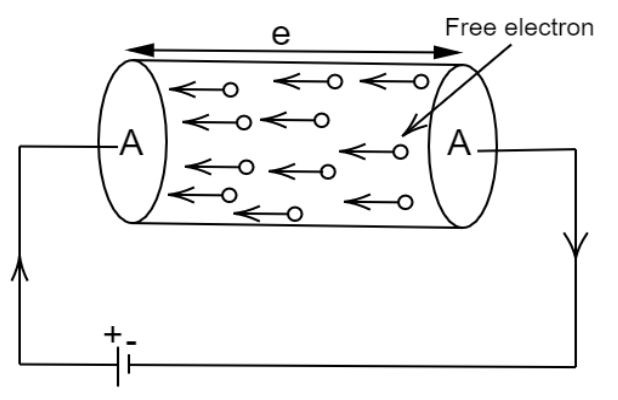
The word current means movement and electricity comes from the word electrons so basically current is the movement of free electrons in the conductor per unit time. Some conductors have a large number of free electrons whereas some have less number of free electrons. When these conductors are connected to each other, the free electrons move from high density of electrons to the low density of electrons. Thus, the current flows in the opposite direction of the flow of electrons. This difference in the density of electrons between the two conductors which promotes current electricity is called potential difference. Therefore, the current flows only when a potential difference is applied at the two ends of the conductor. According to Ohm's law, “In a conductor, potential difference applied across the conductor is directly proportional to the amount of the flow of current.”
2. What is the difference between resistance and resistivity?
Resistance is the hindrance offered to the flow of electrons in a specific material. Resistance can be considered opposite to the potential difference across the conductor because it encourages the flow of electrons while resistance discourages it. The rate at which the charge flows between two conductors is a combination of resistance and potential difference. Resistivity of a substance is the measure it's resisting power to conduct current electricity. In other words, resistivity is a fundamental property that quantifies how strongly a specified material can resist the flow of electrons.
3. What are the topics covered in important questions for class 12 CBSE Physics Chapter 6 by Vedantu?
In the chapter current electricity, vedantu has researched the important questions for CBSE which are basically from the following topics: Resistance and resistivity, potential difference, current electricity, ohm's law, potentiometer, emf of the cell, kirchoff's rule, drift velocity, cells and its combinations, wheatstone bridge, circuit diagrams, numericals, Peltier Effect, Thomson Effect, Thermoelectric Effect and Joule’s Law Of Heating.
4. How to study current electricity for CBSE exam?
The various topics covered in Class 12 Chapter 3 Physics are:
Electric current
Electric currents in conductors
Ohm’s law
Drift of electrons and origin of resistivity
Limitations of Ohm’s law
Resistivity of various materials
Temperature dependence of resistivity
Electrical energy, power
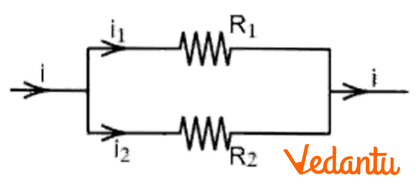
Combination of resistors - series and parallel
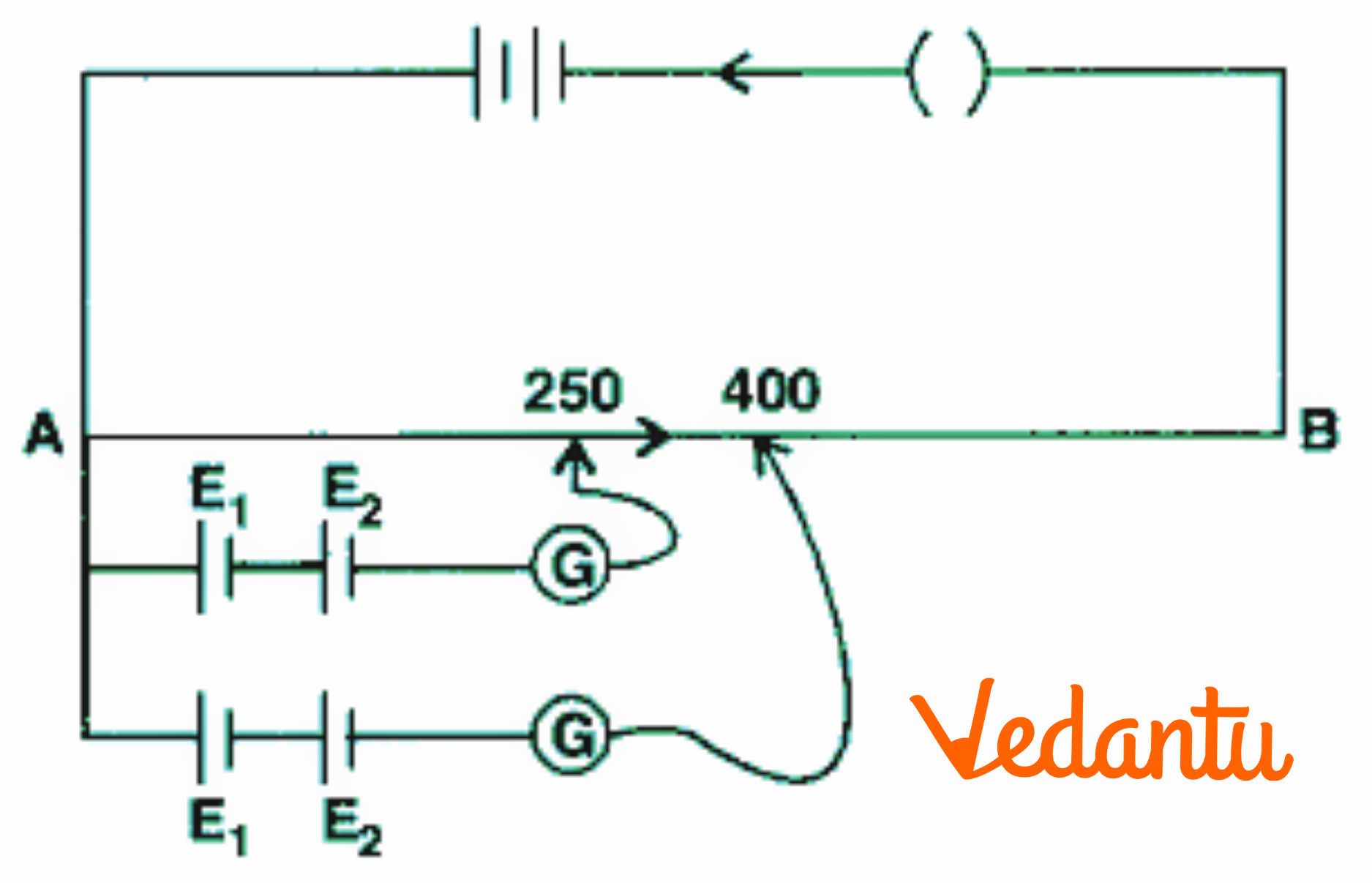
Cells, emf, internal resistance
Cells in series and parallel
Kirchhoff’s rules
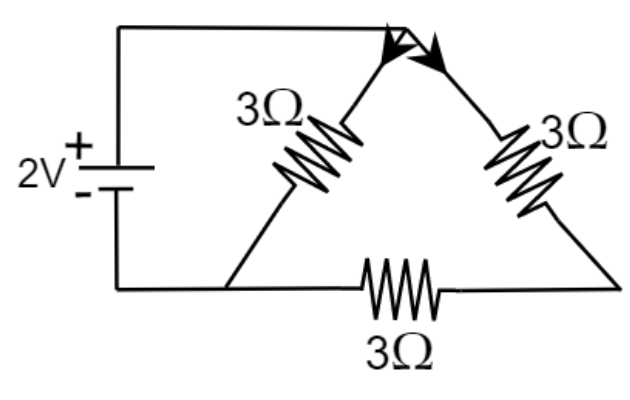
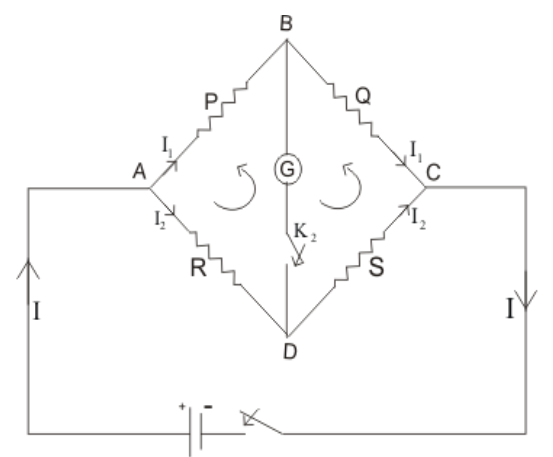
Wheatstone bridge
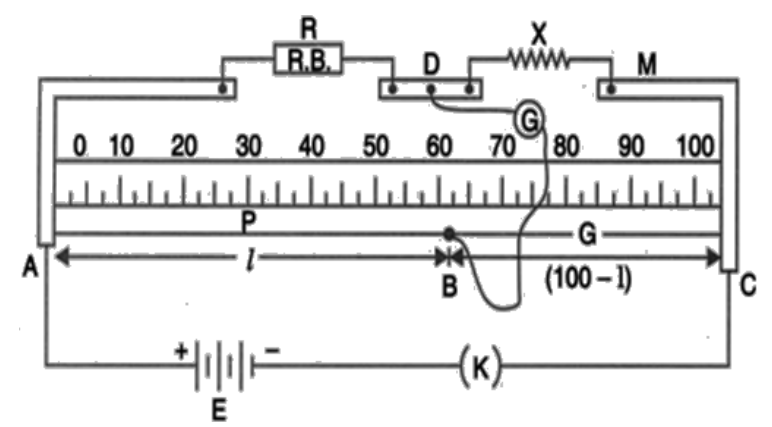
Meter bridge
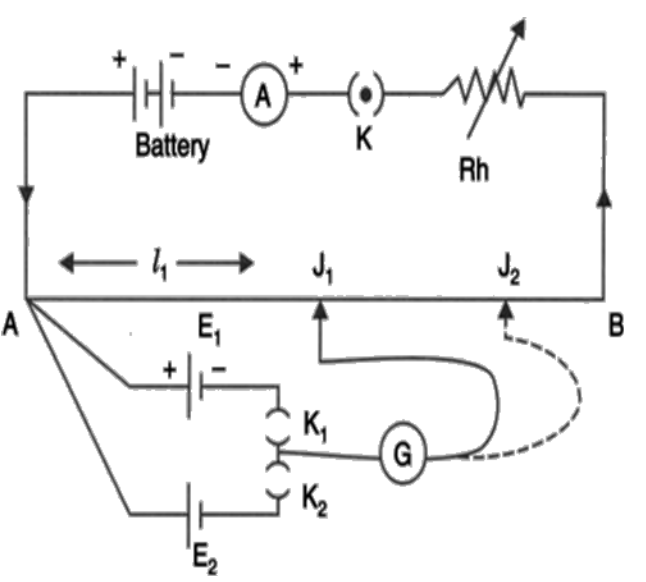
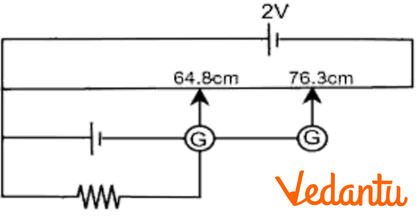
Potentiometer
All these topics are important for the student to study so that they are thorough with all that their syllabus has to offer.
These solutions are available on Vedantu's official website(vedantu.com) and mobile app free of cost.
5. What are the different topics covered in Chapter 3 of Class 12 Physics?
The various topics covered in Class 12 Chapter 3 Physics are:
Electric current
Electric currents in conductors
Ohm’s law
Drift of electrons and origin of resistivity
Limitations of Ohm’s law
Resistivity of various materials
Temperature dependence of resistivity
Electrical energy, power
Combination of resistors - series and parallel
Cells, emf, internal resistance
Cells in series and parallel
Kirchhoff’s rules
Wheatstone bridge
Meter bridge
Potentiometer
All these topics are important for the student to study so that they are thorough with all that their syllabus has to offer.
These solutions are available on Vedantu's official website(vedantu.com) and mobile app free of cost.
6. Are the important questions good reference material?
Yes, Vedantu’s important questions are surely the best reference material students should access to score high marks in their exams. These questions are designed by experts and are organized to help students in every step of their preparation. The questions that these guide books provide help students in practising the various important topics that are essential for the exams. This lets the student know how the question pattern usually is.
7. Is Chapter 3 of Class 12 Physics hard?
If students are clear and confident with all the concepts of Chapter 3, they will find everything easy. Thus, it is important to read the chapter and be thorough with the concepts. The best way to do this is by marking all the important and relevant areas and practising them regularly. Students should be thorough with all the laws, formulas and theorems of Chapter 3, as most questions set in the question papers deal with the understanding of these. Thus, students must practice repeatedly to be confident in what they study. This will make the chapter easy to understand.
8. What is Ohm’s law? Is it universally applicable to all conducting elements?
The current flowing from the conductor from the two points is directly proportional to the voltage across those two points. This is what the Ohm’s law states.
V=IR
Where v stands for Voltage
I stands for current
And R stands for resistance.
Ohm’s law is inapplicable universally when we think about all the conducting elements . For instance, vacuum diode semiconductor is a non-ohmic conductor and thus the Ohm’s law is inapplicable to it.
For more such answers and insights to the chapter, it is advisable that it focuses on the important questions and concepts. To help themselves, they can visit the website of Vedantu and look through all the important questions that are needed for the preparation of the exam.