Watch Video
NCERT Solutions for Class 9 Maths Chapter 7 Triangles Exercise 7.3 - FREE PDF Download
Students will study in detail triangles and their various properties in NCERT solutions for Maths class 9 triangles exercise 7.3. The chapter introduces the definition of a triangle, the congruence of triangles, inequalities in a triangle, rules of congruence, and more. The chapter explains some rules or criteria for the congruence of triangles. Class 9 7.3 Exercise covers the Side- Angle- Side Congruence Rule, Angle- Side- Side Congruence Rule, and Angle- Angle- Side Congruence Rule. Apart from it, other properties of an isosceles triangle, theorems related to triangles, and more concepts are explained in NCERT Solutions Class 9 Maths 7.3. Vedantu’s Class 9 Maths NCERT Solutions provide step-by-step explanations to help you clear your doubts.
- 3.1Exercise 7.3
Glance on NCERT Solutions Maths Chapter 7 Exercise 7.3 Class 9 | Vedantu
NCERT Solutions Maths Chapter 7 Exercise 7.3 Class 9 includes Criteria for Congruence of Triangles, SSS congruence rule, and Right Angle-Hypotenuse-Side.
SSS (Side-Side-Side) Congruence Rule: Two triangles are congruent if all corresponding sides have the same lengths.
RHS (Right Angle-Hypotenuse-Side) Congruence Rule: Two right triangles are congruent if the hypotenuse and a leg (non-right angle side) of one triangle are equal in length to the corresponding hypotenuse and leg of the other triangle.
There are 5 questions in Maths Class 9th Exercise 7.3 which experts at Vedantu have completely solved.
NCERT Solutions for Class 9 Maths Chapter 7 - Triangles Exercise 7.3
ShareShareExercise 7.3
1. \[\mathbf{\text{ }\!\!\Delta\!\!\text{ ABC}}\] and \[\mathbf{\text{ }\!\!\Delta\!\!\text{ DBC}}\] are two isosceles triangles on the same base \[\mathbf{\text{BC}}\] and vertices \[\mathbf{\text{A}}\] and \[\mathbf{\text{D}}\] are on the same side of \[\mathbf{\text{BC}}\] (see the given figure). If \[\mathbf{\text{AD}}\] is extended to intersect \[\mathbf{\text{BC}}\] at \[\mathbf{\text{P}}\], show that:
(i) \[\mathbf{\text{ }\!\!\Delta\!\!\text{ ABD}\cong \text{ }\!\!\Delta\!\!\text{ ACD}}\]
Ans: In \[\text{ }\!\!\Delta\!\!\text{ ABD}\] and \[\text{ }\!\!\Delta\!\!\text{ ACD}\],
\[\text{AB=AC}\] (Given)
\[\text{BD=CD}\] (Given)
\[\text{AD=AD}\] (Common)
Therefore, \[\text{ }\!\!\Delta\!\!\text{ ABD}\cong \text{ }\!\!\Delta\!\!\text{ ACD}\] (By \[\text{SSS}\] rule)
\[\angle \text{BAD=}\angle \text{CAD}\] (By \[CPCT\])
\[\angle \text{BAP=}\angle \text{CAP}\]
(ii) \[\mathbf{\text{ }\!\!\Delta\!\!\text{ ABP }\cong \text{ }\!\!\Delta\!\!\text{ ACP}}\]
Ans: In \[\text{ }\!\!\Delta\!\!\text{ ABP}\] and \[\text{ }\!\!\Delta\!\!\text{ ACP}\],
\[\text{AB=AC}\] (Given)
\[\angle \text{BAP = }\angle \text{CAP}\]
\[\text{AP= AP}\] (Common)
Therefore, \[\text{ }\!\!\Delta\!\!\text{ ABP }\cong \text{ }\!\!\Delta\!\!\text{ ACP}\] (By \[\text{SAS}\] congruence rule)
Therefore, \[\text{BP=CP}\] (By \[CPCT\])
(iii) \[\mathbf{\text{AP}}\] bisects \[\mathbf{\angle \text{A}}\] as well as \[\mathbf{\angle \text{D}}\].
Ans: Since, \[\angle \text{BAP=}\angle \text{CAP}\],
Therefore, \[\text{AP}\] bisects \[\angle \text{A}\].
In \[\text{ }\!\!\Delta\!\!\text{ BDP}\] and \[\text{ }\!\!\Delta\!\!\text{ CDP}\],
\[\text{BD= CD}\] (Given)
\[\text{DP=DP}\] (Common)
\[\text{BP= CP}\]
Therefore, \[\text{ }\!\!\Delta\!\!\text{ BDP}\cong \text{ }\!\!\Delta\!\!\text{ CDP}\] (By \[\text{SSS}\] congruence rule)
Therefore, \[\angle \text{BDP=}\angle \text{CDP}\] (By \[\text{CPCT}\])
Hence, \[\text{AP}\] bisects \[\angle \text{D}\].
(iv) \[\mathbf{\text{AP}}\] is the perpendicular bisector of \[\mathbf{\text{BC}}\].
Ans: Since \[\text{ }\!\!\Delta\!\!\text{ BDP}\cong \text{ }\!\!\Delta\!\!\text{ CDP}\],
Therefore,
\[\angle \text{BPD =}\angle \text{CPD}\] (By \[\text{CPCT}\])
\[\angle \text{BPD+}\angle \text{CPD=18}{{\text{0}}^{\text{o}}}\] (Linear pair of angles)
\[\angle \text{BPD +}\angle \text{BPD = 18}{{\text{0}}^{\text{o}}}\] (Since \[\angle \text{BDP=}\angle \text{CDP}\])
\[\text{2}\angle \text{BPD=18}{{\text{0}}^{\text{o}}}\]
\[\angle \text{BPD = 9}{{\text{0}}^{\text{o}}}\]
Hence \[\text{AP}\] is the perpendicular bisector of \[\text{BC}\].
2. \[\mathbf{AD}\] is an altitude of an isosceles triangles \[\mathbf{ABC}\] in which \[\mathbf{\text{AB=AC}}\]. Show that:
(i) \[\mathbf{AD}\] bisects \[\mathbf{\text{BC}}\]
Ans: In \[\text{ }\!\!\Delta\!\!\text{ BAD}\] and \[\text{ }\!\!\Delta\!\!\text{ CAD}\],
\[\angle \text{ADB= }\angle \text{ADC}\] (Each \[\text{90 }\!\!{}^\text{o}\!\!\text{ }\])
\[\text{AB=AC}\] (Given)
\[\text{AD=AD}\] (Common)
Therefore, \[\text{ }\!\!\Delta\!\!\text{ BAD }\cong \text{ }\!\!\Delta\!\!\text{ CAD}\] (By \[\text{RHS}\] congruence rule)
Therefore, \[\text{BD = CD}\] (By \[\text{CPCT}\])
Hence, \[\text{AD}\] bisects \[\text{BC}\].
(ii) \[\mathbf{AD}\] bisects \[\mathbf{\angle \text{A}}\].
Ans: Also, \[\angle \text{BAD=}\angle \text{CAD}\] (By \[\text{CPCT}\])
Hence, \[AD\] bisects \[BC\].
Therefore, \[AD\] bisects \[\angle A\].
3. Two sides \[\mathbf{\text{AB}}\] and \[\mathbf{\text{BC}}\] and median \[\mathbf{\text{AM}}\] of one triangle \[\mathbf{\text{ABC}}\] are respectively equal to sides \[\mathbf{\text{PQ}}\] and \[\mathbf{\text{QR}}\] and median \[\mathbf{\text{PN}}\] of \[\mathbf{\text{ }\!\!\Delta\!\!\text{ PQR}}\] (see the given figure). Show that:
(i) \[\mathbf{\text{ }\!\!\Delta\!\!\text{ ABM}\cong \text{ }\!\!\Delta\!\!\text{ PQN}}\]
Ans: In \[\text{ }\!\!\Delta\!\!\text{ ABC}\], \[\text{AM}\] is the median to \[\text{BC}\].
Therefore, \[\text{BM=}\frac{1}{2}BC\]
In \[\text{ }\!\!\Delta\!\!\text{ PQR}\], \[PN\] is the median to \[QR\].
Therefore, \[\text{QN=}\frac{1}{2}QR\]
However, \[\text{BC=QR}\]
So, \[\text{BM=QN}\] …… (i)
In \[\text{ }\!\!\Delta\!\!\text{ ABM}\] and \[\text{ }\!\!\Delta\!\!\text{ PQN}\],
\[\text{AB=PQ}\] (Given)
\[\text{BM=QN}\] (From (i))
\[\text{AM=PN}\] (Given)
Therefore, \[\text{ }\!\!\Delta\!\!\text{ ABM}\cong \text{ }\!\!\Delta\!\!\text{ PQN}\] (By \[SSS\] congruence rule)
\[\angle ABM\text{=}\angle \text{PQN}\] (By \[\text{CPCT}\])
So, \[\angle ABC\text{=}\angle \text{PQR}\] …… (ii)
(ii) \[\mathbf{\text{ }\!\!\Delta\!\!\text{ ABC}\cong \text{ }\!\!\Delta\!\!\text{ PQR}}\]
Ans: In \[\text{ }\!\!\Delta\!\!\text{ ABC}\] and \[\text{ }\!\!\Delta\!\!\text{ PQR}\].
\[\text{AB=PQ}\] (Given)
\[\angle ABC\text{=}\angle \text{PQR}\] (from (ii))
\[\text{BC=QR}\] (Given)
Therefore, \[\text{ }\!\!\Delta\!\!\text{ ABC}\cong \text{ }\!\!\Delta\!\!\text{ PQR}\] (By \[SAS\] congruence rule)
4. \[\mathbf{BE}\] and \[\mathbf{CF}\] are two equal altitudes of a triangle \[\mathbf{ABC}\]. Using \[\mathbf{RHS}\] congruence rule, prove that the triangle \[\mathbf{ABC}\] is isosceles.
Ans: In \[\text{ }\!\!\Delta\!\!\text{ BEC}\] and \[\text{ }\!\!\Delta\!\!\text{ CFB}\],
\[\angle \text{BEC= }\angle \text{CFB}\] (Each \[\text{90 }\!\!{}^\text{o}\!\!\text{ }\])
\[\text{BC=CB}\] (Common)
\[\text{BE= CF}\] (Given)
Therefore, \[\text{ }\!\!\Delta\!\!\text{ BEC}\cong \text{ }\!\!\Delta\!\!\text{ CFB}\] (By \[RHS\] congruency)
Therefore, \[\angle \text{BCE=}\angle \text{CBF}\] (By \[\text{CPCT}\])
Therefore, \[\text{AB=AC}\] (Sides opposite to equal angles of a triangle are equal)
Hence, \[\text{ }\!\!\Delta\!\!\text{ ABC}\] is isosceles.
5. \[\mathbf{ABC}\] is an isosceles triangle with \[\mathbf{\text{AB=AC}}\]. Draw \[\mathbf{\text{AP}\bot \text{BC}}\] to show that \[\mathbf{\angle \text{B=}\angle \text{C}}\].
Ans: In \[\text{ }\!\!\Delta\!\!\text{ APB}\] and \[\text{ }\!\!\Delta\!\!\text{ APC}\],
\[\angle \text{APB = }\angle \text{APC}\] (Each \[\text{90 }\!\!{}^\text{o}\!\!\text{ }\])
\[\text{AB=AC}\] (Given)
\[\text{AP=AP}\] (Common)
Therefore, \[\text{ }\!\!\Delta\!\!\text{ APB}\cong \text{ }\!\!\Delta\!\!\text{ APC}\] (Using \[\text{RHS}\] congruence rule)
Therefore, \[\angle \text{B=}\angle \text{C}\] (By \[\text{CPCT}\])
Conclusion
In Class 9 Maths Chapter 7.3, we explored the various properties and theorems related to triangles. This exercise emphasized the application of the Angle Sum Property and the Exterior Angle Property of triangles. By solving the problems in 7.3 class 9 maths, we strengthened our understanding of how to find unknown angles and sides using these fundamental properties.
Class 9 Maths Chapter 7: Exercises Breakdown
CBSE Class 9 Maths Chapter 7 Other Study Materials
NCERT Solutions for Class 9 Maths - Chapter-wise PDF
Given below are the chapter-wise NCERT Solutions for Class 9 Maths. Go through these 9th maths solutions chapter-wise to be thoroughly familiar with the concepts.
Important Study Materials for CBSE Class 9 Maths
FAQs on NCERT Solutions for Class 9 Maths Chapter 7 - Triangles Exercise 7.3
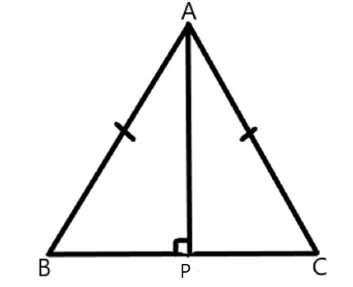
1. How to show AB bisects PQ if AB is an altitude of an isosceles triangle APQ in which AP = AQ?
In the question, it’s given that AB is an altitude and AP = AQ. Now, in a triangle, APB and AQB, angle ABP = 90o and ABQ = 90o. As AP = AQ and AB is the common arm in the triangle APB and AQB, then apply the RHS congruence rule. The rule states that two triangles are said to be congruent if the hypotenuse and one side of a triangle are equal to another triangle’s hypotenuse and its one side. Hence, both the triangles APB and AQB are equal. Now, by applying the CPCT rule, PB is equal to QB. Hence, AB bisects PQ.
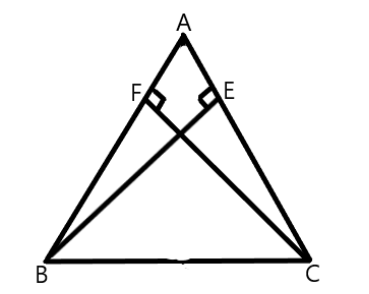
2. How to prove that the ABC is an isosceles triangle if BE and CF are two altitudes equal to each other?
Given that BE and CF are two altitudes of an isosceles triangle that are equal to each other. In the triangle BEC and CFB, angle BEC is equal to 90o and CFB is equal to 90o. Also, BC = CB as it is a common side. Similarly, BE and CF are equal. By applying the RHS Congruence Rule, triangle BEC and CFB are equal to each other. Additionally, by CPCT rule, C = B. It means that sides opposite to the equal angles are equal always. Hence, AB = AC. It shows, that side AB and BC of the triangle ABC form an isosceles triangle.
3. What is a Triangle, and what are its properties?
A triangle is a type of polygon with three sides. It is a closed, two-dimensional shape made of straight lines. Therefore every triangle has a total of three vertices, and each vertex forms an angle. The main properties of a triangle are as follows.
It has three sides and therefore three angles, and the sum of the three angles is 180 degrees.
The addition of all the exterior angles of a triangle is 360 degrees.
The total sum of the lengths of any two sides of a triangle is invariably greater than the length of the third side.
The shortest side of the triangle always lies opposite to the smallest interior angle.
4. How many sums are there in Exercise 7.3 of Class 9 Maths?
There are a total of five sums in Class 9 Maths Chapter 7 Exercise 7.3. Each sum assesses the application of the concepts of triangles and their various properties. The sums are based on the understanding of the properties of a triangle, the congruence of a triangle, rules of congruences, the theorems and corollaries of inequalities of triangles, and many more. In the NCERT Solutions of Class 9 Maths Chapter 7 Ex-7.3 PDF, every sum is followed by a detailed analysis of self-explanatory solutions helping students get a better understanding of the concepts.
5. Is Exercise 7.3 of 9th Maths easy?
Yes, if practised and understood well, this exercise is extremely easy for the students. In order to get help in comprehending the concepts, the students should get help from the NCERT solutions. These solutions have all the exercises with detailed and explanatory answers that will help the student in understanding the concepts in a better light. With the practice of this exercise from the NCERT solutions, the student will attain confidence in their knowledge, which will eventually help them in scoring better marks.
6. What is the importance of this exercise in Mathematics?
Triangles are one of the first shapes that are studied under geometry. They are important in academics because arbitrary polygons with 4, 5, 6, or n sides can be usually decomposed into triangles. Therefore, the basic properties of a triangle will pave the way for learning and analysing a much deeper study of the other polygons. This exercise caters to this basic knowledge of a triangle, and some major concepts such as the Pythagoras Theorem and trigonometry are dependent on the properties of a triangle.
7. Is this the only exercise that deals with the concept of Triangles?
There are a total of 5 sections that deal with different concepts within the study of Triangles. Each of these sections has varied problems based on different theories and formulas, and without the understanding of the previous section, it becomes difficult to get a better hold of the successive exercise. Mastery of each of these sections will help score better marks, as the questions usually asked in the examination are not always straightforward. They are rather a blend of all these concepts present in the various exercises.
