Complete Resource of NCERT Solutions for Maths Chapter 7 Triangles Class 9 - Free PDF Download






FAQs on NCERT Solutions for Class 9 Maths Chapter 7 - Triangles
1. Is triangles Class 9 difficult?
Class 9 triangles may seem difficult since they need an understanding of multiple fundamental geometry principles and theorems. However, the principles become easier to understand with thorough study and application. By providing clear explanations and visual aids, Vedantu's solutions and video tutorials may help in the simplification of these difficult concepts, making the learning experience easier and more enjoyable.
2. What is the basic concept of a triangle Class 9?
In Class 9 triangles, the basic concept of a triangle is to understand its characteristics, varieties, and angles and sides theorems. It covers the study of triangle congruence and similarity, the connection between a triangle's sides and angles, and the use of Pythagoras' Theorem in right-angled triangles.
3. What is the theory of triangles Class 9?
Triangle congruence and similarity criteria (like SSS, SAS, ASA, and RHS), Pythagoras' Theorem, properties like the idea that the sum of a triangle's angles is 180 degrees, and the triangle inequality theorem—which claims that the sum of any two of a triangle's sides is greater than the length of the third side—are the main topics covered in Class 9's theory of triangles.
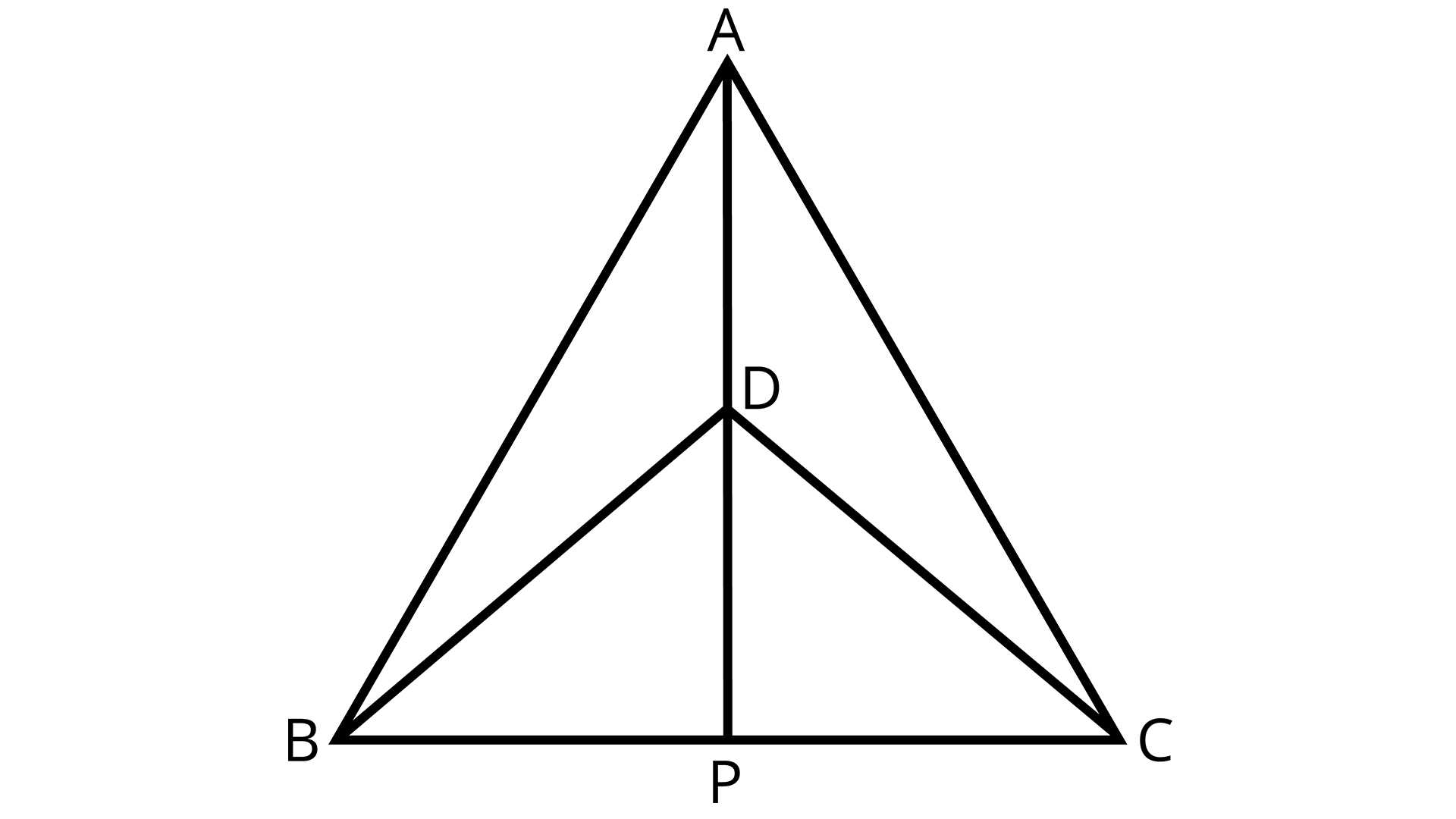
4. What is the median of a triangle in class 9 triangles?
A line segment from a vertex to the opposite side's midway is called a triangle's median. There are three medians in every triangle, and they are significant because they meet at a single location known as the centroid, which is the center of gravity of the triangle.
5. What are the 7 properties of a Triangles Class 9?
The sum of the angles in a triangle is always 180 degrees.
The exterior angle of a triangle is equal to the sum of the opposite interior angles.
The sum of the lengths of any two sides of a triangle is greater than the length of the third side.
In a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
Each angle in an equilateral triangle is 60 degrees.
The medians of a triangle intersect at a single point (centroid), which is the triangle's center of gravity.
The perpendicular bisectors of a triangle's sides intersect at a point (circumcenter), which is equidistant from the triangle’s vertices.