NCERT Solutions for Maths Class 11 Chapter 5 - Linear Inequalities - FREE PDF Download
FAQs on NCERT Solutions for Class 11 Maths Chapter 5 Linear Inequalities
1. What is the number of exercises in the Class 11 Linear Inequalities Class 11 Ncert Solutions book?
A total of two exercises are there in the chapter of Linear Inequalities NCERT including one 'miscellaneous' exercise. The first exercise 5.1 consists of twenty-six questions, and the miscellaneous exercise contains fourteen questions in total.
2. What are the crucial topics of Linear Inequalities Class 11 Ncert Solutions?
The essential topics of the chapter Linear Inequalities are:
Basic problems on linear inequalities
Graphical representation of linear inequality with the help of two variables
Pictorial method to find the solution of linear inequalities
The solution of linear inequalities using algebra (one variable), and representing them on the number line
3. How to plot a linear inequality on a graph in Chapter 5 Class 11 Maths?
Students must arrange the equation in a way such that 'y' is on the left side, while others are on the right. In the next step, learners need to plot the 'y' line (draw a continuous line when y<= or y>= and a dotted line (when y< or y>). Finally, shade the area above the y line when (y> or y>=) or down the line (when y< or y<=), from Chapter 5 Class 11 Maths.
4. How to solve Chapter 5 Class 11 Maths?
You can solve the exercises in Class 11 Chapter 5 with the help of NCERT Solutions Class 11 Maths Chapter 5 Linear Inequalities on Vedantu. This NCERT Solutions can be downloaded as well to get easy accessibility later on even in the absence of the internet. The best thing about the NCERT Solutions Class 11 Chapter 5 is that it has been worked out by experts, who have worked tirelessly to provide precise solutions in a very comprehensive language.
5. Is Maths Class 11 Chapter 5 hard?
No, Class 11 Chapter 5 is not difficult for those who practice the exercises and examples given in the chapter daily. Practising the exercises and examples in the chapter will help you clear your basics and strengthening your understanding of concepts. To get a full solution to Class 11 Chapter 5 Linear Inequalities, download NCERT Solutions Class 11 Chapter 5 Linear Inequalities on Vedantu at free of cost or download the Vedantu app to access them for free.
6. How to express linear inequalities in two variables in Class 11 Chapter 5?
Linear inequalities in two variables can be expressed as ax + by < c or ax + by ≤ c or ax + by > c or ax + by ≥ c. If you want more solutions like these, then choose Vedantu’s best NCERT Solutions. Download and practice the NCERT Solutions Class 11 Chapter 5 Linear Inequalities now. Using this solution will improve how you perform in examinations and tests.
7. What are the important concepts to read to complete Class 11 Maths Chapter 5 Solutions Pdf?
The basics that you need to cover to wholly complete your preparations for Class 11 Maths Chapter 5 are understanding inequalities and linear inequalities, Linear Inequalities' Algebraic Solutions in one variable and two variables and their graphical representation. Class 11 Maths Chapter 5 Solutions Pdf Linear Inequalities on Vedantu will help you understand the chapter easily and in solving all the exercises.
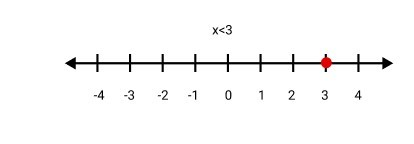
8. What are literal and numerical inequalities in Class 11 Maths Chapter 5 Solutions Pdf?
Literal inequalities are those inequalities that involve both variables and numbers. For eg, x < 3, y > 8, and x < 4 etc. Numerical inequalities, on the other hand, only involve numbers. For e.g., 5 < 7, 9 > 2, and 2 < 1 etc. To clear all other doubts in this chapter, get your hands on the best NCERT Solutions Class 11 Maths Chapter 5 Solutions Pdf Linear Inequalities on Vedantu. You can even download the PDF and keep revising it.
9. What is the main focus of Chapter 5 in Linear Inequalities Class 11 Solutions?
Linear Inequalities Class 11 Solutions is about understanding and solving linear inequalities. It explains how to handle inequalities in one variable and two variables. The chapter also covers how to represent the solutions graphically. By the end, students will be able to solve and graph these inequalities confidently.
10. How do I solve linear inequalities in one variable in Linear Inequalities Class 11 Solutions?
To solve linear inequalities in one variable, start by isolating the variable on one side of the inequality. If you multiply or divide both sides by a negative number, flip the inequality sign. Linear Inequalities Class 11 Solutions will be in the form of a range or interval notation, showing all possible values for the variable.
11. Can you explain how to graph linear inequalities in Class 11 Maths Linear Inequalities?
Graphing linear inequalities involves first plotting the related linear equation as a boundary line. Use a dashed line for '<' or '>' and a solid line for '≤' or '≥'. Then, determine which side of the line satisfies the inequality by testing points, and shade that region to show the solution set.
12. Are there any real-life applications of linear inequalities in Class 11 Maths Linear Inequalities?
Yes, linear inequalities are used in many real-life scenarios. They help in budgeting to ensure spending stays within limits. Businesses use them for optimizing production and profits. Linear inequalities also aid in scheduling tasks and allocating resources effectively under given constraints.