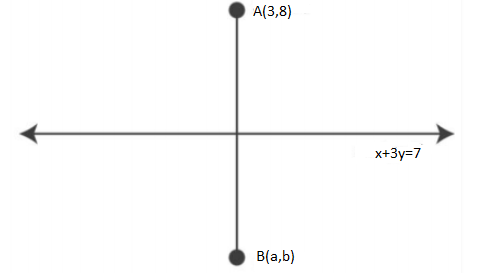
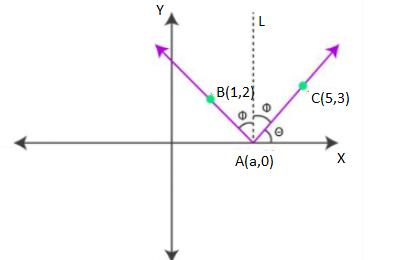
NCERT Solutions for Maths Class 11 Chapter 9 Straight Lines - FREE PDF Download






FAQs on NCERT Solutions for Class 11 Maths Chapter 9 Straight Lines
1. What are the topics discussed in Chapter 10 Maths Class 11?
NCERT Solutions for Class 11 Maths Chapter 10 introduces the students to coordinate geometry, which is a very crucial topic in the Class 11 Maths syllabus. Students will be required to understand the graphical representation of straight lines and their algebraic forms. They will also learn the form and applications of various representations of a first-degree equation besides the general equation of a line.
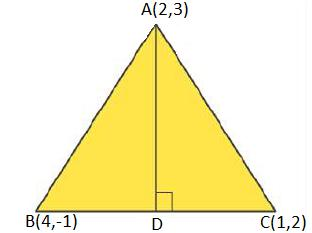
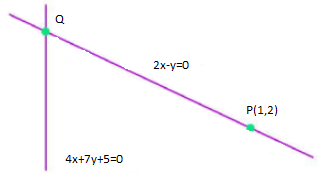
Calculation of the slope and the distance of an external point from a given line is discussed with relevant examples to help students solve problems based on these concepts efficiently.
2. What is the equation of a line written in general form?
There are various forms of an equation that a graphical line can be represented in. The first and most common form of a linear equation is the general equation.
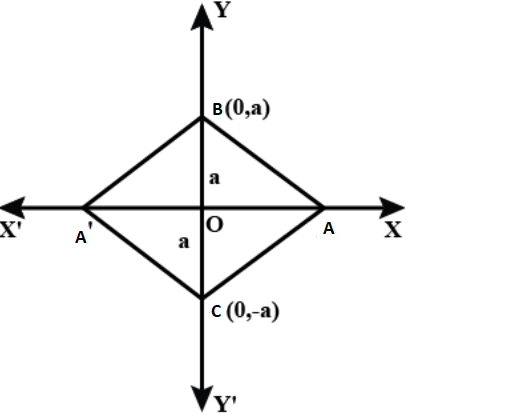
For a line represented in two variables x and y of the first degree, the general equation is given as Ax + By + C = 0, where coefficients A and B cannot be 0. A, B, and C are constants belonging to the set of real numbers, and variables x and y represent the coordinates on the respective axes.
3. How to find the slope of a line?
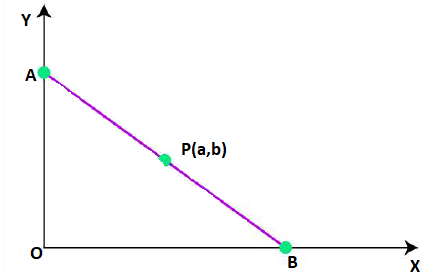
In Mathematics, the slope or gradient of a line, denoted by the letter m, describes the steepness and the direction of that line. Ideally the slope equation of a straight line is a linear function represented as y = f(x) = mx + c.
The average slope of a line is a constant for the same line and is calculated as a ratio between the differences of y-coordinates of two points, the rise, to that of x-coordinates of those points, the run.
You can use the slope equation in determining the slope by marking two points on a line and noting their x and y-coordinates. Next, determine the vertical and horizontal changes between those two points. For the final value, divide the difference between the y-coordinates by that of the x-coordinates. There you have slope m for a given line.
4. How many Exercises are there in Chapter 10 Maths Class 11?
There are a total of 3 Exercises in Class 11 Maths Chapter 10 'Straight Lines'. There are 14 questions in Exercise 10.1, 20 questions in Exercise 10.2 and 18 questions in Exercise 10.3. These exercises consist of questions that explain the fundamentals of coordinate geometry using graphical representations. To learn more, you can go through the NCERT solutions prepared by the team of Vedantu for easy learning.
5. How can I strengthen my concepts of Class 11 Maths Chapter 10?
Many students in Class 11 struggle to grasp the concepts of Mathematics. What they require is excellent study material on which they can rely for high grades. Along with NCERT exercises, students should practise various important questions and familiarise themselves with previous year papers in NCERT solutions. You will be able to maintain a solid grip on the subject if you clear your concepts and practise them regularly.
6. Is Chapter 10 Maths Class 11 important?
Yes, Chapter 10 Maths Class 11 is an important chapter and students should not neglect this chapter at any cost. It comprises 13% of the marks weightage in the exams. Leaving this chapter may be a bad idea for you. So, to prepare for chapter 10 thoroughly, you can take the help of the study guides provided on the website of Vedantu. For easier access, you can download the Vedantu app.
7. Can I download NCERT Solutions for Chapter 10 Maths Class 11?
Absolutely yes. You can download the NCERT solutions of Class 11 Maths Chapter 10 from the website of Vedantu or you can also download the Vedantu app to get easy access through your phone. These solutions are in downloadable PDF format and are totally free of cost. Once downloaded, you can study from your device anytime and anywhere.
8. How can I benefit from using Vedantu?
Choosing Vedantu brings you a load of advantages. Following are some benefits that you can avail yourself by using Vedantu:
It provides NCERT solutions to all the questions prepared by our professional tutors with years of experience.
Prepared notes and important questions are provided after extensive research and study.
Improves your grades as well as prepares you for competitive exams.
Provided study guides that strictly adheres to the CBSE curriculum.
9. What is the significance of understanding the slope of a line in class 11 maths chapter 9 solutions?
The slope of a line is a fundamental concept that indicates how steep a line is and its direction. Understanding the slope is crucial for solving problems related to parallel and perpendicular lines. It also helps in determining the angle of inclination of the line with respect to the x-axis. The slope is widely used in various applications, including physics, engineering, and computer graphics in class 11 maths chapter 9 solutions.
10. How does chapter 9 help in real-world applications in ncert straight lines class 11 solutions?
The concepts learned in ncert straight lines class 11 solutions, such as finding the equation of a line and calculating distances, are vital in fields like engineering, physics, and computer science. They help in designing and analysing structures, understanding motion and trajectories, and solving practical problems involving straight lines.
11. How many questions from chapter 9 were asked in previous year exams in chapter 9 class 11 maths?
On average, about 4–6 questions from chapter 9 class 11 maths have been asked in previous year exams. These questions often cover key topics like the slope, equations of lines, and angles between lines. This indicates the chapter's significance in the Class 11 Maths syllabus and highlights the need for thorough understanding and practice to perform well in exams.