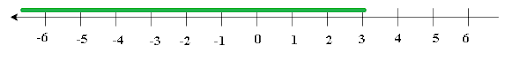
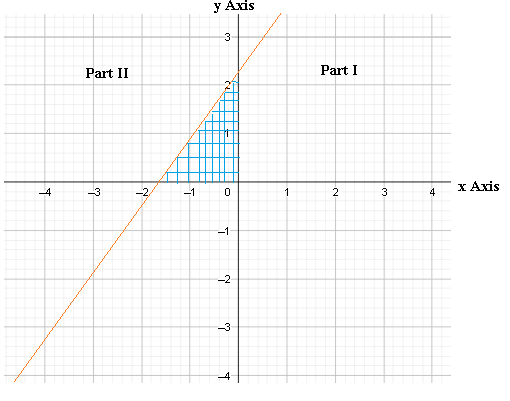
Revision Notes for CBSE Class 11 Maths Chapter 5 (Linear Inequalities) - Free PDF Download
FAQs on Linear Inequalities Class 11 Notes CBSE Maths Chapter 5 (Free PDF Download)
1. How will I get benefited with the revision notes class 11 maths chapter 5?
Linear Inequalities Class 11 Maths Notes designed by Vedantu experts are CBSE quick revision notes which are very helpful to not just revise a particular chapter but the entire syllabus during exam days. The revision notes cover all important concepts and formulas provided in the chapter. Even if you seek to have a general overview of a chapter, quick revision notes will make you have a quick look through in a fraction of time.
2. How do we find A Plane Containing Origin?
If the origin satiates the inequality of the half of the half plane, then the half plane consists of the origin. This inequality depicts the half plane consisting of an origin. On the other hand, if the origin does not satiate the inequality of the half of the half plane, then the half plane does not consist of the origin. This inequality denotes the half plane, which does not consist of the origin.
3. What is linear inequality according to Class 11 Maths Chapter 5?
According to Chapter 5 of Class 11 Maths, linear inequality is defined as:
The relation which is formed between two indifferent values is called an inequality.
Take, for example, t<4. In this, the relationship is between two values, t and 4 which are different.
The inequality relation between two real numbers is formed using comparison operators like <,>, etc.
In the field of mathematics, optimization problems, economics, science, psychology, etc the study of inequality is very useful.
4. Write the rules of inequality given in Chapter 5 of Class 11 Maths.
The rules of inequality mentioned in Chapter 5 of Class 11 Maths are as follows:
You can add or subtract the same number from both sides without affecting the sign of inequality.
Example –Z > 8
Z + 100 > 8 + 100
Z –3 > 8 – 1
Without affecting the sign of inequality, you can multiply or divide the same positive numbers on both sides.
Example –X < 6
X*2 <6*2
X ÷ 4 < 6 ÷ 4
The sign of inequality gets changed when both sides are divided or multiplied with the same negative number.
Example –W > 9
S * (-300) < 4 * (-300)
5. What are the various forms of inequality?
(a) Numerical Inequality – It is the relation between numbers.
Example- 5 > 4
(b) Double Inequality –One single value is related to the other two values.
Example- 2 < y <4
(c) Variable Inequality –The relationship between numbers and variables.
Examples- b > 1
(d) Slack Inequality –In this inequality relationship, more than equal to or less than equal to operators are used.
(e) Strict Inequality –This type of inequality uses the symbols of < or >.
Example- 6 >7 or y < 8
6. Explain quadratic inequality and linear inequality in one and two variables. Also, describe the terms solution set and solution.
Quadratic Inequality –It is the inequality that consists of a quadratic equation.
Linear Inequality in One Variable –The inequality which includes linear equations in one variable. Example- n > 5
Linear Inequality in Two Variables – This inequality has a linear equation in two variables. Example- y + z < 9
Solution Set – It is defined as the set of values for x which satisfies the inequality. Example- (6, 4, 2) is the solution set for x in inequality: x < 8
Solutions – These are values of x to make inequality a true statement. Example- for b > 5, 2 is a solution.
7. How should I prepare Chapter 5 for the Class 11 Maths examination?
Chapter 5 of Class 11 Maths is one of the easiest and scoring chapters. Students can prepare this chapter easily to score well in the Class 11 Maths examination. They can study from the NCERT book to understand the concepts or topics. By solving the exercises and examples, students can comprehend the chapter in a better way. Also, they should practice sample papers and previous years question papers to know about the type of questions asked in the exam.
If you want to understand these concepts more accurately then go through the given link CBSE Class 11 Chapter 5. This link will redirect you to the official website of Vedantu where you can access the content related to Chapter 10 Straight lines for free. Additionally, you can also download its PDF if you want to study offline.