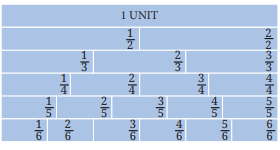
NCERT Solutions for Maths Class 6 Chapter 7 Fractions Exercise 7.6 - FREE PDF Download
NCERT Solutions for Maths Exercise 7.6 Class 6 Chapter 7 - Fractions by Vedantu provides a clear explanation of addition and subtraction of unlike fractions. This exercise focuses on helping students understand how to handle fractions with different denominators, a crucial skill for mastering fractions.
- 3.1Exercise 7.6
The solutions offer step-by-step guidance, making it easier for students to follow the process and solve the problems in their textbooks. By practicing Class 6 Maths NCERT Solutions, students can strengthen their grasp of the concepts and improve their problem-solving abilities. These solutions are a valuable resource for any student looking to excel in this chapter. Find the Class 6 Maths Syllabus here.
Glance on NCERT Solutions Maths Chapter 7 Exercise 7.6 Class 6 | Vedantu
NCERT Solutions Maths Chapter 7 Exercise 7.6 covers addition and subtraction of unlike fractions.
Unlike fractions have different denominators, which need to be made the same before performing operations.
Find a common denominator for the fractions, add the numerators, and simplify the resulting fraction.
The common denominator is the least common multiple (LCM) of the denominators of the fractions being added or subtracted.
Simplify fractions by dividing the numerator and the denominator by their greatest common divisor (GCD).
This article contains exercise notes, important questions, exemplar solutions, exercises, and video links for Exercise 7.6 - Fractions, which you can download as PDFs.
There are 9 fully solved questions in Chapter 7 Exercise 7.6 Fractions.
Exercise 7.6
1. Solve the following:
$\dfrac{2}{3} + \dfrac{1}{7}$
Ans: Take the LCM of denominator to find the above sum.
The LCM of 7 and 3 is 21.
\[\dfrac{2}{3} + \dfrac{1}{7} = \dfrac{{2 \times 7 + 1 \times 3}}{{21}}\]
\[\dfrac{2}{3} + \dfrac{1}{7} = \dfrac{{14 + 3}}{{21}}\]
\[\dfrac{2}{3} + \dfrac{1}{7} = \dfrac{{17}}{{21}}\]
Thus, the sum is $\dfrac{{17}}{{21}}$.
$\dfrac{3}{{10}} + \dfrac{7}{{15}}$
Ans: Take the LCM of denominator to find the above sum.
The LCM of 10 and 15 is 30.
\[\dfrac{3}{{10}} + \dfrac{7}{{15}} = \dfrac{{3 \times 3 + 7 \times 2}}{{30}}\]
\[\dfrac{3}{{10}} + \dfrac{7}{{15}} = \dfrac{{9 + 14}}{{30}}\]
\[\dfrac{3}{{10}} + \dfrac{7}{{15}} = \dfrac{{23}}{{30}}\]
Thus, the sum is \[\dfrac{{23}}{{30}}\].
$\dfrac{4}{9} + \dfrac{2}{7}$
Ans: Take the LCM of denominator to find the above sum.
The LCM of 9 and 7 is 63.
\[\dfrac{4}{9} + \dfrac{2}{7} = \dfrac{{4 \times 7 + 2 \times 9}}{{63}}\]
\[\dfrac{4}{9} + \dfrac{2}{7} = \dfrac{{28 + 18}}{{63}}\]
\[\dfrac{4}{9} + \dfrac{2}{7} = \dfrac{{46}}{{63}}\]
Thus, the sum is \[\dfrac{{46}}{{63}}\].
$\dfrac{5}{7} + \dfrac{1}{3}$
Ans: Take the LCM of denominator to find the above sum.
The LCM of 7 and 3 is 21.
\[\dfrac{5}{7} + \dfrac{1}{3} = \dfrac{{5 \times 3 + 1 \times 7}}{{21}}\]
\[\dfrac{5}{7} + \dfrac{1}{3} = \dfrac{{15 + 7}}{{21}}\]
\[\dfrac{5}{7} + \dfrac{1}{3} = \dfrac{{22}}{{21}}\]
Thus, the sum is \[\dfrac{{22}}{{21}}\].
$\dfrac{2}{5} + \dfrac{1}{6}$
Ans: Take the LCM of denominator to find the above sum.
The LCM of 5 and 6 is 30.
\[\dfrac{2}{5} + \dfrac{1}{6} = \dfrac{{2 \times 6 + 1 \times 5}}{{30}}\]
\[\dfrac{2}{5} + \dfrac{1}{6} = \dfrac{{12 + 5}}{{30}}\]
\[\dfrac{2}{5} + \dfrac{1}{6} = \dfrac{{17}}{{30}}\]
Thus, the sum is \[\dfrac{{17}}{{30}}\].
$\dfrac{4}{5} + \dfrac{2}{3}$
Ans: Take the LCM of denominator to find the above sum.
The LCM of 5 and 3 is 15.
\[\dfrac{4}{5} + \dfrac{2}{3} = \dfrac{{4 \times 3 + 2 \times 5}}{{15}}\]
\[\dfrac{4}{5} + \dfrac{2}{3} = \dfrac{{12 + 10}}{{15}}\]
\[\dfrac{4}{5} + \dfrac{2}{3} = \dfrac{{22}}{{15}}\]
Thus, the sum is \[\dfrac{{22}}{{15}}\].
$\dfrac{3}{4} - \dfrac{1}{3}$
Ans: Take the LCM of denominator to find the above difference.
The LCM of 4 and 3 is 12.
\[\dfrac{3}{4} - \dfrac{1}{3} = \dfrac{{3 \times 3 - 1 \times 4}}{{12}}\]
\[\dfrac{3}{4} - \dfrac{1}{3} = \dfrac{{9 - 4}}{{12}}\]
\[\dfrac{3}{4} - \dfrac{1}{3} = \dfrac{5}{{12}}\]
Thus, the difference is \[\dfrac{5}{{12}}\].
$\dfrac{5}{6} - \dfrac{1}{3}$
Ans: Take the LCM of denominator to find the above difference.
The LCM of 6 and 3 is 6.
\[\dfrac{5}{6} - \dfrac{1}{3} = \dfrac{{5 \times 1 - 1 \times 2}}{6}\]
\[\dfrac{5}{6} - \dfrac{1}{3} = \dfrac{{5 - 2}}{6}\]
\[\dfrac{5}{6} - \dfrac{1}{3} = \dfrac{3}{6}\]
Thus, the difference is \[\dfrac{3}{6}\].
$\dfrac{2}{3} + \dfrac{3}{4} + \dfrac{1}{2}$
Ans: Take the LCM of denominator to find the above sum.
The LCM of 3, 4, and 2 is 12.
$\dfrac{2}{3} + \dfrac{3}{4} + \dfrac{1}{2} = \dfrac{{2 \times 4 + 3 \times 3 + 1 \times 6}}{{12}}$
$\dfrac{2}{3} + \dfrac{3}{4} + \dfrac{1}{2} = \dfrac{{8 + 9 + 6}}{{12}}$
$\dfrac{2}{3} + \dfrac{3}{4} + \dfrac{1}{2} = \dfrac{{23}}{{12}}$
Thus, the sum is $\dfrac{{23}}{{12}}$.
$\dfrac{1}{2} + \dfrac{1}{3} + \dfrac{1}{6}$
Ans: Take the LCM of denominator to find the above sum.
The LCM of 2, 3, and 6 is 6.
$\dfrac{1}{2} + \dfrac{1}{3} + \dfrac{1}{6} = \dfrac{{1 \times 3 + 1 \times 2 + 1 \times 1}}{6}$
$\dfrac{1}{2} + \dfrac{1}{3} + \dfrac{1}{6} = \dfrac{{3 + 2 + 1}}{6}$
$\dfrac{1}{2} + \dfrac{1}{3} + \dfrac{1}{6} = \dfrac{6}{6}$
Thus, the sum is $\dfrac{6}{6}$.
$1\dfrac{1}{3} + 3\dfrac{2}{3}$
Ans: Covert the mixed fraction into proper fractions.
$1\dfrac{1}{3} + 3\dfrac{2}{3} = \dfrac{4}{3} + \dfrac{{11}}{3}$
Take the LCM of denominator to find the above sum.
$\dfrac{4}{3} + \dfrac{{11}}{3} = \dfrac{{4 + 11}}{3}$
$\dfrac{4}{3} + \dfrac{{11}}{3} = \dfrac{{15}}{3}$
Thus, the sum is $\dfrac{{15}}{3}$.
$4\dfrac{2}{3} + 3\dfrac{1}{4}$
Ans: Covert the mixed fraction into proper fractions.
$4\dfrac{2}{3} + 3\dfrac{1}{4} = \dfrac{{14}}{3} + \dfrac{{13}}{4}$
Take the LCM of denominator to find the above sum.
The LCM of 3 and 4 is 12.
$\dfrac{{14}}{3} + \dfrac{{13}}{4} = \dfrac{{14 \times 4 + 13 \times 3}}{{12}}$
$\dfrac{{14}}{3} + \dfrac{{13}}{4} = \dfrac{{56 + 39}}{{12}}$
$\dfrac{{14}}{3} + \dfrac{{13}}{4} = \dfrac{{95}}{{12}}$
Thus, the sum is $\dfrac{{95}}{{12}}$.
$\dfrac{{16}}{5} - \dfrac{7}{5}$
Ans: Take the LCM of denominator to find the above difference.
\[\dfrac{{16}}{5} - \dfrac{7}{5} = \dfrac{{16 - 7}}{5}\]
\[\dfrac{{16}}{5} - \dfrac{7}{5} = \dfrac{9}{5}\]
Thus, the difference is \[\dfrac{9}{5}\].
$\dfrac{4}{3} - \dfrac{1}{2}$
Ans: Take the LCM of denominator to find the above difference.
The LCM of 3 and 2 is 6.
\[\dfrac{4}{3} - \dfrac{1}{2} = \dfrac{{4 \times 2 - 1 \times 3}}{6}\]
\[\dfrac{4}{3} - \dfrac{1}{2} = \dfrac{{8 - 3}}{6}\]
\[\dfrac{4}{3} - \dfrac{1}{2} = \dfrac{5}{6}\]
Thus, the difference is \[\dfrac{5}{6}\].
2. Sarika bought $\dfrac{2}{5}$ meter of ribbon and Lalita $\dfrac{3}{4}$ meter of ribbon. What is the total length of the ribbon they bought?
Ans: The total amount of ribbon bought by Sarita is $\dfrac{2}{5}$ meter and the ribbon bought by Lalita is $\dfrac{3}{4}$ meter.
To find the total length of the ribbon bought, we will add both the measurements.
${\text{Total length}} = \dfrac{2}{5} + \dfrac{3}{4}$
Take the LCM of denominator to find the above sum.
The LCM of 4 and 5 is 20.
\[{\text{Total length}} = \dfrac{{2 \times 4 + 5 \times 3}}{{20}}\]
\[{\text{Total length}} = \dfrac{{8 + 15}}{{20}}\]
\[{\text{Total length}} = \dfrac{{23}}{{20}}\]
Hence, the total length of ribbon bought is $\dfrac{{23}}{{20}}$ meter.
3. Naina was given $1\dfrac{1}{2}$ piece of cake and Najma was given $1\dfrac{1}{3}$ piece of cake. Find the total amount of cake given to both of them.
Ans: Naina got $1\dfrac{1}{2}$piece of cake, that is, $\dfrac{3}{2}$ piece of cake.
Najma bought $1\dfrac{1}{3}$ piece of cake, that is, $\dfrac{4}{3}$ piece of cake.
So, add the fractions to get the total amount of cake that was given to Naina and Najma.
${\text{Total cake}} = \dfrac{3}{2} + \dfrac{4}{3}$.
To find the above addition, we will take the LCM of the denominators and solve.
The LCM of 2 and 3 is 6.
${\text{Total cake}} = \dfrac{{3 \times 3 + 4 \times 2}}{6}$
${\text{Total cake}} = \dfrac{{9 + 8}}{6}$
${\text{Total cake}} = \dfrac{{17}}{6}$
Hence, the total amount of cake they both have is $\dfrac{{17}}{6}$.
4. Fill in the boxes.
$\boxed{} - \dfrac{5}{8} = \dfrac{1}{4}$
Ans:Add $\dfrac{5}{8}$ to both sides and take LCM of 4 and 8.
The LCM of 4 and 8 is 32.
\[\dfrac{1}{4} + \dfrac{5}{8} = \dfrac{{1 \times 2 + 1 \times 5}}{8}\]
$\dfrac{1}{4} + \dfrac{5}{8} = \dfrac{{2 + 5}}{8}$
$\dfrac{1}{4} + \dfrac{5}{8} = \dfrac{7}{8}$
Hence, the missing fraction is $\dfrac{7}{8}$.
$\boxed{} - \dfrac{1}{5} = \dfrac{1}{2}$
Ans:Add $\dfrac{1}{5}$ to both sides and take LCM of 2 and 5.
The LCM of 2 and 5 is 10.
\[\dfrac{1}{5} + \dfrac{1}{2} = \dfrac{{1 \times 2 + 1 \times 5}}{{10}}\]
$\dfrac{1}{5} + \dfrac{1}{2} = \dfrac{{2 + 5}}{{10}}$
$\dfrac{1}{5} + \dfrac{1}{2} = \dfrac{7}{{10}}$
Hence, the missing fraction is $\dfrac{7}{{10}}$.
$\dfrac{1}{2} - \boxed{} = \dfrac{1}{6}$
Ans: Subtract $\dfrac{1}{6}$ from both sides.
Take LCM of 2 and 6.
The LCM of 2 and 6 is 6.
$\dfrac{1}{2} - \dfrac{1}{6} = \dfrac{{1 \times 3 - 1 \times 1}}{6}$
$\dfrac{1}{2} - \dfrac{1}{6} = \dfrac{{3 - 1}}{6}$
$\dfrac{1}{2} - \dfrac{1}{6} = \dfrac{2}{6}$
Hence, the missing fraction is $\dfrac{2}{6}$.
5. Complete the addition-subtraction box:
Ans: To complete the given box, add the rows and subtract the column simultaneously.
Add $\dfrac{2}{3}$and $\dfrac{4}{3}$.
$\dfrac{2}{3} + \dfrac{4}{3} = \dfrac{{2 + 4}}{3}$
$\dfrac{2}{3} + \dfrac{4}{3} = \dfrac{6}{3}$
Add $\dfrac{1}{3}$ and $\dfrac{2}{3}$.
$\dfrac{1}{3} + \dfrac{2}{3} = \dfrac{{1 + 2}}{3}$
$\dfrac{1}{3} + \dfrac{2}{3} = \dfrac{3}{3}$
Subtract $\dfrac{2}{3}$ and $\dfrac{1}{3}$.
$\dfrac{2}{3} - \dfrac{1}{3} = \dfrac{{2 - 1}}{3}$
$\dfrac{2}{3} - \dfrac{1}{3} = \dfrac{1}{3}$
Subtract $\dfrac{4}{3}$ and $\dfrac{2}{3}$.
$\dfrac{4}{3} - \dfrac{2}{3} = \dfrac{{4 - 2}}{3}$
$\dfrac{4}{3} - \dfrac{2}{3} = \dfrac{2}{3}$
Now, complete the third column. To do so, subtract $\dfrac{6}{3}$ and $\dfrac{3}{3}$.
$\dfrac{6}{3} - \dfrac{3}{3} = \dfrac{{6 - 3}}{3}$
$\dfrac{6}{3} - \dfrac{3}{3} = \dfrac{3}{3}$
Therefore, the complete box is as follows.
b)
Ans:
Add $\dfrac{1}{2}$ and $\dfrac{1}{3}$.
Therefore, $\dfrac{1}{2}$ + $\dfrac{1}{3}$
= $\dfrac{{(3\times 1) + (2\times 1)}}{6}$
= $\dfrac{5}{6}$
Add $\dfrac{1}{3}$ and $\dfrac{1}{4}$
$\dfrac{1}{3}$ + $\dfrac{1}{4}$
= $\dfrac{{(4\times 1) + (3\times 1)}}{12}$
= $\dfrac{7}{12}$
Now subtract $\dfrac{1}{3}$ from $\dfrac{1}{2}$
We get,
$\dfrac{1}{2}$ - $\dfrac{1}{3}$
=$\dfrac{{(3\times 1) - (2\times 1)}}{6}$
= $\dfrac{1}{6}$
Subtract $\dfrac{1}{4}$ from $\dfrac{1}{3}$
$\dfrac{1}{3}$-$\dfrac{1}{4}$
=$\dfrac{{(4\times 1) - (3\times 1)}}{12}$
=$\dfrac{1}{12}$
At last add $\dfrac{1}{6}$ and $\dfrac{1}{12}$
$\dfrac{1}{6}$ + $\dfrac{1}{12}$
=$\dfrac{{(2\times 1) + (1\times 1)}}{12}$
=$\dfrac{3}{12}$
=$\dfrac{1}{4}$
Therefore, the complete box is as follows.
6. A piece of wire $\dfrac{7}{8}$ meter long broke into two pieces. One piece was $\dfrac{1}{4}$ meter long. How long is the other piece?
Ans: The total length of the wire is $\dfrac{7}{8}$ meter. The length of the first piece is $\dfrac{1}{4}$ meter.
The length of remaining part will be,
${\text{Remaining part}} = \dfrac{7}{8} - \dfrac{1}{4}$
Take the LCM of denominator to find the above difference.
The LCM of 4 and 8 is 32.
${\text{Remaining part}} = \dfrac{{7 - 2}}{8}$
${\text{Remaining part}} = \dfrac{5}{8}$
Therefore, the length of the remaining part $\dfrac{5}{8}$ meter.
7. Nandini house is $\dfrac{9}{{10}}$ km from her school. She walked some distance and then took as bus for $\dfrac{1}{2}$ km to reach the school. How far did she walk?
Ans: The total distance between school and house is $\dfrac{9}{{10}}$ km and the distance covered by bus is $\dfrac{1}{2}$ km.
The distance she has to walk can be found out by finding the difference between the distance covered by bus and distance between school and house.
\[{\text{Remaining distance}} = \dfrac{9}{{10}} - \dfrac{1}{2}\]
Take the LCM of denominator to find the above difference.
The LCM of 10 and 2 is 10.
\[{\text{Remaining distance}} = \dfrac{{9 - 5}}{{10}}\]
\[{\text{Remaining distance}} = \dfrac{4}{{10}}\]
Thus, the remaining distance is $\dfrac{4}{{10}}$ km.
8. Ahsa and Samuel have bookshelves of the same size partly filled with books. Asha’s shelf is${\dfrac{5}{6}^{{\text{th}}}}$full and Samuel’s shelf is ${\dfrac{2}{5}^{{\text{th}}}}$ full. Whose bookshelf is filled with more books? By what fraction?
Ans: To solve this, we will make the denominator of both the fraction equal.
To do so, multiply the numerator and denominator of $\dfrac{5}{6}$ and $\dfrac{2}{5}$ by 5 and 6 respectively.
$\dfrac{{5 \times 5}}{{6 \times 5}} = \dfrac{{25}}{{30}}$
$\dfrac{{2 \times 6}}{{5 \times 6}} = \dfrac{{12}}{{30}}$
Thus, $\dfrac{{25}}{{30}}\boxed > \dfrac{{12}}{{30}}$.
Therefore, $\dfrac{5}{6}\boxed > \dfrac{2}{5}$. Asha’s bookshelf is more covered than Samuel.
The difference between the books they both have is,
\[{\text{Difference}} = \dfrac{{25}}{{30}} - \dfrac{{12}}{{30}}\]
\[{\text{Difference}} = \dfrac{{25 - 12}}{{30}}\]
\[{\text{Difference}} = \dfrac{{13}}{{30}}\]
Thus, Asha’s book shelf is covered with more books and she has $\dfrac{{13}}{{30}}$ more books in her shelf.
9. Jaidev takes $2\dfrac{1}{5}$ minutes to walk across the school ground. Rahul takes $\dfrac{7}{4}$ minutes to do same. Who takes less time and by what fraction?
Ans: The total time taken by Jaidev is$2\dfrac{1}{5}$ minutes.
On converting $2\dfrac{1}{5}$ we get,
$2\dfrac{1}{5} = \dfrac{{11}}{5}{\text{minutes}}$
The total time taken by Rahul is $\dfrac{7}{4}$ minutes.
To find who takes less time we will find the difference between time taken by Jaidev and Rahul.
$\dfrac{{11}}{5} - \dfrac{7}{4}$
Take LCM of 5 and 4. Thus, the LCM of 5 and 4 is 20.
${\text{Difference}} = \dfrac{{11 \times 4 - 7 \times 5}}{{20}}$
${\text{Difference}} = \dfrac{{44 - 35}}{{20}}$
${\text{Difference}} = \dfrac{9}{{20}}\,{\text{minutes}}$
Thus, Rahul takes less time, which is $\dfrac{9}{{20}}$ minutes.
Conclusion
NCERT Solutions for Maths Exercise 7.6 Class 6 Chapter 7 - Fractions by Vedantu are essential for understanding the addition and subtraction of unlike fractions. It is important to focus on finding the least common denominator and converting fractions to like fractions before performing operations. These solutions provide clear, step-by-step guidance, helping students master these concepts. Practising these exercises will strengthen problem-solving skills and build a solid foundation in fractions, which is important for scoring in maths exams.
Class 6 Maths Chapter 7: Exercises Breakdown
CBSE Class 6 Maths Chapter 7 Other Study Materials
Chapter-Specific NCERT Solutions for Class 6 Maths
Given below are the chapter-wise NCERT Solutions for Class 6 Maths. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.
FAQs on NCERT Solutions for Class 6 Maths Chapter 7 - Fractions Exercise 7.6
1. What is covered in Ex 7.6 Class 6 of Chapter 7 in Maths?
Ex 7.6 Class 6 focuses on the addition and subtraction of unlike fractions. These fractions have different denominators, making it necessary to find a common denominator before performing any arithmetic operations.
2. What are unlike fractions in Ex 7.6 Class 6?
From Ex 7.6 Class 6, Unlike fractions are those with different denominators. To add or subtract them, they must first be converted to like fractions with a common denominator. This process is essential for accurate calculations.
3. Why is it important to learn about unlike fractions in Ex 7.6 Class 6?
Learning about unlike fractions is important because it helps in understanding how to add and subtract fractions with different denominators, a key skill in maths Ex 7.6 Class 6.
4. What should students focus on in Class 6 Ex 7.6?
Students should focus on Class 6 Ex 7.6 finding a common denominator for unlike fractions and then converting them into like fractions to add or subtract them.
5. How do the NCERT Solutions help with Class 6 Ex 7.6?
The NCERT Solutions Class 6 Ex 7.6 provide step-by-step explanations and examples that make it easier to understand and solve problems involving unlike fractions.
6. Are there any tips for solving problems in Class 6 Ex 7.6?
Yes, ensure to find the least common multiple of the denominators and convert all fractions to have this common denominator before performing addition or subtraction.
7. Why are examples included in the NCERT Solutions for Exercise 7.6?
Examples are included to illustrate the steps involved in adding and subtracting unlike fractions, helping students understand the process better.
8. How can practising Class 6 Maths Ex 7.6 improve my maths skills?
Practicing Class 6 Maths Ex 7.6Exercise 7.6 can improve your ability to handle fractions, enhance problem-solving skills, and build a strong foundation in basic arithmetic operations.
9. What are fractions in Class 6 Maths Ex 7.6?
Like fractions are fractions that have the same denominator.
10. Is it necessary to convert unlike fractions to like fractions in Class 6 Maths Ex 7.6?
Yes, converting unlike fractions to like fractions is necessary to perform addition and subtraction correctly.
11. What challenges might students face in Exercise 7.6?
Students might find it challenging to find the least common denominator and convert fractions correctly.
12. How can NCERT Solutions Class 6 Maths Ch 7 Ex 7.6 help overcome these challenges?
The NCERT Solutions Class 6 Maths Ch 7 Ex 7.6, provide clear, detailed steps and examples that guide students through the process, making it easier to understand and apply the concepts.
13. What is the benefit of using the NCERT Solutions by Vedantu for Class 6 Maths Ch 7 Ex 7.6?
The benefit is that these solutions offer expert guidance, making complex concepts simple and accessible, which helps students excel in their studies.