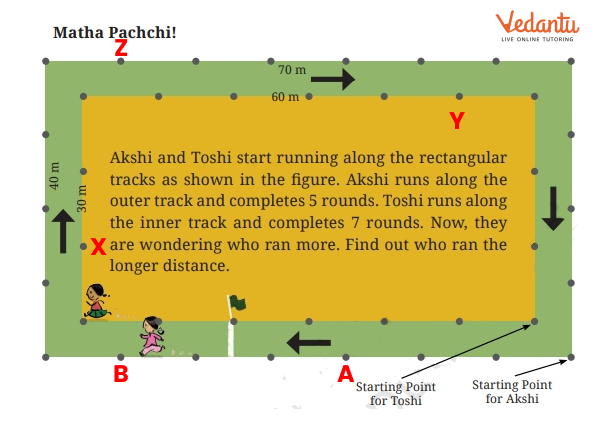
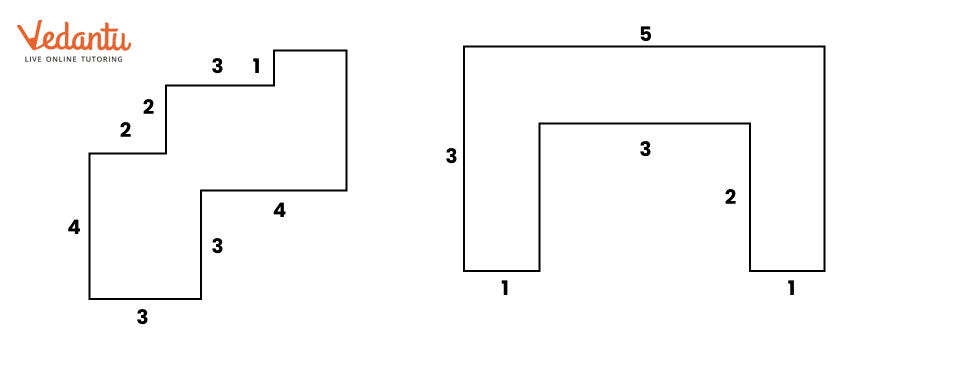
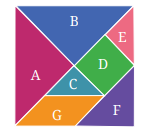
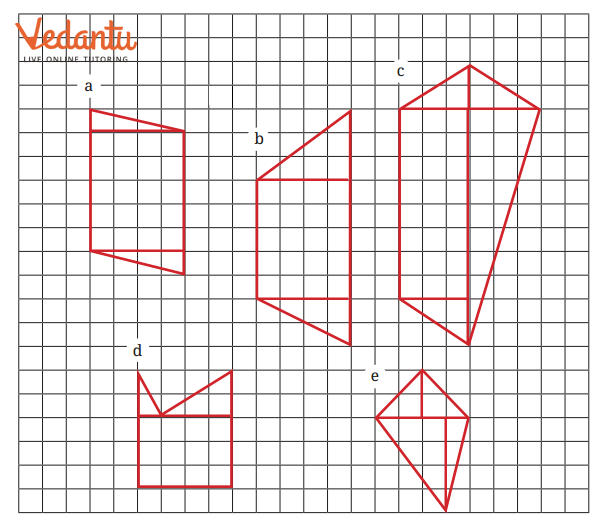
NCERT Solutions for Class 6 Chapter 6 Maths - FREE PDF Download
FAQs on NCERT Solutions for Class 6 Maths Chapter 6: Perimeter and Area
1. What is included in the NCERT Solutions for Chapter 6?
The NCERT Solutions for Chapter 6 include perimeter and area explanations and practice exercises. Each exercise is designed to help students understand key concepts. This makes learning more effective and structured.
2. How are the solutions organised in the notes?
The solutions are organised by exercise, making it easy to find specific topics. Each exercise includes clear steps and examples to guide students. This structure helps in better understanding and practice.
3. Are there any real-life applications in the NCERT Solutions for Maths Class 6 Perimeter and Area chapter-6?
Yes, the NCERT Solutions for Maths Class 6 Perimeter and Area chapter-6 include examples showing how perimeter and area are used in daily life. These applications help students see the relevance of math concepts. It makes learning more engaging and practical.
4. What types of exercises are provided in NCERT Solutions for Maths Class 6 Perimeter and Area chapter-6?
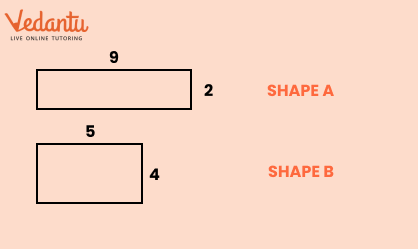
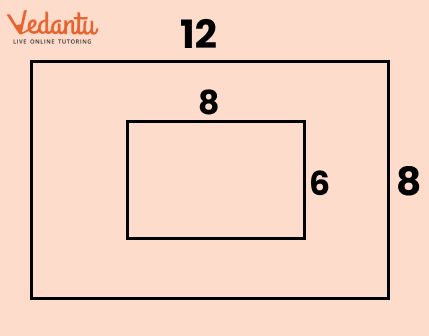
The notes provide a variety of exercises, including calculating the perimeter and area for different shapes. There are also word problems to enhance problem-solving skills. This variety helps students practice comprehensively.
5. Can I find tips for solving problems in the NCERT Solutions for Maths Class 6 Perimeter and Area?
The notes include tips and tricks for solving perimeter and area problems. These tips simplify complex concepts and make it easier to remember formulas. They are helpful for students during practice and exams.
6. How do the NCERT Solutions for Maths Class 6 Perimeter and Area help with understanding shapes?
The solutions break down the properties of different shapes, such as squares and triangles. They explain how to apply the formulas for calculating perimeter and area. This helps students gain a solid understanding of geometric concepts.
7. Is there a focus on measurement units in the NCERT Solutions for Maths Class 6 Perimeter and Area chapter-6?
Yes, the NCERT Solutions for Maths Class 6 Perimeter and Area chapter-6 emphasise different measurement units for perimeter and area, such as meters and square units. Understanding these units is crucial for accurate calculations. It prepares students for real-world applications.
8. Are the explanations in the NCERT Solutions for Maths Class 6 Perimeter and Area chapter-6 easy to follow?
Yes, the explanations are written in simple language. Each step is clear, making it easy for students to understand the concepts. This approach helps build confidence in tackling math problems.
9. What can I do if I struggle with the exercises?
If you struggle with the exercises, review the explanations provided in the NCERT Solutions for Maths Class 6 Perimeter and Area. You can also revisit earlier examples for clarification. Practice is key, and the notes are designed to help reinforce understanding.
10. How can I download the NCERT Solutions?
The NCERT Solutions for Chapter 6 are available for free download in PDF format. You can access them anytime for study and practice. This convenience supports flexible learning for students.