NCERT Solutions for Class 6 Maths Chapter 3: Number Play

3.1 Numbers Can Tell Everything 

1. Can the children rearrange themselves so that the children standing at the ends say ‘2’?

Yes, the children can rearrange themselves so that the children at the ends say ‘2’.

2. Can we arrange the children in a line so that all would say only 0s?

Yes, it is possible to arrange the children in a line where all of them say only 0s.

3. Can two children standing next to each other say the same number?

Yes, it is possible for two children standing next to each other to say the same number.

4. There are 5 children in a group, all of different heights. Can they stand such that four of them say ‘1’ and the last one says ‘0’? Why or why not?

No, they cannot stand in such a way because there are only 5 children, and each child must give an individual number. It is not possible to assign four of them '1' and the last one '0' while maintaining distinct heights for all.

5. For this group of 5 children, is the sequence 1, 1, 1, 1, 1 possible?

No, the sequence 1, 1, 1, 1, 1 is not possible because all children cannot say the same number if the numbers represent distinct values or actions.

6. Is the sequence 0, 1, 2, 1, 0 possible? Why or why not?

Yes, the sequence 0, 1, 2, 1, 0 is possible because the numbers can represent a symmetrical arrangement or relationship among the children, which is achievable.

7. How would you rearrange the five children so that the maximum number of children say ‘2’?

To maximize the number of children saying '2', you could arrange them in such a way that as many children as possible are assigned to say ‘2’ while ensuring that the other conditions or rules are met (though the exact conditions would need clarification).

3.2 Numbers can Tell us Things 3.2 Supercells Figure it Out (Page No. 57-58)

Question 1. Colour or mark the supercells in the table below.

Solution:

Question 2. Fill the table below with only 4-digit numbers such that the supercells are exactly the c cells.

Solution:

Question 3. Fill the table below such that we get as many supercells as possible. Use numbers between 100 and 1000 without repetitions.

Solution:

Question 4. Out of the 9 numbers, how many supercells are there in the table above?
Solution: Out of 9 numbers, there are 5 supercells in the above table.

Question 5. Find out how many supercells are possible for different numbers of cells. Do you notice any pattern? What is the method to fill a given table to get the maximum number of supercells? Explore and share your strategy.
Solution:
If there are n odd cells then number of supercells = \(\frac{n+1}{2}\)
If there are n even cells then number of supercells = \(\frac{n}{2}\)
Yes, there is a pattern. Alternate cells can be supercells.

Method to fill a given table to get the maximum number of supercells.

• Make first cell as supercell. After that each alternate cell is to be made supercell.

• No consecutive cells can be supercell except in case of 4 cells because then first and fourth cell can be supercell.

Question 6. Can you fill a supercell table without repeating numbers such that there are no supercells? Why or why not?
Solution: No, it is not possible to fill a supercell table without repeating numbers such that there are no supercells.
As there are two cases:
Case I: If we fill the cells in descending order then the first cell be supercell.
Case II: If we fill the cells in ascending order then the last cell will be supercell.
If we don’t follow any order, then there will atleast one supercell.

Question 7. Will the cell having the largest number in a table always be a super cell? Can the cell having the smallest number in a table be a supercell? Why or why not?
Solution: Yes, the cell having the largest number in a table always be a supercell because if it is comer cell, then the number adjacent to it (i.e. either second cell or second last cell) will be smaller than it. If it is in between then both its adjacent number would be smaller than it.
No, the cell having smallest number in a table can not be supercell because number adjacent to it will always be larger/greater than it.

Question 8. Fill a table such that the cell having the second largest number is not a supercell.
Solution:

Here 980 is the second largest number but it is not a supercell as 999 is the supercell.

Question 9. Fill a table such that the cell having the second largest number is not a supercell but the second smallest number is a super cell. Is it possible?
Solution:

Here 1870 is the second largest number but the cell having 1870 is not a supercell because number 1895 (adjacent to it) is greater than it.

489 is the second smallest number but the cell having 489 is a supercell because adjacent number 475 is smaller to it.

Question 10. Make other variations of this puzzle and challenge your classmates.
Solution:
Fill a table such that only even numbers are supercell.
Fill a table such that all the supercells are divisible by 5.

Intext Question

Question 1. Complete Table 2 with 5-digit numbers whose digits are ‘1’, ‘0’, ‘6’. ‘3’, and ‘9’ in some order. Only a coloured cell should have a number greater than all its neighbours. (Page 58)
Solution:

The biggest number in the table is 96, 310.
The smallest even number in the table is 10,936.
The smallest number greater than 50,000 in the table is 60,193.

3.3 Patterns of Numbers on the Number Line Figure it Out (Page No. 59)

Question 1. Identify the numbers marked on the number lines below, and label the remaining positions.

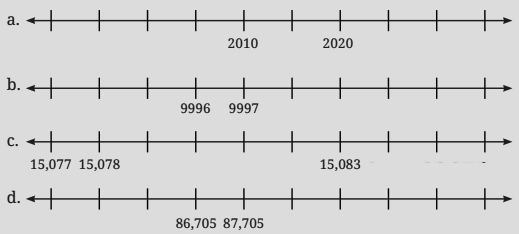
Put a circle around the smallest number and a box around the largest number in each of the sequences above.
Answer:

3.4 Playing with Digits (Page No. 60)

Question 1. Digit sum 14
(a) Write other numbers whose digits add up to 14.
(b) What is the smallest number whose digit sum is 14?
(c) What is the largest 5-digit whose digit sum is 14?
(b) How big a number can you form having the digit sum 14? Can you make an even bigger number?
Solution:
(a) Some numbers whose digits add up to 14 are:
59, 68, 77, 86, 95, 149, 158, 167, 176, 185, 194, 239, 248, 257, 266, 275, 281, 293

(b) The smallest number whose digit sum is 14 = 59.

(c) The largest 5 digit number containing 0 whose digit sum is 14 = 95,000.
The largest 5 digit number not containing 0 whose digit sum is 14 = 92,111.

(d) A very big number having the digit sum 14 can be made. e.g. 95000000000000.
Yes, we can make even bigger number e.g. 9500000000000000000000.

Question 2. Find out the digit sums of all the numbers from 40 to 70. Share your observations with the class.
Solution:

Question 3. Calculate the digit sums of 3-digit numbers whose digits are consecutive (for example, 345). Do you see a pattern? Will this pattern continue?
Solution:

If we take numbers in reverse order, sum of digits will remain same.
Yes, we observe a pattern.
i. e. (first number + 1) × 3 = digit sum.

Intext Question

Question 1. Among the numbers 1-100, how many times will the digit ‘7’occur?
Solution: The total count of 7 that we get is 20.

Question 2. Among the numbers 1-1000, how many times will the digit ‘7’occur?
Solution: The number of times 7 will be written when listing the numbers from 1 to 1000 is 300.

3.5 Pretty Palindromic Patterns 3.6 The Magic Number of Kaprekar 3.7 Clock and Calendar Numbers Figure it Out (Page No. 64-65)

Question 1. Pratibha uses the digits ‘4‘7’, ‘3’and ‘2’ and makes the smallest and largest 4-digit numbers with them: 2347 and 7432. The difference between these two numbers is 7432 – 2347 = 5085. The sum of these two numbers is 9779. Choose 4-digits to make:
(a) the difference between the largest and smallest numbers greater than 508$.
(b) the difference between the largest and smallest numbers less than 5085.
(e) the sum of the largest and smallest numbers greater than 9779.
(d) the sum of the largest and smallest numbers less than 9779.
Solution:
(a) Digits – 8, 7, 3 and 2
Largest Number – 8 7 3 2
Smallest Number – 2 3 7 8
Difference = 6 3 5 4

6354 > 5085

(b) Digits – 1, 2, 3 and 4
Largest Number – 4 3 2 1
Smallest Number – 1 2 3 4
Difference = 3 0 8 7

3087 < 5085

(c) Digits – 9, 8, 7 and 6
Largest Number – 9 8 7 6
Smallest Number – 6 7 8 9
Sum = 166 6 5

16665 > 9779

(d) Digits – 1, 2, 3 and 8
Largest Number 8 3 2 1
Smallest Number 1 2 3 8
Sum = 9 5 5 9
9559 < 9779

Question 2. What is the sum of the smallest and largest 5-digit palindrome? What is their difference?
Solution:

Case-I: Smallest 5 digit palindrome number (different digits) – 1 2 3 2 1
Largest 5 digit palindrome number (different digits) 98789
Sum = 12321 + 98789 = 111110
Difference = 98789 – 12321
= 86468

Case-II: Largest 5 digit palindrome (same digit) – 99999
Smallest 5 digit palindrome (same digit) – 11111
Sum – 99999 + 11111 = 111110
Difference = 99999 – 11111
= 88888

Question 3. The time now is 10:01. How many minutes until the clock shows the next palindromic time? What about the one after that?
Solution:
Time now – 10 : 01
Now next palindrome time is 11 : 11
Hence, 11:11 – 10:01 = 70 minutes.

Question 4. How many rounds does the number 5683 take to reach the Kaprekar constant?

Intext Questions

Question 1. Write all possible 3-digit palindromes using these digits 1,2,3. (Page 61)
Solution:

• 111

• 121

• 131

• 212

• 222

• 232

• 313

• 323

• 333

Question 2. Will reversing and adding numbers repeatedly, starting with a 2-digit number, always give a palindrome? Explore and find out. (Page 62)
Solution: All two-digit numbers eventually become palindromes after repeated reversal and addition. About 80% of all numbers under 10,000 resolves into a palindrome ip four or fewer steps; about 90% of those resolve in seven steps or fewer.

Example 1: Number 12
1. Initial Number: 12
2. Reverse: 21
3. Add: 12 + 21 = 33
4. Palindromp
Check: 33 is a palindrome.
Result: 33 is a palindrome.

Example 2: Number 89
1. Initial Number: 89
2. Reverse: 98
3. Add: 89 + 98 = 187
4. Palindrome Check: 187 is not a palindrome.
5. Reverse 187: 781
6. Add: 187 + 781 =968
7. Palindrome Check: 968 is not a palindrome.

Puzzle time (Page 62)

I am a 5-digit palindrome.
I am an odd number.
My ‘t’ digit is double of my ‘u’ digit.
My ‘h’ digit is double of my ‘t’ digit. Who am I?
Solution:
12421

Explore (Page 63)

Take different 4-digit numbers and try carrying out these steps. Find out what happens.
Solution:
Selected a 4-digit number 1234

• Starting number: 1234

• Descending order: 4321

• Ascending order: 1234

• Subtract: 4321 – 1234 = 3087

Repeat:

• Descending order of 3087: 8730

• Ascending order of 3087: 0378

• Subtract: 8730 – 0378 = 8352

Repeat:

• Descending order of 8352: 8532

• Ascending order of 8352: 2358

• Subtract: 8532 – 2358 = 6174

Result: 6174 (Kaprekar constant)

Question 1. Carry out these same steps with a few 3-digit numbers. What number will start repeating? (Page 63)
Solution: We will do this with help of two examples:
1. Number 123

• Starting number: 123

• Descending order: 321

• Ascending order: 123

• Subtract: 321 – 123 = 198

Repeat:

• Descending order of 198: 981

• Ascending order of 198: 189

• Subtract: 981 – 189 = 792

Repeat:

• Descending order of 792: 972

• Ascending order of 792: 279

• Subtract: 972 – 279 = 693

Repeat:

• Descending order of 693: 963

• Ascending order of 693: 369

• Subtract: 963 – 369 = 594

Repeat:

• Descending order of 594: 954

• Ascending order of 594: 459

• Subtract: 954 – 459 = 495

Repeat:

• Descending order of 495: 954

• Ascending order of 495: 459

• Subtract: 954 – 459 = 495

Result: The number 495 starts repeating.

2. Now let’s take the number 317

• Starting number: 317

• Descending order: 731

• Ascending order: 137

• Subtract: 731 – 137 = 594

Repeat:

• Descending order of 594: 954

• Ascending order of 594: 459

• Subtract: 954 – 459 = 495 ,

Repeat:

• Descending order of 495: 954

• Ascending order of 495: 459

• Subtract: 954 – 459 = 495

Result: The number 495 starts repeating. When applying Kaprekar’s routine to 3- digit numbers, the number 495 is often reached and starts repeating. This number is known as the Kaprekar constant for 3-digit numbers.

Try and find out all possible times on a 12-hour clock of each of these types. For example, 4:44, 10:10, 12:21. (Page 64)
Solution: 01:10, 02:20, 03:30, 04:40, 05:50, 10:01, 11:11, 12:21

Find some other dates of this form from the past like 20/12/2012 where the digits ‘2’, ‘0’, ‘1 ’, and ‘2 ’ repeat in that order. (Page 64)
Solution: 11/02/2011, 22/02/2022, 01/10/2010, 10/01/2010, 02/02/2020

3.6 The Magic Number of Kaprekar

Question: What number will start repeating if you carry out the same steps with 3-digit numbers?

Answer: When you perform the steps with 4-digit numbers, you always reach the magic number 6174, which is called the Kaprekar constant. For 3-digit numbers, the number that will start repeating is 495. This is known as the Kaprekar routine for 3-digit numbers.

PRACTICE QUESTIONS (NOT FROM NCERT)

Question 1: Take any four-digit number where all the digits are not the same (for example, 3524). Perform the Kaprekar process and find out how many steps it takes to reach 6174.

Answer: Step 1: Arrange the digits in descending and ascending order: 5432 - 2345 = 3087

Step 2: Repeat the process: 8730 - 0378 = 8352

Step 3: Repeat the process: 8532 - 2358 = 6174

It took 3 steps to reach 6174.

Question 2: What will happen if you start with the number 1000 in the Kaprekar process? Show the steps.

Answer: Step 1: Arrange the digits in descending and ascending order: 1000 - 0001 = 0999

Step 2: Repeat the process: 9990 - 0999 = 8991

Step 3: Repeat the process: 9981 - 1899 = 8082

Step 4: Repeat the process: 8820 - 0288 = 8532

Step 5: Repeat the process: 8532 - 2358 = 6174

It took 5 steps to reach 6174.

Question 3: If you start with the number 7624, how many steps will it take to reach the Kaprekar constant 6174?

Answer: Step 1: Arrange the digits in descending and ascending order: 7642 - 2467 = 5175

Step 2: Repeat the process: 7551 - 1557 = 5994

Step 3: Repeat the process: 9954 - 4599 = 5355

Step 4: Repeat the process: 5553 - 3555 = 1998

Step 5: Repeat the process: 9981 - 1899 = 8082

Step 6: Repeat the process: 8820 - 0288 = 8532

Step 7: Repeat the process: 8532 - 2358 = 6174

It took 7 steps to reach 6174.

Question 4: Start with the number 8754 and perform the Kaprekar process. How many iterations does it take to reach 6174?

Answer: Step 1: Arrange the digits in descending and ascending order: 8754 - 4578 = 4176

Step 2: Repeat the process: 7641 - 1467 = 6174

It took 2 steps to reach 6174.

Question 5: Why does the Kaprekar process not work if all four digits of the number are the same (e.g., 1111)?

Answer: If all digits of a number are the same, subtracting the number from its reverse results in zero. For example:

1111 - 1111 = 0000

This means the Kaprekar process cannot continue, and you will not reach 6174. Therefore, the process only works for numbers where not all digits are the same.

3.7 Clock and Calender Number

1. Try and find out all possible times on a 12-hour clock of each of these types.

Answer: This is asking you to explore the possible arrangements of digits on a 12-hour clock where the digits repeat in a specific order (such as 2, 0, 1, and 2 in the given example). You can experiment with different combinations to match this arrangement.

2. Manish has his birthday on 20/12/2012 where the digits '2', '0', '1', and '2' repeat in that order.

Answer: Manish’s birthday forms a unique pattern where the digits repeat in the specific order of '2', '0', '1', and '2'. This repetition of digits can be used to find more such dates by identifying other years with similar repeating digits.

3. Find some other dates of this form from the past.

Answer: You can find other dates where the digits repeat in a specific pattern such as:

• 10/02/2001

• 21/02/2012

• 01/02/2010 

Experimenting with other years could give more such examples.

4. His sister Meghana has her birthday on 11/02/2011 where the digits read the same from left to right and right to left.

Answer: This is a palindromic date where the digits form the same pattern when read forward and backward (11/02/2011). Other examples of such palindromic dates could include:

• 10/01/2001

• 21/02/2012

• 12/11/2112

5. Find all possible dates of this form from the past.

Answer: Possible palindromic dates could be:

• 01/02/2010

• 21/02/2012

• 02/02/2020

• 11/11/2011

6. Jeevan was looking at this year's calendar. He started wondering, "Why should we change the calendar every year? Can we not reuse a calendar?"

Answer: Calendars repeat after a specific number of years. A calendar can be reused when the days of the week align again with the dates. This typically happens in cycles of 6, 11, or 28 years, depending on whether leap years are involved.

7. Will any year's calendar repeat again after some years?

Answer: Yes, calendars do repeat after a certain number of years. For example, a common year will repeat every 6 or 11 years, while a leap year might repeat after 28 years.

8. Will all dates and days in a year match exactly with that of another year?

Answer: Not always immediately, but after a certain number of years (like 28 years for leap years), the calendar will exactly match the dates and days of a previous year.

Figure It Out 

1. Pratibha uses the digits ‘4’, ‘7’, ‘3’, and ‘2’ and makes the smallest and largest 4-digit numbers with them: 2347 and 7432. The difference between these two numbers is 7432 - 2347 = 5085. The sum of these two numbers is 9779. Choose 4-digits to make:

a. The difference between the largest and smallest numbers greater than 5085.

Answer: The largest number that can be formed using the digits would be 7432, and the smallest would be 2347. The difference is 5085.

b. The difference between the largest and smallest numbers less than 5085.

Answer: If you consider numbers smaller than 5085, the difference would be between 3472 and 2347, resulting in 1125.

c. The sum of the largest and smallest numbers greater than 9779.

Answer: In this case, there are no numbers greater than 9779 using the same set of digits.

d. The sum of the largest and smallest numbers less than 9779.

Answer: The sum of the largest (7432) and smallest (2347) is 9779.

2. What is the sum of the smallest and largest 5-digit palindrome? What is their difference?

Answer: The smallest 5-digit palindrome is 10001, and the largest is 99999. The sum of these two numbers is 110000. The difference is 89998.

3. The time now is 10:01. How many minutes until the clock shows the next palindromic time? What about the one after that?

Answer: The next palindromic time after 10:01 is 11:11. The number of minutes between 10:01 and 11:11 is 70 minutes. The palindromic time after 11:11 would be 12:21, which is 70 minutes after 11:11.

4. How many rounds does the number 5683 take to reach the Kaprekar constant?

Answer: To reach the Kaprekar constant (6174), you would perform the subtraction process by rearranging the digits of 5683 from highest to lowest and lowest to highest. This process typically takes a few rounds, but since the exact steps aren't given here, you would follow the Kaprekar process iteratively until reaching 6174.

3.8 Mental Math Figure it Out (Page No. 66 – 67)

Question 1. Write an example for each of the below scenarios whenever possible.

Could you find examples for all the cases? If not, think and discuss what could be the reason. Make other such questions and challenge your classmates.
(a) Let’s divide
90, 250 by 2

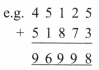
then \(\frac{90,250}{2}\) = 45,125
Hence to get sum more than 90,250 both numbers should be more than 45,125.

(b) To get a 6 digit sum by adding 5 digit and 3 digit, the 5 digit number should be more than 99,001.

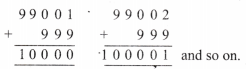
(c) Let’s take minimum 4 digit number 1000
let’s add them

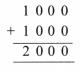
which is a 4 digit number.
Hence 6 digit sum’ from 4 digit number is impossible.

(d) Let’s take 5 digit numbers 67987 and 65783
let’s add them

which is a 6 digit number.

(e) Let’s take minimum 5 digit numbers 1000
let’s add them

which is a 5 digit number.
Hence 6 digit sum from 5 digit numbers is impossible.

(f) 5-digit -5
digit to

give a difference less than 56,503
< 56503

(g) 5-digit-3 digit = 1 008 6 (5 digit)
to give a 4 digit = + 875 (3 digit)
difference = 92 11 (4 digit)

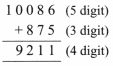
(h) 5-digit digit = 1 2 8 7 6 (5 digit)
to give a 4 digit = -7865 (4 digit)
difference = 5 0 11 (4 digit)

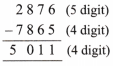
(i) 5-digit -5 digit 7 = 645 3 (5 digit)
to give a 3 digit = 76 145 (5 digit)
difference = 308 (3 digit)

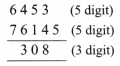
(j) 5-digit -5 digit Not possible to give 91,500

Question 2. Always, Sometimes, Never?
Below are some statements. Think, explore and find out if each of the statement is ‘Always true’, ‘Only sometimes true’ or ‘Never true’. Why do you think so? Write your reasoning; discuss this with the class.
(a) 5-digit number + 5-digit number gives a 5-digit number
(b) 4-digit number + 2-digit number gives a 4-digit number
(c) 4-digit number + 2-digit number gives a 6-digit number
(d) 5-digit number – 5-digit number gives a 5-digit number
(e) 5-digit number – 2-digit number gives a 3-digit number
Solution:

3.9 Playing with Number Patterns (NOT FROM NCERT)

1. Find the next three numbers in the sequence: 2, 4, 8, 16, __, __, __.

Answer: 32, 64, 128.
(Rule: Each number is multiplied by 2.)

2. Complete the number pattern: 5, 10, 20, 40, __, __, __. What is the rule governing this sequence?

Answer: 80, 160, 320.
(Rule: Each number is multiplied by 2.)

3.Identify the rule and fill in the blanks: 7, 14, 28, 56, __, __, __.

Answer: 112, 224, 448.
(Rule: Each number is multiplied by 2.)

4. Create your own number pattern using the rule “multiply by 2 and subtract 1,” starting with the number 3. Write the first five terms.

Answer: 3, 5, 9, 17, 33.
(Rule: Multiply by 2, then subtract 1.)

5. Given the sequence: 1, 3, 6, 10, 15, __, __, __. Find the next three numbers and explain the pattern.

Answer: 21, 28, 36.
(Rule: Add consecutive integers: +2, +3, +4, +5, +6, +7.)

6. Find the missing number in the following pattern: 50, 45, 40, __, 30, 25.

Answer: 35.
(Rule: Subtract 5 each time.)

7. Fill in the missing terms for the sequence: 9, 27, __, 243, __. What pattern do you observe?

Answer: 81, 729.
(Rule: Multiply by 3.)

3.10 An Unsolved Mystery — the Collatz Conjecture (NOT FROM NCERT)

1. What is the Collatz Conjecture?

The Collatz Conjecture is an unsolved mathematical problem that starts with any positive integer. The rules are simple: if the number is even, you divide it by 2, and if it is odd, you multiply it by 3 and add 1. The conjecture states that, no matter which number you start with, you will eventually reach the number 1.

2. What is the rule for even numbers in the Collatz Conjecture?

In the Collatz Conjecture, if the number is even, you divide it by 2.

3. What is the rule for odd numbers in the Collatz Conjecture?

For odd numbers, the rule is to multiply the number by 3 and then add 1.

4. What happens after you reach the number 1 in the Collatz sequence?

Once you reach the number 1, the sequence generally enters a cycle of repeating 4, 2, 1 indefinitely.

5. Can you explain the first few steps of the Collatz sequence for the number 6?

Starting with 6:

1. 6 is even, so divide by 2: 6 ÷ 2 = 3.

2. 3 is odd, so multiply by 3 and add 1: 3 × 3 + 1 = 10.

3. 10 is even, so divide by 2: 10 ÷ 2 = 5.

4. 5 is odd, so multiply by 3 and add 1: 5 × 3 + 1 = 16.

5. And so on, until you eventually reach 1.

3.11 Simple Estimation 

We shall do some simple estimates. It is a fun exercise, and you may find it amusing to know the various numbers around us. Remember, we are not interested in the exact numbers for the following questions. Share your methods of estimation with the class.

1. Steps you would take to walk:

• a. From the place you are sitting to the classroom door: Estimate the number of steps based on the distance and average stride length.

• b. Across the school ground from start to end: Consider the size of the school ground and divide it by your stride length to estimate the steps.

• c. From your classroom door to the school gate: Similarly, estimate the number of steps based on the known distance to the school gate.

• d. From your school to your home: Use the approximate distance from school to home and calculate using your average stride length.

2. Number of times you blink your eyes or number of breaths you take:

• a. In a minute: On average, a person blinks 15-20 times and takes about 12-20 breaths.

• b. In an hour: Multiply the minute estimate by 60. You would blink 900-1,200 times and take around 720-1,200 breaths.

• c. In a day: Over 24 hours, a person blinks around 21,600 to 28,800 times and takes 17,280-28,800 breaths.

3. Name some objects around you that are:

• a. A few thousand in number: Objects like grains of rice in a bowl or pebbles in a small area may number a few thousand.

• b. More than ten thousand in number: Things like grains of sand on a beach, or the number of blades of grass in a field could exceed ten thousand.

3.12 Games and Winning Strategies 

Figure it Out (Page No. 72 – 73)

Question 1. This grid has only one supercell (number greater than all its neighbours). If you exchange two digits of one of the numbers, there will be 4 supercells. Figure out which digits to swap.

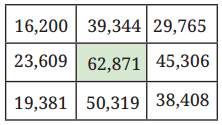
Solution: If we swap first and last digit of central number 62,871, we get desired result.

Question 2. How many rounds does your year of birth take to reacall the Kaprekar constant?
Solution: If your year of birth is 2000
Step 1: Now from digits of number 2000
Here largest number = 2000
and smallest number = 0002
Let’s subtract them = 2000 – 0002 = 1998

Step 2: Now from digits of number 1998
Here largest number = 9981
and smallest number = 1899
Let’s subtract them = 9981 – 1899 = 8082

Step 3: Now from digits of number 8082
Here largest number = 8820
and smallest number = 0288
Let’s subtract them = 8820 – 0288 = 8532

Step 4: Now from digits of number 8532
Here largest number = 8532
and smallest number = 2358

Let’s subtract them = 8532 – 2358 = 6174
which is a Kaprekar constant.
Hence it took 4 rounds to reach the Kaprekar constant from 2000.

Question 3. We are the group of 5-digit numbers between 35,000 and 75,000 such that all of our digits are odd. Who is the largest number in our group? Who is the smallest number in our group? Who among us is the closest to 50,000?
Solution:
The largest number with all odd digits (different) = 73951
The largest number with all odd digits (repetitive) = 73999
The smallest number (non repetitive) = 35,179
The smallest number (repetitive) = 57111
Closest to 50,000 (in case of non-repetition) = 49751
Closest to 50,000 (in case of repetition) = 49999

Question 4. Estimate the number of holidays you get in a year including weekends, festivals and vacation. Then try to get an exact number and see how close your estimate is.
Solution: Will be done by students.

Question 5. Estimate the number of litres a mug, a bucket and an overhead tank can hold.
Solution: Will be done by students

Question 6. Write one 5-digit number and two 3-digit numbers such that their sum is 18,670. ‘
Solution:
5 digit number = 1 8 0 0 0
3 digit number = 6 7 0
Sum = 1 8 000 + 670 = 18670

Question 7. Choose a number between 210 and 390. Create a number pattern similar to those shown in Section 3.9 that will sum up to this number.

Solution:
Sum of No. = 5 × 1 = 5
+ 10 × 3 = 30
+ 15 × 5 = 75
+ 20 × 7 = 140 = 250
which lies between 210 and 390.

Question 8. Recall the sequence of Powers of 2 from Chapter 1, Table 1. Why is the Collatz conjecture correct for all the starting numbers in this sequence?
Solution: The square of power of 2 is :
1,2,4, 8, 16, 32, 64

Let’s take the number’ 64 as per Collatz Conjecture

• 64 is even, divide by 2 = 32

• 32 is even, divide by 2 = 16

• 16 is even, divide by 2 = 8

• 8 is even, divide by 2 = 4

• 4 is even, divide by 2 = 2

• 2 is even, divide by 2 = 1

Hence Collatz conjecture is correct in all numbers in the power of 2 sequence.
As it is power of 2, and in Collatz Conjecture even number is divided by 2 in each step.

Question 9. Check if the Collatz Conjecture holds for the starting number 100.
Solution:

• 100 is even, divide by 2 =50

• 50 is even, divide by 2 = 25

• 25 is odd, so multiply by 3 and add 1 → 76

• 76 is even, divide by 2 = 38

• 38 is even, divide by 2 = 19

• 19 is odd, so multiply by 3 and add 1 → 58

• 58 is even, divide by 2 = 29

• 29 is odd, so multiply by 3 and add 1 → 88

• 88 is even, divide by 2 = 44

• 44 is even, divide by 2 = 22

• 22 is even, divide by 2 = 11

• 11 is odd, so multiply by 3 and add 1 → 34

• 34 is even, divide by 2 = 17

• 17 is odd, so multiply by 3 and add 1 → 52

• 52 is even, divide by 2 = 26

• 26 is even, divide by 2 = 13

• 13 is odd, so multiply by 3 and add 1 → 40

• 40 is even, divide by 2 = 20

• 20 is even, divide by 2 = 10

• 10 is even, divide by 2 = 5

• 5 is odd, so multiply by 3 and add 1 → 16

• 16 is even, divide by 2 = 8

• 8 is even, divide by 2 = 4

• 4 is even, divide by 2 = 2

• 2 is even, divide by 2 = 1

Yes, the Collatz conjecture holds for the starting number 100.

Intext Questions

Example: In the following, there is a number pattern on +3 being followed.

Find out the sum of the numbers in each of the below figures. Should we add them one by one or can we use a quicker way? Share and discuss in class the different methods each of you used to solve these questions. (See figures, NCERT TB, Pages 67-68)
Solution:
(a) In figure (a), number 40 is repeated 12 times and number 50 is repeated 10 times
Hence sum of all numbers = 40 × 12 + 50 × 10
= 480 + 500 = 980

(b) In figure (b), 1 dot (•) is 44 times and 5 dots (•) are 20 times
Hence sum of all dots = 1 × 44 + 5 × 20 = 44 + 100 = 144

(c) In figure (c), number 32 is 32 times and number 64 is 16 times
Hence sum of all numbers = 32 × 32 + 64 × 16 = 1024 + 1024 = 2048

(d) In figure (d), 3 dots (•) are 17 times and 4 dots (•) are 18 times
Hence sum of all dots = 17 × 3 + 18 × 4 = 51 + 72 = 123

(e) In figure (e), number 15 is 22 times, number 25 is 22 times and number 35 is 22 times
Hence sum of all numbers = 15 × 22 + 25 × 22 + 35 × 22 = 330 + 550 + 770 = 1650

(f) In figure (f), number 125 is 18 times, number 250 is 8 times and number 500 is 4 times and number 1000 is one time.
Hence sum of all numbers = 125 × 18 + 250 × 8 + 500 × 4 + 1000 = 2250 + 2000 + 2000 +1000 = 7250. 

Benefits of NCERT Solutions for Class 6 Maths Chapter 3 Number Play

• Get comfortable with the concept of even, odd, prime, and composite numbers, as they are essential for this chapter.

• Memorize the divisibility rules for numbers like 2, 3, 5, 9, and 10, as these are key in solving problems quickly.

• Learn the methods to calculate LCM and GCD using both prime factorization and the division method.

• This is an important concept for solving problems related to LCM, GCD, and prime numbers.

• Regular practice of exercises provided in the NCERT Solutions will enhance your understanding and speed.

• Use the properties of numbers, such as co-prime and multiples, to solve complex problems effectively.

Important Study Material Links for Maths Chapter 3 Class 6

Conclusion

Chapter 3 Number Play offers a comprehensive understanding of numbers, helping students build essential mathematical skills. Mastering concepts like prime numbers, LCM, and GCD is critical for future topics in mathematics. With ample practice and focus on the key concepts, students can excel in this chapter and strengthen their foundational math knowledge. The NCERT Solutions for Class 6 Maths will be an invaluable guide in helping students navigate through the complexities of number theory.

Chapter-wise NCERT Solutions Class 6  Maths

After familiarising yourself with the Class 6 Maths  Chapter 2 Question Answers, you can access comprehensive NCERT Solutions from all Class 6 Maths textbook chapters.

Related Important Links for Class 6  Maths 

Along with this, students can also download additional study materials provided by Vedantu for Maths Class 6-