NCERT Solutions for Class 6 Chapter 4 Maths FREE PDF Download
FAQs on NCERT Solutions for Class 6 Maths Chapter 4 Data Handling and Presentation
1. What is data handling in Chapter 4: Data Handling and Presentation?
Data handling involves collecting, organizing, and interpreting data to make it easier to understand. Vedantu's revision notes explain these steps with examples and diagrams.
2. How is data represented using tally marks in Chapter 4: Data Handling and Presentation?
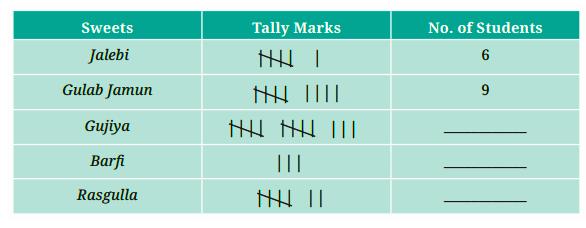
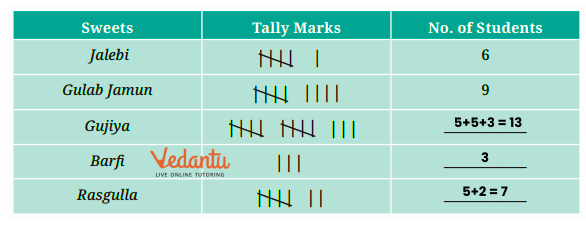
Tally marks are a simple way to represent data in groups of five. Vedantu revision notes provide examples to show how tally marks are used to count and display data.
3. What is the importance of organizing data in Chapter 4: Data Handling and Presentation?
Organizing data helps in making sense of the information, allowing for easy analysis. Vedantu notes explain how to organize raw data into tables and graphs for better understanding.
4. What are the different types of data presentation methods in Chapter 4: Data Handling and Presentation?
Data can be presented using bar graphs, pictographs, and tables. Vedantu notes cover each method with examples, making it simple for students to grasp.
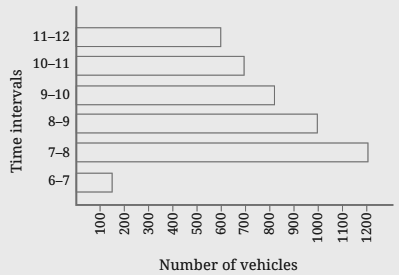
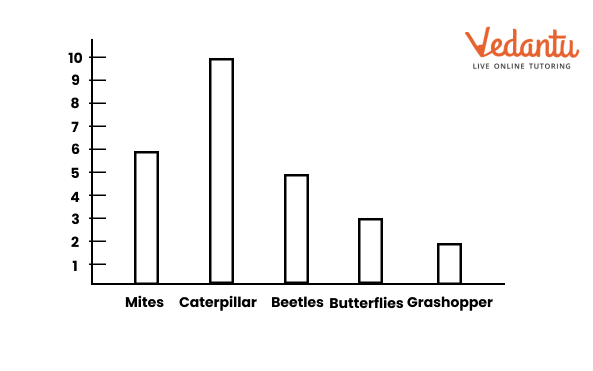
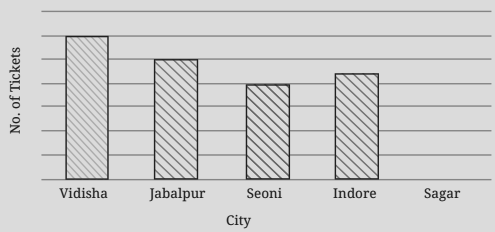
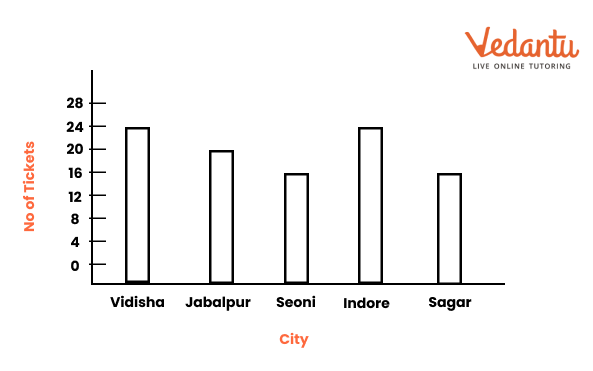
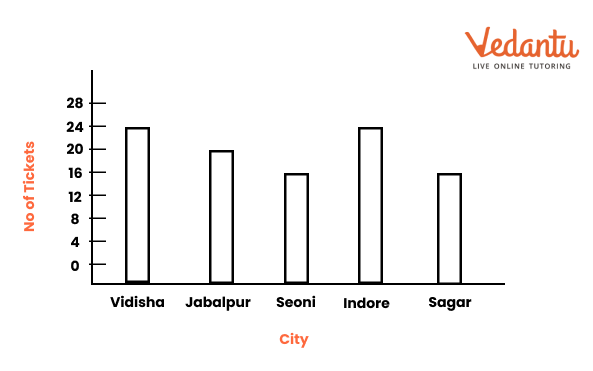
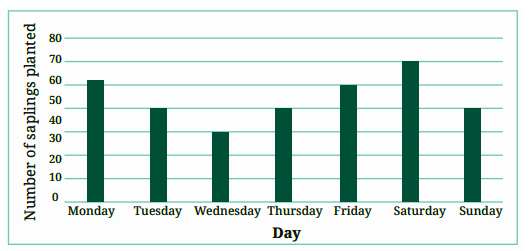
5. What is a bar graph in Chapter 4: Data Handling and Presentation?
A bar graph uses bars of different heights to represent data. Vedantu's revision notes explain how to construct and interpret bar graphs.
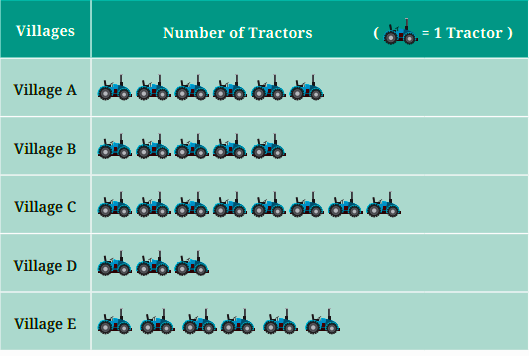
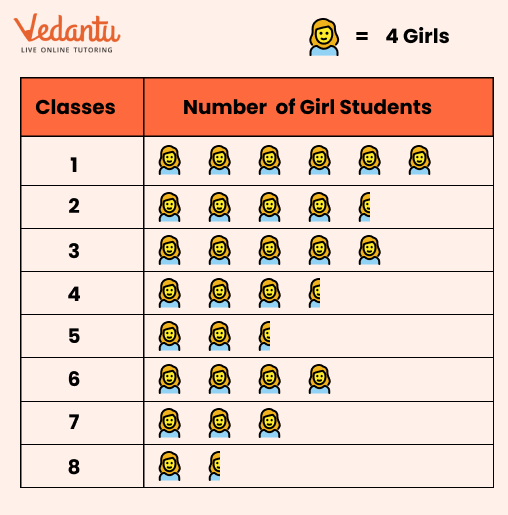
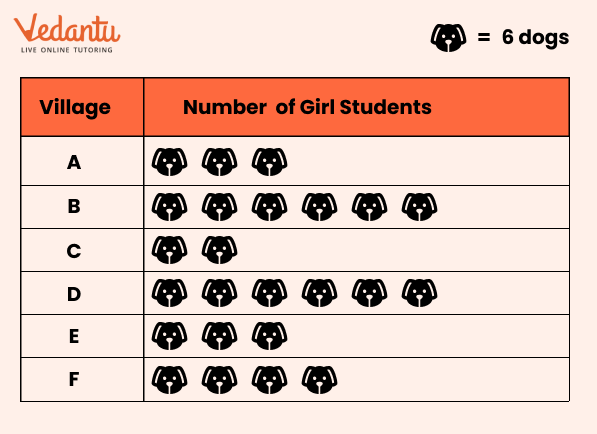
6. How do pictographs represent data in Chapter 4: Data Handling and Presentation?
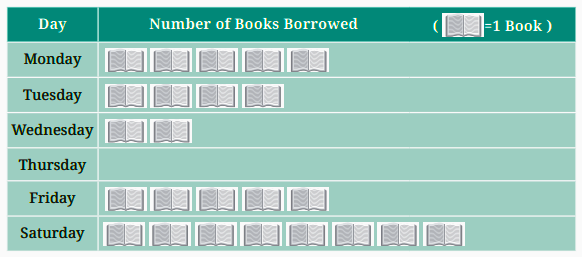
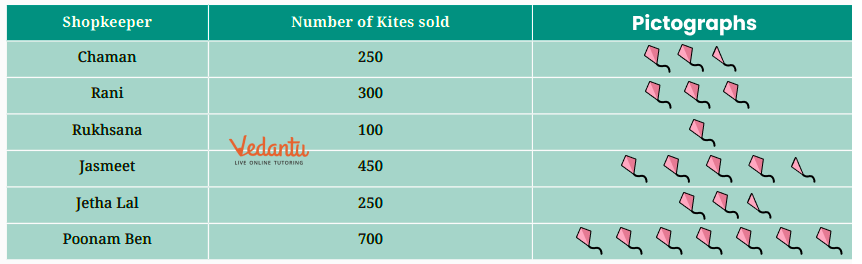
Pictographs use symbols or pictures to represent data. Vedantu revision notes show how pictographs make data easier to understand, especially for younger students.
7. What is the difference between raw data and grouped data in Chapter 4: Data Handling and Presentation?
Raw data is unorganized, whereas grouped data is categorized into classes. Vedantu explains how grouping data helps in simplifying complex information.
8. Why is it important to interpret data correctly in Chapter 4: Data Handling and Presentation?
Correct interpretation of data helps in drawing meaningful conclusions. Vedantu revision notes emphasize the importance of interpreting data accurately for analysis and decision-making.
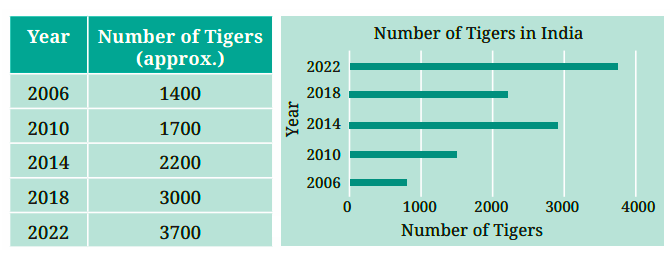
9. What is the use of a frequency table in Chapter 4: Data Handling and Presentation?
A frequency table helps to organize data by showing how often each item appears. Vedantu notes provide step-by-step instructions for creating frequency tables.
10. How can Vedantu revision notes help in understanding Chapter 4: Data Handling and Presentation?
Vedantu revision notes simplify complex concepts, provide clear examples, and offer practice exercises that help students master data handling and presentation effectively.