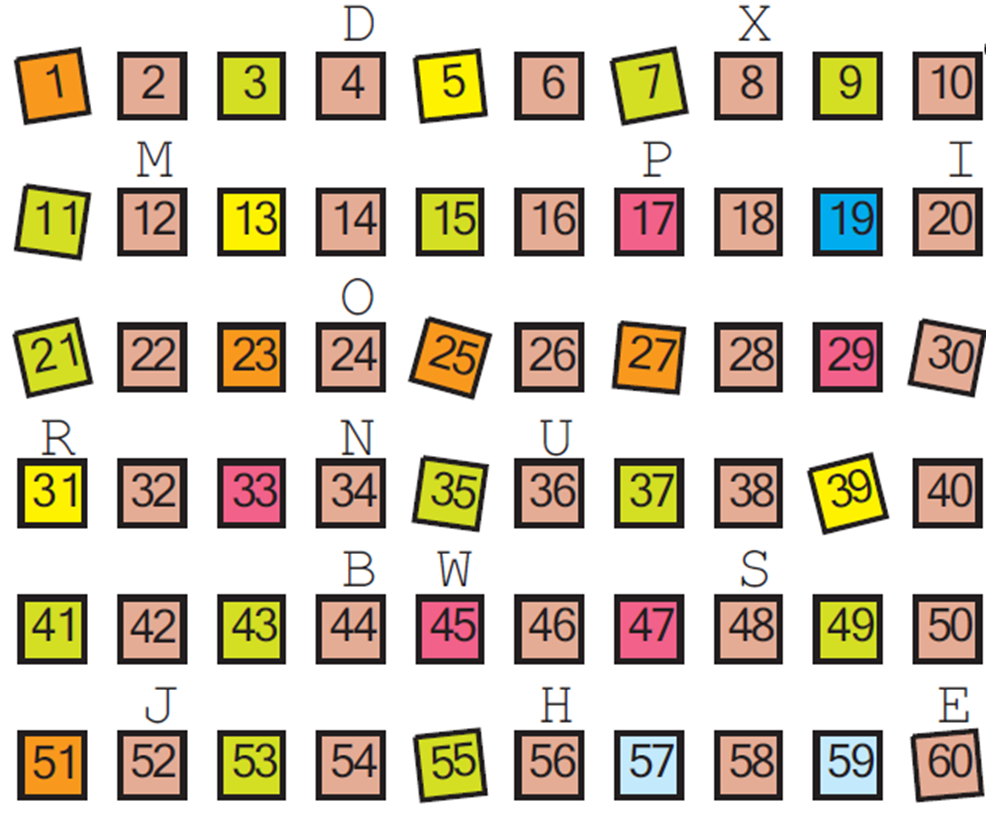
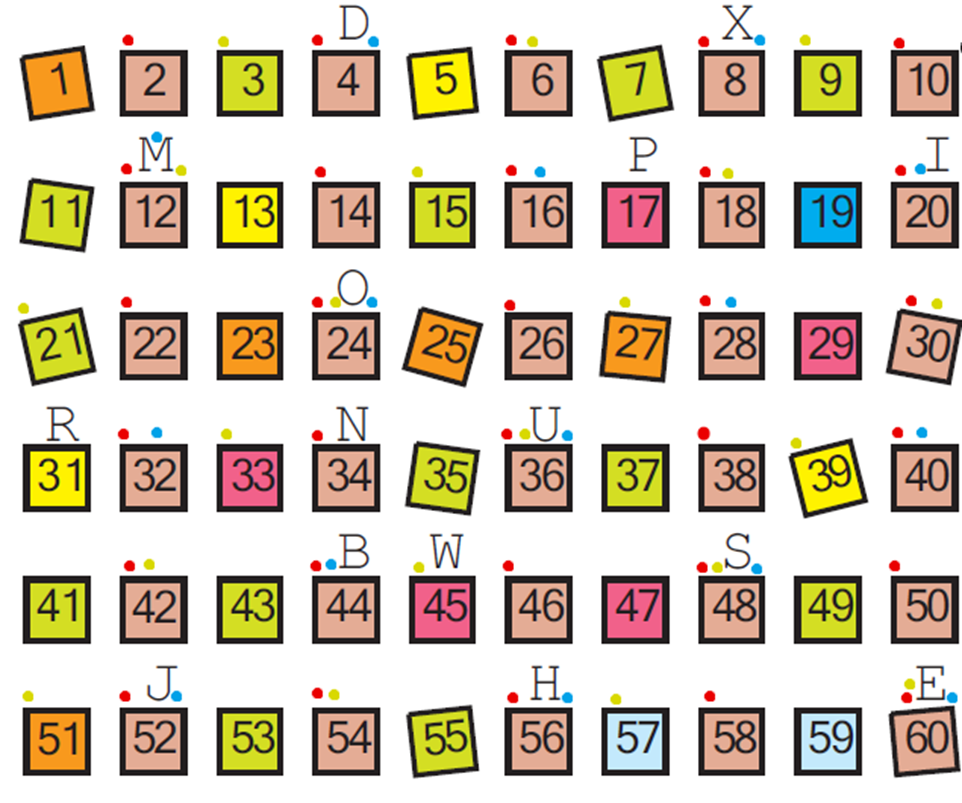
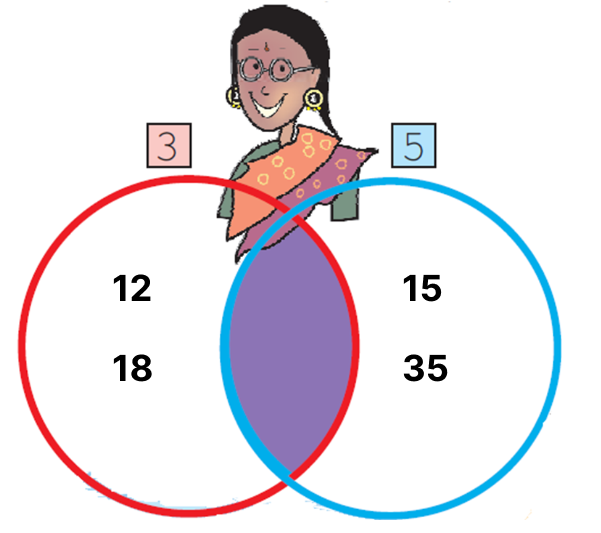
Get NCERT Solutions for Class 5 Chapter 6 Be My Multiple, I’ll Be Your Factor- FREE PDF Download
FAQs on NCERT Solutions for Class 5 Maths Chapter 6 Be My Multiple, I'Ll Be Your Factor
1. How difficult is chapter class 5, chapter 6, ‘Be My Multiple, I’ll be your Factor’?
The chapter ‘Be My Multiple, I’ll be your Factor’ is very easy and not at all hard for students to comprehend. Students should understand that this chapter is one of the foundational branches of mathematics.
However, young students may require a lot of hand-holding through the sums and should start studying with their parents or tuition teachers first before attempting the solutions by themselves.
Working out the Class 5 Maths Chapter 6 solution will help them clearly understand the chapter and increase their confidence in multiples and factors. In the long run, students will greatly benefit from the solution set for Chapter 5, ‘Be My Multiple, I’ll be your Factor’.
2. What are the Factors taught in class 5 maths chapter 6 PDF for NCERT solutions?
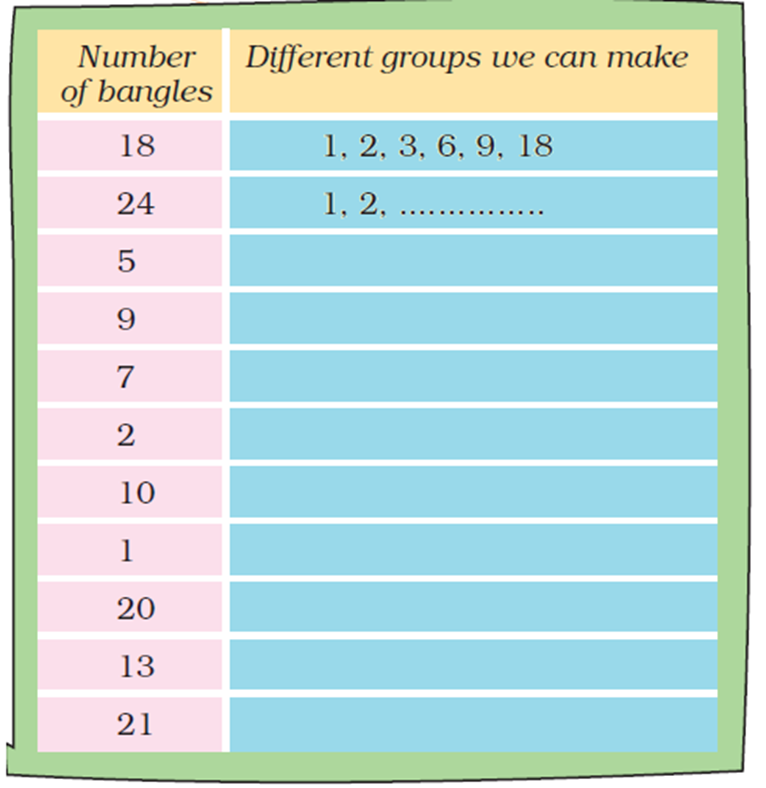
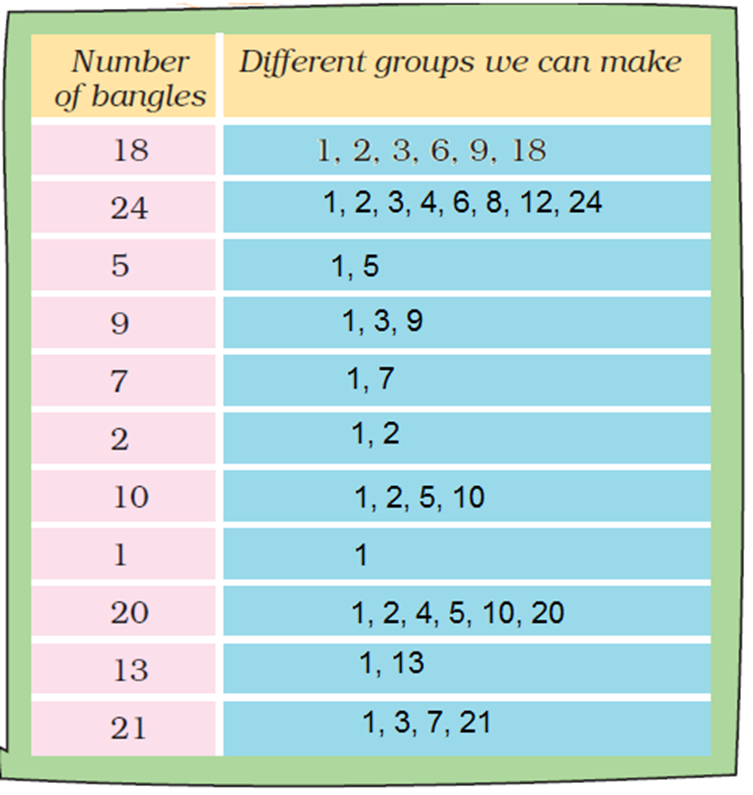
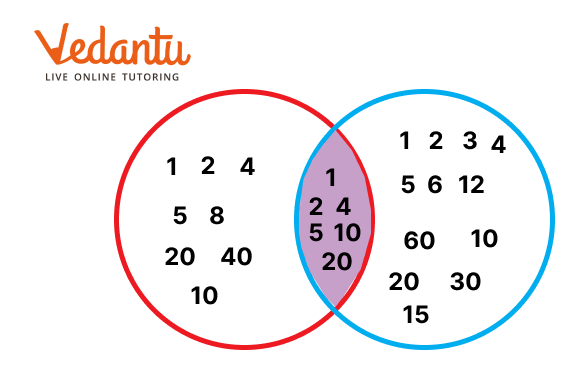
In Mathematics, factors are those numbers that divide another number precisely without any remainder. For example, the factors 24 are 1, 2, 3, 4, 6, 8, 12, and 24. These numbers divide 24 without leaving behind any remainder.
Let us look at another example. The factors of the number 36 are 1, 2, 3, 4, 6, 9, 12, 18, and 36. From the above two examples, students should get a fair idea of the factors.
3. Where can I get answers to class 5 math chapter 6, ‘Be My Multiple, I’ll be your Factor’?
For students who are looking to find answers for the ‘Be My Multiple, I’ll be your Factor’ chapter of the NCERT Class 5 textbook, several sites offer in-depth answers online. But the very best solution set is provided by Vedantu. Their answers are in-depth and to the point. Also, they use a step-by-step approach, which is very useful for students.
4. What topics does class 5 maths chapter 6 PDF with answers deal with?
Chapter 6 of Maths of Class 5 deals with the following topics:
Jumping or skipping two or three numbers to form a series with it.
Figuring out the numbers divisible by two, three and four.
Writing down the multiples of the given numbers in a sequence.
Finding out the smallest common multiple.
5. What is the highest and lowest common factors according to class 5th maths chapter 6?
- Highest Common Factor: While considering the common factor of two given multiples, the highest number that comes in the mixture of all the other common factors is termed as the highest common factor
- Lowest Common Factor: The lowest common factor refers to the smallest number easily divisible by any two given integers.
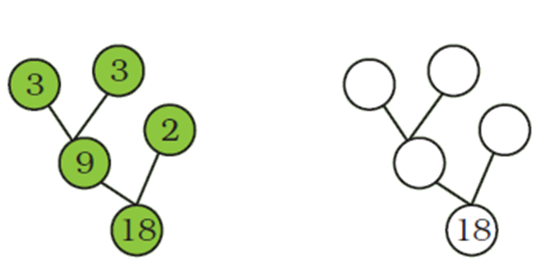
6. Calculate the LCM and HCF of 264 and 624 using the prime factorization method for class 5, chapter 6.
The first step involves finding the given numbers' factors.
Therefore, the factor of 264 is 2*2*3*11.
And the factor of 624 is 2*2*2*2*3*11.
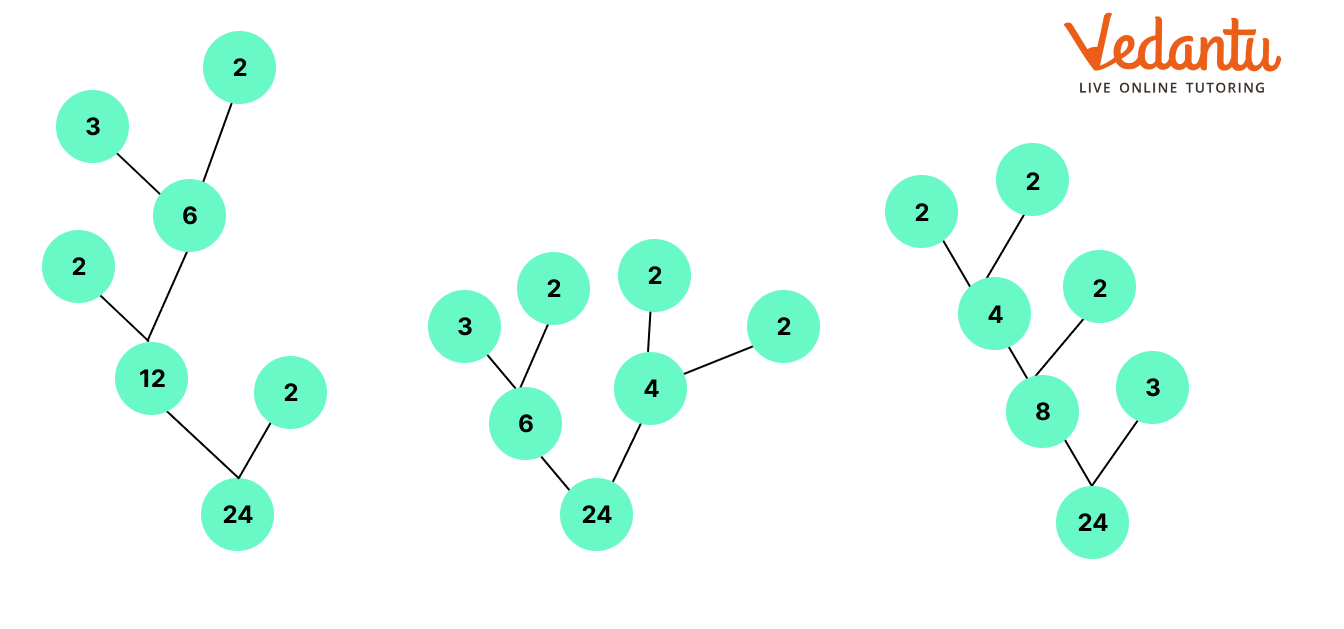
The next step involves finding the factors that are common in both the numbers 2*2*2*3
We attain the highest common factor by multiplying these common factors= 24
To find the LCM, we have to multiply the remaining factors 2*2*2*3*2*11*13= 6864.
7. How to prepare for class 5 maths chapter 6 question answers?
In order to get a full understanding of Chapter 6 of Maths of Class 5, the student needs to practice all the exercises regularly. The sums that this chapter entails are not just important for Class 5 examinations, but also go on to play a very important role in higher education. Therefore, it goes without saying that it is important for every student to be well versed in this chapter. And to achieve this regular practice is required. They need to figure out the areas that they find to be their weak spots and give extra time to these in order to be thorough with the concepts.
8. Are NCERT solutions helpful for class 5 chapter 6 ‘Be My Multiple, I’ll be your Factor’?
Yes, the NCERT Solutions are extremely helpful for Chapter 6 of Maths of Class 5. These exercises are designed by experts in order to prepare the students for the exams. All the questions have detailed and step by step answers that make the job of the student easier. The answers are served to the students and all they have to do is practice and get skilled with the concepts. They can avail these solutions easily and are free of cost from the website.