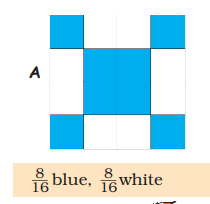
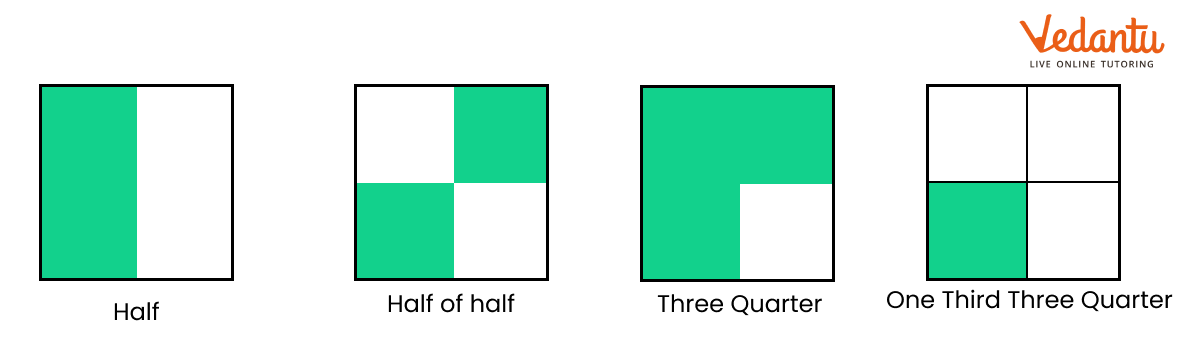NCERT Solutions Class 5 Maths Chapter 4 Parts and Wholes - FREE PDF Download
FAQs on NCERT Solutions for Class 5 Maths Chapter 4 Parts and Wholes
1. What is the chapter on Parts and Wholes?
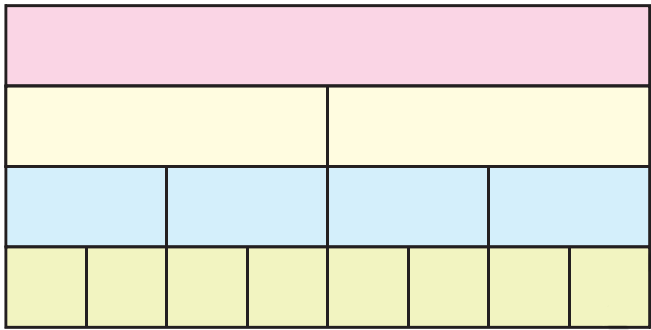
NCERT Class 5 Maths Parts and Wholes is a chapter that involves the basic foundational level of fractions. Students of class five are trained to understand exactly what parts mean and what is the relationship between parts and the whole. Parts are some portion of a total. Instead of building up concepts of fractions, the more straightforward idea of parts and whole is introduced.
Students can make use of Class 5 Maths Chapter 4 solutions to strengthen their concepts and help them in their quest to get flying colours. These solutions will significantly simplify the study time and help them get confidence in mathematics.
2. Where can I find answers to the chapter Parts and Wholes?
Students looking for Class 5 CBSE Maths Chapter 4 solutions can find it online after a simple search. Several online portals have the solution set, but the very best solution provider is Vedantu. Vedantu’s answer is the best among its competitors because of its easy to understand form. Also, it is found in an easy to download format.
NCERT Class 5 Maths solution Chapter 4 comes in a simple, clear and concise manner. Students will find it of great help to them when they sit down to do their studies. Parts and Wholes is an easy chapter and students should not have much difficulty to do the questions.
3. Is Parts and Whole chapter a part of fractions?
Yes, the chapter on parts and wholes is a part of fractions. When in the younger classes, students do not understand the meaning of fractions, so they are taught fractions as parts and whole. It is the foundational level of the fractions chapter which they will come across in higher classes. If students do not understand the chapter, they should make use of Vedantu’s solution set.
Maths NCERT Solutions Class 5 Chapter 4 will help students on their mathematical journey as they go to higher grades. It will help them get their concepts clear so they can climb the rest of the ladder with the ease of an ace student.
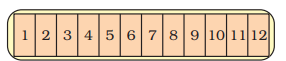
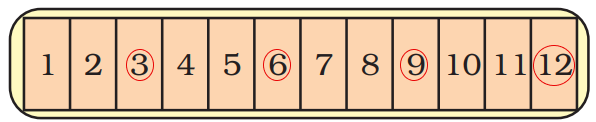
4. Manju had one chocolate. She gave one-fourth of it to Raji, one-third to Sugatha and one-sixth to Sheela. She ate the remaining part. How many pieces of chocolate did each get?
The total number of bites that the chocolate has is 12
Out of these 12 parts, she gave ¼ to Raji
Therefore, = ¼ * 12= 3 bites
Thus. Raji has a total of 3 bites
Manju gave Sheela ⅙ of the chocolate
Therefore, ⅙*12= 2 bites
Thus, Sheela has a total of 2 bites
Sugatha was given 1/3rd of the chocolate
Therefore, ⅓ * 12= 4bites
Thus, Sugata has a total of 4 bites
For more insight into the chapter, the students can refer to the NCERT solutions on Vedantu.
5. Most people sleep about 8 hours a day. Then what part of a day is it?
We know that, a day has a span of 24 hours.
The question tells us that on average every individual sleeps for 8 hours out of the 24 hours.
Therefore = 8/24
=⅓ part of the day
5. Most people sleep about 8 hours a day. Then what part of a day is it?
We know that, a day has a span of 24 hours.
The question tells us that on average every individual sleeps for 8 hours out of the 24 hours.
Therefore = 8/24
=⅓ part of the day
7. Is Chapter 4 of Class 5 Maths hard?
It is to be always remembered that practice is the source to achieve anything. With practice, anything that appears hard can be changed into someone’s strength. Thus, in order to attain a thorough understanding of Chapter 4 Of Class 5 Maths, it goes without saying that a regular practice of the topics is required. The students need to figure out the areas that they find difficult and work extra hard on them. Additionally, the reference to the NCERT Solutions will also prove to be of great guidance in shaping and preparing the students for exams.
8. Are the NCERT solutions helpful for Chapter 4 of Class 5 Maths?
Yes, the Vedantu Solutions for Chapter 4 Of Class 5 Maths are no doubt the best guide books for the students. These solutions are designed by experts to help the student solve any question that might be asked in the question paper. The exercises have detailed answers with them that makes the learning process much easier for the student. The answers are written in the simplest language and cover all the important points and areas so that the student is not left behind on anything. They are free of cost and also available on Vedantu Mobile app.
9. What are parts and wholes in Class 5th Maths Chapter 4?
Imagine a delicious apple! The whole apple is the complete fruit. If you cut it into slices, each slice is a part, and all the slices together make up the whole apple again. Parts and wholes are simply ways to understand how things can be divided into smaller pieces and how those pieces come together to form the complete thing.
10. What are fractions?
Fractions are a way to represent parts of a whole. Think of your apple slices again. Each slice is a fraction of the whole apple. Fractions use numbers to show how many parts you have out of a total number of parts. For example, if you have 2 apple slices from a whole apple cut into 4 slices, then you have 2/4 of the apple.
11. Why is understanding parts and wholes important?
Understanding parts and wholes is the foundation for learning fractions. Fractions are used everywhere in math, from solving problems about sharing things to measuring shapes and distances. By mastering parts and wholes, you'll be well-prepared to conquer fractions in the future!
12. How are parts and wholes used in everyday life?
Parts and wholes Class 5 are all around us! You use them when you share a pizza with friends (each slice is a part), color sections of a picture (each section is a part of the whole picture), or cut a cake into equal pieces for a party (each piece is a part of the whole cake).