Complete Resource of NCERT Maths Chapter 6 Lines and Angles Class 9 - Free PDF Download
NCERT Solutions for Class 9 Maths Chapter 6 Lines and Angles
FAQs on NCERT Solutions for Class 9 Maths Chapter 6 Lines and Angles
1. How can NCERT Solutions for Class 9 Chapter 6 Maths Help in the Exam Preparation?
NCERT Solutions for Class 9 Chapter 6 make comprehensive study material and cover the concepts of the entire chapter to help students build a strong foundation. These solutions follow the latest CBSE curriculum and make students familiar with the types of questions being asked from the lines and angles chapter. Students can get in-depth knowledge about the topic, brief summary, solved questions, and more. Apart from reasoning and logical skills, these chapter solutions offer comprehensive learning to students. The difficult topics like types of angles, interior, and exterior angles are explained in a better way. Students can clear their tough concepts and can analyze their weak areas with the guidance of NCERT solutions class 6.
2. How are the Vertically Opposite Angles Equal when Two Lines Intersect each Other?
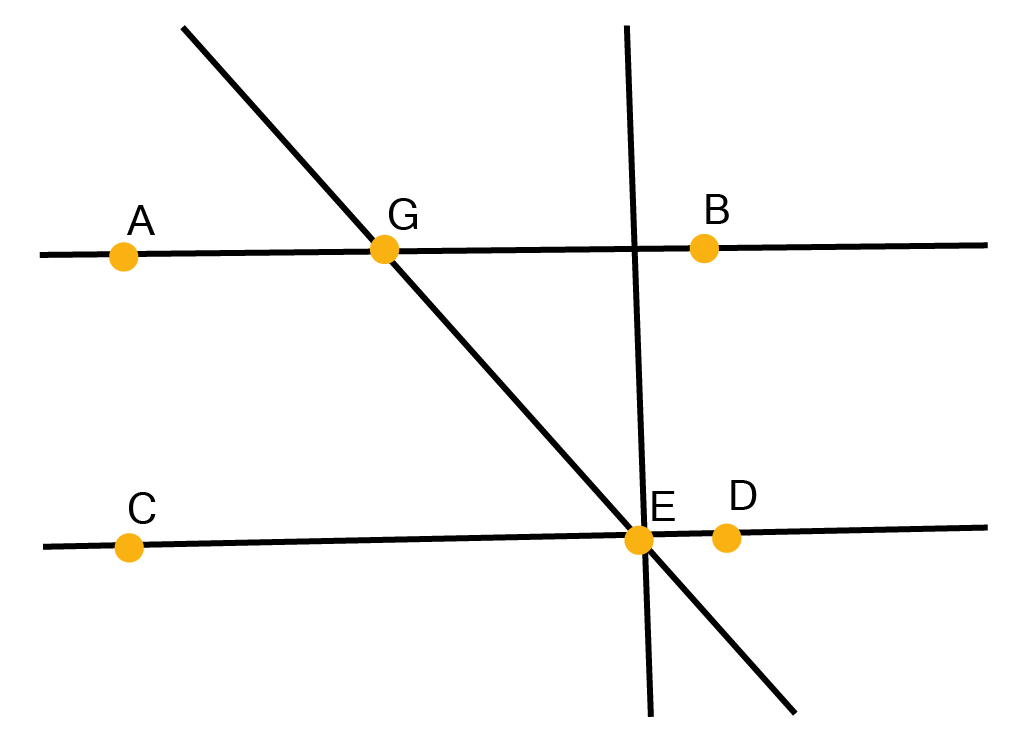
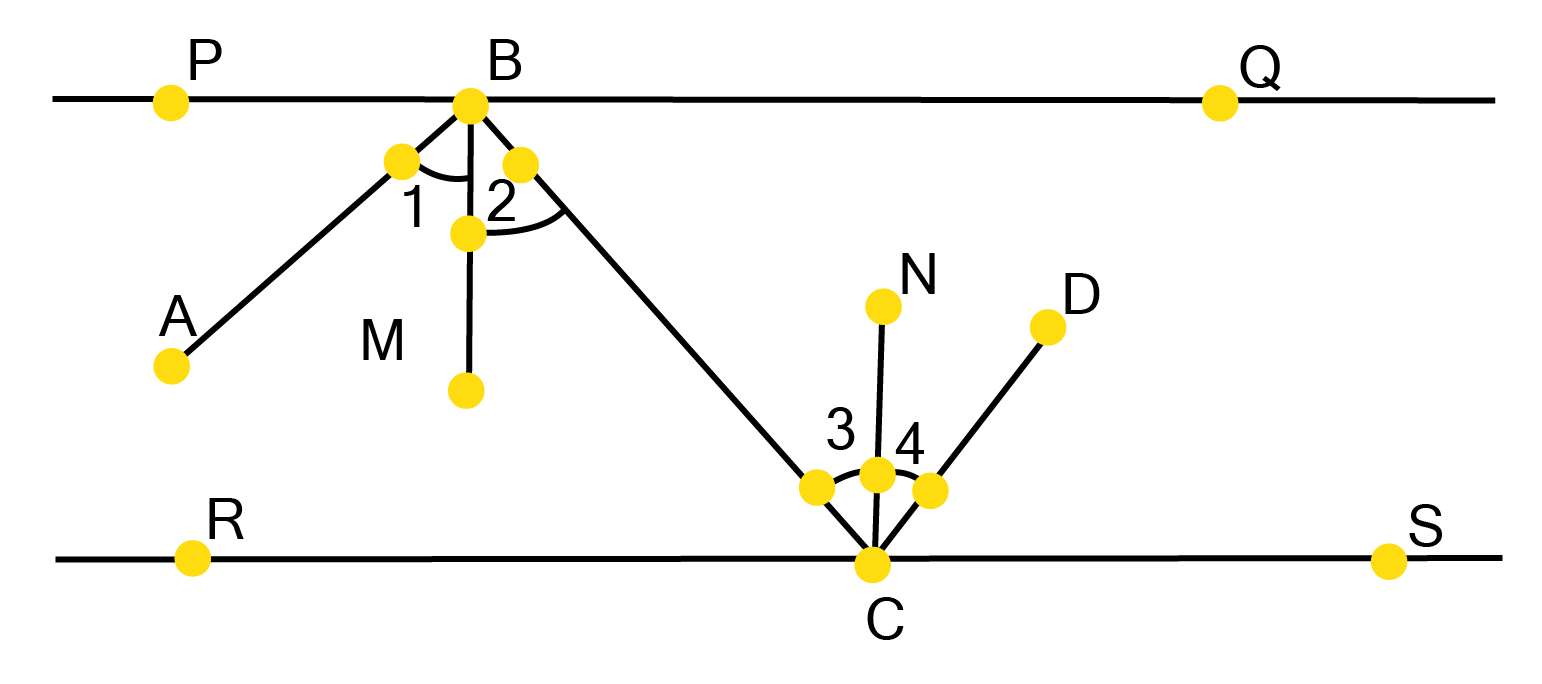
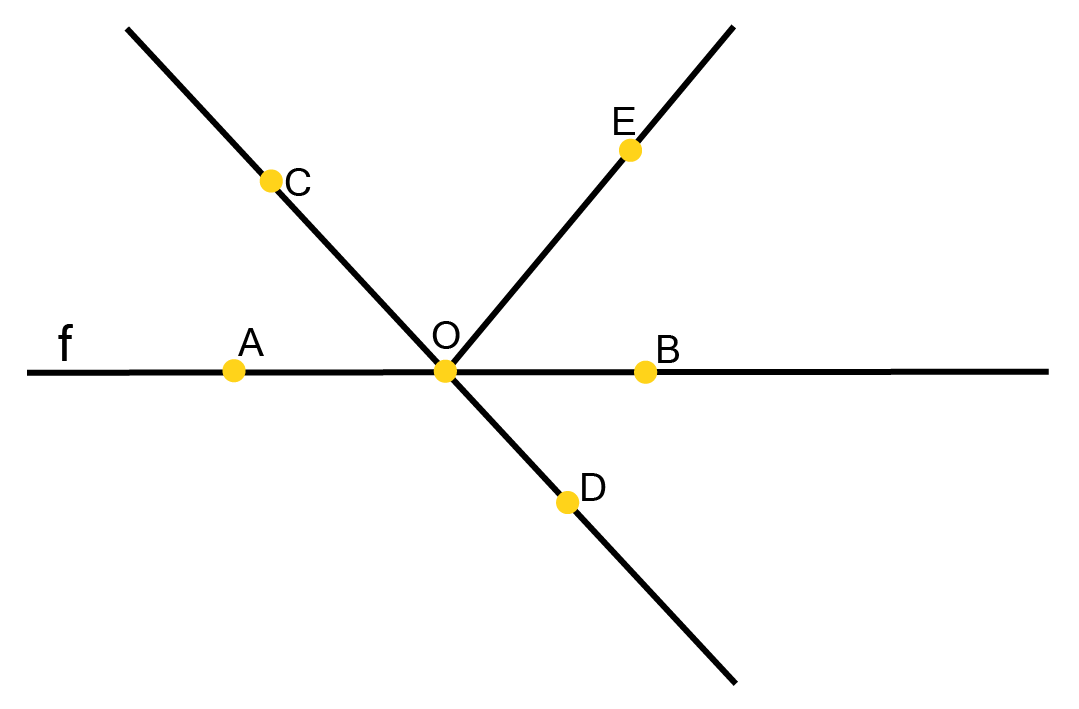
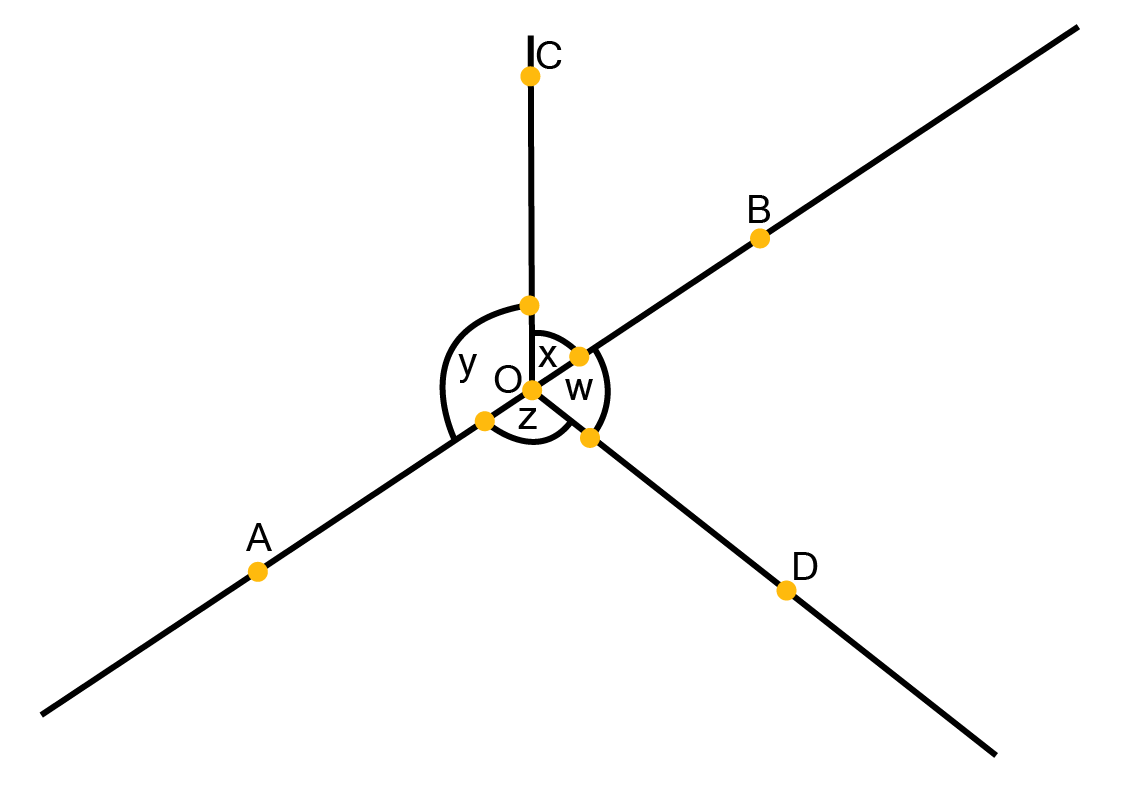
Let AB and CD be two lines intersecting at a single point, say, P. APC and BPD, and APD and BOC form two pairs of vertically opposite angles. Additionally, ray AP is perpendicular on line CD. It implies the sum of APC and APD is equal to 180o. Now, the sum of angles APD and BPD is equal to 180o. By using these two equations, it’s clear that the sum of angles APC and APD is equal to the sum of APD and BPD angles. It implies angle APC is equal to BPD. Similarly, it can be shown that angle APD is equal to angle BPC.
3. Are all the exercises important in Chapter 6 of Class 9 Maths?
All exercises of NCERT Class 9 Maths Chapter 6 are designed in a well-structured hierarchy of difficulty to test the student’s ability in problem-solving. They also help him or her bridge the knowledge gap. Practising all the exercises is of utmost importance to score well and to clearly understand all the necessary concepts. Hence it is advisable to not skip any exercise. All the solutions are also easily available on Vedantu website and the app.
4. What is the best Solution book for Chapter 6 of NCERT Class 9 Maths?
Vedantu is the place where you will get the solutions easily and for free. To download the solutions for Chapter 6 of NCERT Class 9 Maths, follow the steps mentioned below:
Visit the page of Chapter 6 of NCERT Solutions for Class 9 Maths.
The website for solutions for Chapter 6 of Class 9 Maths will open.
The solutions for the selected chapter will be displayed on the screen. One can also download these solutions by clicking on Download PDF to save it offline.
For more information regarding this, you can visit the Vedantu site or app and avail of their services to crack your entrances and perform well in all of your exams.
5. What do you mean by collinear and non-collinear points?
The collection of three or more points that occur on the same straight line is known as collinear points. On multiple planes, collinear points may occur, but not on different lines. Collinearity is the property of points being collinear. As a result, any three or more points are only collinear if they are in the same straight line. If any of the points are not on the same line, they are considered non-collinear as a group. The size of the triangle formed by the three non-collinear points will always be greater than zero.
6. What are the types of lines in Chapter 6 of Class 9 Maths?
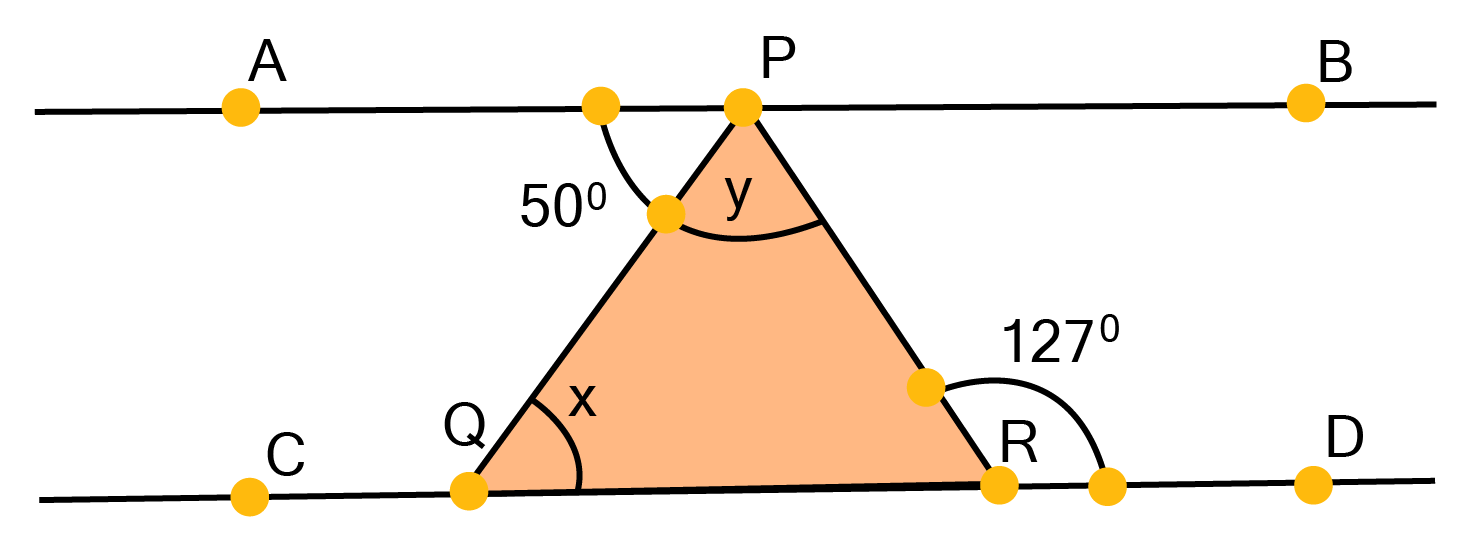
In Geometry, there are two types of lines: straight and curved. Straight lines are divided into two categories: horizontal and vertical. Parallel, intersecting, and perpendicular lines are examples of other types of lines. Knowledge of all these types and the formulae related to them are very crucial and hence you must practice them judiciously.
7. What do you mean by a vertex?
A vertex is a point in geometry where two or more curves, lines, or edges intersect. As a result of this definition, vertices can be described as the junction where two separate lines tend to meet and make an angle, as well as the corners of polygons and polyhedra. In mathematics, it is an angular corner formed by the intersection of two or more lines or edges between two faces. A vertex may be found in 2D shapes such as pentagons and squares, as well as 3D shapes such as pyramids and cuboids.
8. What are lines and angles in brief?
Lines and angles are fundamental elements of geometry, forming infinitely long, straight paths. Understanding different line types and angles is crucial. Vedantu's solutions provide clear explanations, step-by-step breakdowns, and practice problems to help students understand these concepts, laying a strong foundation for future geometrical adventures.
9. What are the types of Line and Angles Class 9?
In the world of geometry of lines and angles class 9 solutions, angles come in various shapes and sizes, just like slices of pie! Acute angles are sharp turns less than 90 degrees, like a thin slice. Right angles are perfect 90-degree corners, like a closed book. Obtuse angles make wider turns between 90 and 180 degrees, like a large slice. Finally, straight angles span 180 degrees, forming a straight line when two lines extend opposite each other.
10. What's covered in the exercises of Maths Chapter 6 Class 9?
Lines & Angles Identification: These exercises will teaches you to recognize lines (rays, segments) and various angles (acute, right, obtuse, straight).
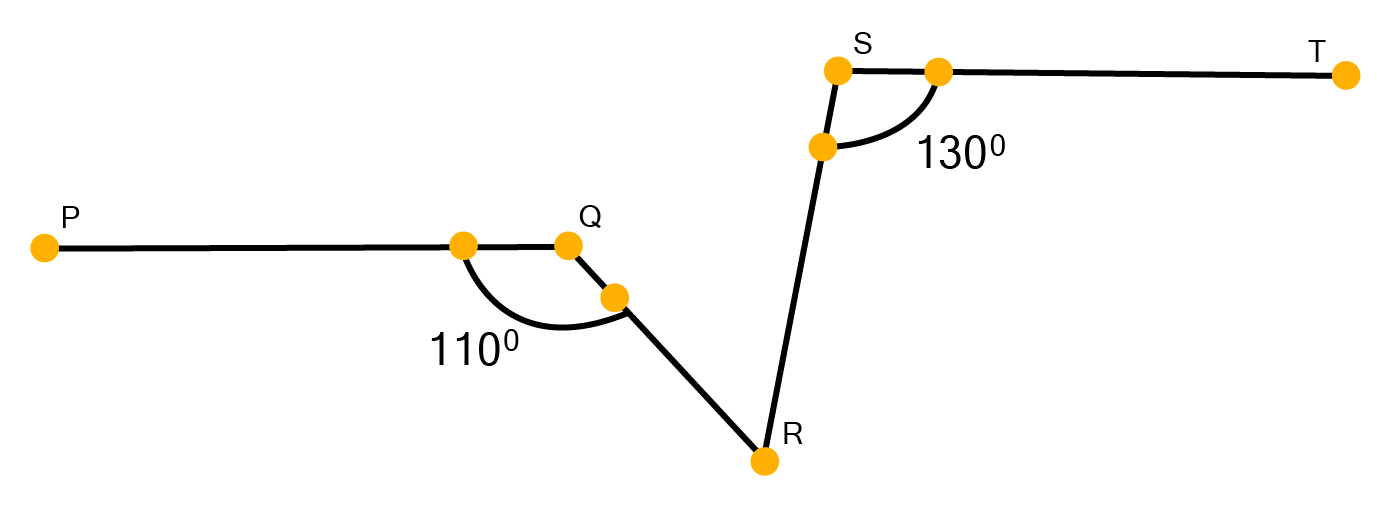
Measurement and Calculations: This exercise will make you become skilled with a protractor and be able to solve issues with missing angle measures, addition, and subtraction of angles.
Real-World Application: Prepare yourself to solve word problems that evaluate your skills with lines and angles.























 Watch Video
Watch Video







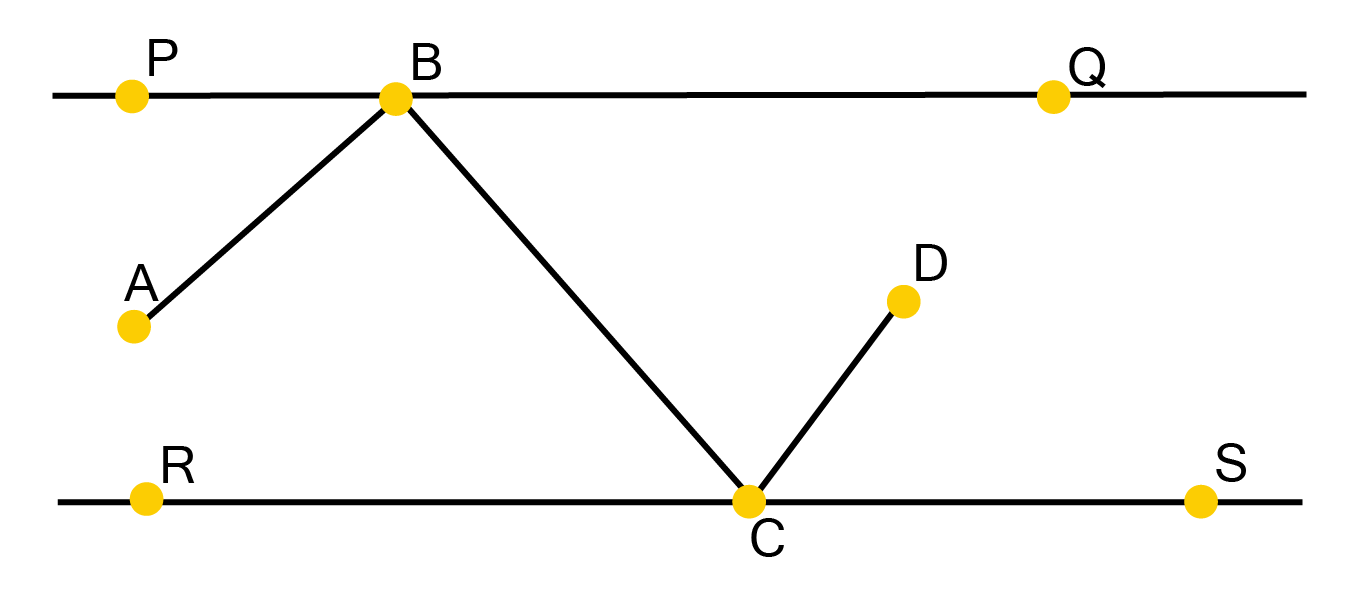
![AB\[\parallel \] CD and CD \[\parallel \] EF](https://www.vedantu.com/seo/content-images/afaa573d-dbd7-4e5d-82bb-36a18cd01a0f.png)