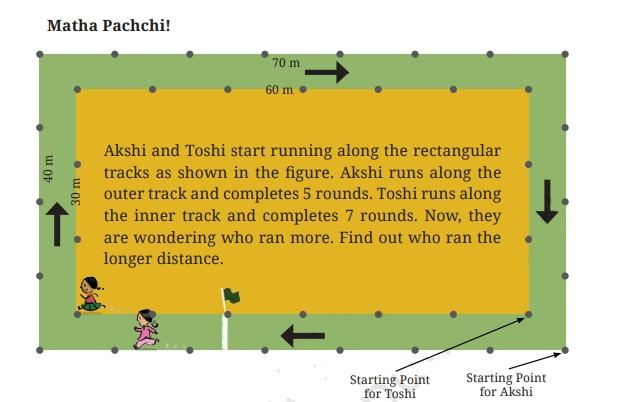
NCERT Solutions for Class 6 Maths Chapter 6 Perimeter And Area Ex 6.1

Exercise 6.1

A- Figure it Out 

1. Find the missing terms: 

a. Perimeter of a rectangle = 14 cm; breadth = 2 cm; length = ?. 

b. Perimeter of a square = 20 cm; side of a length = ?. 

c. Perimeter of a rectangle = 12 m; length = 3 m; breadth = ?. 

Ans:

1. Perimeter of a rectangle = 14 cm; breadth = 2 cm; length =?

Formula: Perimeter = 2 × (length + breadth)

14 = 2 × (length + 2)

14 = 2 × (length + 2)

14 ÷ 2 = length + 2

7 = length + 2

Length = 7 - 2 = 5 cm

2. Perimeter of a square = 20 cm; side length = ?

Formula: Perimeter = 4 × side

20 = 4 × side

Side = 20 ÷ 4 = 5 cm

3. Perimeter of a rectangle = 12 m; length = 3 m; breadth = ?

Formula: Perimeter = 2 × (length + breadth)

12 = 2 × (3 + breadth)

12 ÷ 2 = 3 + breadth

6 = 3 + breadth

Breadth = 6 - 3 = 3 m

Final answers: a. Length = 5 cm b. Side = 5 cm c. Breadth = 3 m

2. A rectangle having sidelengths 5 cm and 3 cm is made using a piece of wire. If the wire is straightened and then bent to form a square, what will be the length of a side of the square? 

Ans: To solve this problem:

1. Find the perimeter of the rectangle:

The formula for the perimeter of a rectangle is:

$\text{Perimeter} = 2 \times (\text{length} + \text{breadth})$

For the given rectangle with side lengths of 5 cm and 3 cm:

$\text{Perimeter} = 2 \times (5 + 3) = 2 \times 8 = 16 \, \text{cm}$

So, the total length of the wire is 16 cm.

2. When the wire is bent to form a square, the perimeter of the square will also be 16 cm.

3. Find the side length of the square:

The formula for the perimeter of a square is:

$\text{Perimeter} = 4 \times \text{side}$

Given that the perimeter is 16 cm:

$16 = 4 \times \text{side}$

Solving for side:

$\text{side} = 16 \div 4 = 4 \, \text{cm}$

Therefore, the length of each side of the square will be 4 cm.

3. Find the length of the third side of a triangle having a perimeter of 55 cm and having two sides of length 20 cm and 14 cm, respectively. 

Ans:

The perimeter of the triangle is the sum of all three sides.

Given two sides are 20 cm and 14 cm, let the third side be \( x \).

$\text{Perimeter} = 20 + 14 + x$

$55 = 34 + x$

$x = 55 - 34 = 21 \, \text{cm}$

The length of the third side is 21 cm.

4. What would be the cost of fencing a rectangular park whose length is 150 m and breadth is 120 m, if the fence costs `40 per metre? 

Ans:

First, find the perimeter of the park.

The perimeter of a rectangle is given by:

$\text{Perimeter} = 2 \times (\text{length} + \text{breadth}) = 2 \times (150 + 120) = 2 \times 270 = 540 \, \text{m}$

Now, calculate the cost of fencing:

$\text{Cost} = \text{Perimeter} \times \text{Cost per metre} = 540 \times 40 = 21,600 \, \text{₹}$

The cost of fencing the park is ₹21,600.

5. A piece of string is 36 cm long. What will be the length of each side, if it is used to form: 

a. A square, 

b. A triangle with all sides of equal length, and 

c. A hexagon (a six sided closed figure) with sides of equal length? 

Ans:

a. A square:

The perimeter of the square is 36 cm, so each side of the square is:

$\text{Side length} = \frac{\text{Perimeter}}{4} = \frac{36}{4} = 9 \, \text{cm}$

b. A triangle with all sides of equal length:

The perimeter of the triangle is 36 cm, so each side of the equilateral triangle is:

$\text{Side length} = \frac{\text{Perimeter}}{3} = \frac{36}{3} = 12 \, \text{cm}$

c. A hexagon (six-sided closed figure) with sides of equal length:

The perimeter of the hexagon is 36 cm, so each side of the regular hexagon is:

$\text{Side length} = \frac{\text{Perimeter}}{6} = \frac{36}{6} = 6 \, \text{cm}$

Final Answer:

- a. Side length of the square = 9 cm

- b. Side length of the triangle = 12 cm

- c. Side length of the hexagon = 6 cm

6. A farmer has a rectangular field having length 230 m and breadth 160 m. He wants to fence it with 3 rounds of rope as shown. What is the total length of rope needed?

Ans: To find the total length of rope needed to fence the rectangular field with 3 rounds of rope, follow these steps:

1. Find the perimeter of the rectangular field:

The formula for the perimeter of a rectangle is:

$\text{Perimeter} = 2 \times (\text{length} + \text{breadth})$

Given the length is 230 m and the breadth is 160 m:

$\text{Perimeter} = 2 \times (230 + 160) = 2 \times 390 = 780 \, \text{m}$

2. Calculate the total length of rope for 3 rounds:

Since the farmer wants 3 rounds of rope, the total length of rope required is:

$\text{Total rope length} = 3 \times \text{Perimeter} = 3 \times 780 = 2340 \, \text{m}$

So, the total length of rope needed is 2340 m.

B- Figure it Out

Each track is a rectangle. Akshi’s track has length 70 m and breadth 40 m. Running one complete round on this track would cover 220 m, i.e., 2 × (70 + 40) m = 220 m. This is the distance covered by Akshi in one round.

1. Find out the total distance Akshi has covered in 5 rounds. 

2. Find out the total distance Toshi has covered in 7 rounds. Who ran a longer distance? 

3. Think and mark the positions as directed— 

a. Mark ‘A’ at the point where Akshi will be after she ran 250 m. 

b. Mark ‘B’ at the point where Akshi will be after she ran 500 m. 

c. Now, Akshi ran 1000 m. How many full rounds has she finished running around her track? Mark her position as ‘C’. 

d. Mark ‘X’ at the point where Toshi will be after she ran 250 m. 

e. Mark ‘Y’ at the point where Toshi will be after she ran 500 m. 

f. Now, Toshi ran 1000 m. How many full rounds has she finished running around her track? Mark her position as ‘Z’

Ans:

1. Total distance Akshi covered in 5 rounds:

Each round is 220 metres (m).

Total distance = 5 × 220 m = 1100 m.

2. Total distance Toshi covered in 7 rounds:

Each round is 200 metres (m).

Total distance = 7 × 200 m = 1400 m.

3. Who ran the longer distance?

Toshi ran the longer distance, covering 1400 m, which is more than Akshi’s 1100 m.

For marking points A, B, C, X, and Y as instructed:

- A: Mark the point where Akshi is after 250 m.

- B: Mark the point where Akshi is after 500 m.

- C: Mark the point where Akshi completes 1000 m.

- X: Mark the point where Toshi is after 250 m.

- Y: Mark the point where Toshi is after 500 m.

Benefits of NCERT Solutions for Class 6 Maths Chapter 1 Exercise 6.1 Perimeter and Area

• These solutions offer step-by-step explanations of perimeter calculations, helping students grasp the fundamental concepts clearly.

• With detailed solutions, students can practise solving perimeter problems accurately and efficiently.

• Learning to calculate the perimeter lays a strong foundation for more advanced geometry topics in higher classes.

• The solutions connect mathematical problems to real-life situations, such as measuring the boundaries of gardens or rooms, making learning more relatable.

• Practising these NCERT solutions enhances problem-solving skills, helping students feel more confident in exams.

• Students can easily download the free PDF to review and practice anywhere, making learning more convenient.

• The solutions reinforce key formulas for perimeter, ensuring that students remember and apply them correctly in different scenarios.

• The step-by-step method in the solutions helps students save time by following a structured problem-solving process.

Class 6 Maths Chapter 1: Exercises Breakdown

Important Study Material Links for Class 6 Maths Chapter 6 - Perimeter and Area

Conclusion

In the NCERT Solutions for Class 6 Maths Chapter 6 Perimeter and Area Exercise 6.1 FREE PDF, we've explored a variety of real-life applications of perimeter and basic geometry. Whether it's calculating the length of rope for fencing a field, finding missing sides of triangles and rectangles, or determining the cost of fencing a park, understanding these fundamental concepts is crucial. These practical problems help build a strong foundation in geometry, enhancing problem-solving skills and preparing students for more advanced mathematical concepts. By mastering these perimeter-related problems, students will be better equipped to handle everyday mathematical challenges.

Chapter-Specific NCERT Solutions for Class 6 Maths

The chapter-wise NCERT Solutions for Class 6 Maths are given below. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.

Related Important Links for Maths Class 6

Along with this, students can also download additional study materials provided by Vedantu for Maths Class 6.