Pair of Linear Equations in Two Variables Class 10 Solutions - PDF
NCERT Solutions for Class 10 Maths Chapter 3 Pair Of Linear Equations In Two Variables 2025-26



FAQs on NCERT Solutions for Class 10 Maths Chapter 3 Pair Of Linear Equations In Two Variables 2025-26
1. How are the NCERT Solutions for Class 10 Maths Chapter 3 structured for each exercise?
The NCERT Solutions for Class 10 Maths Chapter 3, Pair of Linear Equations in Two Variables, are structured to provide a detailed, step-by-step explanation for every problem in each exercise of the NCERT textbook. The solutions follow the CBSE 2025-26 guidelines, ensuring that students learn the correct methodology to present their answers in board exams. Each step is clearly elaborated to help students understand the logic behind the solution.
2. What are the official algebraic methods for solving problems in Chapter 3 as per the latest CBSE syllabus?
As per the CBSE 2025-26 syllabus for Class 10 Maths Chapter 3, there are two primary algebraic methods for solving a pair of linear equations in two variables. The NCERT solutions provide detailed walkthroughs for both:
- Substitution Method: In this method, the value of one variable is expressed in terms of the other from one equation and substituted into the second equation.
- Elimination Method: This method involves manipulating the equations to eliminate one of the variables by making their coefficients equal and then adding or subtracting the equations.
3. How do I decide whether to use the substitution method or the elimination method for a given problem?
Choosing the right method can make solving the problem much faster. Here’s a simple guideline:
- Use the substitution method when the coefficient of at least one variable (x or y) in either equation is 1 or -1. This makes it easy to isolate that variable without creating fractions.
- Use the elimination method when the coefficients of one of the variables in both equations are the same, opposite, or can be made the same by multiplying by a small integer. This is often more efficient for equations with larger coefficients.
4. Why is it important to check the ratio of coefficients (a₁/a₂, b₁/b₂, c₁/c₂) before attempting to solve a pair of linear equations?
Checking the ratio of coefficients is a crucial first step because it reveals the nature of the solution without any calculation. It tells you whether the system of equations is consistent or inconsistent.
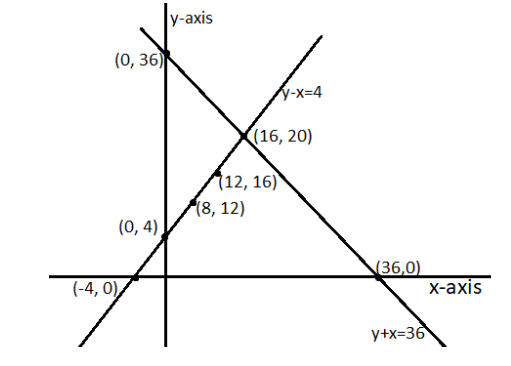
- If a₁/a₂ ≠ b₁/b₂, the lines are intersecting, and there is a unique solution.
- If a₁/a₂ = b₁/b₂ = c₁/c₂, the lines are coincident, and there are infinitely many solutions.
- If a₁/a₂ = b₁/b₂ ≠ c₁/c₂, the lines are parallel, and there is no solution.
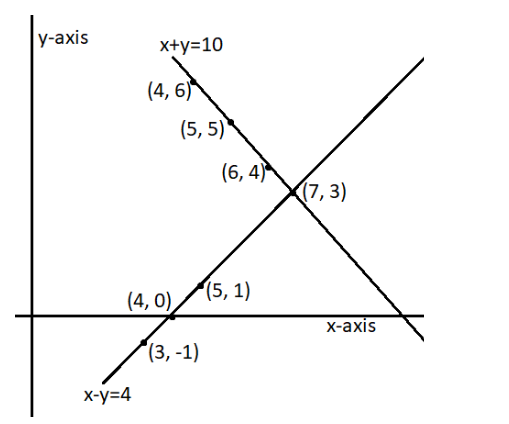
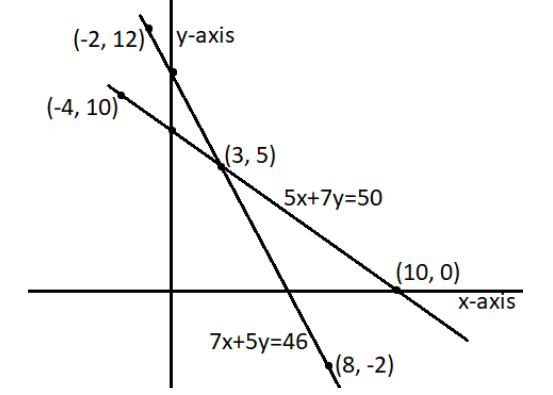
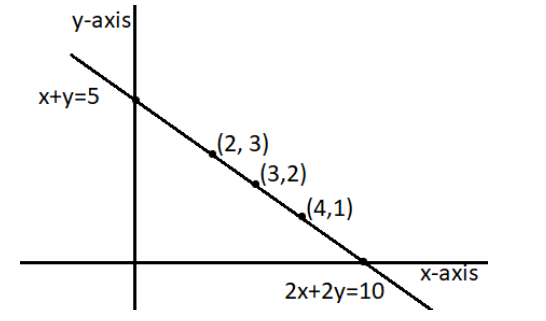
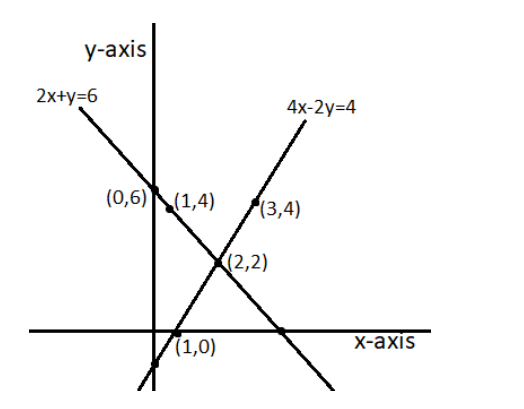
5. How does solving a pair of linear equations graphically relate to the algebraic solution?
The graphical method provides a visual understanding of what an algebraic solution represents. When you solve a pair of linear equations algebraically, you are finding the coordinate (x, y) that satisfies both equations. Graphically, this is the point where the two lines representing the equations intersect. If the lines are parallel, they never intersect, indicating no solution. If the lines are coincident (the same line), they intersect at every point, indicating infinitely many solutions.
6. What is a common mistake to avoid when framing equations from word problems in Chapter 3?
A common and critical mistake is incorrectly translating the conditions of the word problem into mathematical equations. Students often mix up the variables or misinterpret the relationships (e.g., 'five years ago' or 'ten years hence'). To avoid this, always start by clearly defining your variables (e.g., let the present age of the father be 'x' and the son be 'y'). Then, form two separate equations based on the two distinct conditions given in the problem. Double-check that each equation accurately represents the statement it was derived from.
7. Is it necessary to master both the substitution and elimination methods for the Class 10 board exam?
Yes, it is essential to master both the substitution and elimination methods. The CBSE board exam may sometimes specify which method to use for a particular question. Being proficient in both techniques allows you to not only comply with such instructions but also to choose the most efficient method for problems where the choice is yours, thereby saving valuable time during the exam.
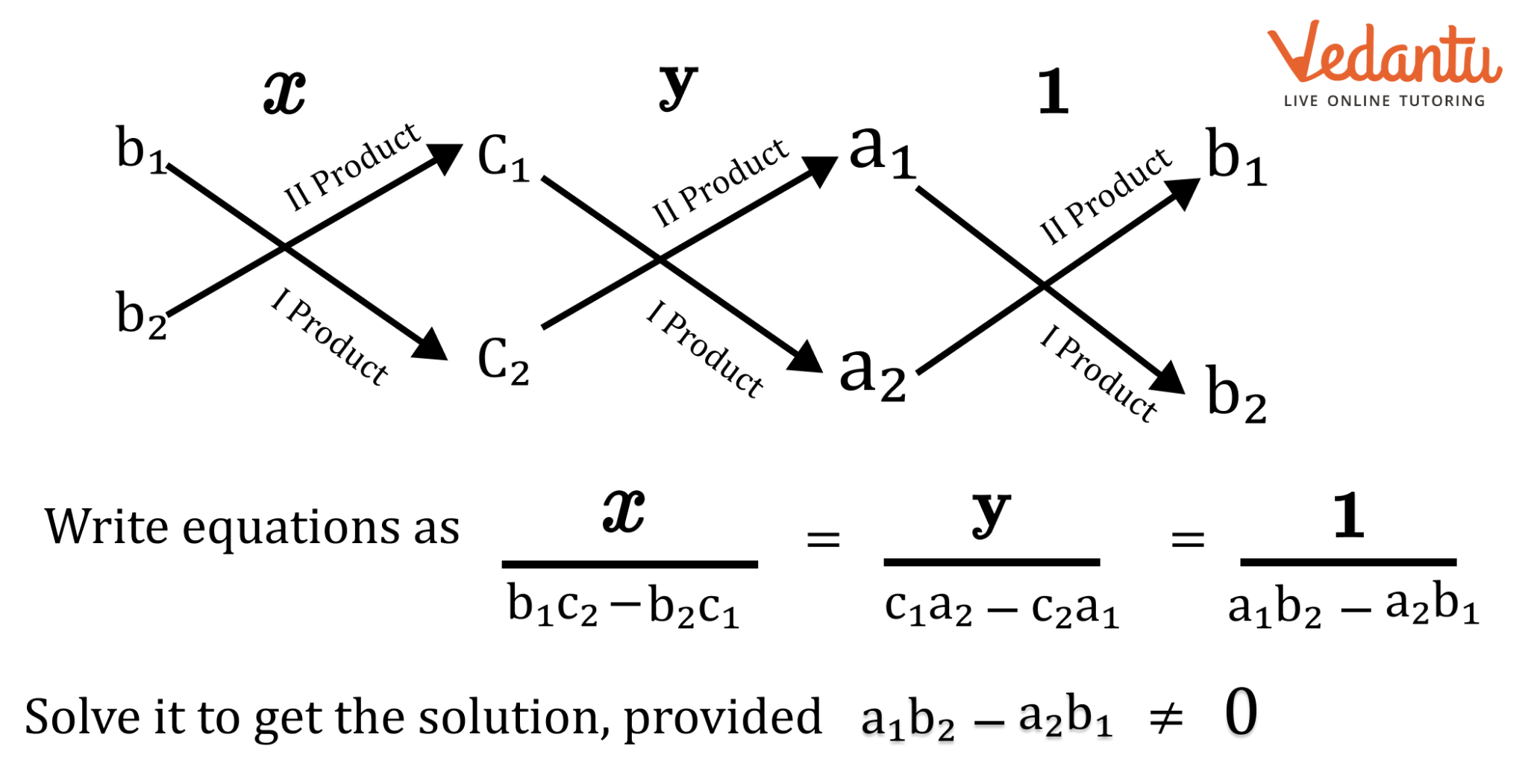
8. How do I correctly apply the elimination method to solve NCERT problems?
To correctly apply the elimination method, follow these steps:
- Step 1: Multiply one or both equations by suitable non-zero constants to make the coefficients of one variable (either x or y) numerically equal.
- Step 2: Add or subtract one equation from the other to eliminate the variable with equal coefficients. This results in a single-variable equation.
- Step 3: Solve the resulting equation to find the value of the remaining variable.
- Step 4: Substitute this value back into either of the original equations to find the value of the variable you first eliminated.




















 Watch Video
Watch Video