NCERT Solutions for Class 12 Maths Chapter 8 - Application of Integrals
NCERT Solutions for CBSE Class 12 Maths Chapter 8 Application of Integrals are available in Vedantu. These NCERT Solutions are created as per the latest syllabus of NCERT Maths for Class 12. This PDF covers solutions for all questions that are covered in the CBSE Class 12 Maths textbook Chapter 8. All the solutions are explained in a step by step manner. Students can refer to these solutions for learning the important questions and prepare for their board exams. The NCERT Solutions for CBSE Class 12 Maths Chapter 8 are available in a PDF format and can be downloaded for free.
Class: | |
Subject: | |
Chapter Name: | Chapter 8 - Application Of Integrals |
Content-Type: | Text, Videos, Images and PDF Format |
Academic Year: | 2024-25 |
Medium: | English and Hindi |
Available Materials: |
|
Other Materials |
|
Applications of Integrals Chapter at a Glance - Class 12 NCERT Solutions
The area of the region bounded by the curve $y-f(x), x$-axis and the lines $x-a$ and $x-b(b>a)$ is given by the formula:
Area $=\int_a^b y x x-\int_a^b f(x) d x$
The area of the region bounded by the curve $x-\phi(y), y$-axis and the limes $y-c, y-d$ is given by the formula: Area $-\int_c^d x \dot{a} y=\int_c^d \phi(y) d y$
The of the region enclosed between two curves $y-f(x) y-g(x)$ and the lines $x-a, x-b$ is given by the formula, Area $-\int_a^b[f(x)-g(x)]$ ki, where, $f(x) \geq g(x)$ in $[a, b]$
If $f(x) \geq g(x)$ in $[a, c]$ and $f(x) \leq g(x)$ in $[c, b], a<c<b$, then, Area $=\int_a^c[f(x)-g(x)] d x+\int_c^b[g(x)-f(x)] d x$
The area of the region bounded by the curve $y-f(x), x$-axis and the lines $x-a$ and $x-b(b>a)$ is given by the formula:
Area $=\int_a^b y x x-\int_a^b f(x) d x$
The area of the region bounded by the curve $x-\phi(y), y$-axis and the limes $y-c, y-d$ is given by the formula: Area $-\int_c^d x \dot{a} y=\int_c^d \phi(y) d y$
The of the region enclosed between two curves $y-f(x) y-g(x)$ and the lines $x-a, x-b$ is given by the formula, Area $-\int_a^b[f(x)-g(x)]$ ki, where, $f(x) \geq g(x)$ in $[a, b]$
If $f(x) \geq g(x)$ in $[a, c]$ and $f(x) \leq g(x)$ in $[c, b], a<c<b$, then, Area $=\int_a^c[f(x)-g(x)] d x+\int_c^b[g(x)-f(x)] d x$



Exercises under NCERT Class 12 Maths Chapter 8 Application of Integrals
Chapter 8 of the Class 12 Maths NCERT textbook deals with the applications of integrals. This chapter covers various topics, such as finding the area of a region, finding the area between two curves, finding the volume of a solid of revolution, and finding the length of a curve.
Exercise 8.1: This exercise teaches students how to find the area of a region bounded by curves using integration.
Exercise 8.2: This exercise covers the concept of finding the area between two curves and helps students understand how to use integration to solve problems related to it.
Miscellaneous Exercise: This exercise contains a collection of problems that require students to apply the concepts they have learned in the previous exercises to solve real-world problems.
In summary, this chapter helps students understand how to apply the concepts of integrals to solve problems related to finding the area of a region, finding the area between two curves, finding the volume of a solid of revolution, and finding the length of a curve. The exercises in this chapter are designed to help students develop a deep understanding of these concepts and their applications.
Topics Covered in the NCERT Solutions for Class 12 Maths Chapter 8 Application of Integrals
Introduction |
Area under Simple Curves |
Area under Two Curves |
Exercise Problems |
What are the Applications of Integrals?
Integrals are mainly used for calculating the area between simple curves or between lines, parabolas, and ellipses. Integration can also be used for calculating the average value of a function, for example, the average rainfall per day can be recorded using Integrals.
Let us consider two apples placed in a round box with a lid, both the apples will occupy some area and some space will remain unoccupied. By using the Integral formula, we can calculate these spaces inside the box. Likewise, we can find the space inside a circle, a line, an ellipse, and a parabola.
Integrals can also be applied to find the area between two curves. Here, the area can be calculated by splitting the regions under the curve into a number of smaller areas and they can be added at last for finding the total area under the curve.
Access NCERT Solutions for Class 12 Maths Chapter 8 – Application of Integral
Exercise 8.1
1. Find the area of the region bounded by the curve \[{{\text{y}}^{\text{2}}}\text{=x}\] and the lines \[\text{x=1,x=4}\] and the \[\text{x-axis}\].
Ans:
(Image Will be Updated Soon)
Area of \[\text{ABCD=}\int_{\text{1}}^{\text{4}}{\text{y}}\text{dx}\]
Substitute \[y=\sqrt{x}\]
\[\text{=}\int_{\text{1}}^{\text{4}}{\sqrt{\text{x}}\text{dx}}\]
Integrating using the power rule
\[\text{=}\left[ \frac{\frac{{{\text{x}}^{\frac{\text{3}}{\text{2}}}}}{\text{3}}}{\text{2}} \right]_{\text{1}}^{\text{4}}\]
Substituting the limits,
\[\text{=}\frac{\text{2}}{\text{3}}\left[ {{\text{(4)}}^{\frac{\text{3}}{\text{2}}}}\text{-(1}{{\text{)}}^{\frac{\text{3}}{\text{2}}}} \right]\]
\[\text{=}\frac{\text{2}}{\text{3}}\text{ }\!\![\!\!\text{ 8-1 }\!\!]\!\!\text{ }\]
\[\text{=}\frac{\text{14}}{\text{3}}\text{ units}\]
Therefore, required area is \[\frac{\text{14}}{\text{3}}\text{ units}\]
2. Find the area of the region bounded by \[{{\text{y}}^{\text{2}}}\text{=9x}\], \[\text{x=2,x=4}\] and the \[\text{x-axis}\] in the first quadrant.
Ans:
(Image Will be Updated Soon)
Area of \[\text{ABCD=}\int_{2}^{\text{4}}{\text{y}}\text{dx}\]
substitute $y=3\sqrt{x}$
\[\text{=}\int_{2}^{\text{4}}{3\sqrt{\text{x}}\text{dx}}\]
Integrating using the power rule
\[\text{=3}\left[ \frac{\frac{{{\text{x}}^{\frac{\text{3}}{\text{2}}}}}{\text{3}}}{\text{2}} \right]_{\text{2}}^{\text{4}}\]
Substituting the limits,
\[\text{=2}\left[ {{\text{(4)}}^{\frac{\text{3}}{\text{2}}}}\text{-(2}{{\text{)}}^{\frac{\text{3}}{\text{2}}}} \right]\]
\[\text{=}\frac{\text{2}}{\text{3}}\text{ }\!\![\!\!\text{ 8-2}\sqrt{\text{2}}\text{ }\!\!]\!\!\text{ }\]
\[\text{=}\left( 16-4\sqrt{2} \right)\text{ units}\]
Therefore, the required area is \[\left( 16-4\sqrt{2} \right)\text{ units}\]
3. Find the area of the region bounded by \[{{\text{x}}^{\text{2}}}\text{=4y,y=2,y=4}\] and the \[\text{y- axis}\] in the first quadrant.
Ans:
(Image Will be Updated Soon)
Area of \[\text{ABCD=}\int_{2}^{\text{4}}{x}\text{dx}\]
Substitute $x=2\sqrt{y}$
\[\text{=}\int_{2}^{\text{4}}{2\sqrt{y}\text{dx}}\]
Integrating using the power rule
\[\text{=2}\left[ \frac{\frac{{{y}^{\frac{\text{3}}{\text{2}}}}}{\text{3}}}{\text{2}} \right]_{\text{2}}^{\text{4}}\]
Substituting the limits,
\[\text{=}\frac{4}{3}\left[ {{\text{(4)}}^{\frac{\text{3}}{\text{2}}}}\text{-(2}{{\text{)}}^{\frac{\text{3}}{\text{2}}}} \right]\]
\[\text{=}\frac{4}{\text{3}}\text{ }\!\![\!\!\text{ 8-2}\sqrt{\text{2}}\text{ }\!\!]\!\!\text{ }\]
\[\text{=}\left( \frac{32-8\sqrt{2}}{3} \right)\text{ units}\]
Therefore, the required area is \[\left( \frac{32-8\sqrt{2}}{3} \right)\text{ units}\]
4. Find the area of the region bounded by the ellipse \[\frac{{{\text{x}}^{\text{2}}}}{\text{16}}\text{+}\frac{{{\text{y}}^{\text{2}}}}{\text{9}}\text{=1}\].
Ans:
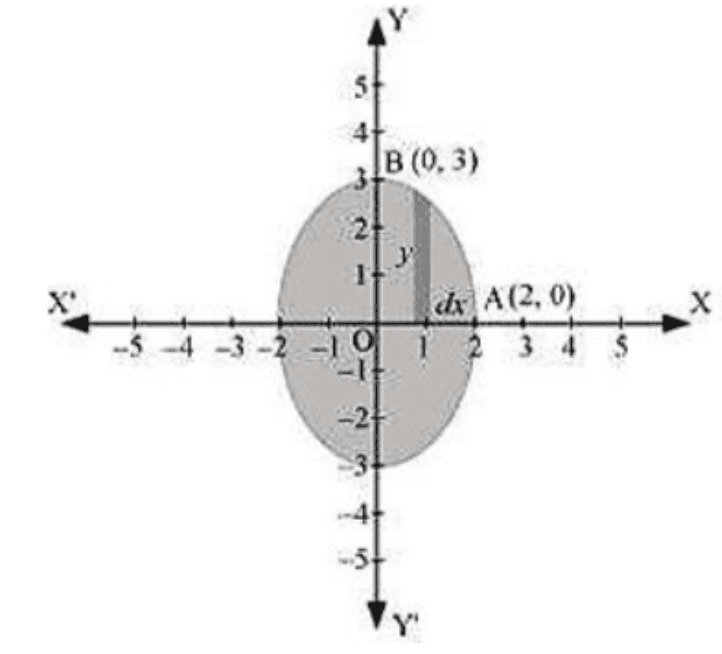
Area Bounded by Ellipse
Area of ellipse \[\text{=4 }\!\!\times\!\!\text{ }\] Area of \[\text{OAB}\]
Area of \[\text{OAB=}\int_{\text{0}}^{\text{4}}{\text{y}}\text{dx}\]
Substitute $y=3\sqrt{1-\frac{{{x}^{2}}}{16}}$
\[\text{=}\int_{\text{0}}^{\text{4}}{\text{3}}\sqrt{\text{1-}\frac{{{\text{x}}^{\text{2}}}}{\text{16}}}\text{dx}\]
simplifying,
\[\text{=}\frac{\text{3}}{\text{4}}\int_{\text{0}}^{\text{4}}{\sqrt{\text{16-}{{\text{x}}^{\text{2}}}}}\text{dx}\]
\[\text{=}\frac{\text{3}}{\text{4}}\left[ \frac{\text{x}}{\text{2}}\sqrt{\text{16-}{{\text{x}}^{\text{2}}}}\text{+}\frac{\text{16}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{x}}{\text{4}} \right]_{\text{0}}^{\text{4}}\]
Substituting the limits,
\[\text{=}\frac{\text{3}}{\text{4}}\left[ \text{2}\sqrt{\text{16-16}}\text{+8si}{{\text{n}}^{\text{-1}}}\text{(1)-0-8si}{{\text{n}}^{\text{-1}}}\text{(0)} \right]\]
\[\text{= }\frac{\text{3}}{\text{4}}\left[ \frac{\text{8 }\!\!\pi\!\!\text{ }}{\text{2}} \right]\]
\[\text{= }\frac{\text{3}}{\text{4}}\text{ }\!\![\!\!\text{ 4 }\!\!\pi\!\!\text{ }\!\!]\!\!\text{ }\]
\[\text{= 3 }\!\!\pi\!\!\text{ }\]
As a result, the ellipse's area is \[\text{= 4}\times \text{3 }\!\!\pi\!\!\text{ = 12 }\!\!\pi\!\!\text{ }\].
5. Find the area of the region bounded by the ellipse \[\frac{{{\text{x}}^{\text{2}}}}{4}\text{+}\frac{{{\text{y}}^{\text{2}}}}{\text{9}}\text{=1}\].
Ans:

Area Bounded by Ellipse
Area of ellipse \[\text{=4 }\!\!\times\!\!\text{ }\] Area of \[\text{OAB}\]
Area of \[\text{OAB=}\int_{\text{0}}^{2}{\text{y}}\text{dx}\]
Substitute $y=3\sqrt{1-\frac{{{x}^{2}}}{4}}$
\[\text{=}\int_{\text{0}}^{2}{\text{3}}\sqrt{\text{1-}\frac{{{\text{x}}^{\text{2}}}}{4}}\text{dx}\]
simplifying,
\[\text{=}\frac{\text{3}}{2}\int_{\text{0}}^{2}{\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}}\text{dx}\]
\[\text{=}\frac{\text{3}}{2}\left[ \frac{\text{x}}{\text{2}}\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}\text{+}\frac{4}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{x}}{2} \right]_{\text{0}}^{2}\]
Substituting the limits,
\[\text{=}\frac{\text{3}}{2}\left[ \frac{\text{2 }\!\!\pi\!\!\text{ }}{\text{2}} \right]\]
\[\text{=}\frac{\text{3 }\!\!\pi\!\!\text{ }}{2}\]
As a result, the ellipse's area is \[\text{4}\times \frac{\text{3 }\!\!\pi\!\!\text{ }}{2}\text{=6 }\!\!\pi\!\!\text{ }\].
6. Find the area of the region in the first quadrant enclosed by \[\text{x-axis}\], line \[\text{x=}\sqrt{3}y\] and the circle \[{{\text{x}}^{\text{2}}}\text{+}{{\text{y}}^{\text{2}}}\text{=4}\].
Ans:
(Image Will be Updated Soon)
Area of \[\text{OAB=}\]Area \[\text{ }\!\!\Delta\!\!\text{ OCA+}\]Area \[\text{ACB}\]
Area of \[\text{ }\!\!\Delta\!\!\text{ OCA=}\frac{\text{1}}{\text{2}}\text{ }\!\!\times\!\!\text{ OC }\!\!\times\!\!\text{ AC}\]
\[\text{=}\frac{\text{1}}{\text{2}}\text{ }\!\!\times\!\!\text{ }\sqrt{\text{3}}\text{ }\!\!\times\!\!\text{ 1}\]
\[\text{=}\frac{\sqrt{\text{3}}}{\text{2}}\text{ }\]
Area of \[\text{ABC=}\int_{\sqrt{\text{3}}}^{\text{2}}{\text{y}}\text{dx}\]
Substitute $y=\sqrt{4-{{x}^{2}}}$
\[\text{=}\int_{\sqrt{\text{3}}}^{\text{2}}{\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}}\text{dx}\]1
\[\text{=}\left[ \frac{\text{x}}{\text{2}}\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}\text{+}\frac{\text{4}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{x}}{\text{2}} \right]_{\sqrt{\text{3}}}^{\text{2}}\]
Substituting the limits,
\[\text{=}\left[ \text{2 }\!\!\times\!\!\text{ }\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}}\text{-}\frac{\sqrt{\text{3}}}{\text{2}}\sqrt{\text{4-3}}\text{-2si}{{\text{n}}^{\text{-1}}}\left( \frac{\sqrt{\text{3}}}{\text{2}} \right) \right]\]
\[\text{=}\left[ \text{ }\!\!\pi\!\!\text{ -}\frac{\sqrt{\text{3}}\text{ }\!\!\pi\!\!\text{ }}{\text{2}}\text{-}\frac{\text{2 }\!\!\pi\!\!\text{ }}{\text{3}} \right]\]
\[\text{=}\left[ \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{3}}\text{-}\frac{\sqrt{\text{3}}}{\text{2}} \right]\]
As a result, the area of the region enclosed in the first quadrant by \[\text{x-axis}\], line \[\text{x=}\sqrt{3}y\] and the circle \[{{\text{x}}^{\text{2}}}\text{+}{{\text{y}}^{\text{2}}}\text{=4}\] is:
\[\frac{\sqrt{\text{3}}}{\text{2}}\text{ +}\left[ \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{3}}\text{-}\frac{\sqrt{\text{3}}}{\text{2}} \right]\text{=}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{3}}\text{units}\]
7. Find the area of the smaller part of the circle \[{{\text{x}}^{\text{2}}}\text{+}{{\text{y}}^{\text{2}}}\text{=}{{\text{a}}^{2}}\] cut off by the line \[\text{x=}\frac{\text{a}}{\sqrt{\text{2}}}\].
Ans:
(Image Will be Updated Soon)
Area \[\text{ABCD=2}\times \]Area \[\text{ABC}\]
Area of \[\text{ABC=}\int_{\frac{\text{a}}{\sqrt{\text{2}}}}^{\text{2}}{\text{y}}\text{dx}\]
Substitute $y=\sqrt{{{a}^{2}}-{{x}^{2}}}$
\[\text{=}\int_{\frac{\text{a}}{\sqrt{\text{2}}}}^{\text{2}}{\sqrt{{{\text{a}}^{\text{2}}}\text{-}{{\text{x}}^{\text{2}}}}}\text{dx}\]
\[\text{=}\left[ \frac{\text{x}}{\text{2}}\sqrt{{{\text{a}}^{\text{2}}}\text{-}{{\text{x}}^{\text{2}}}}\text{+}\frac{{{\text{a}}^{\text{2}}}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{x}}{\text{a}} \right]_{\frac{\text{a}}{\sqrt{\text{2}}}}^{\text{2}}\]
Substituting the limits,
\[\text{=}\left[ \left( \frac{{{\text{a}}^{\text{2}}}}{\text{2}} \right)\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}}\text{-}\frac{\text{a}}{\text{2}\sqrt{\text{2}}}\sqrt{{{\text{a}}^{\text{2}}}\text{-}\frac{{{\text{a}}^{\text{2}}}}{\text{2}}}\text{-}\frac{{{\text{a}}^{\text{2}}}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\left( \frac{\text{1}}{\sqrt{\text{2}}} \right) \right]\]
\[\text{=}\left[ \frac{{{\text{a}}^{\text{2}}}\text{ }\!\!\pi\!\!\text{ }}{\text{4}}\text{-}\frac{{{\text{a}}^{\text{2}}}}{\text{4}}\text{-}\frac{{{\text{a}}^{\text{2}}}\text{ }\!\!\pi\!\!\text{ }}{\text{8}} \right]\]
\[\text{=}\frac{{{\text{a}}^{\text{2}}}}{\text{4}}\left[ \text{ }\!\!\pi\!\!\text{ -1-}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}} \right]\]
\[\text{=}\frac{{{\text{a}}^{\text{2}}}}{\text{4}}\left[ \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}}\text{-1} \right]\]
Therefore, Area of \[\text{ABCD=2 }\!\!\times\!\!\text{ }\left[ \frac{{{\text{a}}^{\text{2}}}}{\text{4}}\left( \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}}\text{-1} \right) \right]=\frac{{{\text{a}}^{\text{2}}}}{2}\left( \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}}\text{-1} \right)\].
As a result, the area of the smaller section of \[{{\text{x}}^{\text{2}}}\text{+}{{\text{y}}^{\text{2}}}\text{=}{{\text{a}}^{2}}\] is \[\frac{{{\text{a}}^{\text{2}}}}{2}\left( \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}}\text{-1} \right)units\].
8. The area between \[\text{x = }{{\text{y}}^{\text{2}}}\] and \[\text{x = 4}\] is divided into two equal parts by line \[\text{x = a}\], find the value of \[\text{a}\].
Ans: It is considered that the parabola-bounded area \[\text{x = }{{\text{y}}^{\text{2}}}\] and \[\text{x = 4}\] is divided into two equal parts by the line \[\text{x = a}\].
Therefore, \[\text{Area OAD = Area ABCD}\]
(Image Will be Updated Soon)
\[\text{Area OED = Area EFCD}\]
\[\text{Area OED=}\int_{0}^{a}{\text{y}}\text{dx}\]
Substitute $y=\sqrt{x}$
Integrating using the power rule
\[\text{=}\int_{0}^{a}{\sqrt{\text{x}}\text{dx}}\]
\[\text{=}\left[ \frac{\frac{{{\text{x}}^{\frac{\text{3}}{\text{2}}}}}{\text{3}}}{\text{2}} \right]_{0}^{a}\]
Substituting the limits,
\[\text{=}\frac{\text{2}}{\text{3}}{{\left( \text{a} \right)}^{\frac{\text{3}}{\text{2}}}}\]
\[\text{Area EFCD=}\int_{0}^{4}{\sqrt{x}}\text{dx}\]
Integrating using the power rule,
\[\text{=}\left[ \frac{\frac{{{\text{x}}^{\frac{\text{3}}{\text{2}}}}}{\text{3}}}{\text{2}} \right]_{0}^{\text{4}}\]
Substituting the limits,
\[\text{=}\frac{\text{2}}{\text{3}}\left[ \text{8-}{{\text{a}}^{\frac{\text{3}}{\text{2}}}} \right]\]
So, \[\frac{\text{2}}{\text{3}}{{\left( a \right)}^{\frac{\text{3}}{\text{2}}}}\text{=}\frac{\text{2}}{\text{3}}\left[ \text{8-}{{\left( \text{a} \right)}^{\frac{\text{3}}{\text{2}}}} \right]\]
\[\Rightarrow \text{2}{{\left( \text{a} \right)}^{\frac{\text{3}}{\text{2}}}}\text{=8}\]
\[\Rightarrow {{\left( \text{a} \right)}^{\frac{\text{3}}{\text{2}}}}\text{=4}\]
\[\Rightarrow \text{a=}{{\left( \text{4} \right)}^{\frac{2}{3}}}\]
Hence, the value of \[\text{a}\] is \[{{\left( \text{4} \right)}^{\frac{2}{3}}}\].
9. Find the area of the region bounded by the parabola \[\text{y=}{{\text{x}}^{\text{2}}}\] and \[\text{y=}\left| x \right|\].
Ans:
(Image Will be Updated Soon)
\[Area\text{ }OACO\text{ }=\text{ }Area\text{ }ODBO\]
The point where \[\text{y=}{{\text{x}}^{\text{2}}}\] and \[\text{y=x}\] intersect is \[\left( \text{1, 1} \right)\].
Area of \[\text{OACM = Area }\Delta \text{OAM - Area OCAM}\]
Area of \[\Delta \text{OAM = }\frac{1}{2}\times OM\times AM\]
\[=\frac{1}{2}\times 1\times 1\]
\[=\frac{1}{2}\]
Area of \[\text{OACM=}\int_{0}^{1}{\text{y}}\text{dx}\]
substitute $y={{x}^{2}}$
\[\text{=}\int_{0}^{1}{{{\text{x}}^{\text{2}}}}\text{dx}\]
Integrating using the power rule
\[\text{=}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{0}}^{\text{1}}\]
Substituting the limits,
\[\text{=}\frac{\text{1}}{\text{3}}\]
So, Area of \[\text{OACO = }\frac{\text{1}}{\text{2}}\text{ - }\frac{\text{1}}{\text{3}}\]
\[\text{=}\frac{\text{1}}{6}\]
As a result, the required area is \[\text{=2}\left[ \frac{\text{1}}{\text{6}} \right]\text{=}\frac{\text{1}}{\text{3}}\].
10. Find the area bounded by the curve \[{{\text{x}}^{\text{2}}}\text{=4y}\] and the line \[\text{x = 4y -- 2}\].
Ans: The parabola and the line intersect each other at positions A and B.
(Image Will be Updated Soon)
The coordinates of point are \[\left( \text{-1, }\frac{1}{4} \right)\].
The coordinates of point are \[\left( \text{2, 1} \right)\].
Draw \[AL\] and \[\text{BM}\] perpendicular to \[\text{x-axis}\].
\[\text{Area OBAO = Area OBCO + Area OACO}\]
\[\text{Area OBCO = Area OMBC - Area OMBO}\]
\[\text{=}\int_{\text{0}}^{\text{2}}{\frac{\text{x+2}}{\text{4}}}\text{dx-}\int_{\text{0}}^{\text{2}}{\frac{{{\text{x}}^{\text{2}}}}{\text{4}}}\text{dx}\]
Integrating using the power rule
\[\text{=}\frac{\text{1}}{\text{4}}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+2x} \right]_{\text{0}}^{\text{2}}\text{-}\frac{\text{1}}{\text{4}}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{0}}^{\text{2}}\]
Substituting the limits,
\[\text{=}\frac{\text{1}}{\text{4}}\text{ }\!\![\!\!\text{ 2+4 }\!\!]\!\!\text{ -}\frac{\text{1}}{\text{4}}\left[ \frac{\text{8}}{\text{3}} \right]\]
\[\text{=}\frac{\text{3}}{\text{2}}\text{-}\frac{\text{2}}{\text{3}}\]
\[\text{=}\frac{\text{5}}{\text{6}}\]
\[\text{AreaOACO=AreaOLAC-AreaOLAO}\]
\[\text{=}\int_{\text{-1}}^{\text{0}}{\frac{\text{x+2}}{\text{4}}}\text{dx-}\int_{\text{-1}}^{\text{0}}{\frac{{{\text{x}}^{\text{2}}}}{\text{4}}}\text{dx}\]
Integrating using the power rule
\[\text{=}\frac{\text{1}}{\text{4}}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+2x} \right]_{\text{1}}^{\text{0}}\text{-}\frac{\text{1}}{\text{4}}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{-1}}^{\text{0}}\]
Substituting the limits,
\[\text{=-}\frac{\text{1}}{\text{4}}\left[ \frac{\text{(-1)}}{\text{2}}\text{+2(-1)} \right]\text{-}\left[ \text{-}\frac{\text{1}}{\text{4}}\left( \frac{{{\text{(-1)}}^{\text{3}}}}{\text{3}} \right) \right]\]
\[\text{=-}\frac{\text{1}}{\text{4}}\left[ \frac{\text{1}}{\text{2}}\text{-2} \right]\text{-}\frac{\text{1}}{\text{2}}\]
\[\text{=}\frac{\text{1}}{\text{2}}\text{-}\frac{\text{1}}{\text{8}}\text{-}\frac{\text{1}}{\text{12}}\]
\[\text{=}\frac{\text{7}}{\text{24}}\]
As a result, the required area is \[\text{=}\left( \frac{\text{5}}{\text{6}}\text{+}\frac{\text{7}}{\text{24}} \right)\text{=}\frac{\text{9}}{\text{8}}\text{units}\].
11. Find the area of the region bounded by the curve \[{{y}^{\text{2}}}\text{=4x}\] and the line \[\text{x = 3}\].
Ans:
(Image Will be Updated Soon)
\[Area\text{ }of\text{ }OACO\text{ }=\text{ }2\text{ }\left( Area\text{ }of\text{ }OAB \right)\]
\[Area\text{ }OACO\text{=2}\left[ \int_{\text{0}}^{3}{\text{y}}\text{dx} \right]\]
Substitute $y=2\sqrt{x}$
\[\text{=2}\left[ \int_{0}^{3}{2\sqrt{x}}\text{dx} \right]\]
Integrating using the power rule
\[\text{=4}\left[ \frac{\frac{{{\text{x}}^{\frac{\text{3}}{\text{2}}}}}{\text{3}}}{\text{2}} \right]_{0}^{3}\]
Substituting the limits,
\[\text{=}\frac{8}{\text{3}}\left[ {{\left( 3 \right)}^{\frac{\text{3}}{\text{2}}}} \right]\]
\[\text{=8}\sqrt{3}\]
As a result, the required area is \[\text{8}\sqrt{\text{3}}\text{ units}\].
12. Area lying in the first quadrant and bounded by the circle \[{{\text{x}}^{\text{2}}}\text{ + }{{\text{y}}^{\text{2}}}\text{ = 4}\] and the lines \[\text{x=0}\]
A. \[\text{ }\!\!\pi\!\!\text{ }\]
B. \[\frac{\text{ }\!\!\pi\!\!\text{ }}{2}\]
C. \[\frac{\text{ }\!\!\pi\!\!\text{ }}{3}\]
D. \[\frac{\text{ }\!\!\pi\!\!\text{ }}{4}\]
Ans:
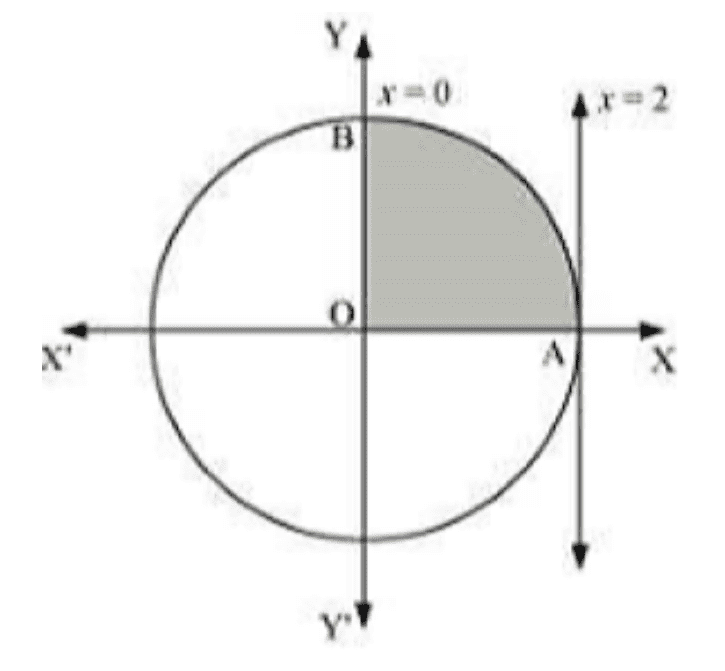
Area Lying in the First Quadrant and Bounded by the Circle
\[\text{Area OAB=}\int_{0}^{\text{2}}{\text{y}}\text{dx}\]
substitute$y=\sqrt{4-{{x}^{2}}}$
\[\text{=}\int_{\text{0}}^{\text{2}}{\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}}\text{dx}\]1
\[\text{=}\left[ \frac{\text{x}}{\text{2}}\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}\text{+}\frac{\text{4}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{x}}{\text{2}} \right]_{\text{0}}^{\text{2}}\]
Substituting the limits,
\[\text{=2}\left( \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}} \right)\]
\[\text{= }\!\!\pi\!\!\text{ }\]
As a result, the required area is \[\text{ }\!\!\pi\!\!\text{ }\] option A.
13. Area of the region bounded by the curve \[{{\text{y}}^{\text{2}}}\text{ = 4x, y-axis}\] and the line \[\text{y=3}\] is
A. \[2\]
B. \[\frac{9}{4}\]
C. \[\frac{9}{3}\]
D. \[\frac{9}{2}\]
Ans:
(Image Will be Updated Soon)
\[\text{Area OAB=}\int_{0}^{3}{x}\text{dy}\]
substitute $x=\frac{{{y}^{2}}}{4}$
\[\text{=}\int_{\text{0}}^{3}{\frac{{{y}^{2}}}{4}}\text{dy}\]
\[\text{=}\frac{1}{4}\left[ \frac{{{y}^{3}}}{4} \right]_{\text{0}}^{3}\]
Substituting the limits,
\[\text{=}\frac{1}{\text{12}}\left( 27 \right)\]
\[\text{=}\frac{\text{9}}{\text{4}}\text{ units}\]
As a result, the correct response is \[\frac{\text{9}}{\text{4}}\text{ units}\] option B.
Exercise 8.2
1. Find the area of the circle \[\text{4}{{\text{x}}^{\text{2}}}\text{ + 4}{{\text{y}}^{\text{2}}}\text{ = 9}\] which is interior to the parabola \[{{\text{x}}^{\text{2}}}\text{ = 4y}\].
Ans: The circle and parabola intersect at points \[\text{B}\left( \sqrt{\text{2}}\text{, }\frac{\text{1}}{\text{2}} \right)\] and \[D\left( -\sqrt{\text{2}}\text{, }\frac{\text{1}}{\text{2}} \right)\].
(Image Will be Updated Soon)
Since the required area is symmetrical about \[\text{y-axis}\], \[\text{Area OBCDO = 2}\times \text{Area OBCO}\]
Sketch a perpendicular to \[OA\], the coordinates of point M are \[\left( \sqrt{\text{2}}\text{, 0} \right)\].
Now,
\[\text{AreaOBCO=AreaOMBCO-AreaOMBO}\]
\[\text{=}\int_{\text{0}}^{\sqrt{\text{2}}}{\sqrt{\frac{\left( \text{9-4}{{\text{x}}^{\text{2}}} \right)}{\text{4}}}}\text{dx-}\int_{\text{0}}^{\sqrt{\text{2}}}{\sqrt{\frac{{{\text{x}}^{\text{2}}}}{\text{4}}}}\]
Simplifying,
\[\text{=}\frac{\text{1}}{\text{2}}\int_{\text{0}}^{\sqrt{\text{2}}}{\sqrt{\text{9-4}{{\text{x}}^{\text{2}}}}}\text{dx-}\frac{\text{1}}{\text{4}}\int_{\text{0}}^{\sqrt{\text{2}}}{{{\text{x}}^{\text{2}}}}\text{dx}\]
\[\text{=}\frac{\text{1}}{\text{4}}\left[ \text{x}\sqrt{\text{9-4}{{\text{x}}^{\text{2}}}}\text{+}\frac{\text{9}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{2x}}{\text{3}} \right]_{\text{0}}^{\sqrt{\text{2}}}\text{-}\frac{\text{1}}{\text{4}}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{0}}^{\sqrt{\text{2}}}\]
Substituting the limits,
\[\text{=}\frac{\text{1}}{\text{4}}\left[ \sqrt{\text{2}}\sqrt{\text{9-8}}\text{+}\frac{\text{9}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{2}\sqrt{\text{2}}}{\text{3}} \right]\text{-}\frac{\text{1}}{\text{12}}{{\text{(}\sqrt{\text{2}}\text{)}}^{\text{3}}}\]
\[\text{=}\frac{\sqrt{\text{2}}}{\text{4}}\text{+}\frac{\text{9}}{\text{8}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{2}\sqrt{\text{2}}}{\text{3}}\text{-}\frac{\sqrt{\text{2}}}{\text{6}}\text{=}\frac{\sqrt{\text{2}}}{\text{12}}\text{+}\frac{\text{9}}{\text{8}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{2}\sqrt{\text{2}}}{\text{3}}\]
\[\text{=}\frac{\text{1}}{\text{2}}\left( \frac{\sqrt{\text{2}}}{\text{6}}\text{+}\frac{\text{9}}{\text{4}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{2}\sqrt{\text{2}}}{\text{3}} \right)\]
Therefore, the required area is \[\text{=}\left( \text{2 }\!\!\times\!\!\text{ }\frac{\text{1}}{\text{2}}\left[ \frac{\sqrt{\text{2}}}{\text{6}}\text{+}\frac{\text{9}}{\text{4}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{2}\sqrt{\text{2}}}{\text{3}} \right] \right)\]
\[\text{=}\left[ \frac{\sqrt{\text{2}}}{\text{6}}\text{+}\frac{\text{9}}{\text{4}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{2}\sqrt{\text{2}}}{\text{3}} \right]\text{units}\]
2. Find the area bounded by curves \[{{\left( \text{x-1} \right)}^{\text{2}}}\text{+}{{\text{y}}^{\text{2}}}\text{=1}\] and \[{{x}^{\text{2}}}\text{+}{{\text{y}}^{\text{2}}}\text{=1}\]
Ans:
(Image Will be Updated Soon)
The point of intersection the two given circles are \[\text{A}\left( \frac{\text{1}}{2},\text{ }\frac{\sqrt{3}}{2} \right)\] and \[B\left( \frac{\text{1}}{2},\text{ -}\frac{\sqrt{3}}{2} \right)\].
The required area is symmetrical about \[\text{x-axis}\].
Therefore, \[\text{Area OBCAO = 2}\times \text{Area OCAO}\]
Join \[\text{AB}\] which intersects \[\text{OC}\] at \[\text{M}\left( \frac{\text{1}}{\text{2}}\text{, 0} \right)\].
\[\text{Area OACO = Area OMAO + Area MCAM}\]
\[\text{=}\left[ \int_{\text{0}}^{\frac{\text{1}}{\text{2}}}{\sqrt{\text{1-(x-1}{{\text{)}}^{\text{2}}}}}\text{dx+}\int_{\frac{\text{1}}{\text{2}}}^{\text{1}}{\sqrt{\text{1-}{{\text{x}}^{\text{2}}}}}\text{dx} \right]\]
\[\text{=}\left[ \frac{\text{x-1}}{\text{2}}\sqrt{\text{1-(x-1}{{\text{)}}^{\text{2}}}}\text{+}\frac{\text{1}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\text{(x-1)} \right]_{\text{0}}^{\frac{\text{1}}{\text{2}}}\text{+}\left[ \frac{\text{x}}{\text{2}}\sqrt{\text{1-}{{\text{x}}^{\text{2}}}}\text{+}\frac{\text{1}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\text{x} \right]_{\frac{\text{1}}{\text{2}}}^{\text{1}}\]
Substituting the limits,
\[\text{=}\left[ \text{-}\frac{\text{1}}{\text{4}}\sqrt{\text{1-}{{\left( \text{-}\frac{\text{1}}{\text{2}} \right)}^{\text{2}}}}\text{+}\frac{\text{1}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\left( \frac{\text{1}}{\text{2}}\text{-1} \right)\text{-}\frac{\text{1}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\text{(-1)} \right]\text{+}\left[ \frac{\text{1}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\text{(-1)-}\frac{\text{1}}{\text{4}}\sqrt{\text{1-}{{\left( \text{-}\frac{\text{1}}{\text{2}} \right)}^{\text{2}}}}\text{-}\frac{\text{1}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\left( \frac{\text{1}}{\text{2}} \right) \right]\]
\[\text{=}\left[ \text{-}\frac{\sqrt{\text{3}}}{\text{8}}\text{+}\frac{\text{1}}{\text{2}}\left( \text{-}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{6}} \right)\text{-}\frac{\text{1}}{\text{2}}\left( \text{-}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}} \right) \right]\text{+}\left[ \frac{\text{1}}{\text{2}}\left( \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}} \right)\text{-}\frac{\sqrt{\text{3}}}{\text{8}}\text{-}\frac{\text{1}}{\text{2}}\left( \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{6}} \right) \right]\]
\[\text{=}\left[ \text{-}\frac{\sqrt{\text{3}}}{\text{4}}\text{-}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{12}}\text{+}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{4}}\text{+}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{4}}\text{-}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{12}} \right]\]
\[\text{=}\left[ \text{-}\frac{\sqrt{\text{3}}}{\text{4}}\text{-}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{6}}\text{+}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}} \right]\]
\[\text{=}\left[ \frac{\text{2 }\!\!\pi\!\!\text{ }}{\text{6}}\text{-}\frac{\sqrt{\text{3}}}{\text{4}} \right]\]
Therefore, the required area \[\text{OBCAO=2 }\!\!\times\!\!\text{ }\left( \frac{\text{2 }\!\!\pi\!\!\text{ }}{\text{6}}\text{-}\frac{\sqrt{\text{3}}}{\text{4}} \right)\]
\[\text{=}\left( \frac{\text{2 }\!\!\pi\!\!\text{ }}{\text{3}}\text{-}\frac{\sqrt{\text{3}}}{\text{2}} \right)\text{units}\]
3. Find the area of the region bounded by the curves \[\text{y=}{{\text{x}}^{\text{2}}}\text{+2,y=x,x=0}\] and \[\text{x=3}\].
Ans:
(Image Will be Updated Soon)
\[\text{Area OCBAO = Area ODBAO - Area ODCO}\]
\[\text{=}\int_{\text{0}}^{\text{3}}{\left( {{\text{x}}^{\text{2}}}\text{+2} \right)}\text{dx-}\int_{\text{0}}^{\text{3}}{\text{x}}\text{dx}\]
Integrating using the power rule,
\[\text{=}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}}\text{+2x} \right]_{\text{0}}^{\text{3}}\text{-}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}} \right]_{\text{0}}^{\text{3}}\]
Substituting the limits,
\[\text{= }\!\![\!\!\text{ 9+6 }\!\!]\!\!\text{ -}\left[ \frac{\text{9}}{\text{2}} \right]\]
\[\text{=15-}\frac{\text{9}}{\text{2}}\]
\[\text{=}\frac{\text{21}}{\text{2}}\text{ units}\]
4. Using integration find the area of the region bounded by the triangle whose vertices are \[\left( -1\text{, 0} \right)\text{,}\left( \text{1, 3} \right)\] and \[\left( \text{3, 2} \right)\].
Ans:
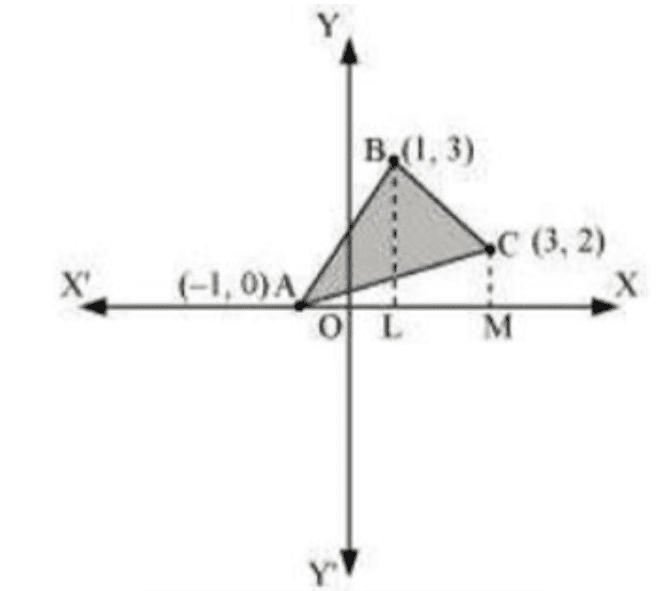
Area Bounded by Triangle with Vertices (-1,0),(3,2) and (1,3)
As shown in the diagram, \[BL\] and \[CM\] are perpendicular to \[\text{x-axis}\].
\[\text{Area }\left( \text{ }\!\!\Delta\!\!\text{ ACB} \right)\text{ = Area }\left( \text{ALBA} \right)\text{ + Area }\left( \text{BLMCB} \right)\text{ - Area }\left( AMCA \right)\]
Write the equation of \[AB\]:
\[\text{y-0=}\frac{\text{3-0}}{\text{1+1}}\text{(x+1)}\]
\[\text{y=}\frac{\text{3}}{\text{2}}\text{(x+1)}\]
\[\text{Area(ALBA)=}\int_{\text{-1}}^{\text{1}}{\frac{\text{3}}{\text{2}}}\text{(x+1)dx}\]
Integrating using the power rule,
\[\text{=}\frac{\text{3}}{\text{2}}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+x} \right]_{\text{-1}}^{\text{1}}\]
Substituting the limits,
\[\text{=}\frac{\text{3}}{\text{2}}\left[ \frac{\text{1}}{\text{2}}\text{+1-}\frac{\text{1}}{\text{2}}\text{+1} \right]\]
\[\text{=3units}\]
Write the equation of \[\text{BC}\]:
\[\text{y-3=}\frac{\text{2-3}}{\text{3-1}}\text{(x-1)}\]
\[\text{y=}\frac{\text{1}}{\text{2}}\text{(-x+7)}\]
Therefore, \[\text{Area(BLMCB)=}\int_{\text{1}}^{\text{3}}{\frac{\text{1}}{\text{2}}}\text{(-x+7)dx}\]
\[\text{=}\frac{\text{1}}{\text{2}}\left[ \text{-}\frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+7x} \right]_{\text{1}}^{\text{3}}\]
\[\text{=}\frac{\text{1}}{\text{2}}\left[ \text{-}\frac{\text{9}}{\text{2}}\text{+21+}\frac{\text{1}}{\text{2}}\text{-7} \right]\]
\[\text{=5units}\]
Write the equation of \[\text{AC}\]:
\[\text{y-0=}\frac{\text{2-0}}{\text{3+1}}\text{(x+1)}\]
\[\text{y=}\frac{\text{1}}{\text{2}}\text{(x+1)}\]
Therefore, \[\text{Area(AMCA)=}\int_{\text{-1}}^{\text{3}}{\frac{\text{1}}{\text{2}}}\text{(x+1)dx}\]
\[\text{=}\frac{\text{1}}{\text{2}}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+x} \right]_{\text{-1}}^{\text{3}}\]
\[\text{=}\frac{\text{1}}{\text{2}}\left[ \frac{\text{9}}{\text{2}}\text{+3-}\frac{\text{1}}{\text{2}}+1 \right]\]
\[\text{=4units}\]
So, \[\text{Area }\left( \text{ }\!\!\Delta\!\!\text{ ABC} \right)\text{ = }\left( \text{3+5-4} \right)\text{ = 4 units}\].
5. Using integration find the area of the triangle whose sides have equations \[\text{y=2x+1,y=3x+1}\] and \[\text{x=4}\].
Ans:
(Image Will be Updated Soon)
As shown in the diagram, vertices of the triangle are \[\text{A}\left( \text{0, 1} \right)\text{, B}\left( \text{4, 13} \right)\] and \[\text{C}\left( \text{4, 9} \right)\].
\[\text{Area( }\!\!\Delta\!\!\text{ ACB)=Area(OLBAO)-Area(OLCAO)}\]
\[\text{=}\int_{\text{0}}^{\text{4}}{\text{(3x+1)}}\text{dx-}\int_{\text{0}}^{\text{4}}{\text{(2x+1)}}\text{dx}\]
Integrating using the power rule
\[\text{=}\left[ \frac{\text{3}{{\text{x}}^{\text{2}}}}{\text{2}}\text{+x} \right]_{\text{0}}^{\text{4}}\text{-}\left[ \frac{\text{2}{{\text{x}}^{\text{2}}}}{\text{2}}\text{+x} \right]_{\text{0}}^{\text{4}}\]
Substituting the limits,
\[\text{=(24+4)-(16+4)}\]
\[\text{=28-20}\]
\[\text{=8units}\]
6. Smaller area enclosed by the circle \[{{\text{x}}^{2}}\text{+}{{\text{y}}^{2}}\text{=4}\] and the line \[\text{x+y=2}\] is
A. \[\text{2}\left( \text{ }\!\!\pi\!\!\text{ -2} \right)\]
B. \[\text{ }\!\!\pi\!\!\text{ -2}\]
C. \[\text{2 }\!\!\pi\!\!\text{ -1}\]
D. \[\text{2}\left( \text{ }\!\!\pi\!\!\text{ +2} \right)\]
Ans:
(Image Will be Updated Soon)
\[\text{ Area ACBA= Area OACBO- Area ( }\!\!\Delta\!\!\text{ OAB)}\]
\[\text{=}\int_{\text{0}}^{\text{2}}{\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}}\text{dx-}\int_{\text{0}}^{\text{2}}{\text{(2-x)}}\text{dx}\]
\[\text{=}\left[ \frac{\text{x}}{\text{2}}\sqrt{\text{4-}{{\text{x}}^{\text{2}}}}\text{+}\frac{\text{4}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{x}}{\text{2}} \right]_{\text{0}}^{\text{2}}\text{-}\left[ \text{2x-}\frac{\text{x}}{\text{2}} \right]_{\text{0}}^{\text{2}}\]
Substituting the limits,
\[\text{=}\left[ \text{2 }\!\!\times\!\!\text{ }\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}} \right]\text{- }\!\![\!\!\text{ 4-2 }\!\!]\!\!\text{ }\]
\[\text{=( }\!\!\pi\!\!\text{ -2) units }\]
As a result, the correct response is \[\text{( }\!\!\pi\!\!\text{ -2) units}\] option B.
7. Area lying between the curve \[{{\text{y}}^{2}}\text{=4x}\] and \[\text{y=2x}\] is
A. \[\frac{2}{3}\]
B. \[\frac{1}{3}\]
C. \[\frac{1}{4}\]
D. \[\frac{3}{4}\]
Ans:
(Image Will be Updated Soon)
As shown in the diagram, the point of intersection of the given curves are \[\text{O}\left( \text{0, 0} \right)\] and \[A\left( \text{1, 2} \right)\], \[AC\] is perpendicular to \[\text{x-axis}\] and the coordinates of \[\text{C}\] are \[\left( \text{1, 0} \right)\].
\[\text{AreaOBAO=Area( }\!\!\Delta\!\!\text{ OCA)-Area(OCABO)}\]
\[\text{=}\int_{\text{0}}^{\text{1}}{\text{2}}\text{xdx-}\int_{\text{0}}^{\text{1}}{\text{2}}\sqrt{\text{x}}dx\]
Integrating using the power rule,
\[\text{=2}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}} \right]_{\text{0}}^{\text{1}}\text{-2}\left[ \frac{{{\text{x}}^{\frac{\text{3}}{\text{2}}}}}{\frac{\text{3}}{\text{2}}} \right]_{\text{0}}^{\text{1}}\]
Substituting the limits,
\[\text{=}\left| \text{1-}\frac{\text{4}}{\text{3}} \right|\]
\[\text{=}\left| \text{-}\frac{\text{1}}{\text{3}} \right|\]
\[\text{=}\frac{\text{1}}{\text{3}}\text{units}\]
Therefore, the correct answer is \[\frac{\text{1}}{\text{3}}\text{units}\] option B.
Miscellaneous Exercise
1. Find the area under the given curves and given lines:
(i) \[\text{y=}{{\text{x}}^{\text{2}}}\text{,x=1,x=2}\] and \[\text{x-axis}\]
Ans:
(Image Will be Updated Soon)
\[\text{AreaADCBA=}\int_{\text{1}}^{\text{2}}{\text{y}}\text{dx}\]
substitute $y={{x}^{2}}$
\[\text{=}\int_{\text{1}}^{\text{2}}{{{\text{x}}^{\text{2}}}}\text{dx}\]
Substituting the limits,
\[\text{=}\frac{\text{8}}{\text{3}}\text{-}\frac{\text{1}}{\text{3}}\]
\[\text{=}\frac{\text{7}}{\text{3}}\text{units}\]
(ii) \[\text{y=}{{\text{x}}^{4}}\text{,x=1,x=5}\] and \[\text{x-axis}\]
Ans:
(Image Will be Updated Soon)
\[\text{AreaofADCBA=}\int_{\text{1}}^{\text{5}}{{{\text{x}}^{\text{4}}}}\text{dx}\]
Integrating using the power rule,
\[\text{=}\left[ \frac{{{\text{x}}^{\text{5}}}}{\text{5}} \right]_{\text{1}}^{\text{5}}\]
Substituting the limits,
\[\text{=}\frac{{{\text{(5)}}^{\text{5}}}}{\text{5}}\text{-}\frac{\text{1}}{\text{5}}\]
Simplifying,
\[\text{=(5}{{\text{)}}^{\text{4}}}\text{-}\frac{\text{1}}{\text{5}}\]
\[\text{=625-}\frac{\text{1}}{\text{5}}\]
\[\text{=624}\text{.8 units}\]
2. Find the area between the curves \[\text{y=x}\] and \[\text{y=}{{\text{x}}^{2}}\].
Ans:
(Image Will be Updated Soon)
The point at which the two curves overlap is \[\text{A}\left( \text{1, 1} \right)\].
\[\text{AC}\] is perpendicular to \[\text{x-axis}\].
Therefore, \[\text{Area (OBAO)= Area ( }\!\!\Delta\!\!\text{ OCA)- Area (OCABO)}\]
\[\text{=}\int_{\text{0}}^{\text{1}}{\text{x}}\text{dx-}\int_{\text{0}}^{\text{1}}{{{\text{x}}^{\text{2}}}}\text{dx}\]
Integrating using the power rule
\[\text{=}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}} \right]_{\text{0}}^{\text{1}}\text{-}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{0}}^{\text{1}}\]
Substituting the limits,
\[\text{=}\frac{\text{1}}{\text{2}}\text{-}\frac{\text{1}}{\text{3}}\]
\[\text{=}\frac{\text{1}}{\text{6}}\text{ units}\]
3. Find the area of the region lying in the first quadrant and bounded by \[\text{y=4}{{\text{x}}^{2}}\text{,x=0,y=1}\] and \[\text{y=4}\].
Ans:
Area Bounded by Curve y=4x2, y=1 and y=4
\[\text{Area ABCD=}\int_{\text{1}}^{\text{4}}{\text{x}}\text{dx}\]
Substitute $x=\frac{\sqrt{y}}{2}$
\[\text{=}\int_{\text{1}}^{\text{4}}{\frac{\sqrt{y}}{\text{2}}}\text{dx}\]
Integrating using the power rule,
\[\text{=}\frac{\text{1}}{\text{2}}\left[ \frac{{{\text{y}}^{\frac{\text{3}}{\text{2}}}}}{\frac{\text{3}}{\text{2}}} \right]_{\text{1}}^{\text{4}}\]
Substituting the limits,
\[\text{=}\frac{\text{1}}{\text{3}}\left[ {{\text{(4)}}^{\frac{\text{3}}{\text{2}}}}\text{-1} \right]\]
Simplifying,
\[\text{=}\frac{\text{1}}{\text{3}}\text{ }\!\![\!\!\text{ 8-1 }\!\!]\!\!\text{ }\]
\[\text{=}\frac{\text{7}}{\text{3}}\text{ units}\]
4. Sketch the graph of \[\text{y=}\left| \text{x+3} \right|\] and evaluate \[\int_{-6}^{0}{\left| \text{x+3} \right|}\text{dx}\].
Ans:
\[\text{x}\] | \[\text{-6}\] | \[\text{-5}\] | \[\text{-4}\] | \[\text{-3}\] | \[\text{-2}\] | \[\text{-1}\] | \[\text{0}\] |
\[\text{y}\] | \[\text{3}\] | \[\text{2}\] | \[\text{1}\] | \[\text{0}\] | \[\text{1}\] | \[\text{2}\] | \[\text{3}\] |
(Image Will be Updated Soon)
\[\left( \text{x+3} \right)\le 0\] for \[\text{-6}\le \text{x}\le \text{-3}\]
\[\left( \text{x+3} \right)\ge \text{0}\] for \[\text{-3}\le \text{x}\le 0\]
Therefore,
\[\int_{\text{-6}}^{\text{0}}{\text{ }\!\!|\!\!\text{ }}\text{(x+3) }\!\!|\!\!\text{ dx=-}\int_{\text{-6}}^{\text{-3}}{\text{(x+3)}}\text{dx+}\int_{\text{-3}}^{\text{0}}{\text{(x+3)}}\text{dx}\]
Integrating using the power rule
\[\text{= -}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+3x} \right]_{\text{-6}}^{\text{-3}}\text{+}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+3x} \right]_{\text{-3}}^{\text{0}}\]
Substituting the limits,
\[\text{=-}\left[ \left( \frac{{{\text{(-3)}}^{\text{2}}}}{\text{2}}\text{+3(-3)} \right)\text{-}\left( \frac{{{\text{(-6)}}^{\text{2}}}}{\text{2}}\text{+3(-6)} \right) \right]\text{+}\left[ \text{0-}\left( \frac{{{\text{(-3)}}^{\text{2}}}}{\text{2}}\text{+3(-3)} \right) \right]\]
Simplifying,
\[\text{= -}\left[ \text{-}\frac{\text{9}}{\text{2}} \right]\text{-}\left[ \text{-}\frac{\text{9}}{\text{2}} \right]\]
\[\text{=9}\]
5. Find the area bounded by the curve \[\text{y=sinx}\] between \[\text{x=0}\] and \[\text{x=2 }\!\!\pi\!\!\text{ }\].
Ans:
Therefore, \[\text{area = Area OABO+ Area BCDB}\]
Area Bounded by Curve y=sinx
\[\text{=}\int_{\text{0}}^{\text{ }\!\!\pi\!\!\text{ }}{\text{sin}}\text{xdx+}\left| \int_{\text{ }\!\!\pi\!\!\text{ }}^{\text{2 }\!\!\pi\!\!\text{ }}{\text{sin}}\text{xdx} \right|\]
\[\text{= }\!\![\!\!\text{ -cosx }\!\!]\!\!\text{ }_{\text{0}}^{\text{ }\!\!\pi\!\!\text{ }}\text{+}\left| \text{ }\!\![\!\!\text{ -cosx }\!\!]\!\!\text{ }_{\text{ }\!\!\pi\!\!\text{ }}^{\text{2 }\!\!\pi\!\!\text{ }} \right|\]
Substituting the limits,
\[\text{= }\!\![\!\!\text{ -cos }\!\!\pi\!\!\text{ +cos0 }\!\!]\!\!\text{ + }\!\!|\!\!\text{ -cos2 }\!\!\pi\!\!\text{ +cos }\!\!\pi\!\!\text{ }\!\!|\!\!\text{ }\]
Simplifying,
\[\text{=1+1+ }\!\!|\!\!\text{ (-1-1) }\!\!|\!\!\text{ }\]
\[\text{=2+ }\!\!|\!\!\text{ -2 }\!\!|\!\!\text{ }\]
\[\text{=2+2}\]
\[\text{=4 units}\]
6. Find the area enclosed between the parabola \[{{\text{y}}^{\text{2}}}\text{=4ax}\] and the line \[\text{y=mx}\].
Ans:
(Image Will be Updated Soon)
The point of intersection for the given curves are \[\left( \text{0, 0} \right)\] and \[\left( \frac{4a}{{{m}^{2}}}\text{, }\frac{4a}{m} \right)\]
\[AC\] is perpendicular to \[\text{x-axis}\].
\[\text{Area OABO= Area OCABO- Area ( }\!\!\Delta\!\!\text{ OCA)}\]
\[\text{=}\int_{\text{0}}^{\frac{\text{4x}}{{{\text{m}}^{\text{2}}}}}{\text{2}}\sqrt{\text{ax}}\text{dx-}\int_{\text{0}}^{\frac{\text{4x}}{{{\text{m}}^{\text{2}}}}}{\text{m}}\text{xdx}\]
\[\text{=2}\sqrt{\text{a}}\left[ \frac{{{\text{x}}^{\frac{\text{3}}{\text{2}}}}}{\frac{\text{3}}{\text{2}}} \right]_{\text{0}}^{\frac{\text{4 }\!\!\pi\!\!\text{ }}{{{\text{m}}^{\text{2}}}}}\text{-m}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}} \right]_{\text{0}}^{\frac{{{\text{4}}_{\text{n}}}}{{{\text{m}}^{\text{2}}}}}\]
Substituting the limits,
\[\text{=}\frac{\text{4}}{\text{3}}\sqrt{\text{a}}{{\left( \frac{\text{4a}}{{{\text{m}}^{\text{2}}}} \right)}^{\frac{\text{3}}{\text{2}}}}\text{-}\frac{\text{m}}{\text{2}}\left[ {{\left( \frac{\text{4a}}{{{\text{m}}^{\text{2}}}} \right)}^{\text{2}}} \right]\]
Simplifying,
\[\text{=}\frac{\text{32}{{\text{a}}^{\text{2}}}}{\text{3}{{\text{m}}^{\text{3}}}}\text{-}\frac{\text{m}}{\text{2}}\left( \frac{\text{16}{{\text{a}}^{\text{2}}}}{{{\text{m}}^{\text{2}}}} \right)\]
\[\text{=}\frac{\text{32}{{\text{a}}^{\text{2}}}}{\text{3}{{\text{m}}^{\text{3}}}}\text{-}\frac{\text{8}{{\text{a}}^{\text{2}}}}{{{\text{m}}^{\text{3}}}}\]
\[\text{=}\frac{\text{8}{{\text{a}}^{\text{2}}}}{\text{3}{{\text{m}}^{\text{3}}}}\text{ units}\]
7. Find the area enclosed by the parabola \[\text{4y=3}{{\text{x}}^{2}}\] and the line \[\text{2y=3x+12}\].
Ans: The given parabola and the line intersect at points \[\text{A}\left( \text{-2, 3} \right)\] and \[\text{A}\left( \text{4, 12} \right)\].
(Image Will be Updated Soon)
\[\text{AC}\] and \[BD\] are perpendicular to \[\text{x-axis}\].
Therefore, \[\text{AreaOBAO=AreaCDBA-(AreaODBO+AreaOACO)}\]
\[\text{=}\int_{\text{-2}}^{\text{1}}{\frac{\text{1}}{\text{2}}}\text{(3x+12)dx-}\int_{\text{-2}}^{\text{4}}{\frac{\text{3}{{\text{x}}^{\text{2}}}}{\text{4}}}\text{dx}\]
Integrating using the power rule,
\[\text{=}\frac{\text{1}}{\text{2}}\left[ \frac{\text{3}{{\text{x}}^{\text{2}}}}{\text{2}}\text{+12x} \right]_{\text{-2}}^{\text{4}}\text{-}\frac{\text{3}}{\text{4}}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{-2}}^{\text{4}}\]
Substituting the limits,
\[\text{=}\frac{\text{1}}{\text{2}}\text{ }\!\![\!\!\text{ 24+48-6+24 }\!\!]\!\!\text{ -}\frac{\text{1}}{\text{4}}\text{ }\!\![\!\!\text{ 64+8 }\!\!]\!\!\text{ }\]
Simplifying,
\[\text{=}\frac{\text{1}}{\text{2}}\text{ }\!\![\!\!\text{ 90 }\!\!]\!\!\text{ -}\frac{\text{1}}{\text{4}}\text{ }\!\![\!\!\text{ 72 }\!\!]\!\!\text{ }\]
\[\text{=45-18}\]
\[\text{=27units}\]
8. Find the area of the smaller region bounded by the ellipse \[\frac{{{\text{x}}^{\text{2}}}}{\text{9}}\text{+}\frac{{{\text{y}}^{\text{2}}}}{\text{4}}\text{=1}\] and the line \[\frac{\text{x}}{3}\text{+}\frac{\text{y}}{2}\text{=1}\].
Ans:
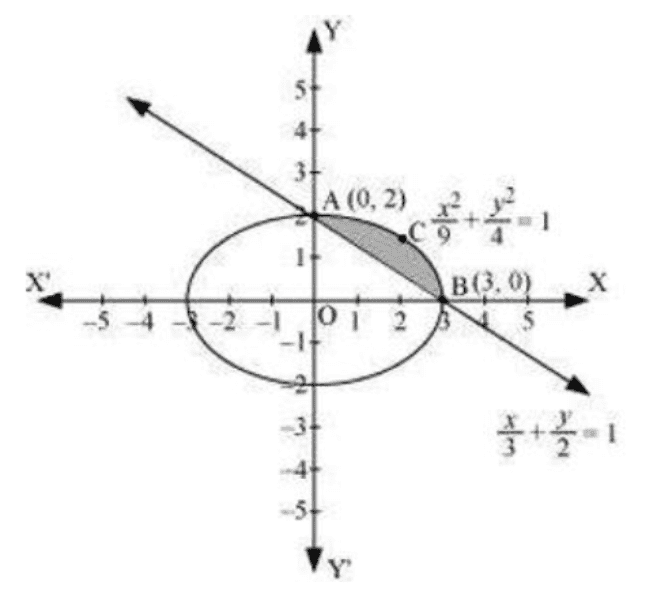
Area Bounded by Smaller Region of Ellipse
\[\text{Area BCAB= Area (OBCAO)- Area (OBAO) }\]
\[\text{=}\int_{\text{0}}^{\text{3}}{\text{2}}\sqrt{\text{1-}\frac{{{\text{x}}^{\text{2}}}}{\text{9}}}\text{dx-}\int_{\text{0}}^{\text{3}}{\text{2}}\left( \text{1-}\frac{\text{x}}{\text{3}} \right)\text{dx}\]
Simplifying,
\[\text{=}\frac{\text{2}}{\text{3}}\left[ \int_{\text{0}}^{\text{3}}{\sqrt{\text{9-}{{\text{x}}^{\text{2}}}}}\text{dx} \right]\text{-}\frac{\text{2}}{\text{3}}\int_{\text{0}}^{\text{3}}{\text{(3-1)}}\text{dx}\]
Substituting the limits,
\[\text{=}\frac{\text{2}}{\text{3}}\left[ \frac{\text{9}}{\text{2}}\left( \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}} \right) \right]\text{-}\frac{\text{2}}{\text{3}}\left[ \text{9-}\frac{\text{9}}{\text{2}} \right]\]
\[\text{=}\frac{\text{2}}{\text{3}}\left[ \frac{\text{9 }\!\!\pi\!\!\text{ }}{\text{4}}\text{-}\frac{\text{9}}{\text{2}} \right]\]
\[\text{=}\frac{\text{2}}{\text{3}}\text{ }\!\!\times\!\!\text{ }\frac{\text{9}}{\text{4}}\text{( }\!\!\pi\!\!\text{ -2)}\]
\[\text{=}\frac{\text{3}}{\text{2}}\text{( }\!\!\pi\!\!\text{ -2) units}\]
9. Find the area of the smaller region bounded by the ellipse \[\frac{{{\text{x}}^{\text{2}}}}{{{a}^{2}}}\text{+}\frac{{{\text{y}}^{\text{2}}}}{{{b}^{2}}}\text{=1}\] and the line \[\frac{\text{x}}{a}\text{+}\frac{\text{y}}{b}\text{=1}\].
Ans:

Area Bounded by Smaller Region of Ellipse
\[\text{AreaBCAB=Area(OBCAO)-Area(OBAO)}\]
\[\text{=}\frac{\text{b}}{\text{a}}\int_{\text{0}}^{\text{a}}{\sqrt{\text{1-}\frac{{{\text{x}}^{\text{2}}}}{{{\text{a}}^{\text{2}}}}}}\text{dx-}\frac{\text{b}}{\text{a}}\int_{\text{0}}^{\text{a}}{\text{(1-}\frac{\text{x}}{\text{a}}\text{)}}\text{dx}\]
\[\text{=}\frac{\text{b}}{\text{a}}\left[ \left\{ \frac{\text{x}}{\text{2}}\sqrt{{{\text{a}}^{\text{2}}}\text{-}{{\text{x}}^{\text{2}}}}\text{+}\frac{{{\text{a}}^{\text{2}}}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{x}}{\text{a}} \right\}_{\text{0}}^{\text{a}}\text{-}\left\{ \text{ax-}\frac{{{\text{x}}^{\text{2}}}}{\text{2}} \right\}_{\text{0}}^{\text{a}} \right]\]
Substituting the limits,
\[\text{=}\frac{\text{b}}{\text{a}}\left[ \left\{ \frac{{{\text{a}}^{\text{2}}}}{\text{2}}\left( \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}} \right) \right\}\text{-}\left\{ {{\text{a}}^{\text{2}}}\text{-}\frac{{{\text{a}}^{\text{2}}}}{\text{2}} \right\} \right]\]
Simplifying,
\[\text{=}\frac{\text{b}}{\text{a}}\left[ \frac{{{\text{a}}^{\text{2}}}\text{ }\!\!\pi\!\!\text{ }}{\text{4}}\text{-}\frac{{{\text{a}}^{\text{2}}}}{\text{2}} \right]\]
\[\text{=}\frac{\text{b}{{\text{a}}^{\text{2}}}}{\text{2a}}\left[ \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}}\text{-1} \right]\]
\[\text{=}\frac{\text{ab}}{\text{2}}\left[ \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}}\text{-1} \right]\]
\[\text{=}\frac{\text{ab}}{\text{4}}\text{( }\!\!\pi\!\!\text{ -2)}\]
10. Find the area of the region enclosed by the parabola \[{{\text{x}}^{\text{2}}}\text{ = y}\], the line \[\text{y = x + 2}\] and \[\text{x-axis}\].
Ans: Point of intersection of the given curves is \[\text{A}\left( \text{-1, 1} \right)\].
(Image Will be Updated Soon)
Therefore, \[\text{AreaOABCO=Area(BCA)+AreaCOAC}\]
\[\text{=}\int_{\text{-2}}^{\text{-1}}{\text{(x+2)}}\text{dx+}\int_{\text{-1}}^{\text{0}}{{{\text{x}}^{\text{2}}}}\text{dx}\]
Integrating using the power rule
\[\text{=}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+2x} \right]_{\text{-2}}^{\text{-1}}\text{+}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{-1}}^{\text{0}}\]
Substituting the limits,
\[\text{=}\left[ \frac{{{\text{(-1)}}^{\text{2}}}}{\text{2}}\text{+2(-1)-}\frac{{{\text{(-2)}}^{\text{2}}}}{\text{2}}\text{-2(-2)} \right]\text{+}\left[ \text{-}\frac{{{\text{(-1)}}^{\text{3}}}}{\text{3}} \right]\]
Simplifying,
\[\text{=}\left[ \frac{\text{1}}{\text{2}}\text{-2-2+4+}\frac{\text{1}}{\text{3}} \right]\]
\[\text{=}\frac{\text{5}}{\text{6}}\text{units}\]
11. Using the method of integration find the area bounded by the curve \[\left| \text{x} \right|\text{+}\left| \text{y} \right|\text{=1}\]. (Hint: the required region is bounded by the lines \[\text{x+y=1, x-y=1, -x+y=1}\] and \[\text{-x-y=1}\])
Ans: the given curves overlap at points \[\text{A(0, 1),B(1, 0),C(0, -1)}\] and \[\text{D}(-1,0)\].
(Image Will be Updated Soon)
As we can see in the diagram, the curve is symmetrical about \[\text{x-axis}\] and \[\text{y-axis}\].
Therefore, \[\text{AreaADCB=4 }\!\!\times\!\!\text{ AreaOBAO}\]
\[\text{=4}\int_{\text{0}}^{\text{1}}{\text{(1-x)}}\text{dx}\]
Integrating using the power rule,
\[\text{=4}\left( \text{x-}\frac{{{\text{x}}^{\text{2}}}}{\text{2}} \right)_{\text{0}}^{\text{1}}\]
Substituting the limits,
\[\text{=4}\left[ \text{1-}\frac{\text{1}}{\text{2}} \right]\]
Simplifying,
\[\text{=4}\left( \frac{\text{1}}{\text{2}} \right)\]
\[\text{=2units}\]
12. Find the area bounded by curves \[\left\{ \text{(x,y):y}\ge {{\text{x}}^{2}} \right.\text{and}\left. \text{y= }\!\!|\!\!\text{ x }\!\!|\!\!\text{ } \right\}\].
Ans:
(Image Will be Updated Soon)
As we can see in the diagram, the required area is symmetrical about \[\text{y-axis}\].
Therefore, Required area \[\text{=2 }\!\![\!\!\text{ Area(OCAO)-Area(OCADO) }\!\!]\!\!\text{ }\]
\[\text{=2}\left[ \int_{\text{0}}^{\text{1}}{\text{x}}\text{dx-}\int_{\text{0}}^{\text{1}}{{{\text{x}}^{\text{2}}}}\text{dx} \right]\]
Integrating using the power rule
\[\text{=2}\left[ \left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}} \right]_{\text{0}}^{\text{1}}\text{-}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{0}}^{\text{1}} \right]\]
Substituting the limits,
\[\text{=2}\left[ \frac{\text{1}}{\text{2}}\text{-}\frac{\text{1}}{\text{3}} \right]\]
Simplifying,
\[\text{=2}\left[ \frac{\text{1}}{\text{6}} \right]\]
\[\text{=}\frac{\text{1}}{\text{3}}\text{units}\]
13. Using the method of integration find the area of the triangle \[\text{ABC}\], coordinate of whose vertices are \[\text{A}\left( \text{2, 0} \right)\text{, B}\left( \text{4, 5} \right)\] and \[\text{C}\left( \text{6, 3} \right)\].
Ans:
(Image Will be Updated Soon)
Write the equation of \[\text{AB}\]:
\[\text{y-0=}\frac{\text{5-0}}{\text{4-2}}\text{(x-2)}\]
\[\text{2y=5x-10}\]
\[\text{y=}\frac{\text{5}}{\text{2}}\text{(x-2)}\]
Write the equation of \[\text{BC}\]:
\[\text{y-5=}\frac{\text{3-5}}{\text{6-4}}\text{(x-4)}\]
\[\text{2y-10= -2x+8}\]
\[\text{2y= -2x+18}\]
\[\text{y= -x+9}\]
Write the equation of \[\text{CA}\]:
\[\text{y-3=}\frac{\text{0-3}}{\text{2-6}}\text{(x-6)}\]
\[\text{-4y+12= -3x+18}\]
\[\text{4y=3x-6}\]
\[\text{y=}\frac{\text{3}}{\text{4}}\text{(x-2)}\]
\[\text{Area( }\!\!\Delta\!\!\text{ ABC)=Area(ABLA)+Area(BLMCB)-Area(ACMA)}\]\[\text{=}\int_{\text{2}}^{\text{4}}{\frac{\text{5}}{\text{2}}}\text{(x-2)dx+}\int_{\text{4}}^{\text{6}}{\text{(-x+9)}}\text{dx-}\int_{\text{2}}^{\text{6}}{\frac{\text{3}}{\text{4}}}\text{(x-2)dx}\]
Integrating using the power rule
\[\text{=}\frac{\text{5}}{\text{2}}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{-2x} \right]_{\text{2}}^{\text{4}}\text{+}\left[ \frac{\text{-}{{\text{x}}^{\text{2}}}}{\text{2}}\text{+9x} \right]_{\text{4}}^{\text{6}}\text{-}\frac{\text{3}}{\text{4}}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{-2x} \right]_{\text{2}}^{\text{6}}\]
Substituting the limits,
\[\text{=}\frac{\text{5}}{\text{2}}\text{ }\!\![\!\!\text{ 8-8-2+4 }\!\!]\!\!\text{ + }\!\![\!\!\text{ -18+54+8-36 }\!\!]\!\!\text{ -}\frac{\text{3}}{\text{4}}\text{ }\!\![\!\!\text{ 18-12-2+4 }\!\!]\!\!\text{ }\]
Simplifying,
\[\text{=5+8-}\frac{\text{3}}{\text{4}}\text{(8)}\]
\[\text{=13-6}\]
\[\text{=7unit}\]
14. Using the method of integration find the area of the region bounded by lines: \[\text{2x+y=4,3x-2y=6}\] and \[\text{x-3y+5=0}\].
Ans:
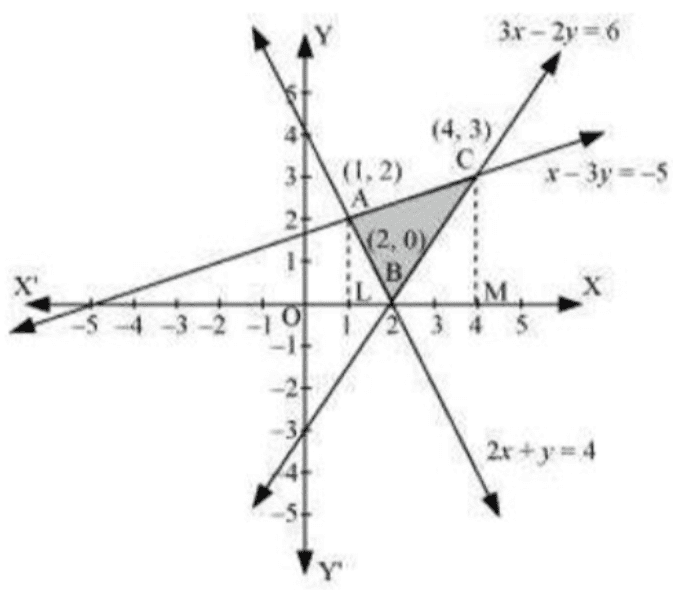
Area Bounded by Lines
As shown in the diagram, \[\text{AL}\] and \[\text{CM}\] are perpendicular on \[\text{x-axis}\].
\[\text{Area( }\!\!\Delta\!\!\text{ ABC)=Area(ALMCA)-Area(ALB)-Area(CMB)}\]
\[\text{=}\int_{\text{1}}^{\text{4}}{\left( \frac{\text{x+5}}{\text{3}}\text{dx} \right)}\text{-}\int_{\text{1}}^{\text{2}}{\text{(4-2x)}}\text{dx-}\int_{\text{2}}^{\text{4}}{\left( \frac{\text{3x-6}}{\text{2}} \right)}\text{dx}\]
Integrating using the power rule,
\[\text{=}\frac{\text{1}}{\text{3}}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+5x} \right]_{\text{1}}^{\text{4}}\text{-}\left[ \text{4x-}{{\text{x}}^{\text{2}}} \right]_{\text{1}}^{\text{2}}\text{-}\frac{\text{1}}{\text{2}}\left[ \frac{\text{3}{{\text{x}}^{\text{2}}}}{\text{2}}\text{-6x} \right]_{\text{2}}^{\text{4}}\]
Substituting the limits,
\[\text{=}\frac{\text{1}}{\text{3}}\left[ \text{8+20-}\frac{\text{1}}{\text{2}}\text{-5} \right]\text{- }\!\![\!\!\text{ 8-4-4+1 }\!\!]\!\!\text{ -}\frac{\text{1}}{\text{2}}\text{ }\!\![\!\!\text{ 24-24-6+12 }\!\!]\!\!\text{ }\]
Simplifying,
\[\text{=}\left( \frac{\text{1}}{\text{3}}\text{ }\!\!\times\!\!\text{ }\frac{\text{45}}{\text{2}} \right)\text{-(1)-}\frac{\text{1}}{\text{2}}\text{(6)}\]
\[\text{=}\frac{\text{15}}{\text{2}}\text{-1-3}\]
\[\text{=}\frac{\text{15}}{\text{2}}\text{-4}\]
\[\text{=}\frac{\text{15-8}}{\text{2}}\]
\[\text{=}\frac{\text{7}}{\text{2}}\text{units}\]
15. Find the area of the region \[\left\{ \left( \text{x,y} \right)\text{:}{{\text{y}}^{2}}\le 4x\text{,4}{{\text{x}}^{2}}\text{+4}{{\text{y}}^{2}}\le \text{9} \right\}\]
Ans:
(Image Will be Updated Soon)
As shown in the diagram, the points of intersection of the given curves are \[\left( \frac{\text{1}}{\text{2}}\text{,}\sqrt{\text{2}} \right)\] and \[\left( \frac{\text{1}}{\text{2}}\text{,-}\sqrt{\text{2}} \right)\].
The required area is \[\text{OABCO}\] and it is symmetrical about \[\text{x-axis}\].
Therefore, \[\text{Area OABCO=2 }\!\!\times\!\!\text{ Area OBC}\]
\[\text{Area OBCO= Area OMC + Area MBC}\]
\[\text{=}\int_{\text{0}}^{\frac{\text{1}}{\text{2}}}{\text{2}}\sqrt{\text{x}}\text{dx+}\int_{\frac{\text{1}}{\text{2}}}^{\frac{\text{3}}{\text{2}}}{\frac{\text{1}}{\text{2}}}\sqrt{\text{9-4}{{\text{x}}^{\text{2}}}}\text{dx}\]
\[\text{=}\int_{\text{0}}^{\frac{\text{1}}{\text{2}}}{\text{2}}\sqrt{\text{x}}\text{dx+}\int_{\frac{\text{1}}{\text{2}}}^{\frac{\text{3}}{\text{2}}}{\frac{\text{1}}{\text{2}}}\sqrt{{{\text{(3)}}^{\text{2}}}\text{-(2x}{{\text{)}}^{\text{2}}}}\text{dx}\]
16. Area bounded by the curve \[\text{y=}{{\text{x}}^{3}}\], the \[\text{x-axis}\] and the ordinates \[\text{x = -2}\] and \[\text{x = 1}\] is
A. \[\text{-9}\]
B. \[\text{-}\frac{\text{15}}{\text{4}}\]
C. \[\frac{\text{15}}{\text{4}}\]
D. \[\frac{\text{17}}{\text{4}}\]
Ans:
(Image Will be Updated Soon)
As shown in the diagram, the required area is:
\[\text{Required area =}\int_{\text{-2}}^{\text{1}}{\text{y}}\text{dx}\]
\[\text{=}\int_{\text{-2}}^{\text{1}}{{{\text{x}}^{\text{3}}}}\text{dx}\]
Integrating using the power rule
\[\text{=}\left[ \frac{{{\text{x}}^{\text{4}}}}{\text{4}} \right]_{\text{-2}}^{\text{1}}\]
Substituting the limits,
\[\text{=}\left[ \frac{\text{1}}{\text{4}}\text{-}\frac{{{\text{(-2)}}^{\text{4}}}}{\text{4}} \right]\]
Simplifying,
\[\text{=}\left( \frac{\text{1}}{\text{4}}\text{-4} \right)\]
\[\text{= -}\frac{\text{15}}{\text{4}}\text{ units}\]
So, the correct answer is \[\text{ -}\frac{\text{15}}{\text{4}}\text{ units}\] option B.
17. The area bounded by the curve \[\text{y=x}\left| \text{x} \right|\text{,x-axis}\] and the ordinate \[\text{x = 1}\] and \[\text{x = -1}\] is given by (Hint \[\text{y = }{{\text{x}}^{2}}\] if \[x>0\] and \[\text{y = -}{{\text{x}}^{2}}\] if \[x<0\])
A. \[0\]
B. \[\frac{\text{1}}{\text{3}}\]
C. \[\frac{2}{\text{3}}\]
D. \[\frac{4}{\text{3}}\]
Ans:
(Image Will be Updated Soon)
\[\text{Required area=}\int_{\text{-1}}^{\text{1}}{\text{y}}\text{dx}\]
\[\text{=}\int_{\text{-1}}^{\text{1}}{\text{x}}\text{ }\!\!|\!\!\text{ x }\!\!|\!\!\text{ dx}\]
\[\text{= -}\int_{\text{-1}}^{\text{0}}{{{\text{x}}^{\text{2}}}}\text{dx+}\int_{\text{0}}^{\text{1}}{{{\text{x}}^{\text{2}}}}\text{dx}\]
Integrating using the power rule
\[\text{= -}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{-1}}^{\text{0}}\text{+}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{0}}^{\text{1}}\]
Substituting the limits,
\[\text{= -}\left( \text{-}\frac{\text{1}}{\text{3}} \right)\text{+}\frac{\text{1}}{\text{3}}\]
\[\text{=}\frac{\text{2}}{\text{3}}\text{units}\]
So, the correct answer is \[\frac{\text{2}}{\text{3}}\text{units}\] option C.
18. The area of the circle \[{{\text{x}}^{\text{2}}}\text{+}{{\text{y}}^{\text{2}}}\text{=16}\] exterior to the parabola \[{{\text{y}}^{\text{2}}}\text{=6}\] is
A. \[\frac{\text{4}}{\text{3}}\text{(4 }\!\!\pi\!\!\text{ -}\sqrt{\text{3}}\text{)}\]
B. \[\frac{\text{4}}{\text{3}}\text{(4 }\!\!\pi\!\!\text{ +}\sqrt{\text{3}}\text{)}\]
C. \[\frac{\text{4}}{\text{3}}\text{(8 }\!\!\pi\!\!\text{ -}\sqrt{\text{3}}\text{)}\]
D. \[\frac{\text{4}}{\text{3}}\text{(8 }\!\!\pi\!\!\text{ +}\sqrt{\text{3}}\text{)}\]
Ans:
(Image Will be Updated Soon)
\[\text{Area=2 }\!\![\!\!\text{ Area(OADO)+Area(ADBA) }\!\!]\!\!\text{ }\]
\[\text{=2}\left[ \int_{\text{0}}^{\text{2}}{\sqrt{\text{16x}}}\text{dx+}\int_{\text{2}}^{\text{4}}{\sqrt{\text{16-}{{\text{x}}^{\text{2}}}}}\text{dx} \right]\]
\[\text{=2}\left[ \sqrt{\text{6}}\left\{ \frac{{{\text{x}}^{\frac{\text{3}}{\text{2}}}}}{\frac{\text{3}}{\text{2}}} \right\}_{\text{0}}^{\text{2}} \right]\text{+2}\left[ \frac{\text{x}}{\text{2}}\sqrt{\text{16-}{{\text{x}}^{\text{2}}}}\text{+}\frac{\text{16}}{\text{2}}\text{si}{{\text{n}}^{\text{-1}}}\frac{\text{x}}{\text{4}} \right]_{\text{2}}^{\text{4}}\]
\[\text{=2}\sqrt{\text{6}}\text{ }\!\!\times\!\!\text{ }\frac{\text{2}}{\text{3}}\left[ {{\text{x}}^{\frac{\text{3}}{\text{2}}}} \right]_{\text{0}}^{\text{2}}\text{+2}\left[ \text{8 }\!\!\times\!\!\text{ }\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}}\text{-}\sqrt{\text{16-4}}\text{-8si}{{\text{n}}^{\text{-1}}}\left( \frac{\text{1}}{\text{2}} \right) \right]\]
Substituting the limits,
\[\text{=}\frac{\text{4}\sqrt{\text{6}}}{\text{3}}\text{(2}\sqrt{\text{2}}\text{)+2}\left[ \text{4 }\!\!\pi\!\!\text{ -}\sqrt{\text{12}}\text{-8}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{6}} \right]\]
Simplifying,
\[\text{=}\frac{\text{16}\sqrt{\text{3}}}{\text{3}}\text{+8 }\!\!\pi\!\!\text{ -4}\sqrt{\text{3}}\text{-}\frac{\text{8}}{\text{3}}\text{ }\!\!\pi\!\!\text{ }\]
\[\text{=}\frac{\text{4}}{\text{3}}\text{ }\!\![\!\!\text{ 4}\sqrt{\text{3}}\text{+6 }\!\!\pi\!\!\text{ -3}\sqrt{\text{3}}\text{-2 }\!\!\pi\!\!\text{ }\!\!]\!\!\text{ }\]
\[\text{=}\frac{\text{4}}{\text{3}}\text{ }\!\![\!\!\text{ }\sqrt{\text{3}}\text{+4 }\!\!\pi\!\!\text{ }\!\!]\!\!\text{ }\]
\[\text{=}\frac{\text{4}}{\text{3}}\text{ }\!\![\!\!\text{ 4 }\!\!\pi\!\!\text{ +}\sqrt{\text{3}}\text{ }\!\!]\!\!\text{ units}\]
Area of circle \[\text{= }\!\!\pi\!\!\text{ (r}{{\text{)}}^{\text{2}}}\]
\[\text{= }\!\!\pi\!\!\text{ (4}{{\text{)}}^{\text{2}}}\]
\[\text{=16 }\!\!\pi\!\!\text{ units}\]
Therefore, \[\text{Required area = 16 }\!\!\pi\!\!\text{ -}\frac{\text{4}}{\text{3}}\text{ }\!\![\!\!\text{ 4 }\!\!\pi\!\!\text{ +}\sqrt{\text{3}}\text{ }\!\!]\!\!\text{ }\]
\[\text{=}\frac{\text{4}}{\text{3}}\text{ }\!\![\!\!\text{ 4 }\!\!\times\!\!\text{ 3 }\!\!\pi\!\!\text{ -4 }\!\!\pi\!\!\text{ -}\sqrt{\text{3}}\text{ }\!\!]\!\!\text{ }\]
\[\text{=}\frac{\text{4}}{\text{3}}\text{(8 }\!\!\pi\!\!\text{ -}\sqrt{\text{3}}\text{) units}\]
Therefore, the correct answer is \[\frac{\text{4}}{\text{3}}\text{(8 }\!\!\pi\!\!\text{ -}\sqrt{\text{3}}\text{) units}\] option C.
19. The area bounded by the \[\text{y-axis, y = cosx}\] and \[\text{y = sinx}\] when \[\text{0}\le \text{x}\le \frac{\text{ }\!\!\pi\!\!\text{ }}{\text{2}}\] is
A. \[\text{2}\left( \sqrt{\text{2}}\text{-1} \right)\]
B. \[\sqrt{\text{2}}\text{-1}\]
C. \[\sqrt{\text{2}}\text{+1}\]
D. \[\sqrt{\text{2}}\]
Ans:
(Image Will be Updated Soon)
Required area \[\text{=Area(ABLA)+area(OBLO)}\]
\[\text{=}\int_{\frac{\text{1}}{\sqrt{\text{2}}}}^{\text{1}}{\text{x}}\text{dy+}\int_{\text{0}}^{\frac{\text{1}}{\sqrt{\text{2}}}}{\text{x}}\text{dy}\]
\[\text{=}\int_{\frac{\text{1}}{\sqrt{\text{2}}}}^{\text{1}}{\text{co}{{\text{s}}^{\text{-1}}}}\text{ydy+}\int_{\frac{\text{1}}{\sqrt{\text{2}}}}^{\text{1}}{\text{si}{{\text{n}}^{\text{-1}}}}\text{xdy}\]
\[\text{=}\left[ \text{yco}{{\text{s}}^{\text{-1}}}\text{y-}\sqrt{\text{1-}{{\text{y}}^{\text{2}}}} \right]_{\frac{\text{1}}{\sqrt{\text{2}}}}^{\text{1}}\text{+}\left[ \text{xsi}{{\text{n}}^{\text{-1}}}\text{x+}\sqrt{\text{1-}{{\text{x}}^{\text{2}}}} \right]_{\text{0}}^{\frac{\text{1}}{\sqrt{\text{2}}}}\]
Substituting the limits,
\[\text{=}\left[ \text{co}{{\text{s}}^{\text{-1}}}\text{(1)-}\frac{\text{1}}{\sqrt{\text{2}}}\text{co}{{\text{s}}^{\text{-1}}}\left( \frac{\text{1}}{\sqrt{\text{2}}} \right)\text{+}\sqrt{\text{1-}\frac{\text{1}}{\text{2}}} \right]\text{+}\left[ \frac{\text{1}}{\sqrt{\text{2}}}\text{si}{{\text{n}}^{\text{-1}}}\left( \frac{\text{1}}{\sqrt{\text{2}}} \right)\text{+}\sqrt{\text{1-}\frac{\text{1}}{\text{2}}}\text{-1} \right]\]
Simplifying,
\[\text{=}\frac{\text{- }\!\!\pi\!\!\text{ }}{\text{4}\sqrt{\text{2}}}\text{+}\frac{\text{1}}{\sqrt{\text{2}}}\text{+}\frac{\text{ }\!\!\pi\!\!\text{ }}{\text{4}\sqrt{\text{2}}}\text{+}\frac{\text{1}}{\sqrt{\text{2}}}\text{-1}\]
\[\text{=}\frac{\text{2}}{\sqrt{\text{2}}}\text{-1}\]
\[\text{=}\sqrt{\text{2}}\text{-1units}\]
Therefore, the correct answer is \[\sqrt{\text{2}}\text{-1units}\] option B.
NCERT Solutions for Class 12 Maths Chapter 8 - Application of Integrals
NCERT Solutions for Class 12 Maths – Free PDF Download
Having easy access to Maths solutions can give the required boost needed by students in today’s hectic lifestyle. With the readily available NCERT Solutions Class 12 Maths Chapter 8 PDF download at our website, students can revise their syllabus on the go.
Chapter 8 – Application of Integrals
NCERT Solutions for Class 12 Maths Chapter 8
8.1 Introduction
In the introduction of Chapter 8 Class 12th Maths, you will be reminded of all the geometrical formulae you learned in the previous chapters. You would recall how these geometric formulae for calculating areas of triangles, rectangles, trapeze, etc. are the basis on which mathematics is applied to real-life problems. However, they are not able to determine areas enclosed by curves. That is where integral calculus comes into the picture.
You will rekindle what you learned about areas bounded by a curve in the previous chapter where definite integrals were calculated as the limit of a sum. In this chapter, you would use those concepts to find out areas between lines and arcs of circles, parabolas, simple curves and ellipses.
AOI Class 12 NCERT Solutions have many solved examples and a variety of questions neatly divided into different exercises. You will get rigorous practice with the set of questions presented in this chapter. Some of the prominent parts discussed here are:
Elementary area i.e. the area located between any arbitrary positions
The area under two curves
The area that is bounded by a curve and a line
Integrals of trigonometric identities
8.2 Area Under Simple Curves
This section of Chapter 8 Class 12 Maths begins with a recap of how definite integrals are calculated as the limit of a sum and the fundamental theorem of calculus. You will then learn how to find the area bounded by the curves. So, if the curve is y = f(x) then to find the area bounded by the curve, the x-axis, and 2 points x=p1 and x=p2 on the x-axis you can consider it as a large number of vertical strips. After this, you would understand how to calculate individual areas of these thin strips. Suppose the height of the strip is y and width is dx then the area of one strip is expressed as dA = ydx, here y = f(x). This area is termed as an elementary strip. The total area can thus be calculated by integrating these elementary strips:
A = \[\int_{p1}^{p2}\] dA = \[\int_{p1}^{p2}\] ydx = \[\int_{p1}^{p2}\] f(x) dx
In case the position of the curve is below the x-axis, the area will come out to be negative, but we take only the absolute value of the integral.
You may learn how to calculate the area of a region which is bounded by a curve and a line. One could consider either vertical or horizontal strips to calculate the area of the region. So if the curve is y = f(x) and the equation of the line is g = ax + k, we can see there are 2 cases; one is the area under the curve, and the other is the area between 2 curves, depending on how many points the line intersects the curve at. For a line intersecting the curve at 2 points, a general formula to find the region between the line and the curve can be given as:
A = \[\int_{p1}^{p2}\] [g(x) - f(x)]dx. Here g(x) is the equation of the line, f(x) is the equation of the curve, and p1, p2 are points of intersection of the curve with the straight line.
Exercise 8.1 Solutions: 13 Questions (11 short questions, 2 MCQs)
8.2 The Area Between Two Curves
In this unit of Application of Integrals Class 12 chapter, you would learn how to calculate area between 2 curves by dividing the common region into elementary areas of vertical strips. So if equation of one curve is y = f(x) and equation of the other curve is y = g(x) and we know that f(x) >= g(x), the elementary area can be given as: dA = [f(x) – g(x)]dx where dx is the width of the strip. The total area is thus calculated by the following integral:
A = \[\int_{p1}^{p2}\] [f(x) - g(x)]dx. Here p1 and p2 are the 2 points of intersections of the curve on the x-axis.
Exercise 8.2 Solutions: 7 Questions (5 Short Questions, 2 MCQs)
Important Questions from Application of Integrals (Short, Long & Practice)
Short Answer Type Questions
1. The smaller area enclosed by the circle x2+ y2 = 4 and the line x + y = 2 is____.
2. If the area cut off from a parabola by any double ordinate is k times the corresponding rectangle contained by that double ordinate and its distance from the vertex, then k is equal to______.
3. The area of the region bounded by the parabola ( y – 2)2 = x – 1, the tangent to the parabola at the point ( 2 , 3 ) and the x – axis is equal to_____.
Long Answer Type Questions
1. Find the area bounded by the parabola y2 = 4ax, latus rectum and the x-axis.
2. Find the area of the region bounded by y =√a and y = a.
3. Find the area of the curve y = sin x between 0 and π.
4. Find the area enclosed between the curve y = x3 and the line y = x.
Practice Questions
1.Find the area of the region bounded by y2= 9x, x =2, x = 4 and the x – axis in the first quadrant.
2. Find the area of the region bounded x2 = 4y, y = 2, y = 4 and the y – axis in the first quadrant.
3. The area between x = y2 and x = 4 is divided into equal parts by the line x = a, find the value of a.
Key Features of Revision Notes for CBSE Class 12 Maths - Application of Integrals
Solutions are curated in a straightforward and well-explained manner to help students in quickly finding solutions.
The PDFs contain detailed solutions for all questions from NCERT that are simple to grasp and learn.
The NCERT Solutions have been prepared by subject experts to match the curriculum.
The solutions will help in developing a strong conceptual foundation for students, which is important in the final stages of preparation for board and competitive exams.
Students can easily prepare all of the concepts covered in their respective classes more efficiently and crack the most difficult competitive exams.
These notes are absolutely free and available in a PDF format.
Access other Chapters of NCERT Solutions for Class 12 Maths
Chapter 8 - Application of Integrals
Conclusion
NCERT Solutions for CBSE Class 12 Maths Chapter 8 Application of Integrals are now available in PDF format on Vedantu. Students can make use of these solutions for Application of Integrals to prepare for both board and competitive exams. All solutions are accurate as they are prepared by master teachers. Along with the solutions we have provided important questions from an examination point of view.





















FAQs on NCERT Solutions for Class 12 Maths Chapter 8 - Application Of Integrals
1. Which chapters are removed from maths Class 12?
According to the revised CBSE syllabus for Class 12 Maths, the syllabus has been reduced by 30%. Full chapters have not been deleted but certain portions from each unit have been removed from the CBSE Class 12 Maths syllabus. To get full NCERT Solutions Maths Chapter 8 based on the latest syllabus, download the NCERT Solutions Maths Chapter 8 PDF available only on Vedantu. Extra important questions are also solved for a better understanding.
2. How many important examples are there in Class 12 Maths Chapter 8 Miscellaneous Exercise?
There are five examples (from Example 11 to Example 15) given in the Miscellaneous Examples section in Class 12 Maths Chapter 8. These miscellaneous examples can be used to solve the Class 12 Maths Chapter 8 Miscellaneous Exercise given in the NCERT textbook. For fully solved exercise questions including the miscellaneous exercise present in Chapter 8, download the NCERT Solutions Class 12 Maths Chapter 8 PDF from Vedantu today and start practising!
3. How can I understand the chapters in Class 12 Maths?
NCERT Class 12 Maths can be intimidating at first, but if you strategize your study, you can overcome your fear of Class 12 Maths easily. The best way to understand all the chapters in Class 12 Maths is to refer to NCERT Solutions Class 12 Maths on Vedantu. All the solutions in Class 12 Maths on Vedantu have full explanations for each topic and sub-topics including exercise questions.
4. What is the underlying concept of Chapter 8 Application of Integrals?
The underlying concept of NCERT Class 12 Maths Chapter 8 Application of Integrals deals with the usage of geometric formulae for calculating the area of different geometric shapes and the area between two curves, area between curve and line, area between arbitrary positions, and trigonometric identities integrals. To get solutions to all exercises, you must refer to NCERT Class 12 Maths Chapter 8 on Vedantu.
5. What are the most important formulas that you need to learn in Chapter 8 Class 12 Maths?
The most important formulae that you need to learn in Class 12 Maths Chapter 8 are - formula for the area under the simple curve (area enclosed by the given circle, area enclosed within ellipse), the formula for the area of the region bounded by line and curve, and formula for the area between two curves. You can get access to all the solutions if you download the NCERT Solutions Class 12 Maths Chapter 8 on Vedantu at free of cost. These solutions are also available on the Vedantu app.



