Important Questions for CBSE Class 12 Maths Chapter 9 - Differential Equations 2024-25

Very Short Answer Type Questions (1 Mark)

1. Write the order and degree of the following differential equations.

(1) $ \dfrac{{dy}}{{dx}} + \cos y = 0.$ 

Ans: $\dfrac{{dy}}{{dx}} + \cos y = 0$

${y^\prime } + \cos x = 0$

Highest order of derivative $ = 1$

$\therefore $ Order $ = {\mathbf{1}}$

Degree = Power of ${y^\prime }$

Degree $ = 1$

(ii)$ {(\dfrac{{dy}}{{dx}})^2} + 3\dfrac{{{d^2}y}}{{d{x^2}}} = 4$ 

Ans:

$ {(\dfrac{{dy}}{{dx}})^2} + 3\dfrac{{{d^2}y}}{{d{x^2}}} = 4$ 

Highest order of derivative = 2

Order = 2

Degree = Power of y

Degree = 1

(iii)  $ \dfrac{{{\partial ^4}y}}{{\partial {x^4}}} + \sin x = {(\dfrac{{{\partial ^2}y}}{{\partial {x^2}}})^5}.$ 

Ans:

$ \dfrac{{{\partial ^4}y}}{{\partial {x^4}}} + \sin x = {(\dfrac{{{\partial ^2}y}}{{\partial {x^2}}})^5}$ 

Highest order of derivative = 4

Order = 4

Degree = Power of y

Degree = 1

(iv) $ \dfrac{{{\partial ^5}y}}{{\partial {x^5}}} + \log (\dfrac{{dy}}{{dx}}) = 0$ 

Ans:

$ \dfrac{{{\partial ^5}y}}{{\partial {x^5}}} + \log (\dfrac{{dy}}{{dx}}) = 0$ 

Highest order of derivative = 5

Order = 5

Degree = Power of y

Degree = not defined

(v)$ \sqrt {1 + \dfrac{{dy}}{{dx}}}  = {(\dfrac{{{\partial ^2}y}}{{\partial {x^2}}})^{\dfrac{1}{3}}}$ 

Ans:

$ \sqrt {1 + \dfrac{{dy}}{{dx}}}  = {(\dfrac{{{\partial ^2}y}}{{\partial {x^2}}})^{\dfrac{1}{3}}}$ 

Highest order of derivative = 2

Order = 2

Degree = Power of y

Degree = 2

(vi)$ {[1 + {(\dfrac{{dy}}{{dx}})^2}]^{\dfrac{3}{2}}} = k\dfrac{{{\partial ^2}y}}{{\partial {x^2}}}$ 

Ans:

$ {[1 + {(\dfrac{{dy}}{{dx}})^2}]^{\dfrac{3}{2}}} = k\dfrac{{{\partial ^2}y}}{{\partial {x^2}}}$ 

Squaring on both sides

$ {[1 + {(\dfrac{{dy}}{{dx}})^2}]^3} = (k\dfrac{{{\partial ^2}y}}{{\partial {x^2}}})2$ 

Highest order of derivative = 2

Order = 2

Degree = Power of y

Degree = 2

(vii)$ {(\dfrac{{{\partial ^3}y}}{{\partial {x^3}}})^2} + {(\dfrac{{{\partial ^2}y}}{{\partial {x^2}}})^3} = \sin x$ 

Ans:

$ {(\dfrac{{{\partial ^3}y}}{{\partial {x^3}}})^2} + {(\dfrac{{{\partial ^2}y}}{{\partial {x^2}}})^3} = \sin x$ 

Highest order of derivative = 3

Order = 3

Degree = Power of y

Degree = 2

(viii) $ \dfrac{{dy}}{{dx}} + \tan (\dfrac{{dy}}{{dx}}) = 0$ 

Ans:

$ \dfrac{{dy}}{{dx}} + \tan (\dfrac{{dy}}{{dx}}) = 0$ 

Highest order of derivative = 1

Order = 1

Degree = Power of y

Degree = Not defined

2 Write the general solution of following differential equations

(i)$ \dfrac{{dy}}{{dx}} = {x^5} + {x^2} - \dfrac{2}{x}$ 

Ans:

$ \dfrac{{dy}}{{dx}} = {x^5} + {x^2} - \dfrac{2}{x}$ 

Integrating on both side 

$ \int {\dfrac{{dy}}{{dx}}dx = \int {{x^5}dx + \int {{x^2}dx - \int {\dfrac{2}{x}} dx} } } $ 

$ y = \dfrac{{{x^6}}}{6} + \dfrac{{{x^3}}}{3} - 2\log |x| + c$ 

(ii) $ ({e^x} + {e^{ - x}})dy = ({e^x} - {e^{ - x}})dx$ 

Ans:

  ${\left( {{e^x} + {e^{ - x}}} \right)dy - \left( {{e^x} - {e^{ - x}}} \right)dx = 0}$

  ${\left( {{e^x} + {e^{ - x}}} \right)dy - \left( {{e^x} - {e^{ - x}}} \right)dx = 0}$

  ${\left( {{e^x} + {e^{ - x}}} \right)dy = \left( {{e^x} - {e^{ - x}}} \right)dx}$ 

  ${\dfrac{{dy}}{{dx}} = \dfrac{{{e^x} - {e^{ - x}}}}{{{e^x} + {e^{ - x}}}}{\text{dx}}}$

${dy = \dfrac{{{e^x} - {e^{ - x}}}}{{{e^x} + {e^{ - x}}}}{\text{dx}}} $

Integrating both sides.

$\int d y = \int {\dfrac{{{e^x} - {e^{ - x}}}}{{{e^x} + {e^{ - x}}}}} {\text{dx}}$

 $ {y = \int {\dfrac{{{e^x} - {e^{ - x}}}}{{{e^x} + {e^{ - x}}}}} {\text{dx}}}$

  ${{\text{ t}} = {e^x} + {e^{ - x}}} $

 $ {\dfrac{{dt}}{{dx}} = \left( {{e^x} - {e^{ - x}}} \right)}$

 $ {{\text{dx}} = \dfrac{{dt}}{{{e^x} - {e^{ - x}}}}}$ 

Putting value of ${\text{t}}$ and ${\text{dt}}$ in (1)

$\int d y = \int {\dfrac{{{e^x} - {e^{ - x}}}}{t}} \dfrac{{dt}}{{{e^x} - {e^{ - x}}}}.$

  ${\int d y = \int {\dfrac{{dt}}{t}} } $  

  ${y = \log |t| + c}$ 

Putting back $t = {e^x} - {e^{ - x}}$

$y = \log \left( {{e^x} - {e^{ - x}}} \right) + C$

(iii) $ \dfrac{{dy}}{{dx}} = {x^3} + {e^x} + {x^e}$ 

Ans:

$ \dfrac{{dy}}{{dx}} = {x^3} + {e^x} + {x^e}$ 

$ dy = ({x^3} + {e^x} + {x^e})dx$ 

Integrating on both sides

$ \int {dy}  = \int {({x^3} + {e^x} + {x^e})dx} $ 

$ y = \dfrac{{{x^4}}}{4} + {e^x} + \int {{x^e}dx} $ 

$ y = \dfrac{{{x^4}}}{4} + {e^x} + \dfrac{{{x^{e + 1}}}}{{e + 1}} + c$ 

(iv)$ \dfrac{{dy}}{{dx}} = {5^{x + y}}$ 

Ans:

$ \dfrac{{dy}}{{dx}} = {5^{x + y}}$ 

$ \dfrac{{dy}}{{dx}} = {5^x}{.5^y}$ 

$ \dfrac{1}{{{5}^{y}}}dy={{5}^{x}}dx$ 

Integrating on Both sides

$\int {\dfrac{1}{{{5^y}}}dy = \int {{5^x}dx} }$

${5^{ - y}} = {5^x} + c$

${5^x} + {5^{ - y}} = c$

(v) $ \dfrac{{dy}}{{dx}} = \dfrac{{1 - \cos 2x}}{{1 + \cos 2y}}$ 

Ans:

$ \dfrac{{dy}}{{dx}} = \dfrac{{1 - \cos 2x}}{{1 + \cos 2y}}$ 

We know that

$\cos 2x = 2{\cos ^2}x - 1$

Putting $x = \dfrac{x}{2}$

  ${\cos \dfrac{{2x}}{2} = 2{{\cos }^2}\dfrac{x}{2} - 1} $  

  ${\cos x = 2{{\cos }^2}\dfrac{x}{2} - 1} $  

  ${1 + \cos x = 2{{\cos }^2}\dfrac{x}{2}}$ 

We know

$\cos 2x = 1 - 2{\sin ^2}x$

Putting $x = \dfrac{x}{2}$

 ${{{\cos }^2}\dfrac{{2x}}{2} = 1 - 2{{\sin }^2}\dfrac{x}{2}} $  

 $ {\cos x = 1 - 2{{\sin }^2}\dfrac{x}{2}} $  

 $ {1 - \cos x = 2{{\sin }^2}\dfrac{x}{2}} $

 $ \dfrac{dy}{dx}=\dfrac{1-(1-2{{\sin }^{2}}x)}{1+1-2{{\sin }^{2}}y} $ 

 $ \dfrac{dy}{dx}=\dfrac{{{\sin }^{2}}x}{(1-{{\sin }^{2}}y)} $ 

 $ (1-{{\sin }^{2}}y)dy={{\sin }^{2}}xdx $

Integrating on both sides

$  \int {(1 - {{\sin }^2}y)dy = \int {{{\sin }^2}xdx} }   $ 

$ y - \dfrac{y}{2} + \dfrac{{\sin 2y}}{4} = \dfrac{x}{2} - \dfrac{{\sin 2x}}{4} + c  $ 

$ \dfrac{y}{2} + \dfrac{{\sin 2y}}{4} = \dfrac{x}{2} - \dfrac{{\sin 2x}}{4} + c  $  

By equating 

$ 2y + \sin 2y = 2x - \sin 2y + c$ 

$ 2y-2x+\sin 2y-\sin 2x=c2(y-x)+\sin 2y-\sin 2x=c$ 

(vi)$ \dfrac{{dy}}{{dx}} = \dfrac{{1 - 2y}}{{3x + 1}}$ 

Ans:

$ \dfrac{{dy}}{{dx}} = \dfrac{{1 - 2y}}{{3x + 1}}$ 

$ 2y + \sin 2y = 2x - \sin 2y + c$ 

$ 2y-2x+\sin 2y-\sin 2x=c2(y-x)+\sin 2y-\sin 2x=c$ 

Integrating on both sides

$ \int {(\dfrac{1}{{1 - 2y}})dy}  = \int {(\dfrac{1}{{3x + 1}})dx} $ 

$  -\dfrac{1}{2}\log (1-2y)=\dfrac{1}{3}\log (3x+1) $ 

$  2\log (3x+1)+3\log (1-2y)=c $

3. Write integrating factor of the following differential equations.

(I) $ \dfrac{{dy}}{{dx}} + y = \cos x - \sin x$ 

Ans:

$ \dfrac{{dy}}{{dx}} + y = \cos x - \sin x$ 

$dy/dx + y = \cos x - \sin x$ is a linear differential equation of the type $\dfrac{{dy}}{{dx}} + py = Q$

Here  $I.F. = {e^{\int 1 .dx}} = {e^x}$

Its solution is given by $ \Rightarrow \quad y{e^x} = \int {{e^x}} (\cos x - \sin x)dx$

$ \Rightarrow \quad y{e^x} = \int {{e^x}} \cos xdx - \int {{e^x}} \sin xdx$

Integrate by parts $ \Rightarrow \quad y{e^x} = {e^x}\cos x - \int  -  \sin x{e^x}dx - \int {{e^x}} \sin dx$

$\therefore \quad y{e^x} = {e^x}\cos x + C$

$ \Rightarrow \quad y = \cos x + C{e^{ - x}}$

(II) $ \dfrac{{dy}}{{dx}} + y{\sec ^2}x = \sec x + \tan x$ 

Ans:

$ \dfrac{{dy}}{{dx}} + y{\sec ^2}x = \sec x + \tan x$ 

$\dfrac{{dy}}{{dx}} + Py = Q$.

$\int {{e^{\tan x}}} \left( {\dfrac{{dy}}{{dx}} + y{{\sec }^\prime }x} \right)dx = \int {{e^{\tan x}}}  \cdot \tan x\sec x$

$y{e^{\tan x}} = \int {{e^{\tan x}}} \left( {\tan x{{\sec }^1}xdx} \right)$

$I = \int {{e^{\tan x}}} \tan x{\sec ^1}x \cdot dx$

$t = \tan x$

$dt = {\sec ^2}udn$

$\int_{\dfrac{1}{I}} {{e^t}} tdt$

$ \Rightarrow \int {{e^t}} t - \int {{e^t}} dt$

$\operatorname{tar} x{e^t}\tan x - {e^t} - {e^{\tan x}} + c$

$y{e^{\tan x}} = \tan x \cdot {e^{\tan x}} - {e^{\tan x}} + C$

(III) $x\dfrac{{dy}}{{dx}} + y\log x = x + y$

Ans:

 $ {\dfrac{{dy}}{{dx}} = \dfrac{y}{x}\left[ {\log \dfrac{y}{x} + 1} \right]} $  

 ${{\text{ }}y = {\text{V}}x} $  

 $  {\int {\dfrac{{d\;{\text{V}}}}{{\;{\text{V}}\log {\text{V}}}}}  = \int {\dfrac{{dx}}{x}} } $  

 $ {\log {\text{V}} = xc} $

 $ {\dfrac{y}{x} = {e^{cx}} \Rightarrow y = x{e^{cx}}}$ 

(IV) $x\dfrac{{dy}}{{dx}} - 3y = {x^2}$

Ans:

$x\dfrac{{dy}}{{dx}} - 3y = {x^2}$

 ${x\dfrac{{dy}}{{dx}} + 3y={x^2}}$  

 ${\dfrac{{dy}}{{dx}}+\dfrac{3}{x} \cdot y = x}$ 

$y.I.F=\int{I}.F\cdot q(x)\cdot dx$

$p(x)=\frac{3}{x}\text{}q(x)=x\text{ }~\text{ }$ 

$I.{{F}_{.}}={{e}^{\int{\frac{3}{x}}\text{}~\text{}\cdot dx}}\text{ }~\text{ }$ 

$={{e}^{3\ln x}}\text{}\!\!~\!\!\text{ }$ 

$={{(e)}^{\ln {{x}^{3}}}}\text{}~\text{ }$

$={{x}^{3}}\text{}\!\!~\!\!\text{ }$ 

 ${y \cdot {x^3} = \int{{x^3}}  \cdot x \cdot dx}$  

 ${y \cdot {x^3} =\dfrac{{{x^5}}}{5} + c}$  

 $y=\frac{{{x}^{2}}}{5}+\frac{c}{{{x}^{3}}}$

(V) $ \dfrac{{dy}}{{dx}} + y\tan x = \sec x$ 

Ans:

We have, $\dfrac{{dy}}{{dx}} + y\tan x = \sec x$

which is a linear differential equation Here, $P = \tan x,Q = \sec x$,

$\therefore $ I.F. $ = {e^{\int {\tan } xdx}} = {e^{\log \sec x}} = \sec x$

$\therefore $ The general solution is

$y\sec x = \int {\sec } x \cdot \sec x + C$

$ \Rightarrow \quad y\sec x = \int {{{\sec }^2}} xdx + C$

$ \Rightarrow \quad y\sec x = \tan x + C$

4. Write order of the differential equation of the family of following curves

(I)$ y{\text{ }} = {\text{ }}A{e^x}{\text{ }} + {\text{ }}B{e^{x + c}}$ 

Ans:

$ y{\text{ }} = {\text{ }}A{e^x}{\text{ }} + {\text{ }}B{e^{x + c}}$ 

$ y={{e}^{x}}(A+B{{e}^{c}})={{\operatorname{Re}}^{x}}\text{ }\!\!~\!\!\text{  }\!\!~\!\!\text{ }$ 

$ \dfrac{dy}{dx}\text{ }=\text{ }A{{e}^{x}}\text{ }+\text{ }B{{e}^{x+c}}\text{ }\!\!~\!\!\text{ }\dfrac{dy}{dx}=y$ 

Therefore, order is 1.

(II)$ Ay{\text{ }} = {\text{ }}B{x^2}$ 

Ans:

$ Ay{\text{ }} = {\text{ }}B{x^2}$ 

Differentiating wrt x

 $  A\dfrac{dy}{dx}=B $ 

 $  (2x)\dfrac{dy}{dx}=\dfrac{2Bx}{A} $ 

Therefore, order is 1.

(III)$ {\left( {x{\text{ }}-{\text{ }}a} \right)^2}{\text{ }} + {\text{ }}{\left( {y{\text{ }}-{\text{ }}b} \right)^2}{\text{ }} = {\text{ }}9$ 

Ans:

$ {\left( {x{\text{ }}-{\text{ }}a} \right)^2}{\text{ }} + {\text{ }}{\left( {y{\text{ }}-{\text{ }}b} \right)^2}{\text{ }} = {\text{ }}9$ 

Differentiate w.r.t x

$ \dfrac{d}{{dx}}{\left( {x{\text{ }}-{\text{ }}a} \right)^2}{\text{ }} + {\text{ }}\dfrac{d}{{dx}}{\left( {y{\text{ }}-{\text{ }}b} \right)^2}{\text{ }} = {\text{ }}9$ 

$ 2(x-a)\dfrac{d}{dx}(x-a)+2(y-b)\dfrac{d}{dx}(y-b)=0$ 

$ 2(x-a)(\dfrac{dy}{dx}-0)+2(y-b)(\dfrac{dy}{dx}-0)=0$ 

$ (x-a)+(y-b)\dfrac{dy}{dx}=0$ 

$ (x-a)=\text{ }\!\!~\!\!\text{ }-(y-b)\dfrac{dy}{dx}\text{ }\!\!~\!\!\text{ }$ 

$ \dfrac{{dy}}{{dx}} = \dfrac{{x - a}}{{ - (y - b)}}$ 

$  \dfrac{d}{dx}(x-a)=\dfrac{d}{dx}[(b-y)\dfrac{dy}{dx}] $ 

$  \dfrac{dx}{dx}-\dfrac{d}{dx}(a)=(b-y)\dfrac{{{d}^{2}}y}{d{{x}^{2}}}+\dfrac{dy}{dx} $ 

$  [\dfrac{d}{dx}(b-y)]1-0=(b-y)\dfrac{{{d}^{2}}y}{d{{x}^{2}}}+\dfrac{dy}{dx}(-\dfrac{dy}{dx}) $ 

$  1=(b-y)\dfrac{{{d}^{2}}y}{d{{x}^{2}}}-{{(\dfrac{dy}{dx})}^{2}} $

Hence, the order is 2.

(IV)$ Ax{\text{ }} + {\text{ }}B{y^2}{\text{ }} = {\text{ }}B{x^2}{\text{ }}-{\text{ }}Ay$ 

Ans:

$ Ax{\text{ }} + {\text{ }}B{y^2}{\text{ }} = {\text{ }}B{x^2}{\text{ }}-{\text{ }}Ay$ 

$Ax+B{{y}^{2}}=B{{x}^{2}}-Ay$

$Ax+Ay=B{{x}^{2}}-B{{y}^{2}}$

$A(x+y)=B\left( {{x}^{2}}-{{y}^{2}} \right)$

$A(x+y)=B(x+y)(x-y)$

$A=B(x-y)\text{ }\!\!~\!\!\text{ }$

$  \dfrac{A}{B}=x-y $ 

$  \dfrac{A}{B}+y=x $ 

Differentiate w.r.t x

$ \dfrac{{dy}}{{dx}}(\dfrac{A}{B}) + \dfrac{{dy}}{{dx}}y = \dfrac{{dy}}{{dx}}x$ 

Therefore, the order is 1.

(V)$ \dfrac{{{x^2}}}{{{a^2}}} - \dfrac{{{y^2}}}{{{b^2}}} = 0$ 

Ans:

$  {\dfrac{{{x^2}}}{{{a^2}}} - \dfrac{{{y^2}}}{{{b^2}}} = 0} $  

 $ {\dfrac{{{x^2}}}{{{a^2}}} = \dfrac{{{y^2}}}{{{b^2}}}} $  

 $ {{x^2} = \dfrac{{{a^2}}}{{{b^2}}}{y^2}} $  

 $ {x =  \pm \dfrac{a}{b}y} $

 $ {x = \dfrac{a}{b}y} $  

$  {1 = \dfrac{a}{b}\dfrac{{dy}}{{dx}}} $  

$  {\dfrac{{dy}}{{dx}} = \dfrac{b}{a}} $

 $ { - \dfrac{a}{b}y = x} $  

 $ { - \dfrac{a}{b}\dfrac{{dy}}{{dx}} = 1} $  

$  {\dfrac{{dy}}{{dx}} = \dfrac{{ - b}}{a}}$

Therefore, the order is 1.

(VI)$ y{\text{ }} = {\text{ }}a{\text{ }}cos{\text{ }}\left( {x{\text{ }} + {\text{ }}b} \right)$ 

Ans:

Given $ y = acos\left( {x + b} \right)$ 

Differentiating it w.r.t x

$ \dfrac{{dx}}{{dy}} =  - asin\left( {x + b} \right)$ 

Therefore, the order is 1.

(VII)$ y{\text{ }} = {\text{ }}a{\text{ }} + {\text{ }}b{e^{x + c}}$ 

Ans:

$ y{\text{ }} = {\text{ }}a{\text{ }} + {\text{ }}b{e^{x + c}}$ 

$y=a+b{{e}^{x+c}}$

$b{{e}^{x+c}}=y-a$  

$\frac{dy}{dx}=b{{e}^{x+c}}$  

 $ {\dfrac{{dy}}{{dx}} = b{e^{x + c}} = y - a} $  

$  {{\text{ diff -  wrt }}x} $  

$\frac{{{d}^{2}}y}{d{{x}^{2}}}=\frac{dy}{dx}\text{ }$

Hence, the order is 2.

5. (i) Show that ${\text{ }}y = {e^{m{{\sin }^{ - 1}}x}}$is a solution of

$  {\text{ }} $  

 $ {\left( {1 - {x^2}} \right)\dfrac{{{d^2}y}}{{d{x^2}}} - x\dfrac{{dy}}{{dx}} - {m^2}y = 0} $

Ans:

Using the Chain Rule, we get,

 $ { \Rightarrow {y_1} = \dfrac{{dy}}{{dx}} = \left( {{e^{m{{\sin }^{ - 1}}x}}} \right) \cdot \dfrac{d}{{dx}}\left( {m{{\sin }^{ - 1}}x} \right)} $  

$  { = y \cdot \left\{ {\dfrac{m}{{\sqrt {1 - {x^2}} }}} \right\}} $  

$  { \Rightarrow {y_1} \cdot \sqrt {1 - {x^2}}  = my.} $  

$  {{\text{ }}y_1^2\left( {1 - {x^2}} \right) = {m^2}{y^2}} $

W.r.t x, using the product and chain rule

 $ {y_1^2 \cdot \dfrac{d}{{dx}}\left( {1 - {x^2}} \right) + \left( {1 - {x^2}} \right) \cdot \dfrac{d}{{dx}}\left( {y_1^2} \right) = {m^2}\dfrac{d}{{dx}}\left( {{y^2}} \right),} $  

 $ { \Rightarrow y_1^2( - 2x) + \left( {1 - {x^2}} \right)\left( {2{y_1}} \right) \cdot \dfrac{d}{{dx}}\left( {{y_1}} \right) = {m^2}(2y) \cdot \dfrac{d}{{dx}}(y)} $

$ \Rightarrow y_1^2( - 2x) + \left( {1 - {x^2}} \right)\left( {2{y_1}} \right)\left( {{y_2}} \right) = {m^2}(2y)\left( {{y_1}} \right)$

Dividing by $2{y_1} \ne 0$, we get,

$\left( {1 - {x^2}} \right){y_2} - x{y_1} = {m^2}y$

Hence, the Proof.

(ii) Show that $y = \sin (\sin x)$ is a solution of differential equation

$\dfrac{{{d^2}y}}{{d{x^2}}} + (\tan x)\dfrac{{dy}}{{dx}} + y{\cos ^2}x = 0$

Ans:

$  \text{y = sin}\left( \text{sin x} \right)\text{ } $ 

$  \text{dy/dx = cos }\left( \text{sinx} \right)\text{. cosx} $ 

$  \text{ and d^2y/d}{{\text{x}}^{2}}\text{ }\!\!~\!\!\text{ = -sin }\left( \text{sinx} \right)\text{. cos2x - sinx cos }\left( \text{sinx} \right) $ 

$  \text{ LHS = - sin}\left( \text{sinx} \right)\text{ cos2x - sinx cos}\left( \text{sinx} \right)\text{ + sinx/ cosx cos }\left( \text{sinx} \right)\text{ cosx + sin }\left( \text{sinx} \right)\text{ cos2 }\!\!~\!\!\text{ x } $ 

$  \text{= 0 = RHS} $

  $  \text{y = sin(sinx)} $ 

 $  \Rightarrow \text{dy/dx}\,\text{=}\,\text{cosxcos(sinx)} $ 

 $  \Rightarrow {{\text{d}}^{\text{2}}}\text{y/d}{{\text{x}}^{\text{2}}}\text{= -cosx}\cdot \text{cosx}\cdot \text{sin(sinx)}\,\text{-}\,\text{sinx}\cdot \text{cos(sinx)} $ 

 $  \Rightarrow {{\text{d}}^{\text{2}}}\text{y/d}{{\text{x}}^{\text{2}}}\text{= -yco}{{\text{s}}^{\text{2}}}\text{x}\,\text{-}\,\text{(sinx/cosx)dy/dx} $ 

 $  \text{= }{{\text{d}}^{\text{2}}}\text{y/d}{{\text{x}}^{\text{2}}}\text{+}\,\text{tanx(dy/dx)}\,\text{+}\,\text{yco}{{\text{s}}^{\text{2}}}\text{x} $ 

 $  \text{= 0} $

(III) Show that${\text{  }}y = Ax + \dfrac{B}{x}{\text{   }}$is a solution  $\dfrac{{{x^2}{d^2}y}}{{d{x^2}}} + x\dfrac{{dy}}{{dx}} - y = 0$

Ans:  

Here ‘a’ and ‘b’ are arbitrary 

Given solution 

$  \text{ }y\text{ }=\text{ }ax\text{ }+\text{ }\left( b/x \right),\text{ }x~\ne \text{ }0 $ 

$  \text{ }y\text{ }=\text{ }ax\text{ }+\text{ }\left( b/x \right)\text{ }...\text{ }\left( 1 \right) $ 

$  ~\text{ }xy\text{ }=\text{ }a{{x}^{2}}\text{ }+\text{ }b $ 

Differentiate with respect to ‘x’

$ xy\text{ }+\text{ }y\text{ }.\text{ }1\text{ }=\text{ }a\text{ }\left( 2x \right)\text{ }=\text{ }2ax\text{ }...\text{ }\left( 2 \right)~$ 

Differentiate with respect to ‘x’

Differentiate again w.r.t ‘x’

$ xy\text{ }+\text{ }y\text{ }.\text{ }1\text{ }+\text{ }y\text{ }=\text{ }2a\text{ }\Rightarrow \text{ }xy\text{ }+\text{ }2y\text{ }=\text{ }2a\text{ }\ldots \text{ }\left( 3 \right)$ 

Substitute (3) in (2)

$  xy\text{ }+\text{ }y\text{ }=\text{ }\left( xy\text{ }+\text{ }2y \right)x~\text{ } $  

$xy\text{ }+\text{ }y\text{ }=\text{ }{{x}^{2}}y\text{ }+\text{ }2xy $ 

$  ={{x}^{2}}y\text{ }+\text{ }xy\text{ }\text{ }y\text{ } $ 

$  =\text{ }0~ $

Hence, Proved

(IV) Show that $y = a\cos (\log x) + b\sin (\log x)$ is a solution of

${x^2}\dfrac{{{d^2}y}}{{d{x^2}}} + x\dfrac{{dy}}{{dx}} + y = 0$

Ans:

$y = a\cos (\log x) + b\sin (\log x)$

Differentiating w.r.t. x, 

$  \dfrac{dy}{dx}=-a\sin (\log x)\times \dfrac{1}{x}+b\cos (\log x)\times \dfrac{1}{x}\dfrac{{{d}^{2}}y}{d{{x}^{2}}} $ 

$  =-a\left[ \dfrac{\cos (\log x)\times \dfrac{1}{x}\times x-1\cdot \sin (\log x)}{{{x}^{2}}} \right]+b\left[ \dfrac{-\sin (\log x)\times \dfrac{1}{x}\times x-1\cdot \cos (\log x)}{{{x}^{2}}} \right] $ 

$  \Rightarrow \quad {{x}^{2}}\dfrac{{{d}^{2}}y}{d{{x}^{2}}}=-a\cos (\log x)+a\sin (\log x)-b\sin (\log x)-b\cos (\log x) $ 

$  \Rightarrow \quad {{x}^{2}}\dfrac{{{d}^{2}}y}{d{{x}^{2}}}=-[a\cos (\log x)+b\sin (\log x)]-[-a\sin (\log x)+b\cos (\log x)] $ 

$  =-y-x\dfrac{dy}{dx}\quad  $ 

$  [\text{ }(1)\text{ and }(2)] $ 

$  \Rightarrow \quad {{x}^{2}}\dfrac{{{d}^{2}}y}{d{{x}^{2}}}+x\dfrac{dy}{dx}+y=0 $ 

(V) Verify that $y = \log \left( {x + \sqrt {{x^2} + {a^2}} } \right)$ satisfies the differential equation:

$\left( {{a^2} + {x^2}} \right)\dfrac{{{d^2}y}}{{d{x^2}}} + x\dfrac{{dy}}{{dx}} = 0$

Ans: Given

$ y{\text{ }} = {\text{ }}l{\text{ }}o{\text{ }}g{\text{ }}\left( {{\text{ }}x{\text{ }} + {\text{ }}\sqrt {{x^2} + {a^2}} } \right)$ 

On differentiating with x, we get

$\dfrac{{dy}}{{dx}} = \dfrac{1}{{x + \sqrt {{x^2} + {a^2}} }}\left( {1 + \dfrac{x}{{\sqrt {{x^2} + {a^2}} }}} \right)$

$ = \dfrac{1}{{\sqrt {{x^2} + {a^2}} }}$

On differentiating again with ${\text{x}}$, we get

$\dfrac{{{d^2}y}}{{d{x^2}}} =  - \dfrac{x}{{{{\left( {{x^2} + {a^2}} \right)}^{\dfrac{3}{2}}}}}$

Now let's see what is the value of $\dfrac{{{d^2}y}}{{d{x^2}}} + x\dfrac{{dy}}{{dx}}$

$ =  - \dfrac{x}{{{{\left( {{x^2} + {a^2}} \right)}^{\dfrac{3}{2}}}}} + \dfrac{x}{{\sqrt {{x^2} + {a^2}} }}$

Conclusion: Therefore,

  ${{\text{  }}y = \log \left( {x + \sqrt {{x^2} + {a^2}} } \right){\text{  }}} $  

Is not the solution of $\dfrac{{{d^2}y}}{{d{x^2}}} + x\dfrac{{dy}}{{dx}} = 0$

(VI) Find the differential equation of the family of curves$ y{\text{ }} = {\text{ }}{e^x}{\text{ }}(A{\text{ }}cos{\text{ }}x{\text{ }} + {\text{ }}B{\text{ }}sin{\text{ }}x)$ , where A and B are arbitrary constants.

Ans:

$  y\text{ }=\text{ }{{e}^{x}}\left( Acosx\text{ }+\text{ }Bsinx \right)\text{ }.....\left( 1 \right) $ 

$  \text{ }\therefore ~\text{ }\left( dy/dx \right)\text{ }=\text{ }{{e}^{x}}\left[ \text{ }A\text{ }sin\text{ }x\text{ }+\text{ }B\text{ }cosx \right]\text{ }+\text{ }\left[ Acosx\text{ }+\text{ }Bsinx \right]ex~ $ 

$  \text{ }\therefore ~\left( dy/dx \right)\text{ }=\text{ }{{e}^{x}}\left[ \left( B\text{ }\text{ }A \right)\text{ }sin\text{ }x\text{ }+\text{ }\left( B\text{ }+\text{ }A \right)\text{ }cos\text{ }x \right] $ 

$  \text{ }\therefore ~\left( dy/dx \right)\text{ }=\text{ }{{e}^{x}}\left[ \left( A\text{ }+\text{ }B \right)\text{ }cos\text{ }x\text{ }\text{ }\left( A\text{ }\text{ }B \right)\text{ }sin\text{ }x \right]\text{ }.....\left( 2 \right) $ 

$  \text{ }\therefore ~\text{ }\left( d^2y/dx^2 \right)\text{ }=\text{ }{{e}^{x}}\left[ \left( A\text{ }+\text{ }B \right)\text{ }cos\text{ }x\text{ }\text{ }\left( A\text{ }\text{ }B \right)\text{ }sin\text{ }x \right]\text{ }+\text{ }{{e}^{x}}\left[ \text{ }\left( A\text{ }+\text{ }B \right)\text{ }sin\text{ }x\text{ }\text{ }\left( A\text{ }\text{ }B \right)\text{ }cos\text{ }x \right] $ 

$  ~\text{ }\therefore ~\text{ }~\text{ }\left( d^2y/dx^2 \right)\text{ }=\text{ }{{e}^{x}}\left[ 2B\text{ }cos\text{ }x\text{ }\text{ }2A\text{ }sin\text{ }x \right]\text{ } $ 

$  \therefore ~\left( d^2y/dx^2 \right)\text{ }=\text{ }2{{e}^{x}}\left[ B\text{ }cos\text{ }x\text{ }\text{ }A\text{ }sin\text{ }x \right]\text{ } $ 

$  \therefore ~\text{ }~\text{ }\left( d^2y/dx^2 \right)\text{ }\times \text{ }\left( 1/2 \right)\text{ }=\text{ }{{e}^{x}}\left[ B\text{ }cos\text{ }x\text{ }\text{ }A\text{ }sin\text{ }x \right]\text{ }.....\left( 3 \right) $ 

$  \text{ }\left( 1 \right)\text{ }+\text{ }\left( 3 \right)\text{  }y\text{ }+\text{ }\left( 1/2 \right)\left( d^2y/dx^2 \right)\text{ }=\text{ }{{e}^{x}}\left[ \left( A\text{ }+\text{ }B \right)\text{ }cosx\text{ }\text{ }\left( A\text{ }\text{ }B \right)\text{ }sinx \right]\text{ } $ 

$  \therefore ~\text{ }~y\text{ }+\text{ }\left( 1/2 \right)\left( d^2y/dx^2 \right)\text{ }=\text{ }\left( dy/dx \right)\text{ }-------\text{  }\left( 2 \right)\text{ } $ 

$  \therefore ~\text{ }\left( d^2y/dx^2 \right)\text{ }\text{ }2\left( dy/dx \right)\text{ }+\text{ }2y\text{ }=\text{ }0 $ 

(vii) Find the differential equation of an ellipse with major and minor axes 2a and 2b respectively.

Ans:

Equation of ellipse

$ \dfrac{{{x^2}}}{{{a^2}}} + \dfrac{{{y^2}}}{{{b^2}}} = 1$ 

Differentiate w.r.t x

  ${2{a^2}\left[ {y\dfrac{{{d^2}y}}{{d{x^2}}} + {{\left( {\dfrac{{dy}}{{dx}}} \right)}^2}} \right] + 2{b^2} = 0} $  

  ${y\dfrac{{{d^2}y}}{{d{x^2}}} + {{\left( {\dfrac{{dy}}{{dx}}} \right)}^2} = \dfrac{{ - p{b^2}}}{{k{a^2}}} = \dfrac{{ - {b^2}}}{{{a^2}}} = \dfrac{y}{x}\dfrac{{dy}}{{dx}}} $  

 $ {y\dfrac{{{d^2}y}}{{d{x^2}}} + {{\left( {\dfrac{{dy}}{{dx}}} \right)}^2} = \dfrac{y}{x}\dfrac{{dy}}{{dx}}} $  

 $ {xy\dfrac{{dy}}{{d{x^2}}} + x{{\left( {\dfrac{{dy}}{{dx}}} \right)}^2} = y\dfrac{{dy}}{{dx}}}$ 

(viii) Form the differential equation representing the family of curves $ {\left( {y{\text{ }}-{\text{ }}b} \right)^2} = {\text{ }}4\left( {x{\text{ }}-{\text{ }}a} \right).$ 

Ans:

The general equation of the given family of curves

${(y - b)^2} = 4(x - a)\; \ldots(i)$ 

Differentiating (i) w.r.t.x, we

get

$2(y-b)\frac{dy}{dx}=4\Rightarrow(y-b){y}'=2\ ...(ii)$ 

Where $\frac{dy}{dx}={y}'.$ 

Differentiating (ii) w.r.t.y, we

get

$(y-b){y}''+{{\left( {{y}'} \right)}^{2}}=0\quad\text{ }\!\!~\!\!\text{ }\ldots 

(iii)$,

where $\frac{{{d}^{2}}y}{d{{x}^{2}}}={y}''$

Putting $(y-b)=\frac{2}{{{y}'}}$ from (ii) in (iii),

 we get $\frac{2{y}''}{{{y}'}}+{{\left( {{y}'}\right)}^{2}}=0\Rightarrow 2{y}''+{{\left( {{y}'} \right)}^{3}}=0$

Hence, $2\dfrac{{{d^2}y}}{{d{x^2}}} + {\left({\dfrac{{dy}}{{dx}}} \right)^3} = 0$ is the required differential equation.

6. Solve the following differential equations.

(I)$ \dfrac{{dy}}{{dx}} + y\cot x = \sin 2x$ 

Ans:

$ \dfrac{{dy}}{{dx}} + y\cot x = \sin 2x$ 

Comparing equation (1) by $\dfrac{{dy}}{{dx}} + Py = Q$ 

$P = \cot x,Q = \sin x$

$\therefore \quad $ I.F. $ = {e^{\int {\cot } xdx}}$

$ \Rightarrow \quad $ I.F $ = {e^{\log \sin x}} = \sin x$

Multiplying equation (1) by $\sin x$ $\sin x\dfrac{{dy}}{{dx}} + \sin x \cdot \cot xy = {\sin ^2}x$

$ \Rightarrow \sin x\dfrac{{dy}}{{dx}} + \cos xy = 1 - {\cos ^2}x$

$ \Rightarrow \quad \dfrac{d}{{dx}}(\sin x \cdot y) = 1 - {\cos ^2}x$

  ${\int {\left[ {\dfrac{d}{{dx}}(\sin x \cdot y)} \right]} dx = \int 1 dx - \int {{{\cos }^2}} xdx} $  

  ${ \Rightarrow \quad y\sin x = x - \int {\dfrac{{1 + \cos 2x}}{2}} dx} $  

  ${ \Rightarrow \quad y\sin x = x - \dfrac{1}{2}\int d x - \dfrac{1}{2}\int {\cos } 2xdx} $  

  ${ \Rightarrow \quad y\sin x = x - \dfrac{1}{2}x - \dfrac{1}{2}\dfrac{{\sin 2x}}{2} + C} $  

  ${ \Rightarrow \quad y\sin x = \dfrac{1}{2}x - \dfrac{1}{4}\sin 2x + C} $

(II)$x\dfrac{{dy}}{{dx}} + 2y = {x^2}\log x$ 

Ans:

$x\dfrac{{dy}}{{dx}} + 2y = {x^2}\log x$ 

$\Rightarrow \frac{dy}{dx}+\frac{2}{x}y=x\log x\ \text{    }......\text{(}1)$

Comparing equation (1) by $\dfrac{{dy}}{{dx}} + Py = Q$

$P = \dfrac{2}{x},Q = x\log x$

. I.F. $= {e^{\int {\dfrac{2}{x}} dx}} = {e^{2\log x}} = {e^{\log {x^2}}} = {x^2}$

Multiplying equation (1) by ${x^2}$

${x^2}\dfrac{{dy}}{{dx}} + {x^2} \times \dfrac{2}{x}y = {x^3}\log x$

$\Rightarrow {x^2}\dfrac{{dy}}{{dx}} + 2xy = {x^3}\log x$

$\Rightarrow \quad \dfrac{d}{{dx}}\left( {{x^2}y} \right) = {x^3}\log x$

Integrating both sides w.r.t. $x$

$  {{x}^{2}}y=\int{{{x}^{3}}}\log xdx+C$ 

$  =\log x\int{{{x}^{3}}}dx-\int{\left\{ \dfrac{d}{dx}(\log x)\int{{{x}^{3}}}dx \right\}}dx+C$ 

(Taking $\log x$ as first function) $= \dfrac{{{x^4}}}{4}\log x - \int {\dfrac{1}{x}}  \times \dfrac{{{x^4}}}{4}dx + C$

$= \dfrac{{{x^4}}}{4}\log x - \dfrac{1}{4}\int {{x^3}} dx + C$

$= \dfrac{{{x^4}}}{4}\log x - \dfrac{1}{4} \times \dfrac{{{x^4}}}{4} + C$

$\Rightarrow \quad {x^2}y = \dfrac{1}{{16}}{x^4}[4\log x - 1] + C$

$16{x^2}y = 4{x^4}\log x - {x^4} + C$

(III)  $\dfrac{{dx}}{{dy}} +\dfrac{1}{x}.y = \cos x + \dfrac{{\sin x}}{x},x > 0$ 

Ans: $\dfrac{{dx}}{{dy}} +\dfrac{1}{x}.y = \cos x + \dfrac{{\sin x}}{x},x > 0$ 

  ${P =\dfrac{1}{x},\quad Q = \cos x + \dfrac{{\sin x}}{x}}$  

$\text{I}\text{.F}={{e}^{\int{P}dx}}={{e}^{\int{\frac{1}{x}}dx}}={{e}^{\log x}}=x$

$\text{ }\!\!~\!\!\text{}y(\text{I}\text{.F})=\int{Q}\cdot \text{(I}\text{.F)}dx$ 

$\text{ }\!\!~\!\!\text{}=\int{\left( \cos x+\frac{\sin x}{x} \right)}xdx$ 

$  =\int{\cos}xxdx+\int{\sin }xdx$ 

$=x\sin x-\int{\sin x dx}+\int{\sin xdx}+c$ 

$=x\sin x+c$

(Iv)$ {\cos ^3}x\dfrac{{dy}}{{dx}} + \cos x = \sin x$ 

Ans:

$ {\cos ^3}x\dfrac{{dy}}{{dx}} + \cos x = \sin x$ 

Differentiate w.r.t y

 $ {\dfrac{{dy}}{{dx}} + \dfrac{y}{{{{\cos }^2}n}} = \dfrac{{\sin x}}{{{{\cos }^3}x}}} $  

 $ {\dfrac{{dy}}{{dx}} + y \cdot {{\sec }^2}x = \dfrac{{\sin x}}{{{{\cos }^3}x}}}$ 

  ${I \cdot F \cdot  = {e^{\int {{{\sec }^2}} xdx}} = {e^{\tan x}}} $  

  ${y \cdot {e^{\tan x}} = \int {{e^{t\tan x}}} \tan x \cdot {{\sec }^2}xdx}$ 

$  =\int{{{e}^{t}}}\cdot tdt $ 

$  ={{e}^{t}}(t-1)+c $ 

$  y\cdot \tan x={{e}^{\tan x}}(\tan x-1)+c $ 

(V)$ ydx + (x - {y^3})dy = 0$ 

Ans:

The given differential equation is

$ ydx + (x - {y^3})dy = 0$ 

$ \Rightarrow \quad y\dfrac{{dx}}{{dy}} + \left( {x - {y^3}} \right) = 0$

$ \Rightarrow \quad y\dfrac{{dx}}{{dy}} + x = {y^3}$

$ \Rightarrow \dfrac{{dx}}{{dy}} + \dfrac{x}{y} = {y^2}$

which is a linear differential equation

 Thus, ${\text{IF}} = {e^{\int {\dfrac{{dy}}{y}} }} = {e^{\log y}} = y$

Multiplying both sides of E (i) by IF and integrating,

we get

$x \cdot ({\text{IF}}) = \int Q  \cdot ({\text{IF}})dy + c$

$ \Rightarrow x \cdot y = \int {{y^3}} dy + c$

$ = \dfrac{{{y^4}}}{4} + c$

which is the required solution.

(VI)$ y{e^y}dx = ({y^3} + 2x{e^y})dy$ 

Ans:

$ y{e^y}dx = ({y^3} + 2x{e^y})dy$ 

$\dfrac{{y{e^y}}}{{\left( {{y^3} + 2x{e^y}} \right)}} = \dfrac{{dy}}{{dx}}$ or, $\dfrac{{dx}}{{dy}} + \left( { - \dfrac{2}{y}} \right)x = \dfrac{{{y^2}}}{{{e^y}}}$,

which is linear in $x$.

I.F. $={{e}^{\int{\frac{-2}{y}}dy}}={{e}^{-2\log y}}=\frac{1}{{{y}^{2}}}$

Multiplying both sides by the I.F. and integrating, we get,

$x\dfrac{1}{{{y^2}}} = \int {{e^{ - y}}} dy$

$x\dfrac{1}{{{y^2}}} =  - {e^{ - y}} + C$ or $x =  - {y^2}{e^{ - y}} + c{y^2}$

When $x = 0,y = 1.0 =  - {e^{ - 1}} + \operatorname{cor} c = \dfrac{1}{e}$.

Hence, the required particular solution is

$x =  - {y^2}{e^{ - y}} + \dfrac{{{y^2}}}{e}$

7. Solve each of the following differential equations:

(I)$ y - x\dfrac{{dy}}{{dx}} = 2({y^2} + \dfrac{{dy}}{{dx}})$ 

Ans:

$ y - x\dfrac{{dy}}{{dx}} = 2({y^2} + \dfrac{{dy}}{{dx}})$ 

Given differential equation can be written as 

 ${(x + 2)\dfrac{{dy}}{{dx}} = y - 2{y^2}{\text{ or }}\dfrac{{dy}}{{y(1 - 2y)}} = \dfrac{{dx}}{{x + 2}}} $  

 $ {{\text{ }}\quad \int {\dfrac{{dy}}{{y(1 - 2y)}}}  = \int {\dfrac{{dx}}{{x + 2}}} } $  

  ${{\text{  }}\int {\left[ {\dfrac{1}{y} + \dfrac{2}{{1 - 2y}}} \right]} dy = \int {\dfrac{{dx}}{{x + 2}}} } $  

 $ {\therefore \log |y| - \log |1 - ay|} $  

 $ { = \log |x + 2| + \log C} $  

  ${{\text{  }}\quad \dfrac{y}{{1 - 2y}} = C(x + 2)} $  

 $ {{\text{  }}\quad y = C(x + 2)(1 - 2y)} $

(II)$ cos{\text{ }}y{\text{ }}dx{\text{ }} + {\text{ }}\left( {1{\text{ }} + {\text{ }}2{e^{ - x}}{\text{ }}} \right){\text{ }}sin{\text{ }}y{\text{ }}dy{\text{ }} = {\text{ }}0.$ 

Ans:

$ cos{\text{ }}y{\text{ }}dx{\text{ }} + {\text{ }}\left( {1{\text{ }} + {\text{ }}2{e^{ - x}}{\text{ }}} \right){\text{ }}sin{\text{ }}y{\text{ }}dy{\text{ }} = {\text{ }}0.$ 

  ${ \Rightarrow \quad \int {\dfrac{{dx}}{{1 + 2{e^{ - x}}}}}  = \int {\dfrac{{ - \sin y}}{{\cos y}}} dy} $  

  ${ \Rightarrow \quad \int {\dfrac{{{e^x}}}{{2 + {e^x}}}} dx = \int {\dfrac{{ - \sin y}}{{\cos y}}} dy} $  

  ${ \Rightarrow \quad \ln \left( {{e^x} + 2} \right) = \ln |\cos y| + \ln C} $  

  ${ \Rightarrow \ln \left( {{e^x} + 2} \right) = \ln |\cos y|C}$ 

 $ { \Rightarrow \quad {{\text{e}}^{\text{x}}} + 2 = {\text{C}}\cos {\text{y}}\quad  \cdots (1)} $  

  ${ \Rightarrow {{\text{e}}^{\text{x}}} + 2 =  \pm {\text{C}}\cos {\text{y}} \Rightarrow {{\text{e}}^{\text{x}}} + 2 = {\text{k}}\cos {\text{y}}} $  

 $ {{\text{ x}} = 0,{\text{y}} = \dfrac{\pi }{4}{\text{ in }}(1){\text{ }}} $  

We get

 $ {1 + 2 = {\text{kcos}}\dfrac{\pi }{4}} $  

 $ { \Rightarrow {\text{k}} = 3\sqrt 2 } $  

 $ {\therefore {{\text{e}}^{\text{x}}} + 2 = 3\sqrt 2 \cos {\text{y  }}}$ 

Is the particular solution.

(III) $ x\sqrt {1 + {y^2}} dx + y\sqrt {1 + {x^2}} dy = 0$ 

Ans:

$ x\sqrt {1 + {y^2}} dx + y\sqrt {1 + {x^2}} dy = 0$ 

It is given that

$x\sqrt {1 + {y^2}} dx + y\sqrt {1 + {x^2}} dy = 0$

We can write it as

$\dfrac{x}{{\sqrt {1 + {x^2}} }}dx + \dfrac{y}{{\sqrt {1 + {y^2}} }}dy = 0$

By integrating the given terms, we get

$\int {\dfrac{x}{{\sqrt {1 + {x^2}} }}} dx + \int {\dfrac{y}{{\sqrt {1 + {y^2}} }}} dy = c$

Using the formula

$\dfrac{d}{{dx}}\left( {\sqrt {1 + {x^2}} } \right) = \dfrac{{2x}}{{2 \cdot \sqrt {1 + {x^2}} }} = \dfrac{x}{{\sqrt {1 + {x^2}} }}$

We get

$\sqrt {1 + {x^2}}  + \sqrt {1 + {y^2}}  = C$

(IV)$ \sqrt {(1 - {x^2})(1 - {y^2})} dy + xydx = 0$ 

Ans:

$ \sqrt {(1 - {x^2})(1 - {y^2})} dy + xydx = 0$ 

By simplifying the equation, we get

$x y \dfrac{d y}{d x}=-\sqrt{1-x^{2}-y^{2}+x^{2} y^{2}} $ 

$\Rightarrow \quad x y \dfrac{d y}{d x}=-\sqrt{\left(1-x^{2}\right)\left(1-y^{2}\right)} $ 

$=-\sqrt{\left(1-x^{2}\right)} \sqrt{\left(1-y^{2}\right)} $ 

$\Rightarrow \quad \dfrac{y}{\sqrt{1-y^{2}}} d y=-\dfrac{\sqrt{1-x^{2}}}{x} d x$

Integrating both sides, we get

$\int {\dfrac{y}{{\sqrt {1 - {y^2}} }}} dy =  - \int {\dfrac{{\sqrt {1 - {x^2}} }}{x}} dx$

$ {{\text{ }}1 - {y^2} = t\quad  \Rightarrow 2ydy = dt} $  

 $ {{\text{ }}1 - {x^2} = {m^2} \Rightarrow 2xdx = 2mdm \Rightarrow xdx = mdm} $  

 $ {\therefore {\text{ (i) }} \Rightarrow \dfrac{1}{2}\int {\dfrac{1}{{\sqrt t }}} dt =  - \int {\dfrac{m}{{{m^2} - 1}}}  \cdot mdm} $

 $ { \Rightarrow \quad } {\dfrac{1}{2}\dfrac{{{t^{1/2}}}}{{1/2}} + \int {\dfrac{{{m^2}}}{{{m^2} - 1}}} dm = 0\quad  \Rightarrow \sqrt t  + \int {\dfrac{{{m^2} + 1 - 1}}{{{m^2} - 1}}} dm = 0} $  

  ${ \Rightarrow \quad } {\sqrt t  + \int {\left( {1 + \dfrac{1}{{{m^2} - 1}}} \right)} dm = 0\quad  \Rightarrow \sqrt t  + m + \dfrac{1}{2}\log \left| {\dfrac{{m - 1}}{{m + 1}}} \right| = 0} $

Now substituting this value of ${\text{t}}$ and ${\text{m}}$, we get

$ \sqrt {1 - {y^2}}  - \sqrt {1 - {x^2}}  + \dfrac{1}{2}\log \left| {\dfrac{{\sqrt {1 - {y^2}}  - 1}}{{\sqrt {1 - {y^2}}  + 1}}} \right| + C = 0$ 

(V)$ \left( {x{y^2}{\text{ }} + {\text{ }}x} \right){\text{ }}dx{\text{ }} + {\text{ }}\left( {y{x^2}{\text{ }} + {\text{ }}y} \right){\text{ }}dy{\text{ }} = {\text{ }}0;{\text{ }}y\left( 0 \right){\text{ }} = {\text{ }}1.$ 

Ans:

$ \left( {x{y^2}{\text{ }} + {\text{ }}x} \right){\text{ }}dx{\text{ }} + {\text{ }}\left( {y{x^2}{\text{ }} + {\text{ }}y} \right){\text{ }}dy{\text{ }} = {\text{ }}0$ 

  ${\left( {x{y^2} + x} \right)dx =  - \left( {{x^2}y + y} \right)dy} $  

 $ {x\left( {{y^2} + 1} \right)dx =  - y\left( {{x^2} + 1} \right)dy} $  

  ${\dfrac{x}{{\left( {{x^2} + 1} \right)}}dx = \dfrac{{ - y}}{{1 + {y^2}}}dy = \dfrac{{2x}}{{\left( {{x^2} + 1} \right)}}dx = \dfrac{{ - 2y}}{{1 + {y^2}}}dy} $  

  ${{\text{  }}} $    

Integrating both sides

$\int {\dfrac{{2x}}{{1 + {x^2}}}} dx =  - \int {\dfrac{{2y}}{{1 + {y^2}}}} dy$

This is the integrals of the type

$  \int{\dfrac{{{f}^{\prime }}(x)}{f(x)}}dx=\log |f(x)|+c\log \left| {{x}^{2}}+1 \right| $ 

$  =-\log \left| {{y}^{2}}+1 \right|+\log c\log \left| {{x}^{2}}+1 \right|+\log \left| {{y}^{2}}+1 \right| $ 

$  =\log c\log \left| {{x}^{2}}+1 \right|\left| {{y}^{2}}+1 \right|=\log c $ 

$  \Rightarrow c=\left| {{x}^{2}}+1 \right|\left| {{y}^{2}}+1 \right| $ 

(VI) $ \dfrac{{dy}}{{dx}} = y{\sin ^3}xco{x^2}x + x{e^x}$ 

Ans:

$ \dfrac{{dy}}{{dx}} = y{\sin ^3}xco{x^2}x + x{e^x}$ 

  ${\dfrac{{dy}}{{dx}} = {{\sin }^3}x \cdot {{\cos }^2}x + x \cdot {e^x} \cdot (1)} $  

 $ {dy = \left[ {\left( {{{\sin }^3}x \cdot {{\cos }^2}x} \right) + x \cdot {e^x}} \right] \cdot dx \to 2} $  

 $ {{\text{  }}} $  

Taking the integral sign in both side of equation (2)

$\int d y = \int {{{\sin }^3}} x \cdot {\cos ^2}xdx + \int x  \cdot {e^x} \cdot dx + c$

  ${\int {{{\sin }^3}} x \cdot {{\cos }^2}xdx\quad {\text{ wt }}\quad \cos x = t} $  

 $ {\int {\sin } x \cdot {{\sin }^2}x \cdot {{\cos }^2}xdx} $  

 $ {\int {\sin } x\left( {1 - {{\cos }^2}x} \right) \cdot {{\cos }^2}xdx} $  

  ${ - \int {\left( {1 - {t^2}} \right)}  \cdot {t^2}dt \Rightarrow  - \int {{t^2} - {t^4}dt} } $

$  =-\left[ \dfrac{{{t}^{3}}}{3}-\dfrac{{{t}^{5}}}{5} \right] $ 

$  \Rightarrow -\left[ \dfrac{{{\cos }^{3}}x}{3}-\dfrac{{{\cos }^{5}}t}{s} \right] $ 

$  \int{x}\cdot {{e}^{x}}dx=\left. x\int{{{e}^{n}}}dx-\int{\dfrac{d}{dx}}x\cdot \int{{{e}^{x}}}dx \right]dx $ 

$  =x{{e}^{x}}-\int{{{e}^{x}}}dx $ 

$  =x{{e}^{x}}-{{e}^{x}} $ 

$  ={{e}^{x}}(x-1) $ 

From Equation (3)

  ${{\text{  }}} $  

 $ {y =  - \left[ {\dfrac{{{{\cos }^3}x}}{3} - \dfrac{{{{\cos }^5}x}}{5}} \right] + {e^n}(x - 1) + C} $

(VII)$ {\sec ^2}x\tan ydx + {\sec ^2}y\tan xdy = 0$ 

Ans:

Given equation:

${\sec ^2}x\tan ydx + {\sec ^2}y\tan xdy = 0$

Dividing both sides by $\tan y\tan x$

 $ {\dfrac{{{{\sec }^2}x\tan ydx + {{\sec }^2}y\tan xdy}}{{\tan y\tan x}} = \dfrac{0}{{\tan x\tan y}}} $  

 $ {\dfrac{{{{\sec }^2}x\tan ydx}}{{\tan y\tan x}} + \dfrac{{{{\sec }^2}y\tan xdy}}{{\tan y\tan x}} = 0} $  

 $ {\dfrac{{{{\sec }^2}x}}{{\tan x}}dx + \dfrac{{{{\sec }^2}y}}{{\tan y}}dy = 0} $

Integrating both sides

 $ {\int {\left( {\dfrac{{{{\sec }^2}x}}{{\tan x}}dx + \dfrac{{{{\sec }^2}y}}{{\tan y}}dy} \right)}  = 0} $  

 $ {\int {\dfrac{{{{\sec }^2}x}}{{\tan x}}} dx + \int {\dfrac{{{{\sec }^2}y}}{{\tan y}}} dy = 0} $  

$  {{\text{  }}u = \tan x{\text{ , }}v = \tan y} $

Diff u w.r.t. x and v w.r.t y

 ${\dfrac{{du}}{{dx}} = {{\sec }^2}x}$

$  {\dfrac{{du}}{{{{\sec }^2}x}} ={\text{dx}}}$

${\dfrac{{dv}}{{dy}} = {{\sec }^2}y}$  

$\frac{dv}{{{\sec }^{2}}y}=\text{dy}$

Therefore, our equation becomes

$\int {\dfrac{{{{\sec }^2}x}}{{\tan x}}} dx + \int {\dfrac{{{{\sec }^2}y}}{{\tan y}}} dy = 0$

 $ {\int {\dfrac{{{{\sec }^2}x}}{{\tan x}}} \dfrac{{du}}{{{{\sec }^2}x}} + \int{\dfrac{{{{\sec }^2}y}}{v}} \dfrac{{dv}}{{{{\sec }^2}y}} = 0} $  

  ${\int {\dfrac{{du}}{u}}  + \int {\dfrac{{dv}}{v}}  = 0} $  

 $ {\log |u| + \log |v| = \log c} $  

 $ {{\text{  }}u = \tan x{\text{ and }}v = \tan y} $  

 $ {\log |\tan x| + \log |\tan y| = \log c} $  

 $ {\log |\tan x + \tan y| = \log c} $ 

 $ {\tan x\tan y = C} $

8. Solve the following differential equations:

(I)$ {x^2}ydx - ({x^3} + {y^3})dy = 0$ 

Ans:

$\left( {{x^3} + {y^3}} \right)dy - {x^2}ydx = 0$ 

Is rearranged as 

  ${\text{ }} $  

$  {\quad \dfrac{{dy}}{{dx}} = \dfrac{{{x^2}y}}{{{x^3} + {y^3}}}} $  

  ${{\text{ }}\dfrac{y}{x} = v \Rightarrow y = vx} $  

 $ {\quad \dfrac{{dy}}{{dx}} = v + x\dfrac{{dv}}{{dx}}} $  

  ${\therefore \quad v + x\dfrac{{dv}}{{dx}} = \dfrac{v}{{1 + {v^3}}}} $  

$  { \Rightarrow \quad x\dfrac{{dv}}{{dx}} = \dfrac{v}{{1 + {v^3}}} - v = \dfrac{{ - {v^4}}}{{1 + {v^3}}}} $  

 $ { \Rightarrow \dfrac{{1 + {v^3}}}{{{v^4}}}dv =  - \dfrac{{dx}}{x}} $

Integrating both sides, we get $\int {\left( {\dfrac{1}{{{v^4}}} + \dfrac{1}{v}} \right)} dv =  - \int {\dfrac{{dx}}{x}} $

$ \Rightarrow  - \dfrac{1}{{3{v^3}}} + \log |v| =  - \log |x| + C$

$ \Rightarrow  - \dfrac{{{x^3}}}{{3{y^3}}} + \log \left| {\left( {\dfrac{y}{x}} \right)} \right| =  - \log |x| + C$

$ \Rightarrow \dfrac{{ - {x^3}}}{{3{y^3}}} + \log |y| = C$

Is the solution of the given differential equation.

(II)$ {x^2}\dfrac{{dy}}{{dx}} = {x^2} + xy + {y^2}$ 

Ans:

Solution of the given differential equation Re-writing the given equation as

$ {x^2}\dfrac{{dy}}{{dx}} = {x^2} + xy + {y^2}$ 

$ \dfrac{{dy}}{{dx}} = {\text{ }}1 + \dfrac{{{y^2}}}{{{x^2}}} + \dfrac{y}{x}$ 

It is clearly a homogenous differential equation

Assuming $ y = vx$ 

Differentiating both sides

$\dfrac{{dy}}{{dx}} = v + x\dfrac{{dv}}{{dx}}$

Substituting $\dfrac{{{\text{dy}}}}{{{\text{dx}}}}$ from the given equation

$1 + \dfrac{{{y^2}}}{{{x^2}}} + \dfrac{y}{x} = v + x\dfrac{{dv}}{{dx}}$

$1 + {{\text{v}}^2} + {\text{v}} = {\text{v}} + {\text{x}}\dfrac{{{\text{dv}}}}{{{\text{dx}}}}$

$1 + {{\text{v}}^2} = {\text{x}}\dfrac{{{\text{dv}}}}{{{\text{dx}}}}$

$\dfrac{{dx}}{x} = \dfrac{{dv}}{{1 + {v^2}}}$

Now integrating both sides

$\int {\dfrac{{dx}}{x}}  = \int {\dfrac{{dv}}{{1 + {v^2}}}} $

$\ln {\text{x}} = \arctan {\text{v}} + {\text{c}}$

Formula 

  ${{\text{  }}\int {\dfrac{{{\text{dx}}}}{{\text{x}}}}  = \ln {\text{x}}\int {\dfrac{{{\text{dv}}}}{{1 + {{\text{v}}^2}}}}  = {{\tan }^{ - 1}}{\text{v}}} $  

 $ {{\text{  v}} = \dfrac{{\text{y}}}{{\text{x}}}} $  

 $ {\ln x = {{\tan }^{ - 1}}\dfrac{y}{x} + c} $  

 $ {\text{ }} $

Is the solution of given differential equation.

(III) $ ({x^2} - {y^2})dx + 2xydy = 0,y(1) = 1$ 

Ans:

  ${\left( {{x^2} - {y^2}} \right)dx + 2xydy = 0} $  

  ${\dfrac{{dy}}{{dx}} =  - \dfrac{{\left( {{x^2} - {y^2}} \right)}}{{2xy}}} $  

$  {\text{ }} $  

It is homogeneous differential equation.

Putting${\text{ }}y = ux \Rightarrow \quad u + \dfrac{{xdu}}{{dx}} = \dfrac{{dy}}{{dx}}$

From (I)

$  {{\text{ }}u + x\dfrac{{du}}{{dx}} =  - {x^2}\dfrac{{\left( {1 - {u^2}} \right)}}{{2{x^2}u}} =  - \left( {\dfrac{{1 - {u^2}}}{{2u}}} \right)} $  

$  { \Rightarrow \quad \quad \dfrac{{xdu}}{{dx}} =  - \left[ {\dfrac{{1 - {u^2}}}{{2u}} + u} \right]} $  

$  { \Rightarrow \quad \quad \dfrac{{xdu}}{{dx}} =  - \left[ {\dfrac{{1 + {u^2}}}{{2u}}} \right]} $  

$  { \Rightarrow \quad \dfrac{{2u}}{{1 + {u^2}}}du =  - \dfrac{{dx}}{x}}$ 

Integrating both sides, we get

$ \Rightarrow \quad \int {\dfrac{{2udu}}{{1 + {u^2}}}}  =  - \int {\dfrac{{dx}}{x}} $

$ \Rightarrow \quad \log \left| {1 + {u^2}} \right| =  - \log |x| + \log C$

$ \Rightarrow \log \left| {\dfrac{{{x^2} + {y^2}}}{{{x^2}}}} \right||x| = \log C$

$ \Rightarrow \quad \dfrac{{{x^2} + {y^2}}}{x} = C$

$ \Rightarrow \quad {x^2} + {y^2} = Cx$

Given that $y = 1$ when $x = 1$ $ \Rightarrow \quad 1 + 1 = C \Rightarrow C = 2$

$\therefore $ Solution is ${x^2} + {y^2} = 2x$.

(IV)$ \dfrac{{dy}}{{dx}} = \dfrac{y}{x} + \tan (\dfrac{y}{x})$ 

Ans:

$  \dfrac{xdy}{dx}=y+x\tan \left( \dfrac{y}{x} \right) $ 

$  \dfrac{dy}{dx}=\dfrac{y}{x}+\tan \left( \dfrac{y}{x} \right) $ 

$  y=vx $ 

$  \dfrac{dy}{dx}=v+x\dfrac{dv}{dx} $ 

   $ v+x\dfrac{dv}{dx}=v+\tan v  $ 

   $x\dfrac{dv}{dx}=\tan v  $ 

$  \int{\dfrac{dv}{\tan v}}=\int{\dfrac{dx}{x}}\Rightarrow  $ 

$  \int{\dfrac{\cos v}{\sin v}}dv=\int{\dfrac{dx}{x}} $ 

 $\log \sin v=\log x+\log c  $ 

$  \log \sin \left( \dfrac{y}{x} \right)=\log \dfrac{c}{x} $ 

$  \Rightarrow \sin \left( \dfrac{y}{x} \right)=\dfrac{c}{x} $ 

$\sin \left( \dfrac{y}{x} \right)=cx  $ 

(V) $ \dfrac{{dy}}{{dx}} = \dfrac{{2xy}}{{{x^2} + {y^2}}}$ 

Ans:

$\left( {{x^2} + {y^2}} \right)\dfrac{{dy}}{{dx}} = 2xy$

$\dfrac{{dy}}{{dx}} = \dfrac{{2xy}}{{{x^2} + {y^2}}}$

$\dfrac{{dy}}{{dx}} = \left( {{x^2} + {y^2}} \right)/2xy \ldots .(i)$

Let $x = vy$

Here, differentiating w.r.t. ${\text{y}}$,

$\dfrac{{dx}}{{dy}} = {\text{v}}.(\dfrac{{dy}}{{dx}}) + {\text{y}}(\dfrac{{dv}}{{dy}})$

$\dfrac{{dx}}{{dy}} = ({\text{v}} + {\text{y}}) \cdot (\dfrac{{dy}}{{dx}})$

Here, from e (i),

  ${v + y(\dfrac{{dv}}{{dy}}) = \left( {{v^2}{y^2} + {y^2}} \right)/2v{y^2}} $  

$  {v + y(\dfrac{{dv}}{{dy}}) = {y^2}\left( {{v^2} + 1} \right)/{y^2}2v} $  

  ${v + y(\dfrac{{dv}}{{dy}}) = \left( {{v^2} + 1} \right)/2v} $  

 $ {y(\dfrac{{dv}}{{dy}}) = \left( {\left( {{v^2} + 1} \right)/2v} \right) - (v/1)} $  

$  {y(\dfrac{{dv}}{{dy}}) = \left( {{v^2} + 1 - 2{v^2}} \right)/2v} $  

 $ {y(\dfrac{{dv}}{{dy}}) = \left( { - {v^2} + 1} \right)/2v} $  

  ${y(\dfrac{{dv}}{{dy}}) = \left( {1 - {v^2}} \right)/2v} $  

  ${2v/\left( {1 - {v^2}} \right)dv = \dfrac{1}{y}dy} $

Integrating both sides

 $ {{\text{  }}} $  

 $ {\int 2 v\left( {{v^2} - 1} \right)dv =  - \int {\dfrac{{dy}}{y}} } $  

$  {\log \left| {{v^2} - 1} \right| =  - \log y + \log c} $  

  ${\log \left| {{v^2} - 1} \right|y = \log c} $  

$  {{v^2}y - y = c} $  

  ${\left( {\dfrac{{{x^2}}}{{{y^2}}}} \right)x(y - y) = c} $  

 $ {\left( {\dfrac{{{x^2}}}{y}} \right) - y = c} $  

 $ {\dfrac{{{x^2} - {y^2}}}{y} = c} $  

  ${{x^2} - {y^2} = cy} $

(VI) $ \dfrac{{dy}}{{dx}} = {e^{x + y}} + {x^2}{e^y}$ 

Ans:

From the integral

${\dfrac{{dy}}{{dx}} = {e^{x + y}} + {x^2} \cdot {e^y}} $  

$  {\dfrac{{dy}}{{dx}} = {e^x} \cdot {e^{\dot y}} + {x^2} \cdot {e^y}} $  

 $ {\dfrac{{dy}}{{dx}} = {e^y}\left( {{e^x} + {x^2}} \right)} $  

 ${\int {\dfrac{{dy}}{{{e^y}}}}  = \int {\left( {{e^x} + {x^2}} \right)} dx} $  

 $ { \Rightarrow \log {e^y} = {e^x} + \dfrac{{{x^3}}}{3} + C} $  

 $ {\therefore \quad y = {e^x} + \dfrac{{{x^3}}}{3} + C} $

(VII) $ \dfrac{{dy}}{{dx}} = \sqrt {\dfrac{{1 - {y^2}}}{{1 - {x^2}}}} $ 

Ans:

$ \dfrac{{dy}}{{dx}} = \sqrt {\dfrac{{1 - {y^2}}}{{1 - {x^2}}}} $ 

It is given that

$\dfrac{{dy}}{{dx}} - \sqrt {\dfrac{{1 - {y^2}}}{{1 - {x^2}}}}  = 0$

We can write it as

$\dfrac{{dy}}{{dx}} = \sqrt {\dfrac{{1 - {y^2}}}{{1 - {x^2}}}} $

By cross multiplication

$\dfrac{1}{{\sqrt {1 - {y^2}} }}dy = \dfrac{1}{{\sqrt {1 - {x^2}} }}dx$

By integrating both sides w.r.t ${\text{x}}$

$ \int {\dfrac{1}{{\sqrt {1 - {y^2}} }}} dy = \int {} \dfrac{1}{{\sqrt {1 - {x^2}} }}dx$ 

We get

${\sin ^{ - 1}}y = {\sin ^{ - 1}}x + c$

(VIII) $ (3xy + {y^2})dx + ({x^2} + xy)dy$ 

Ans:

  ${\dfrac{{dy}}{{dx}} =  - \left( {\dfrac{{3xy + {y^2}}}{{{x^2} + xy}}} \right)} $  

  ${{\text{ }}y = vx \Rightarrow \dfrac{{dy}}{{dx}} = v + x\dfrac{{dv}}{{dx}}} $  

  ${v + x\dfrac{{dv}}{{dx}} =  - \left( {\dfrac{{3x \cdot vx + {v^2}{x^2}}}{{{x^2} + x \cdot vx}}} \right)} $  

$  {x\dfrac{{dv}}{{dx}} = \dfrac{{ - 3v - {v^2}}}{{1 + v}} - v} $  

$ {x\dfrac{{dv}}{{dx}} = \dfrac{{ - 3v - {v^2} - v - {v^2}}}{{1 + v}}} $  

${x\dfrac{{dv}}{{dx}} = \dfrac{{ - 2{v^2} - 4v}}{{1 + v}}} $

$ {\dfrac{{1 + v}}{{2{v^2} + 4v}}dv =  - \dfrac{1}{x}dx} $  

$ {\int {\dfrac{{1 + v}}{{2{v^2} + 4v}}} dv = \int  -  \dfrac{1}{x}dx} $  

  ${\dfrac{1}{4}\int {\dfrac{{2 + 2v}}{{2v + {v^2}}}} dv =  - \int {\dfrac{1}{x}} dx} $  

  ${\dfrac{1}{4}\log \left| {{v^2} + 2v} \right| =  - \log |x| + c} $  

  ${\dfrac{1}{4}\log \left( {\dfrac{{{y^2}}}{{{x^2}}} + 2\dfrac{y}{x}} \right) \cdot x = c} $  

 $ {\log \left( {\dfrac{{{y^2}}}{x} + 2y} \right) = 4c} $

9. (I) Form the differential equation of the family of circles touching y-axis at (0, 0).

Ans:

The centre of the circle touching the y-axis at origin lies on the x-axis.

Let (a, 0) be the centre of the circle.

Since it touches the y-axis at origin, its radius is a.

Now, the equation of the circle with centre (a, 0) and radius (a) is

$  {{{(x - a)}^2} + {y^2} = {a^2}.} $  

$ { \Rightarrow {x^2} + {y^2} = 2ax}$ 

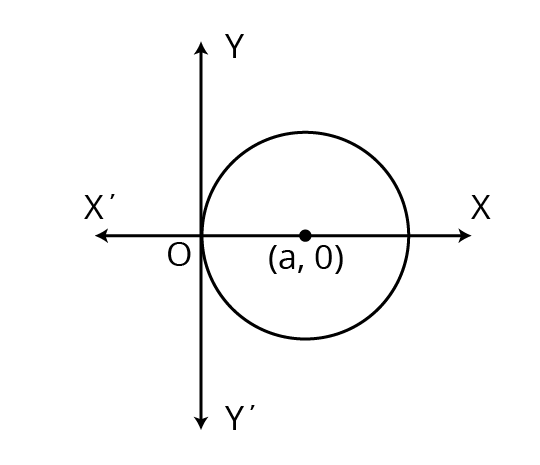
Differentiating equation (1) with respect to ${\text{x}}$, we get:

$2x + 2y{y^\prime } = 2a$

$ \Rightarrow x + y{y^\prime } = a$

Now, on substituting the value of a in equation (1), we get:

${x^2} + {y^2} = 2\left( {x + y{y^\prime }} \right)x$

$ \Rightarrow {x^2} + {y^2} = 2{x^2} + 2xy{y^\prime }$

$ \Rightarrow 2xy{y^\prime } + {x^2} = {y^2}$

This is the required differential equation.

(ii) Form the differential equation of family of parabolas having vertex at $ \left( {{\mathbf{0}},{\text{ }}{\mathbf{0}}} \right)$  and axis along the

 (i) positive y-axis 

Ans:

The equation of the parabola having the vertex at origin and the axis along the positive

${\text{y}}$-axis is:

${x^2} = 4ay$…….1

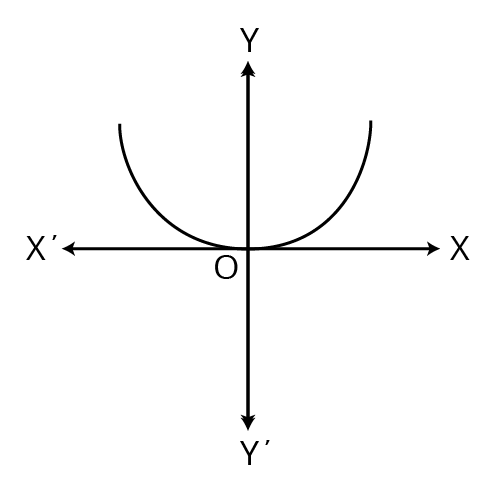
Differentiating equation (1) with respect to $x$, we get:

 $2x = 4a{y^\prime }$……(2)

Dividing equation (2) by equation (1), we get:

$\dfrac{{2x}}{{{x^2}}} = \dfrac{{4a{y^\prime }}}{{4ay}}$

$ \Rightarrow \dfrac{2}{x} = \dfrac{{{y^\prime }}}{y}$

$ \Rightarrow x{y^\prime } = 2y$

$ \Rightarrow x{y^\prime } - 2y = 0$

This is the required differential equation.

(ii) Positive x-axis

Ans: 

Since parabola has axis along positive $x$-axis,

its Equation: ${y^2} = 4ax$...(1)

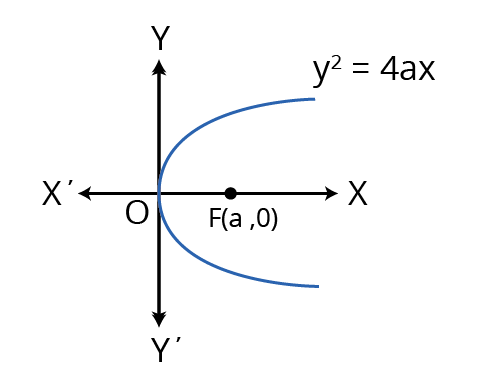
Diff. w.r.t. $x$

${\dfrac{d}{{dx}}\left( {{y^2}} \right) = \dfrac{d}{{dx}}(4ax)} $  

${2y\dfrac{{dy}}{{dx}} = 4a} $

Putting Value of 4 a in $(1)$

 $ {{y^2} = 2y\dfrac{{dy}}{{dx}} \times x} $  

 $ {{y^2} - 2xy\dfrac{{dy}}{{dx}} = 0}$ 

(iii) Form differential equation of family of circles passing through origin and whose Centre lie on x-axis.

Ans:

Equation of circle is $ \left( {x{\text{ }} - {\text{ }}a} \right){\text{ }} + {\text{ }}y{\text{ }} = {\text{ }}a{\text{ }}...{\text{ }}\left( 1 \right)$  ( ' a' arbitrary constant)

Differentiate with respect to 'x'

$2(x - a) + 2y\dfrac{{dy}}{{dx}} = 0$

$(x - a) + y\dfrac{{dy}}{{dx}} = 0 \Rightarrow a = x + y\dfrac{{dy}}{{dx}}$

Substituting in (1) ${y^2}{\left( {\dfrac{{dy}}{{dx}}} \right)^2} + {y^2} = {\left( {x + y\dfrac{{dy}}{{dx}}} \right)^2}$

${y^2}{\left( {\dfrac{{dy}}{{dx}}} \right)^2} + {y^2} = {x^2} + 2xy\dfrac{{dy}}{{dx}} + {y^2}{\left( {\dfrac{{dy}}{{dx}}} \right)^2}$

${x^2} + 2xy\dfrac{{dy}}{{dx}} - {y^2} = 0$

(iv) Form the differential equation of the family of circles in the first quadrant and touching the coordinate axes.

Ans:

Let the equation of the circle be

${(x - a)^2} + {(y - a)^2} = {a^2}$

$ \Rightarrow {x^2} + {y^2} - 2a(x + y) + {a^2} = 0$

$ \Rightarrow 2x + 2y - 2a\left( {1 + {y_1}} \right) = 0$

$ \Rightarrow x + y - a\left( {1 + {y_1}} \right) = 0$

$ \Rightarrow a = \dfrac{{x + y}}{{1 + {y_1}}}$

Hence, the required differential equation is

${x^2} + {y^2} - 2\left( {\dfrac{{x + y}}{{1 + {y_1}}}} \right)(x + y) + {\left( {\dfrac{{x + y}}{{1 + {y_1}}}} \right)^2} = 0$

$ \Rightarrow {\left( {1 + {y_1}} \right)^2}\left( {{x^2} + {y^2}} \right) - 2{(x + y)^2}\left( {1 + {y_1}} \right)$$ + {(x + y)^2} = 0$

10. Show that the differential equation $ \dfrac{{dy}}{{dx}} = \dfrac{{x + 2y}}{{x - 2y}}$  is homogeneous and solve it.

Ans:

Step 1: Find $\dfrac{{dy}}{{dx}}$

${(x - 2y)\dfrac{{dy}}{{dx}} = x + 2y} $  

${\dfrac{{dy}}{{dx}} = \left( {\dfrac{{x + 2y}}{{x - 2y}}} \right)} $

Step 2: Put ${\text{F}}(x,y) = \dfrac{{dy}}{{dx}}{\text{ }}$ Find ${\text{F}}(\lambda x,\lambda y)$

$\dfrac{{dy}}{{dx}} = \left( {\dfrac{{x + 2y}}{{x - 2y}}} \right)$

Put ${\text{F}}(x,y) = \left( {\dfrac{{x + 2y}}{{x - 2y}}} \right)$

Finding

 ${{\text{ F}}(\lambda {\mathbf{x}},\lambda {\mathbf{y}})}$

 ${{\text{F}}(\lambda x,\lambda y)}$ ${ = \dfrac{{\lambda x + 2(\lambda y)}}{{\lambda x - 2\lambda y}}}$  

  ${ = \dfrac{{\lambda (x + 2y)}}{{\lambda (x - 2y)}}}$  

  ${ = \dfrac{{(x + 2y)}}{{x - 2y}}}$  

  ${ = {\text{F}}(x,y)}$

Thus, $\text{ F}(\lambda x,\lambda y)\text{ }=\text{F}(x,y)=\lambda {}^\circ \text{F}(x,y)$

Thus, $F(x,y)$ is Homogeneous function of degree zero

Therefore, the given Differential Equation is Homogeneous

differential Equation

Step 3: Solving $\dfrac{{dy}}{{dx}}$ by Putting $y = vx$

$\dfrac{{dy}}{{dx}} = \left( {\dfrac{{x + 2y}}{{x - 2y}}} \right)$

Let $y = vx$

So, $\dfrac{{dy}}{{dx}} = \dfrac{{d(vx)}}{{dx}}$

  ${\dfrac{{dy}}{{dx}} = \dfrac{{dv}}{{dx}} \cdot x + v\dfrac{{dx}}{{dx}}} $  

 $ {\dfrac{{dy}}{{dx}} = \dfrac{{dv}}{{dx}}x + v}$ 

Putting in eqn, (1)

  ${{\text{ }}\dfrac{{dy}}{{dx}}{\text{ , }}\dfrac{y}{x}{\text{ }}} $  

  ${\dfrac{{dy}}{{dx}} = \dfrac{{x + 2y}}{{x - 2y}}} $  

  ${\dfrac{{dv}}{{dx}}x + v = \dfrac{{x + 2vx}}{{x - 2vx}}} $  

  ${\dfrac{{dv}}{{dx}}x + v = \dfrac{{x(1 + 2v)}}{{x(1 - 2v)}}} $  

  ${\dfrac{{dv}}{{dx}}x + v = \dfrac{{1 + 2v}}{{1 - 2v}}} $  

  ${\dfrac{{dv}}{{dx}}x = \dfrac{{1 + 2v}}{{1 - 2v}} - v} $  

 $ {\dfrac{{dv}}{{dx}} \cdot x = \dfrac{{1 + 2v - v + 2{v^2}}}{{1 - 2v}}}$ 

  ${\dfrac{{dv}}{{dx}} \cdot x = \dfrac{{2{v^2} + v + 1}}{{1 - 2v}}} $  

 $ {\dfrac{{dv}}{{dx}}x =  - \left( {\dfrac{{2{v^2} + v + 1}}{{2v - 1}}} \right)} $  

 $ {dv\left( {\dfrac{{2v - 1}}{{2{v^2} + v + 1}}} \right) = \dfrac{{ - dx}}{x}} $

Integrating Both Sides

  ${\int {\dfrac{{2v - 1}}{{2{v^2} + v + 1}}} dv = \int {\dfrac{{ - dx}}{x}} } $  

$  {\int {\dfrac{{2v - 1}}{{2{v^2} + v + 1}}} dv =  - \int {\dfrac{{dx}}{x}} } $  

  ${\int {\dfrac{{(2v - 1)dv}}{{2{v^2} + v + 1}}} dv =  - \log |x| + c}$ 

By equating

$\log \left| {{x^2} + xy + {y^2}} \right| = 2\sqrt 3 {\tan ^{ - 1}}\left( {\dfrac{{x + 2y}}{{\sqrt {3x} }}} \right) + c$

12. Solve the following differential equations:

(i) $\dfrac{{dy}}{{dx}} - 2y = \cos 3x$

Ans:

$\dfrac{{dy}}{{dx}} + ( - 2)y = \cos 3x \ldots  \ldots (1)$

This is a linear differential equation of the form

$\dfrac{{dy}}{{dx}} + Py = Q$, where $P =  - 2$ and $Q = \cos 3x$

Multiplying both sides by (1), we get

${e^{ - 2x}}\dfrac{{dy}}{{dx}} - 2y{e^{ - 2x}} = \cos 3x{e^{ - 2x}}$

Integrating both sides w.r.t. $x$, we get

  $ y{{e}^{-2x}}=\int{{{e}^{-2x}}}\cos 3xdx+C  $  

  $  \Rightarrow y{{e}^{-2x}}=I+C,\text{ } $ 

  $  I={{e}^{-tx}}\cos 3x\ldots . $ 

  $ \text{  }I=\int{{{e}^{-2x}}}\cos 3xdx  $ 

  $=\dfrac{1}{3}{{e}^{-2x}}\sin 3x-\int{\dfrac{(-2)}{3}}{{e}^{-2x}}\sin 3xdx  $ 

  ${ = \dfrac{1}{3}{e^{ - 2x}}\sin 3x + \dfrac{2}{3}\left[ {\dfrac{{ - 1}}{3}{e^{ - 2x}}\cos 3x - \int {( - 2)} {e^{ - 2t}}\left( {\dfrac{{ - \cos 3x}}{3}} \right)dx} \right.} $  

  ${ = \dfrac{1}{3}{e^{ - 2\pi }}\sin 3x + \dfrac{2}{3}\left[ {\dfrac{{ - 1}}{3}{e^{ - 2x}}\cos 3x - \dfrac{2}{3}\int {{e^{ - 2x}}} \cos 3xax} \right]} $  

 $ { = \dfrac{1}{3}{e^{ - 2x}}\sin 3x - \dfrac{2}{9}{e^{ - 2x}}\cos 3x - \dfrac{4}{9}I}$ 

  ${ \Rightarrow \left( {I + \dfrac{4}{9}I} \right) = \dfrac{{{e^{ - 2x}}}}{9}(3\sin 3x - 2\cos 3x)} $  

  ${ \Rightarrow I = \dfrac{{{e^{ - 2x}}}}{{13}}(3\sin 3x - 2\cos 3x)} $  

  ${{\text{  }}} $  

Substituting the value of 1 in (2)

$y{e^{ - 2x}} = \dfrac{{{e^{ - 2x}}}}{{13}}(3\sin 3x - 2\cos 3x) + C$

$y = \dfrac{{3\sin 3x}}{{13}} - \dfrac{{2\cos 3x}}{{13}} + c{e^{2x}}$

(II) $\sin x\dfrac{{dy}}{{dx}} + y\cos x = 2{\sin ^2}x\cos x{\text{ if }}y\left( {\dfrac{\pi }{2}} \right) = 1$

Ans: 

Given

  ${{\text{ }}(\sin x)\dfrac{{dy}}{{dx}} + y\cos x = 2{{\sin }^2}x\cos x} $  

  ${ \Rightarrow \dfrac{1}{{\sin x}}\left[ {(\sin x)\dfrac{{dy}}{{dx}} + y\cos x} \right] = 2{{\sin }^2}x\cos x \times \dfrac{1}{{\sin x}}} $  

  ${ \Rightarrow \dfrac{{dy}}{{dx}} + \left( {\dfrac{{\cos x}}{{\sin x}}} \right)y = 2\sin x\cos x} $  

 ${ \Rightarrow \dfrac{{dy}}{{dx}} + (\cot x)y = 2\sin x\cos x}$ 

This is a first order linear differential equation of the form

$\dfrac{{dy}}{{dx}} + Py = Q$

Here, ${\text{P}} = \cot {\text{x}}$ and ${\text{Q}} = 2\sin {\text{x}}\cos {\text{x}}$

The integrating factor (I.F) of this differential equation is,

I. $F = {e^{\int P dx}}$

$ \Rightarrow $ I. $F = {e^{\int {\cot } xdx}}$

We have

 $ {{\text{ }}\int {\cot } xdx = \log (\sin x) + c} $  

  ${ \Rightarrow {\text{ I}}{\text{. }}F = {e^{\log (\sin x)}}} $  

 $ {\therefore {\text{ I}}{\text{. F}} = \sin {\text{x}}\left[ {\because {{\text{e}}^{\log {\text{x}}}} = {\text{x}}} \right]} $  

  ${\text{ }} $

Hence, the solution of the differential equation is,

$y($ I. $F) = \int {(Q \times I.F)} dx + c$

$ \Rightarrow {\text{y}}(\sin {\text{x}}) = \int {(2\sin {\text{x}}\cos {\text{x}} \times \sin {\text{x}})} {\text{dx}} + {\text{c}}$

$ \Rightarrow {\text{y}}\sin {\text{x}} = 2\int {{{\sin }^2}} {\text{x}}\cos {\text{xdx}} + {\text{c}}$

Let $\sin x = t$

$ \Rightarrow \cos xdx = $ dt (Differentiating both sides)

By substituting this in the above integral, we get

  ${yt = 2\int {{t^2}} dt + c} $  

  ${{\text{ }}\int {{x^n}} dx = \dfrac{{{x^{n + 1}}}}{{n + 1}} + c} $  

  ${ \Rightarrow yt = 2\left( {\dfrac{{{t^{2 + 1}}}}{{2 + 1}}} \right) + c} $  

  ${ \Rightarrow yt = 2\left( {\dfrac{{{t^3}}}{3}} \right) + c} $  

  ${ \Rightarrow yt = \dfrac{{2{t^3}}}{3} + c} $  

$  { \Rightarrow y = \dfrac{{2{t^2}}}{3} + \dfrac{c}{t}} $

 $ { \Rightarrow {\text{y}} = \dfrac{{2{{(\sin {\text{x}})}^2}}}{3} + \dfrac{{\text{c}}}{{\sin {\text{x}}}}[\because {\text{t}} = \sin {\text{x}}]} $  

 $ {\therefore {\text{y}} = \dfrac{2}{3}{{\sin }^2}{\text{x}} + {\text{c}}\operatorname{cosec} {\text{x}}} $  

$  {{\text{  }}} $

Thus, the solution of the given differential equation is

${\text{y}} = \dfrac{2}{3}{\sin ^2}{\text{x}} + {\text{c cosec x}}$

(III) $3{e^x}\tan ydx + \left( {1 - {e^x}} \right){\sec ^2}ydy = 0$

Ans:

The given differential equation is

$3{e^x}\tan ydx + \left( {1 - {e^x}} \right){\sec ^2}ydy = 0$

$\dfrac{{3{e^x}}}{{\left( {1 - {e^x}} \right)}}dx = \dfrac{{{{\sec }^2}y}}{{\tan y}}dy$

On Integrating, we get

$\int {\dfrac{{3{e^x}}}{{\left( {1 - {e^x}} \right)}}} dx = \int {\dfrac{{{{\sec }^2}y}}{{\tan y}}} dy$

$ - 3\log \left| {1 - {e^x}} \right| = \log |\tan y| + c$

$\tan y = k{\left( {1 - {e^x}} \right)^3}$ which is the required solution of the given differential equation.

13. Solve the following differential equations:

(i) $ ({x^3} + {y^3})dy - {x^2}ydx = 0$  

Ans:

 $ ({x^3} + {y^3})dy - {x^2}ydx = 0$ 

 ${ \Rightarrow \dfrac{{dy}}{{dx}} = \dfrac{{{x^2}y}}{{{x^3} + {y^3}}}} $  

 $ { \Rightarrow \dfrac{{dy}}{{dx}} = \dfrac{1}{{\dfrac{x}{y} + \dfrac{{{y^2}}}{{{x^2}}}}}} $  

  ${{\text{ Let }}\dfrac{y}{x} = v \Rightarrow y = vx \Rightarrow \dfrac{{dy}}{{dx}} = v + x\dfrac{{dv}}{{dx}}} $  

  ${\text{ }}$ 

So,our differential equations becomes

  ${\text{ }} $  

  ${v + x\dfrac{{dv}}{{dx}} = \dfrac{1}{{\dfrac{1}{v} + {v^2}}} = \dfrac{v}{{1 + {v^3}}}} $  

  ${ \Rightarrow x\dfrac{{dv}}{{dx}} = \dfrac{v}{{1 + {v^3}}} - v =  - \dfrac{{{v^4}}}{{1 + {v^3}}}} $  

 $ { \Rightarrow  - \left( {\dfrac{{1 + {v^3}}}{{{v^4}}}} \right)dv = \dfrac{{dx}}{x}}$ 

Integrating both sides

$ \Rightarrow  - \left( {\dfrac{{1 + {v^3}}}{{{v^4}}}} \right)dv = \dfrac{{dx}}{x}$

  ${{\text{  }}} $  

  ${ \Rightarrow \int  -  \left( {\dfrac{{1 + {v^3}}}{{{v^4}}}} \right)dv = \int {\dfrac{{dx}}{x}} } $  

  ${ \Rightarrow \int  -  \left( {{v^{ - 4}} + \dfrac{1}{v}} \right)dv = \int {\dfrac{{dx}}{x}} } $  

  ${ \Rightarrow \dfrac{{{v^{ - 3}}}}{3} - \log v = \log x + c} $  

  ${ \Rightarrow \dfrac{1}{{3{v^3}}} - c = \log x + \log v} $  

 $ { \Rightarrow \dfrac{1}{{3v_0^3}} - c = \log (vx)} $  

  ${ \Rightarrow \dfrac{{{x^3}}}{{3{y^3}}} = \log y + c{\text{, }}}$

Which is required solution.

(ii) $xdy - ydx = \sqrt {{x^2} + {y^2}} dx$

Ans:

 $ {{\text{ Given }}} $  

 ${xdy - ydx = {{\sqrt x }^2} + {y^2}dx} $  

 ${xdy = \left( {y + \sqrt {{x^2} + {y^2}} } \right)dx \Rightarrow \dfrac{{dy}}{{dx}} = \dfrac{{y + \sqrt {{x^2} + {y^2}} }}{x}}$ 

$\text{ }\!\!~\!\!\text{ }$$F(x,y)=\dfrac{y+\sqrt{{{x}^{2}}+{{y}^{2}}}}{x}$

$F(\lambda x,\lambda y)=\dfrac{\lambda y+\sqrt{{{\lambda }^{2}}{{x}^{2}}+{{\lambda }^{2}}{{y}^{2}}}}{\lambda x}$

$  =\dfrac{\lambda \left\{ y+\sqrt{{{x}^{2}}+{{y}^{2}}} \right\}}{\lambda x} $ 

$  =\lambda {}^\circ .F(x,y) $ 

${\text{F}}({\text{x}},{\text{y}})$ is a homogeneous function of degree zero.

Now, $\dfrac{{dy}}{{dx}} = \dfrac{{y + \sqrt {{x^2} + {y^2}} }}{x}$

Let $\quad y = vx$

$ \Rightarrow \quad \dfrac{{dy}}{{dx}} = v + x \cdot \dfrac{{dv}}{{dx}}$

Putting above value, we have

$  v+x\cdot \dfrac{dv}{dx}=\dfrac{vx+\sqrt{{{x}^{2}}+{{v}^{2}}{{x}^{2}}}}{x} $ 

$  v+x\cdot \dfrac{dv}{dx}=v+\sqrt{1+{{v}^{2}}} $ 

$  \Rightarrow \quad x\cdot \dfrac{dv}{dx}=\sqrt{1+{{v}^{2}}} $ 

$  \dfrac{dx}{x}=\dfrac{dv}{\sqrt{1+{{v}^{2}}}} $ 

Integrating both sides, we get

$\int {\dfrac{{dx}}{x}}  = \int {\dfrac{{dv}}{{\sqrt {1 + {v^2}} }}} $

  $ \log x+\log c=\log \left| v+\sqrt{1+{{v}^{2}}} \right|\left[ \because \int{\dfrac{dx}{\sqrt{{{x}^{2}}+{{a}^{2}}}}}=\log \left| x+\sqrt{{{x}^{2}}+{{a}^{2}}} \right|+c \right]  $ 

$  cx=v+\sqrt{1+{{v}^{2}}}\quad  $ 

$  \Rightarrow \quad cx=\dfrac{y}{x}+\sqrt{1+\dfrac{{{y}^{2}}}{{{x}^{2}}}} $ 

$  cx=\dfrac{y}{x}+\dfrac{\sqrt{{{x}^{2}}+{{y}^{2}}}}{x} $ 

$  \Rightarrow \quad c{{x}^{2}}=y+\sqrt{{{x}^{2}}+{{y}^{2}}} $ 

11. Show that the differential equation $\left( {{x^2} + 2xy - {y^2}} \right)dx + \left( {{y^2} + 2xy - {x^2}} \right)dy = 0$

is homogeneous and solve it.

Ans:

  ${\left( {{x^2} + 2xy - {y^2}} \right) \cdot dx + \left( {{y^2} + 2xy - {x^2}} \right) \cdot dy = 0} $  

  ${{\text{ or}}{\text{. }}\dfrac{{dy}}{{dx}} = \dfrac{{({y^2} - 2xy - {x^2})}}{{\left( {{y^2} + 2xy - {x^2}} \right)}}} $  

 $ {{\text{ y}} = {\text{V}} \cdot {\text{X}}} $  

  ${\dfrac{{dy}}{{dx}} = v.1 + x \cdot \dfrac{{dv}}{{dx}}}$ 

  $ v+x\cdot \dfrac{dv}{dx}=\dfrac{{{v}^{2}}\cdot {{x}^{2}}-2v{{x}^{2}}-{{x}^{2}}}{{{v}^{2}}\cdot {{x}^{2}}+2v{{x}^{2}}-{{x}^{2}}}  $ 

  $ =\left( {{v}^{2}}-2v-1 \right)/\left( {{v}^{2}}+2v-1 \right)  $ 

  $ x\cdot \dfrac{dv}{dx}=\dfrac{{{v}^{2}}-2v-1}{{{v}^{2}}+2v-1}  $ 

  ${x \cdot \dfrac{{dv}}{{dx}} = \dfrac{{{v^2} - 2v - 1 - {v^3} - 2{v^2} + v}}{{{v^2} + 2v - 1}}} $  

  ${{\text{ or}}{\text{. }}\left[ {\dfrac{{{v^ \wedge }2 + 2v - 1}}{{ - {v^3} - {v^2} - v - 1}}} \right] \cdot dv = \dfrac{1}{x} \cdot dx} $  

$\dfrac{{{v^2} + 2v - 1}}{{v + 1}} \cdot \left( {{v^2} + 1} \right) = \dfrac{A}{{v + 1}} + \dfrac{{Bv + C}}{{\left( {{v^2} + 1} \right)}}$

 Equating the coeff. of ${v^2},v$ and constant terms.

$A+B=1\ldots \ldots \ldots \ldots \ldots ..(1)$

$B+C=2\ldots \ldots \ldots \ldots \ldots ..(2)$

$A+C=-1\ldots \ldots \ldots \ldots \ldots ..(3)$

Subtracting eqn. (2) from (1)

$A - C =  - 1 \ldots  \ldots  \ldots  \ldots ..{\text{ (4) }}$

 by eqn. (3) and (4) $A =  - 1,C = 0$

But

$  A+B=1.\text{ } $ 

$  \text{ }-1+B=1=>B=2 $ 

$\left[ {\dfrac{{ - 1}}{{v + 1}} + \dfrac{{2v}}{{{v^2} + 1}}} \right] \cdot dv =  - \dfrac{1}{x} \cdot dx$

or. Integ.of $\left[ {1/(v + 1) - 2v/\left( {{v^ \wedge }2 + 1} \right)} \right] \cdot dv = $ integ. of $1/x.dx$

or. $\log (v + 1) - \log \left( {{v^{}} + 1} \right) = \log x + \log C$

or. $\log (v + 1)/\left( {{v^2} + 1} \right) = \log x.C$

(IV) ${x^2}dy + y(x + y)dx = 0{\text{  }}$given that $y = 1{\text{ }}$when$x = 1.{\text{ }}$

 Ans:

Given, ${x^2}dy + \left( {xy + {y^2}} \right)dx = 0$

$\dfrac{{dy}}{{dx}} = \dfrac{{ - \left( {xy + {y^2}} \right)}}{{{x^2}}}$

Put $\quad y = vx$

or $\dfrac{{dy}}{{dx}} = v + x\dfrac{{dv}}{{dx}}$

The differential equation becomes

$v + x\dfrac{{dv}}{{dx}} =  - \left( {v + {v^2}} \right)$

or $\quad \dfrac{{dv}}{{{v^2} + 2v}} =  - \dfrac{{dx}}{x}$

or $\int {\dfrac{{dv}}{{{{(v + 1)}^2} - {1^2}}}}  =  - \int {\dfrac{{dx}}{x}} $

or. $\dfrac{1}{2}\log \dfrac{v}{{v + 2}} =  - \log x + \log C$

or $\quad \dfrac{{\text{C}}}{x} = \sqrt {\dfrac{y}{{y + 2x}}} $

 $ {{\text{ If }}x = 1,y = 1,{\text{  }}C = \dfrac{1}{{\sqrt 3 }}} $  

 ${{\text{ or }}} $  

 ${\dfrac{1}{{\sqrt 3 x}} = \sqrt {\dfrac{y}{{y + 2x}}} }$ 

(V) $x{e^{\dfrac{y}{x}}} + y + x\dfrac{{dy}}{{dx}} = 0{\text{  }}$if$y(e) = 0$

Ans: Given differential equation is, $\left( {x{e^{\dfrac{y}{x}}} + y} \right)dx = xdy$

$ \Rightarrow \dfrac{{dy}}{{dx}} = \dfrac{{x \cdot {e^{\dfrac{y}{x}}} + y}}{x} \ldots ..({\text{i}})$

Let $F(x,y) = \dfrac{{x \cdot {e^{\dfrac{y}{x}}} + y}}{x}$

$\therefore F(\lambda x,\lambda y) = \dfrac{{\lambda x \cdot {e^{\dfrac{{\lambda y}}{{\lambda x}} + \lambda y}}}}{{\lambda x}} = {\lambda ^0}\dfrac{{x \cdot {e^{\dfrac{y}{x}}} + y}}{x} = {\lambda ^0}F(x,y)$

Hence, given differential equation (i) is homogenous.

Let ${\text{y}} = {\text{vx}} \Rightarrow \dfrac{{dy}}{{dx}} = v + x \cdot \dfrac{{dv}}{{dx}}$

Now, given differential equation (i) would become

 $ {v + x \cdot \dfrac{{dv}}{{dx}} = \dfrac{{x \cdot {e^{\dfrac{{vx}}{x} + vx}}}}{x}} $  

 $ { \Rightarrow v + x \cdot \dfrac{{dv}}{{dx}} = {e^v} + v} $  

  ${ \Rightarrow x \cdot \dfrac{{dv}}{{dx}} = {e^v}} $  

  ${\dfrac{{dv}}{{{e^v}}} = \dfrac{{dx}}{x}} $  

 $ { \Rightarrow \int {{e^{ - v}}} dv = \int {\dfrac{{dv}}{v}} } $  

  ${ \Rightarrow \dfrac{{{e^{ - v}}}}{{ - 1}} = \log x + C} $  

 $ { - {e^{ - \dfrac{y}{x}}} = \log x + C} $  

  ${ \Rightarrow  - \dfrac{1}{{{e^{\dfrac{y}{x}}}}} = \log x + C} $  

$  { \Rightarrow {e^{\dfrac{y}{x}}} \cdot \log x + C{e^{\dfrac{y}{x}}} + 1 = 0} $

Putting $y(e) = 0$, we get

$\therefore {1^{\dfrac{y}{x}}}\log x + C{1^{\dfrac{y}{x}}} + 1 = 0$

$ \Rightarrow \quad C =  - \dfrac{1}{e}$

 The required particular solution is

${e^{\dfrac{y}{x}}} \cdot \log x - \dfrac{1}{e}{e^{\dfrac{y}{x}}} + 1 = 0$

or ${e^{\dfrac{y}{x}}}\log x - {e^{\dfrac{y}{x} - 1}} + 1 = 0$

(VI) $\left( {{x^3} - 3x{y^2}} \right)dx = \left( {{y^3} - 3{x^2}y} \right)dy$

Ans:

Given differential equation is

 $ {\left( {{x^3} - 3x{y^2}} \right)dx = \left( {{y^3} - 3{x^2}y} \right)dy} $  

 $ { \Rightarrow \dfrac{{dy}}{{dx}} = \dfrac{{{x^3} - 3x{y^2}}}{{{y^3} - 3{x^2}y}}} $  

 $ { = \dfrac{{1 - 3{{\left( {\dfrac{y}{x}} \right)}^2}}}{{{{\left( {\dfrac{y}{3}} \right)}^3} - 3\left( {\dfrac{y}{x}} \right)}}} $

  ${\left. { \Rightarrow v + x\dfrac{{dv}}{{dx}} = \dfrac{{1 - 3{v^2}}}{{{v^3} - 3v}},{\text{ Let }}v = \dfrac{y}{x}} \right)} $  

 $ { \Rightarrow x\dfrac{{dv}}{{dx}} = \dfrac{{1 - 3{v^2}}}{{{v^3} - 3v}} - v} $  

 $ { = \dfrac{{1 - 3{v^2} - {v^4} + 3{v^2}}}{{{v^3} - 3v}}} $  

 $ { = \dfrac{{1 - {v^4}}}{{{v^3} - 3v}}} $  

  ${ \Rightarrow \left( {\dfrac{{{v^3} - 3v}}{{1 - {v^4}}}} \right)dv = \dfrac{{dx}}{x}} $  

  ${ \Rightarrow \left( {\dfrac{{{v^3}}}{{1 - {v^4}}}} \right)dv - \left( {\dfrac{{3v}}{{1 - {v^4}}}} \right)dv = \dfrac{{dx}}{x}}$ 

Integrating, we get

$ \Rightarrow \left( {\dfrac{{{v^3}}}{{1 - {v^4}}}} \right)dv - \left( {\dfrac{{3v}}{{1 - {v^4}}}} \right)dv = \dfrac{{dx}}{x}$

Integrating, we get

$ - \dfrac{1}{4}\log \left| {1 - {v^4}} \right| + \dfrac{3}{4}\log \left| {\dfrac{{{v^2} - 1}}{{{v^2} + 1}}} \right| = \log |x| + \log c$

$ - \dfrac{1}{4}\log \left| {1 - {{\left( {\dfrac{y}{x}} \right)}^4}} \right| + \dfrac{3}{4}\log \left| {\dfrac{{{{\left( {\dfrac{y}{x}} \right)}^2} - 1}}{{{{\left( {\dfrac{y}{x}} \right)}^2} + 1}}} \right| = \log |xc|$

which is the required solution

(VII) $\dfrac{{dy}}{{dx}} - \dfrac{y}{x} + \operatorname{cosec} \left( {\dfrac{y}{x}} \right) = 0{\text{  }}$given that $y = 0$ when $x = 1$

Ans:

Differential equation is

$\dfrac{{dy}}{{dx}} = \dfrac{y}{x} - \operatorname{cosec} \left( {\dfrac{y}{x}} \right)$

Let ${\text{F}}({\text{x}},{\text{y}}) = \dfrac{{dy}}{{dx}} = \dfrac{y}{x} - \operatorname{cosec} \left( {\dfrac{y}{x}} \right)$

Finding $F(\lambda x,\lambda y)$

$\text{F}(\lambda \text{x},\lambda \text{y})=\dfrac{\lambda y}{\lambda x}-\operatorname{cosec}\left( \dfrac{\lambda y}{\lambda x} \right)=\dfrac{y}{x}-\operatorname{cosec}\left( \dfrac{y}{x} \right)=\lambda {}^\circ \text{F}(\text{x},\text{y})$

$\therefore {\text{F}}({\text{x}},{\text{y}})$ is $a$ homogenous function of degree zero

$F(\lambda x,\lambda y)=\lambda {}^\circ F(x,y)$

Putting $y = vx$

Diff w.r.t. ${\text{x}}$

$\dfrac{{dy}}{{dx}} = x\dfrac{{dv}}{{dx}} + v$

Putting value of $\dfrac{{dy}}{{dx}}$ and $y = vx$ in (1)

  ${\dfrac{{dy}}{{dx}} = \dfrac{y}{x} - \operatorname{cosec} \left( {\dfrac{y}{x}} \right)} $  

  ${v + x\dfrac{{dv}}{{dx}} = \dfrac{{vx}}{x} - \operatorname{cosec} \left( {\dfrac{{vx}}{x}} \right)} $  

 ${v + x\dfrac{{dv}}{{dx}} = v - \operatorname{cosec} v}$ 

 $ {\dfrac{{xdv}}{{dx}} =  - \operatorname{cosec} v} $  

 $ {\dfrac{{ - dv}}{{\operatorname{cosec} v}} = \dfrac{{dx}}{x}}$ 

Integrating both sides

$\int {\dfrac{{ - dv}}{{\operatorname{cosec} v}}}  = \int {\dfrac{{dx}}{x}} $

$\int  -  \sin vdv = \log |x| + c$

Put value of $v = \dfrac{y}{x}$

$\cos \dfrac{y}{x} = \log |x| + C$

Putting $x = 1{\text{ }}y = 0$

 $ {\cos \dfrac{0}{1} = \log 1 + C} $  

  ${\qquad 1 = 0 + C\quad [\log 1 = 0]} $

Putting value in (2)

$  c=1 $ 

$  \cos \dfrac{y}{2}=\log |x|+1 $ 

$  \cos \dfrac{y}{2}=\log |x|+\log e $ 

$  \cos \dfrac{y}{2}=\log |x| $ 

16. Solve the following differential equations:

(I)$ {\cos ^2}x\dfrac{{dy}}{{dx}} = \tan x - y$ 

Ans:

Given differential equation is

 ${{{\cos }^2}x \cdot \dfrac{{dy}}{{dx}} + y = \tan x} $  

 $ { \Rightarrow \dfrac{{dy}}{{dx}} + y{{\sec }^2}x = \tan x \cdot {{\sec }^2}x} $

Given differential equation is a linear differential equation of the type $ \dfrac{{dy}}{{dx}} + py = Q$ 

I.F. $ = {e^{\int P dx}} = {e^{\int {{{\sec }^2}} xdx}} = {e^{\tan x}}$

$\therefore \quad $ Solution is given by ${e^{\tan x}}y = \int {\tan } x \cdot {\sec ^2}x \cdot {e^{\tan x}}dx$

Let $I = \int {\tan } x \cdot {\sec ^2}x \cdot {e^{\tan x}}dx$

Let $\tan x = t,\quad {\sec ^2}xdx = dt$

$ \Rightarrow I = \int t {e^t}dt$

Integrating by parts $\therefore \quad I = t{e^t} - \int {{e^t}} dt = t{e^t} - {e^t} + C$

$ \Rightarrow I = \tan x{e^{\tan x}} - {e^{\tan x}} + C$,

Hence ${e^{\tan x}}y = {e^{\tan x}}(\tan x - 1) + C$

$ \Rightarrow y = \tan x - 1 + C{e^{ - \tan x}}$

(II) $x\cos x\dfrac{{dy}}{{dx}} + y(x\sin x + \cos x) = 1$.

Ans:

Given $x\cos x(dy/dx) + y(x\sin x + \cos x) = 1$.

$\dfrac{{dy}}{{dx}} + \dfrac{{y(x\sin x + \cos x)}}{{x\cos x}} = \dfrac{1}{{x\cos x}}$

$\dfrac{{dy}}{{dx}} + {\left( {\dfrac{{x\sin x}}{{x\cos x}} + \dfrac{{\cos x}}{{x\cos x}}} \right)^y} = \dfrac{1}{{x\cos x}}$

$\dfrac{{dy}}{{dx}} + \left( {\tan x + \dfrac{1}{x}} \right)y = \dfrac{1}{{x\cos x}}$

It is linear differential equation in the form $\dfrac{{dy}}{{dx}} + Py = Q$

where $P = \tan x + (\dfrac{1}{x})$ and $\dfrac{{\sec x}}{x}$

$\therefore ({\text{I}}.{\text{F}}.) = {{\text{e}}^{\int {(\tan x + (} 1/{\text{x}})){\text{dx}}}}$

$ = {{\text{e}}^{\int {\tan } x + \int d x/x}} = {e^{\log \sec x + \log x}} = {e^{\log (x\sec x)}}$

$ = x\sec x$

Now, multiplying (1) by I.F. and integration, we get

$y \times I.F = \int Q  \times I.F + C$

$yx\sec x = \int {(\sec x/x)} x(x\sec x)dx + c$

$xy\sec x = \int {{{\sec }^2}} xdx + c = \tan x + c$

$xy\sec x = \tan x + C$

Which is the required solution

(III).$\left( {1 + {e^{\dfrac{x}{y}}}} \right)dx + {e^{\dfrac{x}{y}}}\left( {1 - \dfrac{x}{y}} \right)dy = 0$

Ans:

 $ {\left( {1 + {e^{\dfrac{x}{y}}}} \right)dx = \left( {\dfrac{x}{y} - 1} \right){e^{\dfrac{x}{y}}}dy} $  

 $ {\dfrac{{dx}}{{dy}} = \dfrac{{\left( {\dfrac{x}{y} - 1} \right){e^{\dfrac{x}{y}}}}}{{\left( {1 + {e^y}} \right)}} = f\left( {\dfrac{x}{y}} \right)} $  

  ${{\text{  }}}$ 

Hence, homogeneous

$\dfrac{{dx}}{{dy}} = \dfrac{{\left( {\dfrac{x}{y} - 1} \right){e^{\dfrac{x}{y}}}}}{{\left( {1 + {e^y}} \right)}} = f\left( {\dfrac{x}{y}} \right)$

Equating Homogeneous,

  ${x - vy \Rightarrow \dfrac{{dx}}{{dy}} = v + y\dfrac{{dv}}{{dy}}} $  

  ${v + y\dfrac{{dv}}{{dy}} = \dfrac{{(v - 1){e^\gamma }}}{{1 + {e^p}}}} $  

  ${\int {\dfrac{{1 + {e^v}}}{{{e^v} + v}}} dv =  - \int {\dfrac{{dy}}{y}} } $  

 $ {{{\log }_e}\left| {{e^v} + v} \right| =  - {{\log }_e}|y| + {{\log }_e}C} $  

  ${{{\log }_e}\left| {\left( {{e^v} + v} \right)y} \right| = {{\log }_c}C} $  

  ${\left( {{e^\sigma } + v} \right)y = C = A} $  

 $ {\left( {\dfrac{x}{{{e^y}}} + \dfrac{x}{y}} \right)y = A,} $

$  {{\text{  }}} $

The General solution

(IV) ${\text{  }}(y - \sin x)dx + \tan xdy = 0,y(0) = 0.{\text{ }}$

Ans:

The given diff. equation can be written as

$\dfrac{{dx}}{{dy}} + (\cot x)y = \cos x$

This is linear differential equation.

I.F. $ = {e^{\int {\cot } xdy}} = {e^{\log \sin x}} = \sin x$

The solution is:

  $  y\sin x=\int{\sin }x\cos xdy+C $ 

 $  =\dfrac{1}{2}\int{\sin }2xdy+Cy\sin x $ 

 $  =\dfrac{-1}{4}\cos 2x+C $ 

It is given that , when

  $  c-\dfrac{1}{4}=0\text{ or }c=\dfrac{1}{4} $ 

 $  y\sin x=\dfrac{1}{4}(1-\cos 2x)=\dfrac{1}{2}{{\sin }^{2}}x $ 

 $  2y=\sin x $ 

which is the required solution.

LONG ANSWER TYPE QUESTIONS (6 MARKS EACH)

17. Solve the following differential equations:

(I) $(xdy - ydx)y\sin \left( {\dfrac{y}{x}} \right) = (ydx + xdy)x\cos \left( {\dfrac{y}{x}} \right)$

Ans:

Given Differential equation can be written as

  ${{\text{  }}} $  

  ${(xdy - ydx)y\sin \left( {\dfrac{y}{x}} \right) = (ydx + xdy)x\cos \left( {\dfrac{y}{x}} \right)} $  

 $ {xy\sin \left( {\dfrac{y}{x}} \right)dy - {y^2}\sin \left( {\dfrac{y}{x}} \right)dx} $  

 $ { = yx\cos \left( {\dfrac{y}{x}} \right)dx + {x^2}\cos \left( {\dfrac{y}{x}} \right)dy}$ 

  ${xy\sin \left( {\dfrac{y}{x}} \right)\dfrac{{dy}}{{dx}} - {y^2}\sin \left( {\dfrac{y}{x}} \right)} $  

  ${ = xy\cos \left( {\dfrac{y}{x}} \right) + {x^2}\cos \left( {\dfrac{y}{x}} \right)\dfrac{{dy}}{{dx}}} $  

  ${\dfrac{{dy}}{{dx}} = \dfrac{{{y^2}\sin (y/x) + xy\cos (y/x)}}{{xy\sin (y/x) - {x^2}\cos (y/x)}}}$

Put $(\dfrac{y}{x}) = v$ to get $y = vx$ and $\dfrac{{dy}}{{dx}} = v + x\dfrac{{dy}}{{dx}}$

$v + x\dfrac{{dv}}{{dx}} = \dfrac{{{v^2}\sin v + v\cos v}}{{v\sin v - \cos v}}$

or $x\dfrac{{dv}}{{dx}} = \dfrac{{2v\cos v}}{{v\sin v - \cos v}}$

or $\int {\dfrac{{\cos v - v\sin v}}{{v\cos v}}} dv =  - 2\int {\dfrac{{dx}}{x}} $

or $\log |v\cos v| + \log {x^2}$

$ = \log C$

or ${x^2}v\cos v = C$ or $xy\cos (\dfrac{y}{x}) = C$

(II) 

  ${3{e^x}\tan ydx + \left( {1 - {e^x}} \right){{\sec }^2}ydy = 0{\text{   }}} $  

  ${\text{ }}$ 

Given that $ y = \dfrac{\pi }{4}$ , when $ x = 1$ 

Ans:

The given differential equation is

$3{e^x}\tan ydx + \left( {1 - {e^x}} \right){\sec ^2}ydy = 0$

$\dfrac{{3{e^x}}}{{\left( {1 - {e^x}} \right)}}dx = \dfrac{{{{\sec }^2}y}}{{\tan y}}dy$

On Integrating, we get

$\int {\dfrac{{3{e^x}}}{{\left( {1 - {e^x}} \right)}}} dx = \int {\dfrac{{{{\sec }^2}y}}{{\tan y}}} dy$

$ - 3\log \left| {1 - {e^x}} \right| = \log \operatorname{ltan} y\mid  + c$

By putting 

$ y = \dfrac{\pi }{4}{\text{ }},{\text{ }}and{\text{ }}x = 1$ 

${(1 - e)^3}\tan y = {\left( {1 - {e^x}} \right)^3}$

which is the required solution of the given differential equation.

(III) $\dfrac{{dy}}{{dx}} + y\cot x = 2x + {x^2}\cot x{\text{  }}$Given that $y(0) = 0$

Ans:

$\dfrac{{dy}}{{dx}} + y \cdot \cot x = 2x + {x^2} \cdot \cot x$

Let $P = \cot x,Q = 2x + {x^2}\cot x$

I.F $ = {{\text{e}}^{\int {{\text{pdx}}} }} = {{\text{e}}^{\int {\cot } x\;{\text{dx}}}} = {{\text{e}}^{\int {\log } (\sin {\text{x}})}} = \sin {\text{x}}$

Sol is

$y(I.F) = \int {(Qx\mid .F)} dx + C$

$y\sin x = \int {\left( {2x + {x^2}\cot x} \right)} \sin dx + c$

$ = \int {\left( {2x + {x^2}\cot x} \right)} dx + c$

$ = 2\int x \sin xdx + \int {{x^2}} \cos xdx + c$

$  =2[x(-\cos x)-(1)(-\sin x)]+\left[ {{x}^{2}}\sin x-2x(-\cos x)+2c(-\sin x) \right] $ 

$  =2x\cos x+2\sin x+{{x}^{2}}\sin xy\sin x $ 

$ = {x^2}\sin x + c$

Then Y (0) =0

$Y=x^2$

This is the Required General solution for the given differential Equation.

Significance of Important Questions for Class 12 Maths Chapter 9 Differential Equations

Apart from the exercises given in the NCERT textbook, these important questions will act as the perfect guide to learning how to use the concepts of differential equations and calculus to solve.

Students will study the syllabus of this chapter and will learn the concepts, fundamental principles and formulas of differential equations and will proceed to complete the syllabus accordingly. To test their knowledge and mathematical skills, they will need a vivid platform. This platform is provided with these important questions. They can solve and compare their answers to the solutions provided and find out where they need to study more.

Benefits of Important Questions for Class 12 Maths Chapter 9 Differential Equations

• Use these important questions as an evaluation tool for your preparation.

• Learn to efficiently use time and solve these questions.

• Resolve doubts faster by using the solutions provided.

• Focus on the stepwise answering formats and score more.

Download Important Questions for Class 12 Maths Chapter 9 Differential Equations PDF

Get the free PDF version of these important questions and answers to complete your study material. Learn to manage time and build focus while solving these problems. Follow the solutions of these answers to develop your answering skills.

Important Related Links for CBSE Class 12 Maths