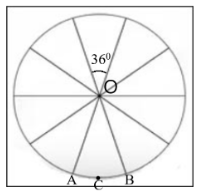
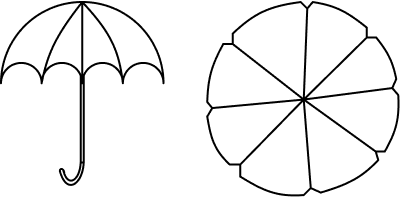
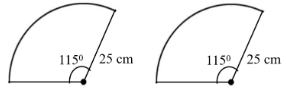
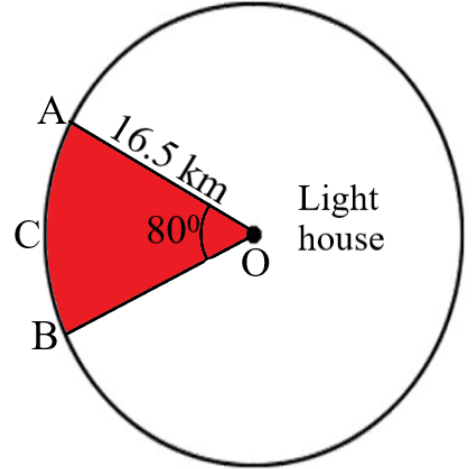
NCERT Solutions for Class 10 Maths Chapter 11 Exercise 11.1 - FREE PDF Download
NCERT Solutions for Class 10 Maths Chapter 11 Areas Related To Circles Ex 11.1
FAQs on NCERT Solutions for Class 10 Maths Chapter 11 Areas Related To Circles Ex 11.1
1. What is the formula for the area of a circle in 11.1 Class 10 Maths NCERT Solutions?
The formula for the area of a circle is A=πr², where r is the radius of the circle.
2. What is a segment of a circle, and how is its area calculated?
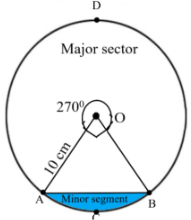
A segment of a circle is covered in Class 10 Ch 11 Maths Ex 11.1 and it is the region between a chord and the corresponding arc. Its area is found by subtracting the area of the triangle formed by the chord and the radius from the area of the sector.
3. What is the relationship between the radius, diameter, and circumference of a circle?
According to Class 10th Maths Chapter 11 Exercise 11.1, The radius is half the diameter, and the circumference is the distance around the circle. The relationships are given by C=2πr and D=2r, where CC is the circumference, r is the radius, and D is the diameter.
4. Can you give an example of a real-life application of calculating the area of a sector?
One real-life application is calculating the area of a slice of pizza, which is essentially a sector of a circle. By knowing the radius of the pizza and the angle of the slice, one can determine the area of the slice using the sector area formula. This is how Ex 11.1 Class 10 NCERT Solutions helps students in real world scenarios.
5. In which questions of exercise 11.1 of 10th Maths, students face difficulty while solving?
Exercise 11.1 of Class 10 Maths deals with Circles. It is a very important and comparatively easy and interesting chapter in the NCERT syllabus from which the students can score good marks. However, a few questions in Exercise 11.1 are a bit difficult for the students to solve. These are questions 3, 7, 9, 10, and 13. One must carefully analyse and understand these five questions to be able to solve them and also refer to the NCERT Solutions for Class 10 maths for help.
6. Which questions are easy in Exercise 11.1 of 10th Maths?
Exercise 11.1 from NCERT Maths Class 10 is a vital exercise that will give the students a potential edge to score well in the board exams. In this exercise, most of the questions are quite easy to understand and solve. The problems that are easy and therefore the favourites of students are 1, 2, 4, 5, 6, 8, 11, 12 and 14. Students enjoy solving these questions.
7. Is Exercise 11.1 important?
Exercise 11.1 of Class 10 Maths Chapter 11 is an important exercise in Class 10. This exercise deals with the questions related to the area of circles. These topics form a major part of the CBSE examination and also a part of the study curriculum in higher classes. Students must be clear with this chapter to ace their examinations. If you want proper guidance, refer to NCERT Solutions for Class 10 Maths for help.
8. From where can I download the NCERT Solutions for Exercise 11.1 of 10th Maths?
The NCERT Solutions for Exercise 11.1 of Class 10 Maths is available free of cost on the Vedantu website (vedantu.com). If you want to download CBSE NCERT Solutions for Exercise 11.1 of 10th Maths, you can follow these steps:
1. Click NCERT Solutions for Class 10 Maths.
2. The next page will contain the Exercise 11.1 Solutions PDF.
3. Click on it, and it will redirect you to the next page, where you will find the link to download.
9. How to get full marks in Class 10 Maths?
The only way to get full marks in Maths is by regular practice. Firstly, you will have to understand the concept of the chapter and then start solving the exercise questions. You can take help from Vedantu. Once you get acquainted with the topic, you can repeatedly practise the NCERT Solutions for Class 10 Maths to bring in the accuracy and eventually score full marks in the exam.























 Watch Video
Watch Video