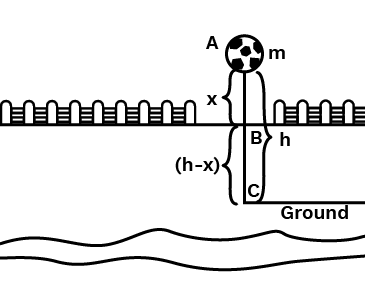
CBSE Science Chapter 10 Work And Energy Class 9 Notes: FREE PDF Download






Work and Energy Class 9 Notes: CBSE Science Chapter 10
FAQs on Work and Energy Class 9 Notes: CBSE Science Chapter 10
1. How are Energy and Work Related to Physics?
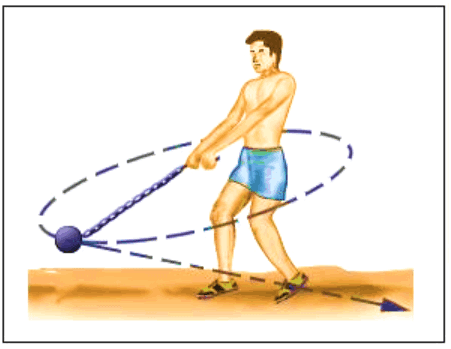
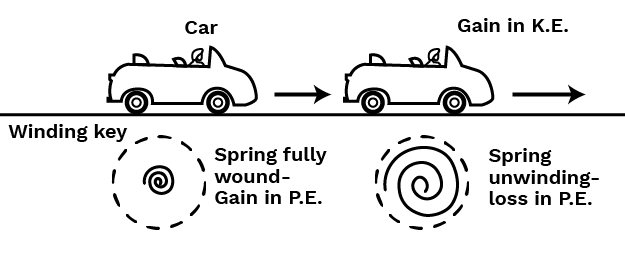
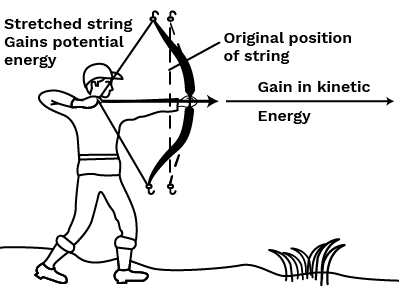
Energy is very closely related to the work. The principle of work-energy states that the increase in the kinetic energy of the rigid body is caused by a positive work with an equal amount, which is done on a body by the resultant force.
2. Why Does Work-energy Have the Same Units?
This is due to the change in objects' kinetic energy. As energy is conserved we can notice that the work which is done on the object is equivalent to the energy supplied to the same object. So here we can conclude work is a type of energy and it is measured in the same units that are Joules.
3. Do Transfers of Energy Take Place When Work is Done?
Energy can transfer from one to another by performing work. Energy is transferred When work is done from the agent to the object, which results in a change in the motion of the object more specifically we can say a change in the object's kinetic energy.
4. What is the Output Power?
Output Power can be defined as the average rate of electric energy delivery during one interval metering which is converted to an hourly rate of electric energy delivery in the unit kWh per hour.
5. What is covered in the Work And Energy Class 9 Notes PDF?
The Work And Energy Class 9 Notes PDF covers key concepts like work, kinetic energy, potential energy, power, and the law of conservation of energy. It also includes important formulas and examples to help students understand and solve related problems.
6. How can I download the Class 9 Work And Energy Notes?
You can download the Class 9 Work And Energy Notes from Our Vedantu website offering CBSE study materials. These notes are available for free.
7. Why are Work, Power And Energy Class 9 Notes PDFs important for exam preparation?
Work, Power, and Energy Class 9 Notes PDFs are important as they provide simplified explanations of concepts, key formulas, and solved examples. These notes help students revise efficiently and prepare better for their exams.
8. Where can I find solved examples in the Class 9 Work And Energy Notes?
Solved examples can be found in most Class 9 Work And Energy Notes, usually alongside explanations of concepts like work, power, and energy. These examples help students understand how to apply formulas in real-life situations.
9. Are the Work, Power And Energy Class 9 Notes PDF available for offline access?
Yes, once you download the Work, Power And Energy Class 9 Notes PDF, you can access them offline for easy and convenient studying without needing an internet connection.
10. How are the concepts of kinetic and potential energy explained in Work And Energy Notes Pdf Download?
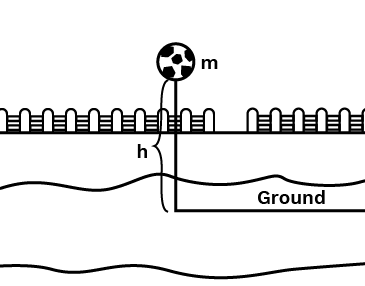
The Work And Energy Notes PDF Download explains kinetic energy as the energy possessed by an object in motion and potential energy as the energy stored due to an object's position or height. Formulas and examples are provided for better understanding.
11. What are the benefits of using Class 9 Work And Energy Notes for revision?
Using Class 9 Work And Energy Notes for revision ensures that students can quickly review key concepts, formulas, and examples. These notes simplify the chapter, making it easier to focus on important points during exam preparation.
12. How can the Work And Energy Notes PDF Download improve problem-solving skills?
The Work And Energy Notes PDF Download includes solved examples and practice questions, helping students apply the concepts of work, power, and energy in numerical problems, thus improving their problem-solving skills.




















 Watch Video
Watch Video