CBSE Class 11 Physics Chapter 10 Thermal Properties of Matter Notes - FREE PDF Download






FAQs on Thermal Properties of Matter Class 11 Notes: CBSE Physics Chapter 10
1. What Do You Mean by the Thermal Properties of Matter?
Thermal properties are the physical properties of matter, which are related to the conductivity of heat.
Thermal properties explain the response of a material, i.e., how it reacts when subjected to varying heat, either excessive heat or low heat.
2. What is Heat Capacity?
We define heat capacity as the heat required to raise the temperature of a body by 1℃. The amount of heat is expressed in Joule or Calories, while the temperature is expressed in Kelvin/Celsius. The heat capacity of a material is calculated by using the following formula:
Q = mCΔT
Where,
Q = heat capacity in Joules
m = mass in grams
C = specific heat in J/K
ΔT = Change in temperature in K
3. Write the Major Components of the Thermal Properties of Matter.
The major components of thermal properties of matter are:
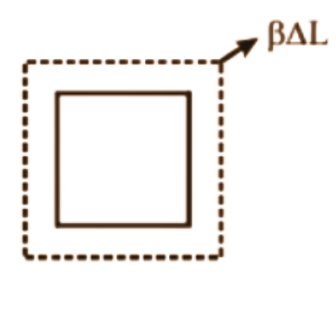
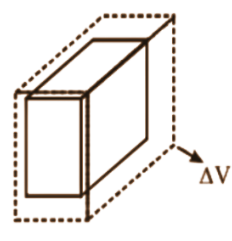
Thermal Expansion
Thermal Stress
Thermal Conductivity
Heat Capacity (Q)
4. How Can I Improve My Numerical Solving Ability in Physics?
Our subject experts suggest the following tips to improve your numerical questions solving ability in Physics.
Go through NCERT numerical-type questions to strengthen your concepts.
Do not jump to a new topic until you’ve solved all the numerical questions related to it.
Start from the basic concepts, and slowly, and gradually move to the advanced topics.
Keep on practising until you gain confidence in your numerical-solving ability.
5. Give the definition of the following:
Thermal Conduction
Coefficient of Thermal Conductivity
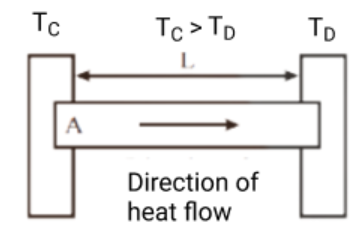
Thermal Conduction –The process of transferring the heat energy from one part of the solid to another part without the actual motion of the particles at a lower temperature is known as thermal conduction or conduction of heat.
Coefficient of Thermal Conductivity –The amount of heat flowing across the opposite faces of the unit cubes per second is known as the coefficient of the thermal conductivity of a material. This flowing of heat takes place when the opposite faces of the unit cubes are maintained at a temperature difference of 1K.
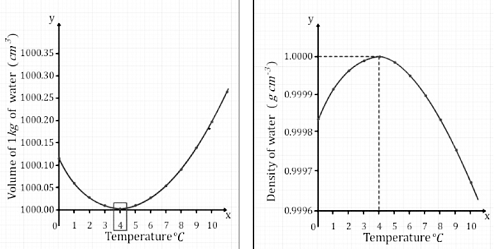
6. Explain why food in a hot case remains warm for a long time during winters.
The hot vessel is double-walled. There is an evacuation in the space between the walls of the hot case. The steel is used in making the food container. So, neither the heat from the food container can escape nor the outside air can enter the food container. There is no chance of loss of heat due to radiation because of the highly polished surface of the food container. This is the reason why food remains warm in hot cases during winters.
7. Write the different properties of the thermometric substances.
The characteristics of the thermometric substances are:
The substances should have low freezing points and high boiling points.
The property of volatility should not be there in the substance.
The thermal conductivity of the substance should be good.
For the sensitivity of the substance, the coefficient of expansion should be high.
Thermometric substances should be available in a pure state.
For easy calibration, there should be uniformity in the rate of expansion.
It should be easily accessible.
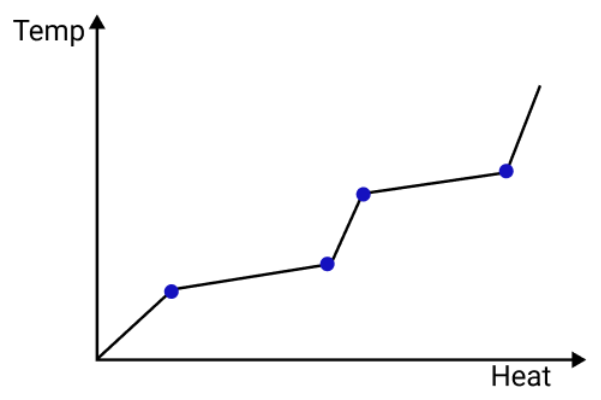
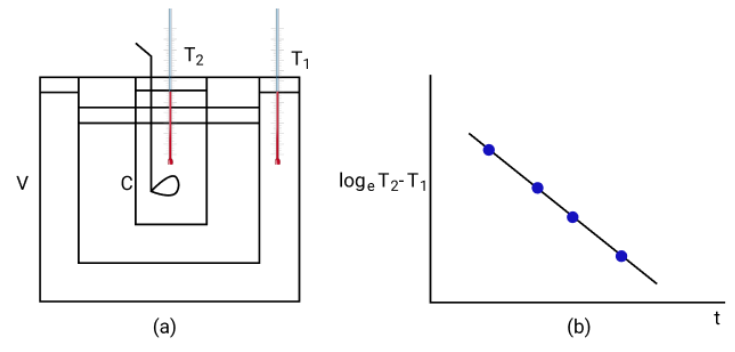
8. What do you mean by Newton's Law of Cooling? Also, define the cooling curve.
Newton's Law of Cooling tells that the temperature difference between the surroundings and the body is directly proportional to the rate of heat loss per unit surface area of a body.
Note: The temperature difference should not be too high.
The cooling curve is described as a graph between the time and the temperature of the body. The rate of fall of the temperature is given by the tangent drawn to the curve at any point.
9. Why do we use cotton threads, plastic or rubber coverings on steering wheels or handles of cycles or scooters?
As we know metals are good conductors of heat, we use them in making steering wheels or handles of scooters and cycles.
During summers, the temperature of these handles is high and in winters, these have low temperatures so in both cases, there will be heat transfer between these handles and the body.
So, to avoid this transferring of heat these handles and steering wheels are covered with insulators like rubber or plastic. By this, the person is protected from heat or cold.
10. Where can I download the Thermal Properties Of Matter PDF Notes for Class 11 Physics?
You can download the Thermal Properties Of Matter PDF Notes from the Vedantu Website, which provides comprehensive coverage of the topic, including important concepts and formulas for better exam preparation.
11. What are the benefits of studying Thermal Properties Of Matter Short Notes?
Thermal Properties Of Matter Short Notes provide concise explanations, making it easier to revise key concepts quickly. They focus on important topics like heat transfer, thermal expansion, and specific heat, helping students save time while reviewing for exams.
12. How can Thermal Properties Of Matter PDF Notes help in exam preparation?
The Thermal Properties Of Matter PDF Notes simplify complex ideas, highlight essential formulas, and offer solved examples. These notes are useful for revising key topics and practising numerical problems, helping students prepare efficiently for their Class 11 Physics exams.