Revision Notes for CBSE Class 11 Physics Chapter 9 (Mechanical Properties of Fluids) - Free PDF Download
As per the latest CBSE syllabus, Class 11 Physics Chapter 9 Mechanical Properties of Fluids comes under Unit 7, i.e., Properties of Bulk Matter. You can expect questions of a total of 20 marks from this unit. The revision notes of Mechanical Properties of Fluids on Vedantu, will help you to get a clear idea of all the important concepts and theories covered in this chapter. Our subject matter experts have listed below the main topics of Chapter - 9 Mechanical Properties of Fluids as per the syllabus of the upcoming CBSE Class 11 exam.
(Table will be uploaded soon)
Download CBSE Class 11 Physics Revision Notes 2024-25 PDF
Also, check CBSE Class 11 Physics revision notes for other chapters:
CBSE Class 11 Physics Chapter-wise Notes | |
Chapter 10 Mechanical Properties of Fluids Notes | |
Mechanical Properties of Fluids Chapter-Related Important Study Materials
It is a curated compilation of relevant online resources that complement and expand upon the content covered in a specific chapter. Explore these links to access additional readings, explanatory videos, practice exercises, and other valuable materials that enhance your understanding of the chapter's subject matter.
Mechanical Properties of Fluids Related Other Study Materials |
Mechanical Properties of Fluids Class 11 Notes Physics - Basic Subjective Questions
Section-A (1 Mark Questions)
1. Why are the cars and Aero planes given streamline shape?
Ans. Cars and aero plans are given streamline shape to minimize the backward drag of atmosphere.
2. We can cut an apple easily with a sharp knife as compared to with a blunt knife. Explain why?
Ans. The area of sharp edge is much less than the area of a blunt edge. For the same total force, the effective force per unit area (or pressure) is more for the sharp edge than the blunt one. Hence a sharp knife cuts easily than a blunt knife.
3. Why two streamlines cannot cross each other?
Ans. If two streamlines cross each other, there will be two directions of flow at the point of intersection which is impossible.
4. Small insects can move on the surface of water. Why?
Ans. Due to surface tension, the free surface of water behaves like stretched elastic membrane which is able to support the small weight of insects and they can move on the surface of water.
5. A force of 100 N is applied on an area of 4 m2. Compute pressure being applied on the area.
Ans. Given, Force = 100N
And Area = 4 m2
So, pressure $=\dfrac{Force}{Area}=\dfrac{100}{4}=25\;Pa$
So, the pressure being applied on the area is 25 Pa.
6. How is a straw able to pull up water when we drink through it?
Ans. When you drink from a straw, you create a little space of low pressure inside your mouth and in the top of the straw. Then the air outside at atmospheric pressure pushes down the surface of the drink and forces the liquid up through the straw and into your mouth.
7. What is atmospheric pressure?
Ans. Atmospheric pressure, also known as ‘air pressure,’ can basically be defined as ‘the exertion of force by the weight of the atmospheric air that is above a particular place or surface’. The atmospheric air contains gases that are pulled down to the earth by the effect of ‘gravity’. The weight of the air will produce an air pressure on the objects in it.
8. What happens if the buoyant force of liquid is equal to or greater than or less than the Ansid object which is to be immersed into the liquid?
Ans. If the buoyant force is more than or equal to the weight of the Ansid object, object will float and if the buoyant force is less than the weight of the object then the object will sink into the liquid.
9. Why should the lubricant oil be of high viscosity?
Ans. Lubricant oil is used to reduce the dry friction between various machine parts. Due to high viscosity, lubricant oil sticks to the machine parts and cannot be squeezed out during the working of the machine.
10. Hotter liquids move faster than colder ones. Why?
Ans. The viscosity or the internal force of friction of a liquid decreases with the increase in temperature. Hence hotter liquids move faster than colder ones.
Section-B (2 Marks Questions)
11. Provide the required explanation for the statement given below.
“It is easier to walk on soft sand if we have flat shoes rather than shoes with sharp heels”.
Ans. A flat shoe has a greater area in contact with the soft sand and exerts less pressure on the soft ground. Due to this, the ‘flat’ shoe do not sink much in soft sand and it is easy to walk on the sand. On the other hand, a sharp heel has a small contact area with the soft sand and thus exerts a greater pressure on the sand. Due to this, the sharp heels sink deep into soft sand and thus making it difficult for the wearer to walk on it.
12. Water is placed in 4 different vessels as shown below in the figure.
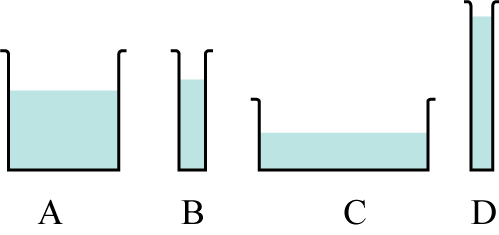
Arrange the vessels in order of increasing pressure applied at the bottom point of the vessel and also state reasons for the same.
Ans. The pressure would be lowest at the bottom in case of vessel C, followed by vessel A, then vessel B and maximum in vessel D. The comparative magnitude of pressure will be:
D > B > A > C
The pressure would be maximum at the bottom point in vessel D because the column of water above the bottom point is the largest in dimensions causing more pressure at the bottom as compared to the rest of the vessels.
13. Define pressure. What are its units? On what factors does the magnitude of pressure depends on?
Ans. Thrust acting on a surface per unit area is called pressure.
Pressure = Force/Area

It's. S.I units is Pascal (Pa)
Pressure depends upon the applied force or weight and the area of contact. Pressure is directly proportional to the thrust and inversely proportional to the area of contact.
14. Two thigh bones (femur), each of cross-sectional area 10 cm2 support the upper part of a human body of mass 40 kg. Estimate the average pressure sustained by the femur.
Ans. Total cross-sectional area of the femur is A = 2 × 10 cm2 = 20 × 10-4 m2.
The force acting on them is F = 40kgwt = 400 N (taking g = 10ms-2).
$P_{av}=\dfrac{F}{A}=2\times 10^{5}Nm^{-2}$
15. A hydraulic automobile lift is designed to lift cars with a maximum mass of 3000 kg. The area of cross-section of the piston carrying the load is 425 cm2. What maximum pressure would the smaller piston have to bear?
Ans. The maximum mass of a car that can be lifted, m = 3000 kg
Area of cross-section of the load-carrying piston, A = 425 cm2 = 425 × 10-4 m2
The maximum force exerted by the load, F = mg
= 3000 × 9.8
= 29400 N
The maximum pressure exerted on the load-carrying piston, $P=\dfrac{F}{A}$
$\dfrac{29400}{425\times 10^{-4}}$
$=6\cdot 917\times 10^{5}\;Pa$
Pressure is transmitted equally in all directions in a liquid. Therefore, the maximum pressure that the smaller piston would have to bear is $6\cdot 917\times 10^{5}\;Pa$ .
16.A glass plate of 0.20 m in area is pulled with a velocity of 0.1 m/s over a larger glass plate that is at rest. What force in necessary to pull the upper plate if the space between them is 0.003m and is filled with oil of η = 0.01 Ns/m2
Ans. $F=\eta A\dfrac{dv}{dy}$
$=0\cdot 01\times 0\cdot 20\times \dfrac{0\cdot 1}{0\cdot 003}=66\cdot 7\times 10^{-3}N$
PDF Summary - Class 11 Physics Mechanical Properties Of Fluids Notes (Chapter 9)
1. Fluid Mechanics:
The term ‘fluids’ are generally used to express both liquids and gases. In other words, it can be said that the substances which have the potential to flow are termed as fluids.
Fluids are assumed to be incompressible (i.e., the density of liquid is not dependent on the variation in pressure and remains constant).
Fluids are also assumed to be non-viscous (i.e., the two liquid surfaces in contact are not pressing any tangential force on each other).
1.1. Fluid Statics:
1.1.1. Fluid Pressure:
Fluid pressure \[\text{p}\] at each point is defined as the normal force acting per unit area. Mathematically,
\[p=\frac{d{{F}_{l}}}{dA}\]
The S.I. unit of pressure is the \[\text{pascal (Pa)}\] and \[1\text{ p}ascal=1N/{{m}^{2}}\]
Fluid force exerts itself perpendicularly to any surface in the fluid, no matter the orientation of that surface. Thus, fluid pressure has no intrinsic direction of its own and can be considered as a scalar quantity.
Pressure:
Pressures at two positions in a horizontal plane or at an equal level when the fluid is at rest or moving with constant velocity are the same.
Pressures at two positions that are separated at a depth of \[\text{h}\] when fluid is at rest of moving with constant velocity are related by the expression:\[{{p}_{2}}-{{p}_{1}}=\rho gh\], where \[\text{ }\!\!\rho\!\!\text{ }\] is the density of liquid.
Pressures at two positions in a horizontal plane when a fluid container is having a constant horizontal acceleration are related by the expression:
\[{{p}_{1}}-{{p}_{2}}=l\rho a\]
Also, \[\tan \theta =a/g\], where \[\text{ }\!\!\theta\!\!\text{ }\] is the angle between the liquid’s free surface and the horizontal.
Pressures at two positions within a liquid at a vertical distance of \[\text{h}\] when the liquid container is accelerating up are related by expression: \[{{p}_{2}}-p{}_{1}=\rho (g+a)h\]
When the container accelerates down, then
\[{{p}_{2}}-p{}_{1}=\rho (g-a)h\].
1.1.2. Atmospheric Pressure
The pressure of the atmosphere of the earth is termed as atmospheric pressure. Normal atmospheric pressure at sea level (an average value) is \[\text{1}\] atmosphere (atm), which is equal to \[\text{1}\text{.013 }\!\!\times\!\!\text{ 1}{{\text{0}}^{\text{5}}}\text{Pa}\].
The pressure which is excess above the atmospheric pressure is termed gauge pressure, and the total pressure is termed absolute pressure.
The barometer is a device utilized to measure the atmospheric pressure whereas a U–tube manometer or simply a manometer is a device utilized to measure the gauge pressure.
1.1.3. Pascal’s Law:
A pressure change, on its application to an enclosed fluid is transmitted undiminished to every portion of the fluid and to the walls of the containing vessel.
Among the many practical applications of Pascal’s law, a popular application is the hydraulic lift.
1.1.4. Archimedes Principle:
Consider a body partially or fully dipped in a fluid. The fluid exerts a contact force on this body. The resultant of all these contact forces is termed buoyant force or upthrust.
\[F=weight\text{ }of\text{ }fluid\text{ }displaced\text{ }by\text{ }the\text{ }body\]
This force is termed buoyant force and it acts vertically upwards (opposite to the weight of the body) through the center of gravity of the displaced fluid. Mathematically,
\[F=V\sigma g\]
where V is the volume of displaced liquid and \[\sigma \] is the density of the liquid
The apparent reduction in weight of body\[=\]upthrust\[=\]weight of liquid displaced by the body.
Flotation:
A body is said to float in a liquid when the average density of the body is less than that of the liquid.
The weight of the liquid displaced by the immersed part of the body should be the same as the weight of the body.
The center of gravity of the body and center of buoyancy should be along the same vertical line.
1.2. Fluid Dynamics:
Steady Flow (Stream line Flow)
The kind of flow in which the velocity of fluid particles crossing a particular position is the same at all the times. Clearly, each particle follows the same path as followed by the previous particle through that point.
Line of flow
Line of flow can be referred to as the path followed by a particle in a flowing liquid. With respect to steady flow, it is termed streamline. Two streamlines are never said to intersect with each other.
1.2.1. Equation of Continuity:
In a time \[\text{t}\], the volume of liquid entering the tube of flow in a steady flow is given by: \[{{\text{A}}_{\text{1}}}{{\text{V}}_{\text{1}}}\text{ }\!\!\Delta\!\!\text{ t}\].
The same volume should flow out of this tube since the liquid is incompressible in nature. The volume flowing out in \[\text{t}\] is given by\[{{\text{A}}_{\text{2}}}{{\text{V}}_{\text{2}}}\text{ }\!\!\Delta\!\!\text{ t}\].
In the given diagram, \[{{A}_{1}}{{V}_{1}}={{A}_{2}}{{V}_{2}}\]
Also, mass flow rate is given by \[\text{ }\!\!\rho\!\!\text{ AV}\], where \[\text{ }\!\!\rho\!\!\text{ }\] is the density of the liquid.
1.2.2. Bernoulli’s Theorem:
When a streamlined flow of an ideal fluid is considered, the sum of pressure energy per unit volume, potential energy per unit volume and kinetic energy per unit volume are always constant at all cross-sections of the liquid. Mathematically,
\[p+\rho gh+\frac{\rho {{V}^{2}}}{2}=const.\]
Bernoulli’s equation is applicable only for an incompressible steady flow of a fluid with no viscosity.
Applications of Bernoulli’s Theorem.
Velocity of efflux
Considering the diagram above, let us determine the velocity with which liquid comes out of a hole at a depth h below the liquid surface.
Using Bernoulli’s theorem,
\[{{P}_{A}}+\frac{1}{2}\rho {{V}_{A}}^{2}+\rho g{{h}_{A}}={{P}_{B}}+\frac{1}{2}\rho {{V}_{B}}^{2}+\rho g{{h}_{B}}\]
\[\Rightarrow {{P}_{atm}}+\frac{1}{2}\rho {{V}_{A}}^{2}+\rho gh={{P}_{atm}}+\frac{1}{2}\rho {{V}^{2}}+0\]
(Notice that \[{{P}_{B}}={{P}_{atm}}\], because we have opened the liquid to the atmosphere)
\[\Rightarrow {{V}^{2}}={{V}_{A}}^{2}+2gh\]
Using the equation of continuity,
\[A{{V}_{A}}=aV\]
Where, A is the area of cross-section of the vessel and a is the area of hole.
\[\Rightarrow {{V}^{2}}=\frac{{{a}^{2}}}{{{A}^{2}}}{{V}^{2}}+2gh\]
\[\Rightarrow V=\frac{\sqrt{2gh}}{\sqrt{1-{{a}^{2}}/{{A}^{2}}}}\approx \sqrt{2gh}\] (when the hole is very small)
Venturi Meter
A venturi meter is an instrument used for measuring the rate of flow of fluids.
Considering the diagram above,
If \[{{\text{P}}_{\text{A}}}\] is pressure at \[\text{A}\] and \[{{\text{P}}_{\text{B}}}\] is pressure at \[\text{B}\],
\[{{P}_{A}}-{{P}_{B}}=h\rho g\]
Where, h is the difference of heights of liquids of density \[\text{ }\!\!\rho\!\!\text{ }\] in vertical tubes.
Now, when \[{{\text{V}}_{\text{1}}}\] is velocity at \[\text{A}\] and \[{{\text{V}}_{\text{2}}}\] is velocity at \[\text{B}\], using the equation of continuity,
\[Q={{A}_{1}}{{V}_{1}}={{A}_{2}}{{V}_{2}}\];
Using Bernoulli’s theorem,
\[{{P}_{A}}+\rho \frac{{{V}_{1}}^{2}}{2}={{P}_{B}}+\rho \frac{{{V}_{2}}^{2}}{2}\]
\[\Rightarrow {{V}_{2}}^{2}-{{V}_{1}}^{2}=\frac{2}{\rho }({{P}_{A}}-P{}_{B})=\frac{2}{\rho }h\rho g\]
\[\Rightarrow \frac{{{Q}^{2}}}{{{A}_{2}}^{2}}-\frac{{{Q}^{2}}}{{{A}_{1}}^{2}}=2hg\text{ }(Q=AV)\]
\[\Rightarrow Q={{A}_{1}}{{A}_{2}}\sqrt{\frac{2hg}{{{A}_{1}}^{2}-{{A}_{2}}^{2}}}\], which is an expression for the rate of flow of fluids using a venturi meter.
1.3. Viscosity:
The characteristic of a fluid by virtue of which it opposes the relative motion between its different layers is termed viscosity and the force which comes into action is termed the viscous force. Mathematically, viscous force is given by:
\[F=-\eta A\frac{dv}{dx}\]
Where, \[\eta \] is a constant dependent on the nature of the liquid and is termed the coefficient of viscosity and \[\frac{dv}{dx}\] is the velocity gradient.
S.I. unit of coefficient of viscosity is \[Pa.s\text{ }or\text{ }Ns{{m}^{-2}}\].
CGS unit of viscosity is \[poise(1Pa.s=10\text{ }poise)\].
1.3.1. Stoke’s Law:
When a solid travels through a viscous medium, its motion is opposed by a viscous force dependent on the velocity, shape and size of the body.
The viscous drag acting on a spherical body of radius \[r\], travelling with velocity \[v\], in a viscous medium of viscosity \[\eta \] is given by \[{{F}_{viscous}}=6\pi \eta rv\]
This formula is termed Stoke’s law.
Importance of Stoke’s law:
This law is utilized in finding the electronic charge with the help of Millikan’s experiment.
This law talks about the formation of clouds.
This law talks about why the speed of raindrops is less than that of an object falling freely with a constant velocity from the height of clouds.
This law allows a person to fly down with the help of a parachute.
1.3.2. Terminal Velocity:
It refers to the maximum constant velocity acquired by the body under free fall in a viscous medium. Mathematically,
\[{{v}_{r}}=\frac{2{{r}^{2}}(\rho -{{\rho }_{0}})g}{9\eta }\]
1.3.3. Poiseuille’s Formula:
Poiseuille learnt the stream-line flow of liquid in capillary tubes.
Volume of liquid coming out of the tube per second is equal to \[\frac{\pi {{\Pr }^{4}}}{8\eta l}\].
1.3.4. Reynold Number:
The maintenance of stability of laminar flow is facilitated by viscous forces. But it is seen that laminar or steady flow is disrupted when the rate of flow is large. Irregular, unsteady motion and turbulence occur at high flow rates.
Reynold’s number refers to a dimensionless number whose value provides an approximate idea on the flow rate, if it would be turbulent or not. Mathematically, it is given by
\[{{R}_{e}}=\frac{\rho vD}{\eta }\]
Where,
\[\rho =the\text{ }density\text{ }of\text{ }the\text{ }fluid\text{ }flowing\text{ }with\text{ }a\text{ }speed\text{ }v\] \[D=the\text{ }diameter\text{ }of\text{ }the\text{ }tube\]\[\eta =\text{ }the\text{ }coefficient\text{ }of\text{ }vis\cos ity\text{ }of\text{ }the\text{ }fluid\]
It is observed that flow is streamline or laminar for \[{{\text{R}}_{\text{e}}}\] less than \[\text{1000}\] whereas the flow is turbulent for \[{{\text{R}}_{\text{e}}}>2000\]. Additionally, the flow becomes unsteady for \[{{\text{R}}_{\text{e}}}\] between \[\text{1000}\] and \[\text{2000}\].
1.4. Surface Tension:
The surface tension of a liquid refers to the force per unit length in the plane of a liquid surface perpendicular to either side of an imaginary line drawn on that surface. Mathematically,
\[S=\frac{F}{l}\]
Where,
\[S=\text{ }surface\text{ }tension\text{ }of\text{ }liquid.\]
Unit of surface tension in MKS system: \[N/m\text{ }or\text{ }J/{{m}^{2}}\]
Unit of surface tension in CGS system: \[dyne/cm\text{ }or\text{ }erg/c{{m}^{2}}\]
1.4.1. Surface Energy:
In order to increase the surface area, work needs to be done over the surface of the liquid. This work is stored in the liquid surface as its potential energy. Thus, the surface energy of a liquid refers to the excess potential energy per unit area of the liquid surface. Mathematically,
\[W=S\Delta A;\text{ }where\text{ }\Delta A=increase\text{ }in\text{ }surface\text{ }area.\]
1.4.2. Excess Pressure:
Excess pressure in a liquid drop or bubble in a liquid is given by \[P=\frac{2T}{R}\].
Excess pressure in a soap bubble is given by \[P=\frac{4T}{R}\], as it has two free surfaces.
1.4.3 Angle of Contact:
The angle between the tangent to the liquid surface at the point of contact and the solid surface inside the liquid is termed the angle of contact $(\theta )$, as shown in the diagram in the following section.
When the glass plate is immersed in mercury, the surface becomes curved and the mercury gets depressed below. Angle of contact turns out to be obtuse for mercury.
When the plate is dipped in water with its side vertical, the water gets drawn-up along the plane and forms a curved shape. Angle of contact turns out to be acute for water.
1.4.4 Capillary Tube and Capillary Action:
A very narrow glass tube with a fine borehole and open at both ends is called a capillary tube. When a capillary tube is dipped in a liquid, the liquid would rise or fall in the tube and this reaction is known as capillarity.
Mathematically, capillary rise or fall (h) is given by
\[h=\frac{2S\cos \theta }{r\rho g}=\frac{2S}{R\rho g}\]
Where,
\[S=\text{ }surface\text{ }tension\]
\[\theta =\text{ }angle\text{ }of\text{ }contact\]
\[r=\text{ }radius\text{ }of\text{ }capillary\text{ }tube\]
\[R=\text{ }radius\text{ }of\text{ }meniscus\]
\[\rho =\text{ }density\text{ }of\text{ }liquid\]
Capillary rise in a tube of insufficient length:
When the actual height to which a liquid would rise in a capillary tube is ‘h’, then a capillary tube of length less than ‘h’ can be considered as a tube of “insufficient length”.
In this case, liquid rises to the top of the capillary tube of length \[l(l<h)\] and adjusts the radius of curvature of its meniscus till the excess pressure is equalised by the pressure of liquid column of length \[\text{l}\]. (Notice that liquid does not overflow here).
Consider the following figure:
Mathematically,
\[\frac{2\sigma }{r'}=l\rho g...(i)\]
where, $r'$ is the new curvature.
Now, when \[\text{r}\] is the actual radius of curvature,
\[\frac{2\sigma }{r}=h\rho g...(ii)\]
Comparing \[\text{(i) and (ii)}\];
\[\Rightarrow \frac{2\sigma }{\rho g}=lr'=hr\]
\[\Rightarrow r'=\frac{hr}{l}\], which is an expression for the new curvature in the case of capillary rise in a tube of insufficient length.
Capillarity for different cases of adhesion and cohesion:
\[Adhesion>Cohesion\] | \[Adhesion=Cohesion\] | \[Adhesion<Cohesion\] |
Liquid would wet the solid | Critical | Liquid will not wet the solid. |
Meniscus turns out to be concave | Meniscus would be plane | Meniscus is convex. |
Angle of contact turns out to be acute \[(\theta =90{}^\circ )\] | Angle of contact would be \[\text{9}{{\text{0}}^{\text{o}}}\] | Angle of contact is obtuse \[(\theta =90{}^\circ )\] |
Pressure below the meniscus turns out to be lesser than above it by \[(2T/r)\]i.e., \[P={{P}_{0}}-\frac{2T}{r}\] | Pressure below the meniscus would be the same as above it, i.e., \[P={{P}_{0}}\] | Pressure below the meniscus more than above it by \[(2T/r)\]. i.e., \[P={{P}_{0}}+\frac{2T}{r}\] |
In capillary, there would be rise | No capillary rise/fall | In capillary, there would be fall |
Mechanical Properties of Fluids Class 11 Notes
Fluids and Their Properties
Fluids are substances that have no definite shape and can flow easily from higher levels to lower levels. For example, liquids and gases are fluids.
They don’t have a definite shape, and they take the shape of a container.
Properties of Fluid
Hydrostatic - Fluids at rest
Hydro-dynamic - Fluids in motion
Thrust
Thrust is a force exerted by the fluid at the walls of the container. The S.I. unit of thrust is Newton, and the CGS unit is Dyne.
Pascal’s Law
Pascal’s law states that if gravity’s effect is ignored, then pressure applied to any point in an enclosed incompressible fluid is transmitted equally in all directions throughout the fluid.
We know that P1 - P2 = hρg
At g = 0, P1 - P2 = 0
⇒ P1 = P2
This means at g = 0, the pressure at every two points inside the liquid is the same.
Archimedes’ Principle
When a body or object is completely or partially immersed in a liquid, at rest, the body loses some of its weight. This apparent loss in the weight of the body is equal to the weight of the liquid displaced by the immersed part of the body.
Specific Gravity of the body
The specific gravity of the body is equivalent to the relative density of the body.
It is given by:
\[\frac{\text{Weight of body in air}}{\text{Loss of weight of the body in the water at } 4^{\circ}C}\]
The Density of the Mixture of a Substance
If two liquids of different masses m1 and m2, and densities ρ1 and ρ2 are mixed, then the density of a mixture of the substances is given by:
\[\rho_{Mixture} = \frac{\rho_{1} \rho_{2} (m_{1} + m_{2})}{m_{1} \rho_{2} + m_{2} \rho_{1}}\]
If the mass is the same, but densities are different, i.e., ρ1 and ρ2. Then ρMixture is:
\[= \frac{\rho_{1} + \rho_{2}}{2}\]
If the number of substances of volume V1, V2 and densities ρ1 and ρ2 are mixed, then the mixture’s density is:
\[\rho_{Mixture} = \frac{\rho_{1} V_{1} + \rho_{2} V_{2}}{V_{1} + V_{2}}\]
Law of Floatation
When a body of density ρ, and volume V is immersed completely in a liquid, two forces act on it. If W is the weight of the body and w is the buoyant force, then three cases are possible. They are:
Case 1: W > w. In this case, the body will completely sink in the liquid.
Case 2: W < w. In this case, the body will float partially and immerse partially in water.
Case 3: W = w. In this case, the body will float in the liquid in a balanced position.
Viscosity
Stoke’s Law
Terminal Velocity
Poiseuille’s Formula
Reynold Number
Fluid mechanics is filled with various observations conducted by eminent scientists. This section in class 11th Physics Chapter 9 Notes also include some of the essential laws and principles you must prepare to score considerably in exams. Explanations, equations, SI and CGI units, etc. all are laid out concisely such that you do not miss out anything while revising.
Surface Tension
Surface Energy
Excess Pressure
Angle of Contact
Capillary Tube and Capillary Action
The final section in the notes of Chapter 9 Physics Class 11 discusses all essential details related to surface tension. Furthermore, you will also study how to increase surface area, how to determine excess pressure in a liquid bubble and angle of contact. Also, an experiment using a capillary tube is provided to rule out capillarity action and radius of curvature.
If you have exams around the corner, we recommend you to download notes on Mechanical Properties of Fluids Class 11 offered by Vedantu, immediately. Drafted by experienced Physics lecturers, this revision guide ensures to provide you with outstanding grades.
Solved Sample Numerical Problem
Q1. A vessel 6m high is half-filled with water and then filled to the top with oil of density 0.96 g/c.c. What is the pressure at the bottom of the vessel due to these liquids?
Given: h = 6 m, ρ0 = 0.96 g/c.c. = 0.96 x 103kgm-3,ρW = 103kgm-3
To find: P
Solution: Let’s find out the mean density. It is given by:
\[\rho_{Mean} = \frac{\rho_{w} + \rho_{0}}{2} = \frac{10^{3} + 0.96 \times 10^{3}}{2} = 0.98 \times 10^{3} kgm^{-3}\]
Therefore, the pressure at the bottom is given by:
P = hρg
\[\Rightarrow P = 6 \times 0.98 \times 10^{3} \times 9.8 = 5.7624 \times 10^{4} Nm^{-2}\].
For downloading the notes on mechanical properties of fluids class 11, click on the link given on this page for Class 11 Mechanical Properties of Fluids Notes.
Key Features of Vedantu’s Solved Previous Years’ Question Papers
Advantages of going through Vedantu’s Solved PYQP are:
After extensive research on the latest CBSE syllabus, our subject matter experts have prepared the solved PYQP.
The solutions are concise, and practicing them will help you stay updated with the latest CBSE exam pattern and with the weightage for each topic in the previous years’ papers.
You can download the study materials, sample papers, revision notes, all for free from Vedantu.
Providing all the things to the students worldwide is our aim. For any queries or doubts, get in touch with out experts, download our App and stay connected with us for the latest updates.
FAQs on Mechanical Properties of Fluids Class 11 Notes CBSE Physics Chapter 9 (Free PDF Download)
1. Find the excess pressure inside the mercury drop if its radius is 6mm, and surface tension at room temperature is 5.5 x 10-1Nm-1. Given that the atmospheric pressure is 1.10 x 105Pa.
Ans: Here, r = 6mm = 6 x 10-3m, P = 1.10 x 105Pa, and S = 5.5 x 10-1Nm-1
Excess pressure inside the drop is:
PEXCESS PRESSURE = 2S/r = 2 x 5.5 x 10-1/6 x 10-3 = 183.33 Pa.
2. Why does Chalk Emit Bubbles in all Directions When Immersed in Water?
Ans: A piece of chalk has pores in all directions that act as narrow capillaries. When we immerse chalk in water, the water enters through the pores and forces the air out in the form of bubbles.
3. How does Vedantu Stand Out of the Queue Worldwide?
Ans: Vedantu stands out of the queue because it provides concise study materials to all students and for exam preparation like JEE Main & Advanced, NEET for free.
These materials are updated consistently according to the latest exam pattern.
4. How can I Prepare for Physics?
Ans: The following tips will help you to prepare for Physics exam.
Revise all the concepts from NCERT.
Solve the previous year question papers.
Take mock tests.



























