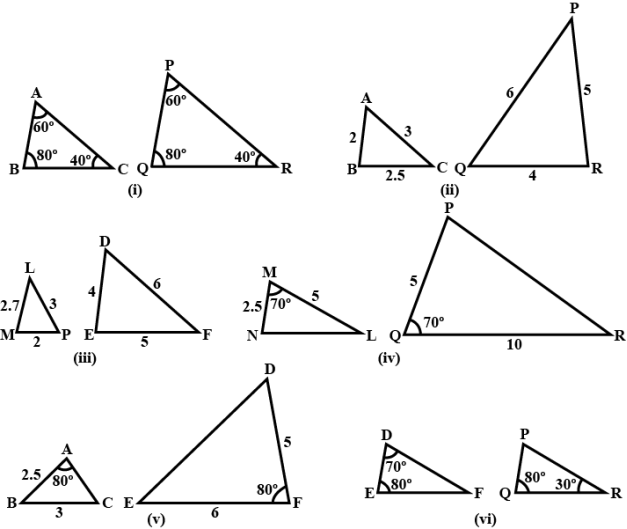
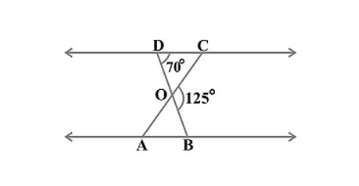
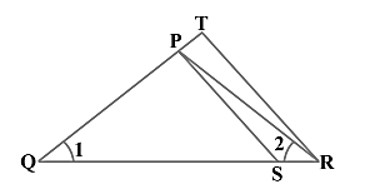
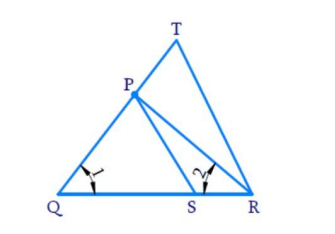
NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.3

1. State which pairs of triangles in the following figure are similar? Write the similarity criterion used by you for answering the question and also write the pairs of similar triangles in the symbolic form:

Ans: 

1. In $\triangle \mathrm{ABC}$ and $\triangle \mathrm{PQR}$

$\angle \mathrm{A}=\angle \mathrm{P}$

$\angle \mathrm{B}=\angle \mathrm{Q}$

$\angle \mathrm{C}=\angle \mathrm{R}$

$\therefore$ By AAA criterion of similarity, $\triangle \mathrm{ABC} \sim \triangle \mathrm{PQR}$

2. In $\triangle \mathrm{ABC}$ and $\triangle \mathrm{QRP}$

$\frac{\mathrm{AB}}{\mathrm{QR}}=\frac{\mathrm{BC}}{\mathrm{RP}}=\frac{\mathrm{AC}}{\mathrm{QP}}=\frac{1}{2}$

$\therefore$ By SSS criterion of similarity, $\triangle \mathrm{ABC} \sim \triangle \mathrm{QRP}$

3. In $\triangle \mathrm{LMP}$ and $\triangle \mathrm{DEF}$

$\frac{\mathrm{LM}}{\mathrm{DE}}=\frac{2.7}{4}, \frac{\mathrm{LP}}{\mathrm{DF}}=\frac{1}{2}$

The sides are not in the equal ratios, Hence the two triangles are not similar.

4. In $\triangle \mathrm{MNL}$ and $\triangle \mathrm{QPR}$

$\angle \mathrm{M}=\angle \mathrm{Q}$

$\frac{\mathrm{MN}}{\mathrm{QP}}=\frac{\mathrm{ML}}{\mathrm{QR}}=\frac{1}{2}$

$\therefore$ By SAS criterion of similarity, $\triangle \mathrm{MNL} \sim \triangle \mathrm{QP} \mathrm{R}$

5. In $\triangle \mathrm{ABC}$ and $\triangle \mathrm{EFD}$

$\angle \mathrm{A}=\angle \mathrm{F}, $

$\frac{AB}{FD}=\frac{BC}{FD}=\frac{1}{2}$

$\therefore$ By SAS criterion of similarity, $\triangle \mathrm{ABC} \sim \triangle \mathrm{EFD}$

6. In $\triangle \mathrm{DEF}$ and $\triangle \mathrm{PQR}$

Since, sum of angles of a triangle is $180^{\circ}$, Hence, $\angle \mathrm{F}=30^{\circ}$ and $\angle \mathrm{P}=70^{\circ}$

$\angle \mathrm{D} =\angle \mathrm{P}$

$\angle \mathrm{E} =\angle \mathrm{Q}$

$\angle \mathrm{F} =\angle \mathrm{R}$

$\therefore$ By AAA criterion of similarity, $\triangle \mathrm{DEF} \sim \triangle \mathrm{PQR}$

2. In the following figure, $\Delta \mathrm{ODC} \sim \Delta \mathrm{OBA}, \angle \mathrm{BOC}=125^{\circ}$ and $\angle \mathrm{CDO}=70^{\circ}$. Find $\angle \mathrm{DOC}, \angle \mathrm{DCO}$ and $\angle \mathrm{OAB}$

Ans: Given:

$\triangle \mathrm{ODC} \sim \triangle \mathrm{OBA}$

$\angle \mathrm{BOC}=125^{\circ}$

$\angle \mathrm{CDO}=70^{\circ}$

To find: $\angle D O C, \angle D C O$ and $\angle O A B$

Sol: Here, $B D$ is a line,

So, we can apply a linear pair on it.

$\angle B O C+\angle D O C=180^{\circ} (Linear Pair)$

$125^{\circ}+\angle D O C=180^{\circ}$

$\angle D O C=180^{\circ}-125^{\circ}$

$\angle D O C=180^{\circ}-125^{\circ}$

$\angle D O C=55^{\circ}$

Now in $\triangle \mathrm{DCO}$

$\angle C D O+\angle D C O+\angle D O C=180^{\circ}$

$70^{\circ}+\angle D C O+55^{\circ}=180^{\circ}$

$125^{\circ}+\angle D C O=180^{\circ}$

$\angle D C O=180^{\circ}-125^{\circ}$

$\angle D C O=55^{\circ}$

Now it is given that

$\triangle O D C \sim \triangle O B A$

Hence,

$\angle \mathrm{DCO}=\angle \mathrm{OAB}$

$55^{\circ}=\angle \mathrm{OAB}$

$\angle \mathrm{OAB}=55^{\circ}$

Now in $\triangle \mathrm{DCO}$

$\angle \mathrm{CDO}+\angle \mathrm{DCO}+\angle \mathrm{DOC}=180^{\circ} \quad$ (Sum of all angles of triangle is $180^0$ 

$70^{\circ}+\angle \mathrm{DCO}+55^{\circ}=180^{\circ}$ 

$125^{\circ}+\angle \mathrm{DCO}=180^{\circ}$ 

$\angle \mathrm{DCO}=180^{\circ}-125^{\circ}$ $\angle \mathrm{DCO}=55^{\circ}$

Now, it is given that

$\Delta \mathrm{ODC} \sim \triangle \mathrm{OBA}$

Hence.

$\angle D C O=\angle O A B$(Corresponding angles of a similar triangles are equal)

$55^{\circ}=\angle O A B$

3. Diagonals AC and BD of a trapezium ABCD with AB \|DC intersect each other at the point $\mathrm{O}$. Using a similarity criterion for two triangles, show that $\frac{\mathrm{AO}}{\mathrm{OC}}=\frac{\mathrm{OB}}{\mathrm{OD}}$

Ans: In $\Delta \mathrm{DOC}$ and $\triangle \mathrm{BOA}$

$\angle C D O=\angle A B O$ (Alternate interior angles as $A B \| C D)$

$\angle \mathrm{DCO}=\angle \mathrm{BAO}$ (Alternate interior angles as $\mathrm{AB} \| \mathrm{CD})$

$\angle \mathrm{DOC}=\angle \mathrm{BOA}$ (Vertically opposite angles $)$

$\therefore \Delta \mathrm{DOC} \sim \Delta \mathrm{BOA}$ (AAA similarity criterion)

$\therefore \frac{\mathrm{DO}}{\mathrm{BO}}=\frac{\mathrm{OC}}{\mathrm{OA}} \quad($ Corresponding sides are proportional)

$\Rightarrow \frac{\mathrm{OA}}{\mathrm{OC}}=\frac{\mathrm{OB}}{\mathrm{OD}}$

4.  In the figure, $\frac{\mathrm{QR}}{\mathrm{QS}}=\frac{\mathrm{QT}}{\mathrm{PR}} \text { and } \angle 1=\angle 2 \text {.Show that } \Delta \mathrm{PQS} \sim \Delta \mathrm{TQR}$

Ans: In $\delta \mathrm{PQR}, \angle \mathrm{PQR}=\angle \mathrm{PRQ}$

$\therefore \mathrm{PQ}=\mathrm{PR}(\mathrm{i})$

Given 

$\frac{\mathrm{QR}}{\mathrm{QS}}=\frac{\mathrm{QT}}{\mathrm{PR}}$

Using (i), we obtain

$\frac{\mathrm{QR}}{\mathrm{QS}}=\frac{\mathrm{QT}}{\mathrm{QP}}$

In $\triangle \mathrm{PQS}$ and $\triangle \mathrm{TQR}$,

$\frac{\mathrm{QR}}{\mathrm{QS}}=\frac{\mathrm{QT}}{\mathrm{QP}}[\text { [Using (ii) }]$

$\angle \mathrm{Q}=\angle \mathrm{Q}$

$\therefore \Delta \mathrm{PQS} \sim \Delta \mathrm{TQR} \quad[\text { SAS similarity criterion }]$

5. $\mathrm{S}$ and $\mathrm{T}$ are point on sides $\mathrm{PR}$ and $\mathrm{QR}$ of $\triangle \mathrm{PQR}$ such that $\angle \mathrm{P}=\angle \mathrm{RTS}$. Show that $\triangle \mathrm{RPQ} \sim \Delta \mathrm{RTS}$.

Ans: Given: $\Delta P Q R$

and the points $S$ and $T$ on sides PR and QR.

Such that $\angle P=\angle R T S$

To Prove: $\triangle \mathrm{RPQ} \sim \Delta$ RTS.

Proof:

In $\triangle \mathrm{RPQ}$ and $\triangle \mathrm{RTS}$.

$\angle P=\angle R T S$

(Given)

And $\angle \mathrm{PRQ}=\angle \mathrm{TRS}=\angle \mathrm{R}$

(Common)

So, $\triangle \mathrm{RPQ} \sim \Delta \mathrm{RTS}$.

(AA similarity)

Hence proved

6. In the following figure, if $\triangle \mathrm{ABE} \cong \triangle \mathrm{ACD}$, show that $\triangle \mathrm{ADE} \sim \Delta \mathrm{ABC}$.

Ans: 

We know that the corresponding portions of two triangles that are congruent to each other are equal.

The two triangles are comparable if one of their angles is equal to one of the other triangle's angles, and the sides that include these angles are proportionate.

For two triangles, this is known as the SAS (Side - Angle - Side) similarity criteria.

In $\triangle \mathrm{ABE}$ and $\triangle \mathrm{ACD}$

$\mathrm{AD}=\mathrm{AE}(\triangle \mathrm{ABE} \cong \Delta \mathrm{ACD} \text { given }) \ldots \ldots \ldots \text { (1) }$

$\mathrm{AB}=\mathrm{AC}(\triangle \mathrm{ABE} \cong \triangle \mathrm{ACD} \text { given })$

Now Consider $\triangle A D E$ and $\triangle A B C$

and $\angle$ DAE $=\angle B A C$ (Common angle)

Thus, $\triangle$ ADE $\sim A$ ABC (SAS criterion)

7. In the following figure, altitudes $\mathrm{AD}$ and $\mathrm{CE}$ of $\Delta \mathrm{ABC}$ intersect each other at the point, P. Show, that:

1. $\triangle \mathrm{AEP} \sim \Delta \mathrm{CDP}$

Ans: When two angles from one triangle are equivalent to two angles from another triangle, the two triangles are said to be comparable.

For two triangles, this is known as the AA similarity criteria.

In $\triangle \mathrm{AEP}$ and $\triangle \mathrm{CDP}$

$[\because \mathrm{CE} \perp \mathrm{AB}$ and $\mathrm{AD} \perp \mathrm{BC} ;$ altitudes $]$

$\angle A P E=\angle C P D$ (Vertically opposite angles)

$\Rightarrow \triangle$ AEP $\sim \triangle$ CPD (AA criterion)

2. $\triangle \mathrm{ABD} \sim \Delta \mathrm{CBE}$

Ans: When two angles from one triangle are equivalent to two angles from another triangle, the two triangles are said to be comparable.

For two triangles, this is known as the AA similarity criteria.

In $\triangle \mathrm{ABD}$ and $\triangle \mathrm{CBE}$

$\angle \mathrm{ADB}=\angle C E B=90^{\circ}$

$\angle \mathrm{ABD}=\angle C B E \text { (Common angle) }$

$\Rightarrow \triangle \mathrm{ABD} \sim \Delta C B E \text { (AA criterion) }$

3. $\triangle \mathrm{AEP} \sim \triangle \mathrm{ADB}$

Ans: When two angles from one triangle are equivalent to two angles from another triangle, the two triangles are said to be comparable.

For two triangles, this is known as the AA similarity criteria.

In $\triangle \mathrm{AEP}$ and $\triangle \mathrm{ADB}$

$\angle \mathrm{AEP}=\angle \mathrm{ADB}=9 \mathrm{O}^{\circ}$

$\angle \mathrm{PAE}=\angle \mathrm{BAD} \text { (Common angle) }$

$\Rightarrow \triangle \mathrm{AEP} \sim \triangle \mathrm{ADB} \text { (AA criterion) }$

4. $\Delta \mathrm{PDC} \sim \Delta \mathrm{BEC}$

Ans: When two angles from one triangle are equivalent to two angles from another triangle, the two triangles are said to be comparable.

For two triangles, this is known as the AA similarity criteria.

In $\triangle \mathrm{PDC}$ and $\triangle \mathrm{BEC}$

$\angle \mathrm{PDC}=\angle \mathrm{BEC}=9 \mathrm{O}^{\circ}$

$\angle \mathrm{PCD}=\angle \mathrm{BCE} \text { (Common angle) }$

$\Rightarrow \triangle \text { PDC } \sim \triangle \mathrm{BEC} \text { (AA criterion)}$

8. $\mathrm{E}$ is a point on the side AD produced of a parallelogram $\mathrm{ABCD}$ and $\mathrm{BE}$ intersects $\mathrm{CD}$ at $\mathrm{F}$. Show that $\triangle \mathrm{ABE} \sim \Delta \mathrm{CFB}$

Ans:

In $\triangle \mathrm{ABE}$ and $\triangle \mathrm{CFB}$,

$\angle \mathrm{A}=\angle \mathrm{C}$ (Opposite angles of a parallelogram)

$\angle \mathrm{AEB}=\angle \mathrm{CBF}$ (Alternate interior angles as $\mathrm{AE} \| \mathrm{BC})$

$\therefore \Delta \mathrm{ABE} \sim \Delta \mathrm{CFB}$ (By AA similarity criterion)

9. In the following figure, $\mathrm{ABC}$ and AMP are two right triangles, right angled at B and M respectively, prove that:

1. $\Delta \mathrm{ABC} \sim \Delta \mathrm{AMP}$

2. $\frac{\mathrm{CA}}{\mathrm{PA}}=\frac{\mathrm{BC}}{\mathrm{MP}}$

Ans: In $\triangle \mathrm{ABC}$ and $\triangle \mathrm{AMP}$

$\angle \mathrm{ABC}=\angle \mathrm{AMP}\left(\operatorname{Each} 90^{\circ}\right)$ $\angle \mathrm{A}=\angle \mathrm{A}(\mathrm{Common})$

$\therefore \Delta \mathrm{ABC} \sim \Delta \mathrm{AMP}$ (By AA similarity criterion)

$\Rightarrow \frac{\mathrm{CA}}{\mathrm{PA}}=\frac{\mathrm{BC}}{\mathrm{MP}}$ (Corresponding sides of similar triangles are proportional)

10. $\mathrm{CD}$ and $\mathrm{GH}$ are respectively the bisectors of $\angle \mathrm{ACB}$ and $\angle \mathrm{EGF}$ such that $\mathrm{D}$ and $\mathrm{H}$ lie on sides $\mathrm{AB}$ and $\mathrm{FE}$ of $\triangle \mathrm{ABC}$ and $\triangle \mathrm{EFG}$ respectively. If $\triangle \mathrm{ABC} \sim$ $\Delta \mathrm{FEG}$, Show that:

(i) $\frac{\mathrm{CD}}{\mathrm{GH}}=\frac{\mathrm{AC}}{\mathrm{FG}}$

Ans: $\text { It is given that } \triangle \mathrm{ABC} \sim \Delta \mathrm{FEG}.$

$\therefore \angle \mathrm{A}=\angle \mathrm{F}, \angle \mathrm{B}=\angle \mathrm{E}, \text { and } \angle \mathrm{ACB}=\angle \mathrm{FGE}$

$\text { Since, } \angle \mathrm{ACB}=\angle \mathrm{FGE} $

$\therefore \angle \mathrm{ACD}=\angle \mathrm{FGH} \text { (Angle bisector) } $

$\text { And, } \angle \mathrm{DCB}=\angle \mathrm{HGE} \text { (Angle bisector) } $

$\text { In } \triangle \mathrm{ACD} \text { and } \Delta \mathrm{FGH} \text {, }$

$\angle \mathrm{A}=\angle \mathrm{F} \text { (Proved above) }$

$\angle \mathrm{ACD}=\angle \mathrm{FGH} \text { (Proved above) }$

$\therefore \Delta \mathrm{ACD} \sim \Delta \mathrm{FGH} \text { (By AA similarity criterion) }$

$\Rightarrow \frac{\mathrm{CD}}{\mathrm{GH}}=\frac{\mathrm{AC}}{\mathrm{FG}}$

(ii) $\triangle \mathrm{DCB} \sim \Delta \mathrm{HGE}$

Ans: $\text { In } \triangle \mathrm{DCB} \text { and } \triangle \mathrm{HGE} \text {, } $

$\angle \mathrm{DCB}=\angle \mathrm{HGE} \text { (Proved above) } $

$\angle \mathrm{B}=\angle \mathrm{E} \text { (Proved above) } $

$\therefore \triangle \mathrm{DCB} \sim \triangle \mathrm{HGE} \text { (By AA similarity criterion) }$

(iii) $\triangle \mathrm{DCA} \sim \Delta \mathrm{HGF}$

Ans: In $\Delta \mathrm{DCA}$ and $\Delta \mathrm{HGF}$,

$\angle \mathrm{ACD}=\angle \mathrm{FGH}$ (Proved above)

$\angle A=\angle F$ (Proved above)

$\therefore \Delta \mathrm{DCA} \sim \Delta \mathrm{HGF}$ (By AA similarity criterion)

11. In the following figure, $\mathrm{E}$ is a point on side CB produced of an isosceles triangle $\mathrm{ABC}$ with $\mathrm{AB}=\mathrm{AC}$. If $\mathrm{AD} \perp \mathrm{BC}$ and $\mathrm{EF} \perp \mathrm{AC}$, prove that $\triangle \mathrm{ABD} \sim$ $\triangle \mathrm{ECF}$

Ans: It is given that $\mathrm{ABC}$ is an isosceles triangle.

$\therefore \mathrm{AB}=\mathrm{AC}$

$\Rightarrow \angle \mathrm{ABD}=\angle \mathrm{ECF}$

In $\Delta \mathrm{ABD}$ and $\triangle \mathrm{ECF}$

$\angle \mathrm{ADB}=\angle \mathrm{EFC}\left(\operatorname{Each} 90^{\circ}\right)$

$\angle \mathrm{BAD}=\angle \mathrm{CEF}$ (Proved above)

$\therefore \Delta \mathrm{ABD} \sim \triangle \mathrm{ECF}$ (By using AA similarity criterion)

12. Sides $\mathrm{AB}$ and $\mathrm{BC}$ and median AD of a triangle $\mathrm{ABC}$ are respectively proportional to sides PQ and QR and median PM of $\triangle \mathrm{PQR}$ (see the given figure). Show that $\triangle \mathrm{ABC} \sim \triangle \mathrm{PQR}$.

Ans: Median equally divides the opposite side.

$\therefore \mathrm{BD}=\frac{\mathrm{BC}}{2}$ and $\mathrm{QM}=\frac{\mathrm{QR}}{2}$

Given that,

$\frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{BC}}{\mathrm{QR}}=\frac{\mathrm{AD}}{\mathrm{PM}}$

$\Rightarrow \frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\frac{1}{2} \mathrm{BC}}{\frac{1}{2} \mathrm{QR}}=\frac{\mathrm{AD}}{\mathrm{PM}}$

$\Rightarrow \frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{BD}}{\mathrm{QM}}=\frac{\mathrm{AD}}{\mathrm{PM}}$

In $\triangle \mathrm{ABD}$ and $\triangle \mathrm{PQM}$,

$\frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{BD}}{\mathrm{QM}}=\frac{\mathrm{AD}}{\mathrm{PM}} \text { (Proved above) }$

$\therefore \Delta \mathrm{ABD} \sim \Delta \mathrm{PQM}$ (By SSS similarity criterion)

$\Rightarrow \angle \mathrm{ABD}=\angle \mathrm{PQM}$ (Corresponding angles of similar triangles)

In $\triangle \mathrm{ABC}$ and $\Delta \mathrm{PQR}$,

$\angle \mathrm{ABD}=\angle \mathrm{PQM} \text { (Proved above) }$

$\frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{BC}}{\mathrm{QR}}$

$\therefore \Delta \mathrm{ABC} \sim \Delta \mathrm{PQR}$ (By SAS similarity criterion)

13. $\mathrm{D}$ is a point on the side $\mathrm{BC}$ of a triangle $\mathrm{ABC}$ such that $\angle \mathrm{ADC}=\angle \mathrm{BAC}$. Show that $\mathrm{CA}^{2}= \mathrm{CB.CD}$

Ans: $\text { In } \triangle \mathrm{ADC} \text { and } \triangle \mathrm{BAC} \text {, }$

$\angle \mathrm{ADC}=\angle \mathrm{BAC}$ (Given) $\angle \mathrm{ACD}=\angle \mathrm{BCA}$ (Common angle)

$\therefore \Delta \mathrm{ADC} \sim \triangle \mathrm{BAC}$ (By AA similarity criterion)

We know that corresponding sides of similar triangles are in proportion. $\therefore \frac{\mathrm{CA}}{\mathrm{CB}}=\frac{\mathrm{CD}}{\mathrm{CA}}$ $\Rightarrow \mathrm{CA}^{2}=\mathrm{CB} \cdot \mathrm{CD}$

14. Sides $\mathrm{AB}$ and AC and median AD of a triangle $\mathrm{ABC}$ are respectively proportional to sides PQ and PR and median PM of another triangle PQR. Show that $\triangle \mathrm{ABC} \sim \Delta \mathrm{PQR}$

Ans: Given that,

$\frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{AC}}{\mathrm{PR}}=\frac{\mathrm{AD}}{\mathrm{PM}}$

Let us extend AD and PM up to point $E$ and $L$ respectively, such that $A D=$ DE and PM $=$ ML. 

Then, join $B$ to $E, C$ to $E, Q$ to $L$, and $R$ to $L .$

We know that medians divide opposite sides.

Therefore, $\mathrm{BD}=\mathrm{DC}$ and $\mathrm{QM}=\mathrm{MR}$

Also, $\mathrm{AD}=\mathrm{DE}$ (By construction)

And, $\mathrm{PM}=\mathrm{ML}$ (By construction)

In quadrilateral ABEC, diagonals AE and BC bisect each other at point D. Therefore, quadrilateral ABEC is a parallelogram.

$\therefore \mathrm{AC}=\mathrm{BE}$ and $\mathrm{AB}=\mathrm{EC}$ (Opposite sides of a parallelogram are equal) Similarly, we can prove that quadrilateral PQLR is a parallelogram and PR = QL, $P Q=L R$

It was given that

$\frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{AC}}{\mathrm{PR}}=\frac{\mathrm{AD}}{\mathrm{PM}}$

$\Rightarrow \frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{BE}}{\mathrm{QL}}=\frac{2 \mathrm{AD}}{2 \mathrm{PM}}$

$\Rightarrow \frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{BE}}{\mathrm{QL}}=\frac{\mathrm{AE}}{\mathrm{PL}}$

$\therefore \triangle \mathrm{ABE} \sim \triangle \mathrm{PQL}$ (By SSS similarity criterion)

We know that corresponding angles of similar triangles are equal.

$\therefore \angle \mathrm{BAE}=\angle \mathrm{QPL} \ldots$ (1)

Similarly, it can be proved that $\triangle \mathrm{AEC} \sim \triangle \mathrm{PLR}$ and

$\angle \mathrm{CAE}=\angle \mathrm{RPL} \ldots$ (2)

Adding equation (1) and (2), we obtain

$\angle \mathrm{BAE}+\angle \mathrm{CAE}=\angle \mathrm{QPL}+\angle \mathrm{RPL}$

$\Rightarrow \angle \mathrm{CAB}=\angle \mathrm{RPQ} \ldots$ (3)

In $\triangle \mathrm{ABC}$ and $\triangle \mathrm{PQR}$,

$\frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{AC}}{\mathrm{PR}}$ (Given)

$\angle \mathrm{CAB}=\angle \mathrm{RPQ}[\mathrm{Using}$ equation $(3)]$

$\therefore \triangle \mathrm{ABC} \sim \triangle \mathrm{PQR}$ (By SAS similarity criterion)

15. A vertical pole of a length $6 \mathrm{~m}$ casts a shadow $4 \mathrm{~m}$ long on the ground and at the same time a tower casts a shadow $28 \mathrm{~m}$ long. Find the height of the tower.

Ans: Let $\mathrm{AB}$ and $\mathrm{CD}$ be a tower and a pole respectively.

Let the shadow of $\mathrm{BE}$ and DF be the shadow of $\mathrm{AB}$ and $\mathrm{CD}$ respectively.

At the same time, the light rays from the sun will fall on the tower and the pole at the same angle.

Therefore, $\angle \mathrm{DCF}=\angle \mathrm{BAE}$

And, $\angle \mathrm{DFC}=\angle \mathrm{BEA}$

$\angle \mathrm{CDF}=\angle \mathrm{ABE}$ (Tower and pole are vertical to the ground)

$\therefore \Delta \mathrm{ABE} \sim \Delta \mathrm{CDF}$ (AAA similarity criterion)

$\Rightarrow \frac{\mathrm{AB}}{\mathrm{CD}}=\frac{\mathrm{BE}}{\mathrm{DF}}$

$\Rightarrow \frac{\mathrm{AB}}{6 \mathrm{~cm}}=\frac{28}{4}$

$\Rightarrow \mathrm{AB}=42 \mathrm{~m}$

Therefore, the height of the tower will be 42 metres.

16. If $\mathrm{AD}$ and $\mathrm{PM}$ are medians of triangles $\mathrm{ABC}$ and $\mathrm{PQR}$, respectively where $\Delta \mathrm{ABC} \sim \Delta \mathrm{PQR}$ Prove that $\frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{AD}}{\mathrm{PM}}$

Ans: It is given that $\triangle \mathrm{ABC} \sim \Delta \mathrm{PQR}$

We know that the corresponding sides of similar triangles are in proportion. 

$\therefore \frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{AC}}{\mathrm{PR}}=\frac{\mathrm{BC}}{\mathrm{QR}} \ldots(1)$

Also, $\angle \mathrm{A}=\angle \mathrm{P}, \angle \mathrm{B}=\angle \mathrm{Q}, \angle \mathrm{C}=\angle \mathrm{R} \quad \ldots$ (2)

Since AD and PM are medians, they will divide their opposite sides. $\therefore \mathrm{BD}=\frac{\mathrm{BC}}{2}$ and $\mathrm{QM}=\frac{\mathrm{QR}}{2}$

From equations (1) and (3), we obtain

$\frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{BD}}{\mathrm{QM}}$

In $\triangle \mathrm{ABD}$ and $\triangle \mathrm{PQM}$,

$\angle B=\angle Q$ (Using equation (2))

$\frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{BD}}{\mathrm{QM}}$

(Using equation (4))

$\therefore \triangle \mathrm{ABD} \sim \triangle \mathrm{PQM}$ (By SAS similarity criterion)

$\Rightarrow \frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{BD}}{\mathrm{QM}}=\frac{\mathrm{AD}}{\mathrm{PM}}$

Conclusion

Vedantu's NCERT Solutions for Class 10 Maths Chapter 6 Exercise 6.3 offers a comprehensive guide to mastering the concepts covered in this exercise. Chapter 6 focuses on triangles, and Exercise 6.3 specifically deals with the properties of triangles, including the Pythagorean theorem and its applications. Regular practice with NCERT solutions provided by platforms like Vedantu can enhance comprehension and problem-solving skills. Pay attention to the step-by-step solutions provided, grasp the underlying principles, and ensure clarity on the concepts before moving forward. Consistent practice and understanding will lead to proficiency in Triangles related problems.

Class 10 Maths Chapter 6: Exercises Breakdown

CBSE Class 10 Maths Chapter 6 Other Study Materials

Chapter-Specific NCERT Solutions for Class 10 Maths

Given below are the chapter-wise NCERT Solutions for Class 10 Maths. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.

NCERT Study Resources for Class 10 Maths

For complete preparation of Maths for CBSE Class 10 board exams, check out the following links for different study materials available at Vedantu.