Maths Chapter 6 Triangles Exercise 6.2 Class 10 NCERT Solutions - FREE PDF Download






NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2
FAQs on NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2
1. There is a vertical pole with length 6m casting a shadow of 4m on the ground. At the same time, the other tower casts a shadow 28m long. What is the height of the other tower?
(Image to be added soon)
Given:
AB= 6m
BC= 4m
Similarly, DF= h
EF= 28m
In the given triangles,
∠C = ∠E
∠B = ∠F (right angles)
By the angle-angle similarity,
Triangles ABC and DEF are similar.
Following this, the ratio of the length of their sides will be proportional.
AB/DF = BC/EF
6/h = 4/48
h= 42m
Therefore, the height of the tower is 42m.
2. The angles of a given quadrilateral are in the ratio 3:5:9:13. What are the angles of the quadrilateral?
Consider the common ratio between the angles as x
Then,
3x + 5X + 9x + 13x= 360°
x= 12°
Therefore, the angles of the quadrilateral will be:
36°, 60°, 108° and 156°.
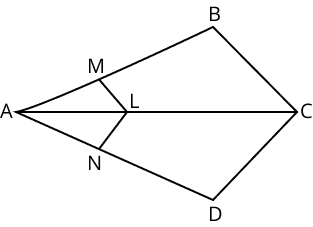
3. The figure is given with the conditions PS/SQ = PT/TR, and ∠PST = ∠PRQ. Prove that PQR is an isosceles triangle.
(Image to be added soon)
If a line divides the given sides of a triangle in the same ratio, the line is parallel to the third side.
Therefore, ST||QR
And also, ∠PST = ∠PQR (being corresponding angles)
∠PST = ∠PRQ (given)
Therefore,
∠PRQ = ∠PQR
So, the sides opposite to equal angles are also equal,
Therefore, PQ = PR.
So, PQR is an isosceles triangle.
Hence, proved.
4. Which Theorem is important from Exercise 6.2 of Chapter 6 of Class 10 Maths?
The main theorem from Exercise 6.2 of Chapter 6 of Class 10 Maths on which the majority of questions are based in the 'Basic Proportionality Theorem'. According to Basic Proportionality Theorem, which is also abbreviated as BPT, if one line is parallel to a side of the triangle and also intersects the other sides of the triangle at two different points, then that line divides those two sides of the triangle in equal proportions.
5. How many questions are present in Exercise 6.2 of Chapter 6 of Class 10 Maths?
There are a total of 10 questions in Exercise 6.2 of Chapter 6 of Class 10 Maths and each question is framed on different concepts and fundamentals, but majorly of the questions are based on the Basic Proportionality Theorem of Triangles. These questions also test the fundamentals learned by the students in the previous classes. Some questions are also based on properties of similarities of the triangles. There are various quadrilaterals, trapeziums, etc. involved through which concepts of triangles can be very easily understood and applied.
6. What main things are discussed in Exercise 6.2 of Chapter 6 of Class 10 Maths?
NCERT Solutions for Exercise 6.2 of Chapter 6 ‘Triangles’ of Class 10 Maths analyses if the proportions of the related angles and sides of triangles are the same. Equiangular triangles have corresponding angles that are identical in two separate triangles.
Two theorems are also introduced in this section:
The two sides of a triangle are divided in the same ratio if a parallel line is drawn down one of the triangle's sides and crosses the other two sides at specified points.
Any line that splits two triangle sides in the same ratio is parallel to the triangle's third side.
7. How can Exercise 6.2 of Chapter 6 of Class 10 Maths be mastered?
If students remember and comprehend the application of the theorems, they can master NCERT Solutions for Exercise 6.2 of Chapter 6 of Class 10 Maths. Children can also make a theory chart that they can consult from time to time. Using everyday objects is another fun and easy approach to study theorems. Students can also seek assistance from their teachers and parents. As a result, students are encouraged to go through the logical explanations of the theorems' proofs as well as practice them using well-explained visuals. Also, the solution PDF’s and any study material can be accessible on Vedantu absolutely free of cost.
8. What are all the things students must remember while solving Exercise 6.2 of Chapter 6 of Class 10 Maths?
In a triangle, the sum of the inner opposite angles equals the outer angle. In NCERT solutions for Exercise 6.2 of Chapter 6 of Class 10 Maths, this relationship, known as the exterior angle property of the triangle, is discussed. The students must determine the unknown exterior or interior angles based on the values provided in the practice questions. In this second exercise of the NCERT Solutions for Exercise 6.2 of Chapter 6 of Class 10 Maths, students can apply the above-mentioned theorem to readily get answers to two problems.




















 Watch Video
Watch Video