Watch Video
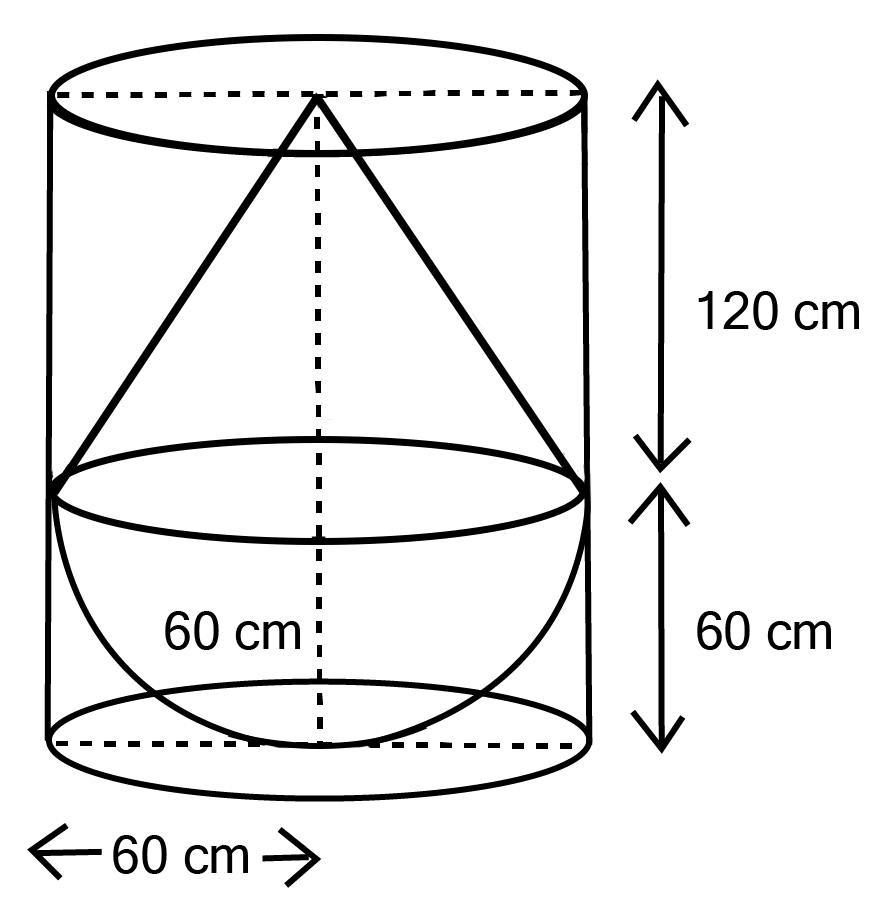
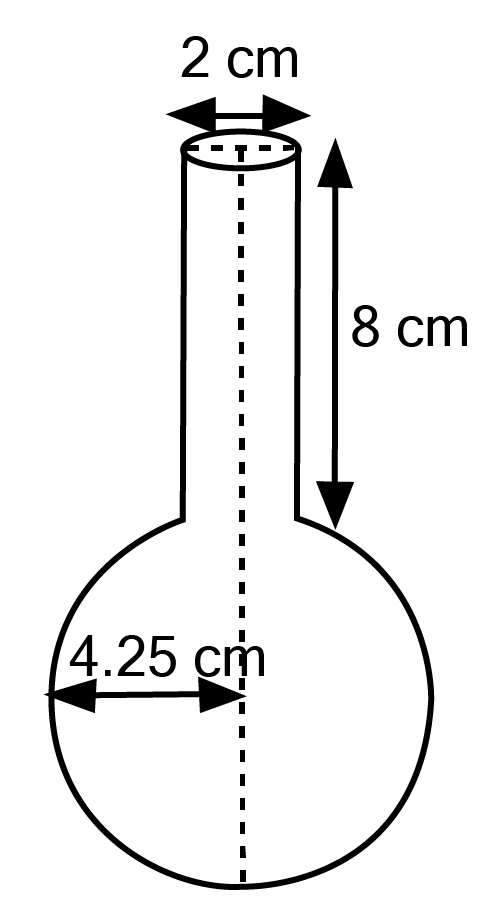
NCERT Solutions for Class 10 Maths Chapter 12 Exercise 12.2 - FREE PDF Download
You may download the PDF of Exercise 12.2 Class 10 Maths NCERT Solutions Chapter 12, Surface Areas and Volumes here. To help students better understand the topics, the knowledgeable maths professors at Vedantu have produced the class 10 Exercise 12.2 NCERT solutions PDF.
Table of Content
The subjects discussed in this page align with the most recent NCERT curriculum and recommendations. As a result, students feel that the NCERT Solutions for Maths Class 10 is a great resource for getting ready for and doing well on the CBSE Board exams.
Glance on NCERT Solutions Maths Chapter 12 Exercise 12.2 Class 10 | Vedantu
Solve problems where two or more basic 3D shapes (like cones, cylinders, spheres, cubes, etc.) are joined together.
Exercise 12.2 might include questions asking you to calculate the surface area and/or volume of various 3D shapes using the formulas it involves worded problems where you have to identify the 3D shape from the description and then apply the relevant formula.
Pay attention to the units (cm, m, etc.) while using the formulas.
Sketch a diagram of the 3D shape if it helps you visualize the problem.
Always pay attention to the specific instructions for each question. There are variations in the formulas depending on whether you need to calculate the total surface area (including the base) or the curved surface area only.
Formulas Used
Cube: Volume = a³, where a is the side length.
Cuboid: Volume = l × b × h, where l, b, and h are length, breadth, and height respectively.
Sphere: Volume = (4/3)πr³, where r is the sphere's radius.
Surface Area and Volume of Hemisphere
The volume of Hemisphere = (2πr^3)/3
Surface Area of Hemisphere = 3πr^2
Curved Surface Area of a Hemisphere = 2πr^2
The Formulas of Right Circular Cone are as follows:
Curved surface area of a cone = πr√(r^2 + h^2)
Volume of a Cone (V) = (1/3) × πr^2 × h
Total surface area of a cone = πr^2 + πr√(r^2 + h^2)
The Formulas for Cylinder are as Follows:
Curved surface area of cylinder = 2πrh
Total surface area of cylinder = 2πr(r+h)
The volume of a cylinder, V = πr^2h
NCERT Solutions for Class 10 Maths Chapter 12 Surface Areas And Volumes Ex 12.2
ShareShareClass 10 Ex 12.2
1. A solid is in the shape of a cone standing on a hemisphere with both their radii being equal to 1 cm and the height of the cone is equal to its radius. Find the volume of the solid in terms of π.
Ans. From the given figure, we know that
Height (h) of conical part = Radius(r) of conical part \[{\text{ = 1cm}}\]
Radius(r) of hemispherical part = Radius of conical part (r) \[{\text{ = 1cm}}\]
Volume of solid = Volume of conical part + Volume of hemispherical part
\[{\text{ = }}\dfrac{1}{3}\pi {r^2}h + \dfrac{2}{3}\pi {r^3}\]
\[{\text{ = }}\dfrac{1}{3}\pi {\left( 1 \right)^2}\left( 1 \right) + \dfrac{2}{3}\pi {\left( 1 \right)^3} = \dfrac{{2\pi }}{3} + \dfrac{1}{3} = \pi {\text{c}}{{\text{m}}^3}\]
2. Rachel, an engineering student, was asked to make a model shaped like a cylinder with two cones attached at its two ends by using a thin aluminum sheet. The diameter of the model is \[{\text{3cm}}\] and its length is\[{\text{12cm}}\]. If each cone has a height of \[{\text{2cm}}\], find the volume of air contained in the model that Rachel made. (Assume the outer and inner dimensions of the model to be nearly the same.)
Ans:
From the given figure, we know that
Height (h1) of each conical part \[{\text{ = 2cm}}\]
Height (h2) of cylindrical part \[{\text{ = 12 - 2}} \times {\text{ Height of conical part}}\]
\[{\text{ = 12 - 2}} \times {\text{2 = 8cm}}\]
Radius (r) of cylindrical part = Radius of conical part \[{\text{ = }}\dfrac{3}{2}{\text{cm}}\]
Volume of air present in the model = Volume of cylinder \[ + {\text{ }}2\, \times \]Volume of cones
\[ = \pi {r^2}{h_2} + 2 \times \dfrac{1}{3}\pi {r^2}{h_1}\]
\[ = \pi \times {\left( {\dfrac{3}{2}} \right)^2} \times 8 + 2 \times \dfrac{1}{3}\pi {\left( {\dfrac{3}{2}} \right)^2} \times 2\]
\[ = \pi \times \dfrac{9}{4} \times 8 + \dfrac{2}{3}\pi \times \dfrac{9}{4} \times 2\]
\[ = 18\pi + 3\pi = 21\pi = 66{\text{c}}{{\text{m}}^3}\]
3. A gulab jamun, contains sugar syrup up to about 30% of its volume. Find approximately how much syrup would be found in 45 gulab jamuns, each shaped like a cylinder with two hemispherical ends with length 5 cm and diameter 2.8 cm (see the given figure).
Ans:
We can observe that,
Radius (r) of cylindrical part = Radius (r) of hemispherical part
\[{\text{ = }}\dfrac{{{\text{2}}{\text{.8}}}}{{\text{2}}}{\text{ = 1}}{\text{.4cm}}\]
Length of each hemispherical part = Radius of hemispherical part \[{\text{ = 1}}{\text{.4cm}}\]
Length (h) of cylindrical part \[{\text{ = 5 - }}\,{\text{2}} \times {\text{Length of hemispherical part }}\]
\[{\text{ = 5 - }}\,{\text{2}} \times {\text{1.4 = 2}}{\text{.2cm}}\]
Volume of one gulab jamun = Vol. of cylindrical part + 2 × Vol. of hemispherical part
\[ = \pi {r^2}h + 2 \times \dfrac{2}{3}\pi {r^3} = \pi {r^2}h + \dfrac{4}{3}\pi {r^3}\]
\[ = \pi {\left( {1.4} \right)^2}\left( {2.2} \right) + \dfrac{4}{3}\pi {\left( {1.4} \right)^3}\]
\[ = \dfrac{{22}}{7} \times 1.4 \times 1.4 \times 2.2 + \dfrac{4}{3} \times \dfrac{{22}}{7} \times 1.4 \times 1.4 \times 1.4\]
\[{\text{ = 13}}{\text{.552 + 11}}{\text{.498 = 25}}{\text{.05c}}{{\text{m}}^{\text{3}}}\]
Volume of \[{\text{45}}\] gulab jamuns \[{\text{ = 45}} \times {\text{25}}{\text{.05 = 1127}}{\text{.25c}}{{\text{m}}^{\text{3}}}\]
Volume of sugar syrup \[ = 30\% \] of volume
\[ = \dfrac{{30}}{{100}} \times 1127.25\]
\[{\text{ = 338}}{\text{.17c}}{{\text{m}}^{\text{3}}}\]
\[ \cong {\text{338c}}{{\text{m}}^{\text{3}}}\]
4. A pen stand made of wood is in the shape of a cuboid with four conical depressions to hold pens. The dimensions of the cuboids are \[{\text{15cm}}\] by \[{\text{10cm}}\] by \[{\text{3}}{\text{.5cm}}\]. The radius of each of the depressions is \[{\text{0}}{\text{.5cm}}\] and the depth is \[{\text{1}}{\text{.4cm}}\]. Find the volume of wood in the entire stand (see the following figure).
Ans:
Depth (h) of each conical depression\[{\text{ = 1}}{\text{.4cm}}\]
Radius (r) of each conical depression\[{\text{ = 0}}{\text{.5cm}}\]
Volume of wood = Volume of cuboid \[ - {\text{ }}4 \times \] Volume of cones
\[{\text{ = l * b * h}}\]\[ - 4 \times \dfrac{1}{3}\pi {r^2}h\]
\[ = 15 \times 10 \times 3.5 - 4 \times \dfrac{1}{3} \times \dfrac{{22}}{7} \times {\left( {\dfrac{1}{2}} \right)^2} \times 1.4\]
\[ = 525 - 1.47\]
\[{\text{ = 523}}{\text{.53c}}{{\text{m}}^{\text{3}}}\]
5. A vessel is in the form of an inverted cone. Its height is \[{\text{8cm}}\] and the radius of its top, which is open, is \[{\text{5cm}}\]. It is filled with water up to the brim. When lead shots, each of which is a sphere of radius \[{\text{0}}{\text{.5cm}}\]are dropped into the vessel, one-fourth of the water flows out. Find the number of lead shots dropped in the vessel.
Ans:
Height (h) of conical vessel\[{\text{ = 8cm}}\]
Radius (r1) of conical vessel\[{\text{ = 5cm}}\]
Radius (r2) of lead shots\[{\text{ = 0}}{\text{.5cm}}\]
Let the number of lead shots dropped in the vessels be n.
Volume of water spilled = Volume of dropped lead shots
\[\dfrac{1}{4} \times \]Volume of cone \[{\text{ = n *}}\dfrac{{\text{4}}}{{\text{3}}}{{\text{r}}_{\text{2}}}^{\text{3}}\]
\[\dfrac{1}{4} \times \dfrac{1}{3}\pi {{\text{r}}_1}^2{\text{h = n * }}\dfrac{{\text{4}}}{{\text{3}}}{{\text{r}}_{\text{2}}}^{\text{3}}\]
\[{{\text{r}}_1}^2{\text{h = n *16}}{{\text{r}}_{\text{2}}}^{\text{3}}\]
\[{5^2} \times {\text{8 = n *16}} \times {\left( {0.5} \right)^{\text{3}}}\]
\[n = \dfrac{{25 \times 8}}{{16 \times {{\left( {\dfrac{1}{2}} \right)}^3}}} = 100\]
Therefore, the number of lead shots dropped in the vessel is \[100\]
6. A solid iron pole consists of a cylinder of height \[{\text{220cm}}\] and base diameter \[{\text{24cm}}\], which is surmounted by another cylinder of height \[{\text{60cm}}\] and radius \[{\text{8cm}}\]. Find the mass of the pole, given that \[{\text{1c}}{{\text{m}}^3}\]of iron has approximately \[{\text{8g}}\] mass.
Ans:
From the above figure, we observe that
Height (h1) of larger cylinder \[{\text{ = 220cm}}\]
Radius (r1) of larger cylinder \[{\text{ = }}\dfrac{{24}}{2}{\text{ = 12cm}}\]
Height (h2) of smaller cylinder \[{\text{ = 60cm}}\]
Radius (r2) of smaller cylinder \[{\text{ = 8cm}}\]
Total volume of pole = Volume of larger cylinder + volume of smaller cylinder
\[{\text{ =}}\pi{{\text{r}}_{\text{1}}}^{\text{2}}{{\text{h}}_{\text{1}}}{\text{ +}} \pi {{\text{r}}_{\text{2}}}^{\text{2}}{{\text{h}}_{\text{2}}}\]
\[{\text{ = }} \pi {\left( {12} \right)^{\text{2}}}* {\text{220 + }} \pi {\left( 8 \right)^{\text{2}}} * 60\]
${\text{ = }}\pi\left[ {{\text{(144 * 220) + (64 * 60)}}} \right] $
${\text{ = 35520 * 3}}{\text{.14 = 111532}}{\text{.8c}}{{\text{m}}^{\text{3}}} $
Mass of \[{\text{1c}}{{\text{m}}^3}\]iron = \[{\text{8g}}\]
Mass of \[{\text{111532}}{\text{.8c}}{{\text{m}}^{\text{3}}}\]iron \[{\text{ = 111532}}{\text{.8 * 8 = 892262}}{\text{.4g = 892}}{\text{.262kg}}\]
7. A solid consisting of a right circular cone of height \[{\text{120cm}}\] and radius \[{\text{60cm}}\] standing on a hemisphere of radius \[{\text{60cm}}\] is placed upright in a right circular cylinder full of water such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is \[{\text{60cm}}\] and its height is \[{\text{180cm}}\].
Ans:
Radius (r) of hemispherical part = Radius (r) of conical part\[{\text{ = 60cm}}\]
Height (h2) of conical part of solid\[{\text{ = 120cm}}\]
Height (h1) of cylinder\[{\text{ = 180cm}}\]
Radius (r) of cylinder\[{\text{ = 60cm}}\]
Volume of water left = Volume of cylinder − Volume of solid
= Volume of cylinder – (Volume of cone + Volume of hemisphere)
\[{\text{ = }}\pi{{\text{r}}^{\text{2}}}{{\text{h}}_{\text{1}}}{\text{ - }}\left( {\dfrac{{\text{1}}}{{\text{3}}}{\pi}{{\text{r}}^{\text{2}}}{{\text{h}}_{\text{2}}}{\text{ + }}\dfrac{{\text{2}}}{{\text{3}}} \pi {{\text{r}}^{\text{3}}}} \right)\]
\[{\text{ = }}\pi {\left( {60} \right)^{\text{2}}} \times 180{\text{ - }}\left( {\dfrac{{\text{1}}}{{\text{3}}}{\pi }{{\left( {60} \right)}^{\text{2}}} \times {\text{120 + }}\dfrac{{\text{2}}}{{\text{3}}}{\pi }{{\left( {60} \right)}^{\text{3}}}} \right)\]
\[{\text{ = }}\pi{\left( {60} \right)^{\text{2}}}\left[ {\left( {180} \right) - \left( {40 + 40} \right)} \right]\]
\[{\text{ = }}\pi\left( {{\text{3600}}} \right)\left( {{\text{100}}} \right){\text{ = 3,60,000 }}\pi{\text{c}}{{\text{m}}^{\text{3}}}{\text{ = 11311428}}{\text{.57c}}{{\text{m}}^{\text{3}}}{\text{ = 1}}{\text{.131}}{{\text{m}}^{\text{3}}}\]
8. A spherical glass vessel has a cylindrical neck \[{\text{8cm}}\] long, \[{\text{2cm}}\] in diameter; the diameter of the spherical part is \[{\text{8}}{\text{.5cm}}\]. By measuring the amount of water it holds, a child finds its volume to be \[{\text{345c}}{{\text{m}}^3}\]. Check whether she is correct, taking the above as the inside measurements, and \[\pi = 3.14\].
Ans:
Height (h) of cylindrical part \[{\text{ = 8cm}}\]
Radius (r2) of cylindrical part \[{\text{ = }}\dfrac{2}{2}{\text{ = 1cm}}\]
Radius (r1) spherical part \[{\text{ = }}\dfrac{{8.5}}{2}{\text{ = 4}}{\text{.25cm}}\]
Volume of vessel = Volume of sphere + Volume of cylinder \[{\text{ = }}\dfrac{4}{3}\pi {\left( {\dfrac{{8.5}}{2}} \right)^3}{\text{ + }}\pi {\left( 1 \right)^2}\left( 8 \right)\]
\[{\text{ = }}\dfrac{4}{3} \times 3.14 \times 76.765625{\text{ + 8}} \times {\text{3}}{\text{.14}}\]
\[ = 321.392 + 25.12\]
\[ = 346.512\]
\[{\text{ = 346}}{\text{.51c}}{{\text{m}}^{\text{3}}}\]
Therefore, she is wrong.
Conclusion
Ex 12.2 Class 10 Maths Chapter 12, Surface Areas and Volumes, is all about the volumes of different solid shapes and how they can be combined. Understanding how to compute the volumes of various geometric solids, such as cubes, cuboids, cylinders, cones, and spheres, as well as their combinations, requires completion of this task. For detailed solutions and step-by-step explanations, refer to the NCERT Solutions provided by Vedantu.
Class 10 Maths Chapter 12: Exercises Breakdown
CBSE Class 10 Maths Chapter 12 Other Study Materials
Chapter-Specific NCERT Solutions for Class 10 Maths
Given below are the chapter-wise NCERT Solutions for Class 10 Maths. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.
Study Resources for Class 10 Maths
For complete preparation of Maths for CBSE Class 10 board exams, check out the following links for different study materials available at Vedantu.
FAQs on NCERT Solutions for Class 10 Maths Chapter 12 Surface Areas And Volumes Ex 12.2
1. What will I study in this chapter?
Surface Areas and Volumes is one of the most fascinating chapters of Class 10 Maths. In CBSE Class 10 Maths Book Solutions, you will understand about the cuboid and its surface area, cube and its surface area, cylinder and it’s surface area, right circular cone, sphere and volume of cube, cuboid and cylinder. You will also come across the hemisphere and its surface area, combination of solids, shape conversion of solids and volume of a frustum. Our NCERT Solutions for Class 10 Maths Chapter 13 consists of 5 exercises with 38 questions in total and they are prepared by expert teachers based on the current Class 10 Maths syllabus.
2. How many questions are there in this exercise?
This exercise of Surface Areas and Volumes NCERT Solutions for Class 10 Maths Chapter 13 consist of 8 questions. Question 1, 2 4 and 7 are based on finding the volume of different shapes like the solid, the volume of air, the volume of wood, the volume of water. Question 3, 5 and 8 are scenario-based questions. Where incidents or situations are mentioned and you will have to find whether the given numbers are correct or not. Question 6 is based on mass, where you will have to find the mass of a pole.
3. Explain about Volume of Cube and Cylinder.
Volume of Cube
Here, in CBSE Class 10 Maths Chapter 13, you will learn that all the dimensions of the cube are identical, Volume = l3
Here, l will be the length of the cube of the edge
The volume of a cube = base area×height
Volume of Cuboid
Volume of a cuboid =(base area)×height=(lb)h=lbh
Volume of Cylinder
Volume of a cylinder = Base area × its height = (π×r2)×h=πr2h
In the later part of Class 10 Maths Surface Areas and Volumes, you will also come across the volume of a right circular cone, the volume of a sphere, the volume of a hemisphere, and hemisphere and it’s surface area.
4. How are the solutions for the exercise questions provided by Vedantu?
NCERT Solutions are explained in an easy to understand way. The solutions are to the extremity and suitable with high precision solutions for all the exercise questions. NCERT Solutions for Class 10 Maths are the most high-grade preparation materials which are equipped with the test point of representation. The answers are sketched as per the latest CBSE maths syllabus and CBSE NCERT Books.
All our NCERT answers will make learners build their conceptual knowledge of the concepts. They will also develop a strong foundation with the concepts as they are explained with examples and diagrams. NCERT Solutions by Vedantu are the most reliable study materials provided for smart education and effective answering of questions.
5. Which is the important section of Exercise 12.2 of Class 10 Maths?
Surface Area Combination Of Solids is the most important section for solving exercise 12.2 of NCERT class 10 Maths. But all other sections and exercises present in the chapter too are equally important from the exam point of view. To have a holistic understanding of the section, download NCERT Solutions Class 10 Maths Chapter 13 on Vedantu and get free of cost and full solutions to the 12.2 exercise. Practice these questions to improve your marks.
6. What to study in Exercise 12.2 of class 10 Mathematics?
In order to successfully solve exercise 12.2 of NCERT Class 10 Maths, you need to study the section called Surface Area Combination of Solids. This section will help you in solving the questions that have been asked in exercise 12.2 of the NCERT Maths textbook. For full step by step solutions of the exercise, download NCERT Solutions Class 10 Maths Chapter 13 Exercise 12.2 PDF available free of cost only on the Vedantu website (vedantu.com) and app.
7. Can you please brief me about the Class 10 Maths Chapter 13?
In Chapter 13 of Class 10 Maths, it's all about surface area and volume of 3-dimensional solids. On Vedantu, you can download the NCERT Solutions Class 10 Maths Chapter 13 PDF and get access to the full explanation and solutions of the Class 10 Maths Chapter 13 on Surface Area and Volume. All the exercises and examples have been solved step by step by experts.
8. What are the basics of Class 10 Maths Chapter 13?
The basics that you need to cover in Class 10 Maths Chapter 13 are Surface Area Of Combination Of Solids, Volume Of A Combination Of Solids, Conversion Of Shape From One Solid To Another, and Frustum Of A Cone. To get solutions to exercises related to these topics, you can download the NCERT Solutions Class 10 Maths Chapter 13 on Vedantu and practice daily to ensure good grades.
9. What are the important formulae to remember in Class 10 Maths Chapter 13?
Some of the most important formulae that you need to remember in completing your Class 10 Maths Chapter 13 are - Curved Surface Area Of Cone, Curved Surface Area Of Hemisphere, Height Of The Cone, Slant Height Of The Cone, Curved Surface Area Of Cylinder, Volume Of A Frustum Of Cone, Curved Surface Area Of A Frustum Of Cone, and Total Surface Area Of A Frustum Of Cone.
