Important Questions for CBSE Class 9 Maths Chapter 9 - Areas of Parallelograms and Triangles

1 Mark Questions

1. Find the area of the parallelogram in the adjoining figure.

(a) \[1759\] foot

(b) \[48\] square

(c) \[84\] square foot

(d) \[60\] square foot

Ans: (d) \[60\] square foot

2. A triangle has an area of \[45\] square foot. Base of the triangle is \[9\] foot. What is corresponding height of triangle

(a) \[90\]foot

(b) \[5\]foot

(c) \[10\]foot

(d) \[40\]square foot

Ans:  (c) \[10\] foot.

3. What is area of parallelogram whose base \[ = 8\] and corresponding altitude is \[5\].

(a) \[40\]

(b) \[45\]

(c) \[13\]

(d) \[3\]

Ans:  (a) \[40\]

4. Parallelograms on the same base and between the same parallels have equal

(i) corresponding angle

(ii) area

(iii) congruent area

(iv) same parallel
Ans:  (ii) Area

5. Any side of a parallelogram is called,
(i) Altitude

(ii) base

(iii) corres. Altitude

(iv) area
Ans:  (ii) base

6. A diagonal of a parallelogram divides into triangles of equal area

(i) \[1\]

(ii) \[2\]

(iii) \[3\]

(iv) none of these

Ans:  (ii) \[2\]

7. Find the area of parallelogram, if Base \[ = {\text{ }}3\]and altitude is \[4\]

(i) \[7\]

(ii) \[1\]

(iii) \[12\]

(iv) none of these

Ans:  (iii) \[12\]

8. Find the area of parallelogram ${\text{gm}}$, if base $ = 8\;{\text{cm}}$ and altitude $ = 10\;{\text{cm}}$,

(a) $80{\text{sq}}.{\text{cm}}$

(b) $80\;{\text{cm}}$

(c) \[30{\text{ }}sq.cm\]

(d) $50{\text{sq}}.{\text{cm}}$

Ans: (a) \[80{\text{ }}sq.cm\]

9. If Base \[ = 9\]and corresponding altitude \[ = 4.\]Find area of || gram

(a) \[4\]

(b) \[40\]

(c) \[36\]

(d) none of these

Ans: (c) \[36\]

10. If a triangle and a Parallelogram are on the same base and between the same parallel, the area of the triangle is equal to ____ that of | | gram.

(a) $\dfrac{1}{2}$

(b) $\dfrac{1}{3}$

(c) $\dfrac{1}{4}$

(d) none of these

Ans: (a) $\dfrac{1}{2}$

11. A parallelogram has an area of \[36\]square an and base of the parallelogram is $9\;{\text{cm}}$.

what is the corresponding altitude of parallelogram?

(a) $6\;{\text{cm}}$.

(b) $5\;{\text{cm}}$.

(c) $4\;{\text{cm}}$

(d) $3\;{\text{cm}}$.

Ans: (c) $4\;{\text{cm}}$.

12. A median of a triangle divides if into triangles of equal areas.

(a) \[1\]

(b) same triangle

(c) \[2\]

(d) none

Ans: (c) \[2\]

13. The area of a rhombus is equal to____ of the product of its two diagonals.

(a) $\dfrac{1}{2}$

(b) $\dfrac{1}{3}$

(c) $\dfrac{1}{4}$

(d) none

Ans: (a) $\dfrac{1}{2}$

14. Area of a triangle is half the product of any of its sides and the

(a) Corresponding altitude

(b) altitude

(c) median

(d) base

Ans: (a) Corresponding altitude

15. Given below are the measurements of a parallelogram. Find the missing measurement. Area $ = 90$ square ${\text{cm}}$, Base $ = 5\;{\text{cm}}$, Height ?

(a) \[18\]

(b) \[450\]

(c) \[85\]

(d) \[15\;{\text{cm}}\]

Ans: (a) \[18\]

16. How many square feet are in a square yard

(a) \[{\mathbf{6}}\]

(b) \[{\mathbf{9}}\]

(c) \[{\mathbf{12}}\]

(d) \[{\mathbf{10}}\]

Ans: (b) \[9\]

17. The perimeter of an equilateral triangle is \[21\] yard. what is the length of its each sides

(a) \[7\]yard

(b) \[14\]yard

(c) \[8\]yard

(d)\[\;12\] yard

Ans: (a) \[7\] yard

18. What is the area of a triangle with base $12\;{\text{m}}$ and a height of $18\;{\text{m}}$

(a) $208{m^2}$

(b) $126{m^2}$

(c) $108{m^2}$

(d) $98{m^2}$

Ans: (c) $108{m^2}$

19. Find the area of parallelogram if base $ = 8$ and corresponding Altitude $ = 4$

(a) \[12\]

(b) \[32\]

(c) \[4\]

(d) \[8\]

Ans: (b) \[32\]

2 Marks Questions

1. Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and the two parallels.

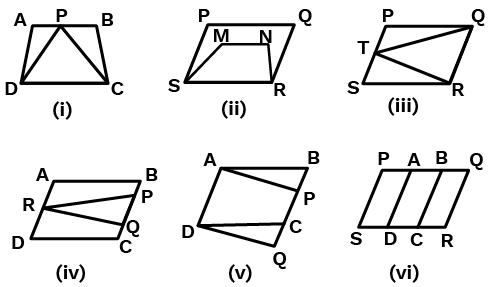
Ans: In figure (i): $\Delta {\text{DPC}}$ and trap. ${\text{ABCD}}$ are on the same base ${\text{DC}}$ and between same parallel ${\text{DC}}$ and ${\text{AB}}$.

In figure (iii): $\Delta $ RTQ and parallelogram PQRS are on the same base QR and between same parallel QR and PS.

In figure (v): Parallelogram ABCD and parallelogram APQD are on the same base ${\text{AD}}$ and between the same parallels \[AD\]and \[BQ.\]

2. In figure, ABCD is a parallelogram. $AE \bot DC$ and $CF \bot $ AD. If $AB = 16\;{\text{cm}},AE = 8\;{\text{cm}}$ and ${\text{CF}} = 10\;{\text{cm}}$, find ${\text{AD}}$.

Ans: ${\text{ABCD}}$ is a parallelogram.

$\therefore {\text{DC}} = {\text{AB}} \Rightarrow {\text{DC}} = 16\;{\text{cm}}$

$AE \bot DC$ [Given]

Now Area of parallelogram ${\text{ABCD}} = $ Base $ \times $ Corresponding height

$ = DC \times AE = 16 \times 8 = 128\;{\text{c}}{{\text{m}}^2}$

Using base AD and height CF, we can find,

Area of parallelogram $ = AD \times CF$

$ \Rightarrow 128 = AD \times 10$

$ \Rightarrow {\text{AD}} = \dfrac{{128}}{{10}} = 12.8\;{\text{cm}}$

3. If E, F, G and H are respectively the mid-points of the sides of a parallelogram ABCD, show that $\operatorname{ar} ({\text{EFGH}}) = \dfrac{1}{2}\operatorname{ar} ({\text{ABCD}})$

Ans: Given: A parallelogram ABCD. E, F, G and H are mid-points of AB, BC, CD and DA respectively.

To prove: \[ar\left( {EFGH} \right){\text{ }} = \;ar\dfrac{1}{2}\left( {ABCD} \right)\]

Construction: Join HF

Proof:  (i)

And  HABF $) \ldots  \ldots  \ldots ..$ (ii)

(If a triangle and a parallelogram are on the same base and between the same parallel then the area of triangle is half of area of parallelogram)

Adding eq. (i) and (ii),

$\operatorname{ar} (\Delta {\text{GHF}}) + \operatorname{ar} (\Delta {\text{HEF}})$

4. In the figure, PQRS and ABRS are parallelograms and $X$ is any point on the side BR. Show that:

(i) $\operatorname{ar} ({\mathbf{PQRS}}) = \operatorname{ar} ({\text{ABRS}})$

 Ans:  Parallelogram PQRS and ABRS are on the same base SR and between the same parallels SR and PB. $\therefore \operatorname{ar} ({\mathbf{PQRS}}) = \operatorname{ar} ({\text{ABRS}})$

(ii) $\operatorname{ar} ({\text{AXS}}) = \dfrac{1}{2}\operatorname{ar} ({\text{PQRS}})$

Ans: $\Delta {\text{AXS}}$ and  ABRS are on the same base AS and between the same parallels AS and BR.

5. In figure, $E$ is any point on median AD of a $\Delta ABC.$ Show that $\operatorname{ar} (\Delta ABE) = \operatorname{ar} (\Delta ACE)$

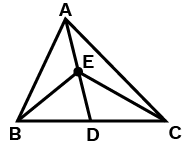
Ans: In $\Delta {\text{ABC}},{\text{AD}}$ is a median.

$\operatorname{ar} (\Delta {\text{ABD}}) = \operatorname{ar} (\Delta {\text{ACD}})$

$[\because $ Median divides $\Delta $ into two $\Delta {\text{s}}$ of equal area]

Again in $\Delta {\text{EBC}},{\text{ED}}$ is a median

$\operatorname{ar} (\Delta {\text{EBD}}) = \operatorname{ar} (\Delta {\text{ECD}}) \ldots  \ldots  \ldots ..{\text{ (ii) }}$

${\text{Subtracting}}{\kern 1pt} {\kern 1pt} \,{\text{eq}}{\text{.(ii)}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{from}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{(i)}},$

$\operatorname{ar} (\Delta {\text{ABD}}) - \operatorname{ar} (\Delta {\text{EBD}}) = \operatorname{ar} (\Delta {\text{ACD}}) - \operatorname{ar} (\Delta {\text{ECD}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{ABE}}) = \operatorname{ar} (\Delta {\text{ACE}})$

6. Show that ${\mathbf{DE}}$||${\mathbf{BC}}$ if $ar(\Delta BCE) = \operatorname{ar} (\Delta BCD)$

Ans: Given: $\triangle \mathrm{ABC}$

where $D$ and $E$ are points on sides $A B$ and $A C$ such that $\operatorname{ar}(\mathrm{DBC})=\operatorname{ar}(\mathrm{EBC})$

To prove: DE || BC

Proof:$\triangle \mathrm{DBC}$ and $\triangle \mathrm{EBC}$ are lying on a common base BC and have equal areas,

\& they lie between the lines $D E$ and $B C$.

So, DE ॥ BC

As two triangles having the same base and equal areas lie between the same parallels.

Hence proved.

7. In a triangle ABC, E is the mid-point of median AD. Show that ${\mathbf{ar}}({\text{BED}}) = \dfrac{1}{4}\operatorname{ar} ({\text{ABC}})$.

Ans: Given: A $\Delta {\text{ABC}},{\text{AD}}$ is the median and ${\text{E}}$ is the mid-point of median AD.

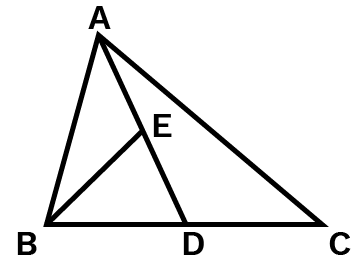
To prove: $\operatorname{ar} (\Delta {\text{BED}}) = \dfrac{1}{4}\operatorname{ar} (\Delta {\text{ABC}})$

Proof: In $\Delta {\text{ABC}},{\text{AD}}$ is the median.

$\therefore \operatorname{ar} (\Delta {\text{ABD}}) = \operatorname{ar} (\Delta {\text{ADC}})$

$[\because $ Median divides $\Delta $ into two $\Delta {\text{s}}$ of equal area]

$ \Rightarrow \operatorname{ar} (\Delta {\text{ABD}}) = \dfrac{1}{2}\operatorname{ar} ({\text{ABC}}) \ldots  \ldots  \ldots ..({\text{i}})$

In $\vartriangle {\text{ABD}},{\text{BE}}$ is the median.

$\therefore \operatorname{ar} (\Delta {\text{BED}}) = \operatorname{ar} (\Delta {\text{BAE}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{BED}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABD}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{BED}}) = \dfrac{1}{2} \times \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}}) = \dfrac{1}{4}\operatorname{ar} (\Delta {\text{ABC}})$

8. D and $E$ are points on sides\[AB\]and AC respectively of $\Delta $ ABC such that $ar(DBC) = ar\left( {EBC} \right).$ Prove that DE||BC.

(Image will be uploaded soon)

Ans: Given: $\operatorname{ar} (\Delta {\text{DBC}}) = \operatorname{ar} (\Delta {\text{EBC}})$

Since two triangles of the equal area have a common base BC.

$\therefore$  DE||BC

9. Diagonals AC and BD of a trapezium ABCD with AB  DC intersect each other at 0 . Prove that $\operatorname{ar} ({\text{AOD}}) = \operatorname{ar} ({\text{BOC}})$

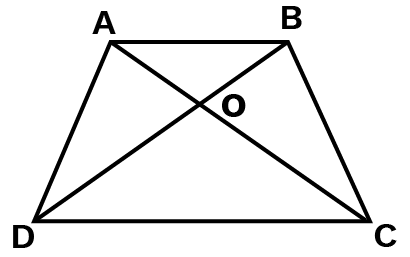
Ans: $\vartriangle {\text{ABD}}$ and $\Delta {\text{ABC}}$ lie on the same base ${\text{AB}}$ and between the same parallels ${\text{AB}}$ and ${\text{DC}}$.

$\therefore \operatorname{ar} (\Delta {\text{ABD}}) = \operatorname{ar} (\Delta {\text{ABC}})$

${\text{Subtracting}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{ar(}}\vartriangle {\text{AOB}})$ from both sides,

$\operatorname{ar} (\Delta {\text{ABD}}) - \operatorname{ar} (\Delta {\text{AOB}})$

$ = \operatorname{ar} (\Delta {\text{ABC}}) - \operatorname{ar} (\Delta {\text{AOB}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{AOD}}) = \operatorname{ar} (\Delta {\text{BOC}})$

10. In figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that:

(i) $ar(ACB = \operatorname{ar} (ACF)$

Ans: Given that BF  AC

$\Delta {\text{ACB}}$ and $\Delta {\text{ACF}}$ lie on the same base ${\text{AC}}$ and between the same parallels ${\text{AC}}$ and ${\text{BF}}$.

$\therefore \operatorname{ar} (\Delta {\text{ACB}}) = \operatorname{ar} (\Delta {\text{ACF}}) \ldots  \ldots  \ldots ..{\text{ (i) }}$

(ii)\[ar\left( {AEDF} \right){\text{ }} = {\text{ }}ar\left( {ABCDE} \right)\]

Ans: Now $ar({\text{ABCDE}}) = {\text{ar}}(trap.\;{\text{AEDC}}) + \operatorname{ar} (\Delta {\text{ABC}}) \ldots  \ldots  \ldots $(ii)

$ \Rightarrow \operatorname{ar} ({\text{ABCDE}}) = \operatorname{ar} ($ trap. ${\text{AEDC}}) + \operatorname{ar} (\Delta {\text{ACF}}) = \operatorname{ar} \left( {quad.{\text{ }}AEDF} \right)$ 

[Using (i)]

$ \Rightarrow \operatorname{ar} ({\text{AEDF}}) = \operatorname{ar} ({\text{ABCDE}})$

11. In figure, . Prove that $\operatorname{ar} ({\text{AQC}}) = \operatorname{ar} ({\mathbf{PBR}})$.

Ans: $\vartriangle {\text{ABQ}}$ and BPQ lie on the same base BQ and between same parallels AP and BQ.

$\therefore \operatorname{ar} (\Delta {\text{ABQ}}) = \operatorname{ar} (\Delta {\text{BPQ}}) \ldots  \ldots  \ldots ..({\text{i}})$

$\Delta {\text{BQC}}$ and $\Delta {\text{BQR}}$ lie on the same base ${\text{BQ}}$ and between same parallels ${\text{BQ}}$ and ${\text{CR}}$.

$\therefore \operatorname{ar} (\Delta {\text{BQC}}) = \operatorname{ar} (\Delta {\text{BQR}}) \ldots  \ldots  \ldots $ (ii)

Adding eq (i) and (ii), 

$ar(\Delta {\text{ABQ}}) + \operatorname{ar} (\Delta {\text{BQC}}) = \operatorname{ar} (\Delta {\text{BPQ}}) + \operatorname{ar} (\Delta {\text{BQR}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{AQC}}) = \operatorname{ar} (\Delta {\text{PBR}})$

12. In figure, $D$ and $E$ are two points on \[BC\]such that $BD = DE = EC$. Show that $\operatorname{ar} (ABD) = ar{\text{ }}\left( {ADE} \right){\text{ }} = {\text{ }}ar{\text{ }}\left( {AEC} \right).$Can you know answer the question that you have left in the 'introduction' of this chapter, whether the field of Budhia has been actually divided into three parts of equal area?

Ans: In $\Delta {\text{ABC}}$, points ${\text{D}}$ and ${\text{E}}$ divides ${\text{BC}}$ in three equal parts such that ${\text{BD}} = {\text{DE}} = {\text{EC}}$.

$\therefore {\text{BD}} = {\text{DE}} = {\text{EC}} = \dfrac{1}{3}{\text{BC}}$

Draw AF $ \bot BC$

$\operatorname{ar} (\Delta {\text{ABC}}) = \dfrac{1}{2} \times BC \times AF$

and $ar(\Delta {\text{ABD}}) = \dfrac{1}{2} \times BD \times A{F_{ \ldots  \ldots  \ldots ...}}$ (ii)

$ = \dfrac{1}{2} \times \dfrac{{{\text{BC}}}}{3} \times {\text{AF}} = \dfrac{1}{3} \times \left[ {\dfrac{1}{2} \times {\text{BC}} \times {\text{AF}}} \right]$

$ = \dfrac{1}{3}\operatorname{ar} (\Delta {\text{ABC}}) \ldots  \ldots  \ldots ..$ (iii)

And $\operatorname{ar} (\Delta {\text{AEC}}) = \dfrac{1}{3}\operatorname{ar} (\Delta {\text{ABC}}) \ldots  \ldots  \ldots $ (iv)

From (ii), (iii) and (iv),

$\operatorname{ar} (\Delta {\text{ABD}}) = \operatorname{ar} (\Delta {\text{ADE}}) = \operatorname{ar} (\Delta {\text{AEC}})$

13. In figure, ABCD, DCFE and ABFE are parallelograms. Show that \[ar\left( {ADE} \right){\text{ }} = \;ar\left( {BCF} \right).\]

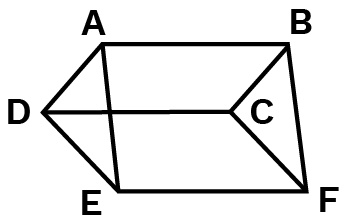
Ans: As we know that opposite sides of a parallelogram are always equal.

$\therefore $ In parallelogram ${\text{ABFE}},{\text{AE}} = {\text{BF}}$ and ${\text{AB}} = {\text{EF}}$

In parallelogram ${\text{DCFE}},{\text{DE}} = {\text{CF}}$ and ${\text{DC}} = {\text{EF}}$

In parallelogram ${\text{ABCD}},{\text{AD}} = {\text{BC}}$ and ${\text{AB}} = {\text{DC}}$

Now in $\Delta {\text{ADE}}$ and $\Delta {\text{BCF}}$,

${\text{AE}} = {\text{BF}}$ (Opposite sides of parallelogram ABFE)

${\text{DE}} = {\text{CF}}$ (Opposite sides of parallelogram DCFE)

And AD = BC (Opposite sides of parallelogram ABCD)

$\therefore \Delta {\text{ADE}} \cong \Delta {\text{BCF}}[{\text{By SSS congruency }}]$

$\therefore \operatorname{ar} (\Delta {\text{ADE}}) = \operatorname{ar} (\Delta {\text{BCF}})$

$[\because $ Area of two congruent figures is always equal]

14. Prove that ABCD is a parallelogram. If ABCD is a quadrilateral and BD is one of its diagonal.

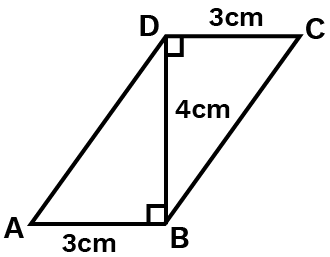
Ans: Given quadrilateral ${\text{ABCD}}$ in which ${\text{AB}} = {\text{DC}} = 3,{\text{BD}} = 4$ and $\angle AB = \angle BDC = {90^\circ }$ BD intersects ${\text{AB}}$ and ${\text{DC}}$ such that.

$\angle ABD = \angle BDC = {90^\circ }$ 

(alternate interior angles are equal) but $AB = DC = 3$

Thus, ${\text{ABCD}}$ is a parallelogram.

15. In a parallelogram ${\text{ABCD}},{\text{AB}} = 20.$ The altitude DM to sides ${\text{AB}}$ is ${\mathbf{10}}{\text{cm}}$. Find area of the parallelogram.

Ans: Area of parallelogram ABCD

$ = {\text{AB}} \times {\text{DM}}$

$ = 20 \times 10$

$ = 200$ square ${\text{cm}}$.

16. If $L$ be any Point on AB and the area of rectangle \[ABCD\]is 100 square ${\text{cm}}$. find area of $\Delta LCD$

Ans: Area of rectangle ${\text{ABCD}} = 100$ square ${\text{cm}}$

Area $\Delta LCD = \dfrac{1}{2}$ area rectangle ABCD

$ = \dfrac{1}{2} \times 100{\text{ square cm}}$

$ = 50$ square ${\text{cm}}$

17. Find the area of parallelogram ABCD, BD is perpendicular on AB. ${\text{AB}} = 7$ and ${\text{BD}}$ is 5.

Ans: Area of parallelogram \[ = \] Base $ \times $ Corresponding Altitude $ = 7 \times 5$

$ = 35$ square ${\text{cm}}$

Area of parallelogram $ = 35$ square ${\text{cm}}$

18. Show that $\operatorname{ar} ({\text{ABC}}) = \operatorname{ar} $ (ABD). ${\text{ABC}}$ and ${\text{ABD}}$ are two triangles on the same base ${\text{AB}}$ if line segment ${\text{CD}}$ is bisected by ${\text{A}}{\mathbf{O}}$ at ${\mathbf{O}}$

Ans: AO is the median of $\vartriangle ACD$

$\operatorname{ar} (\Delta AOC) = \operatorname{ar} (\Delta AOD)$

$\operatorname{ar} (\Delta BOC) = \operatorname{ar} (\vartriangle BOD)$

$\operatorname{ar} (\Delta AOC) + \operatorname{ar} (\Delta BOC) = \operatorname{ar} (\Delta AOD) + \operatorname{ar} (\Delta BOD)$

$\operatorname{ar} (\Delta ABC) = \operatorname{ar} (\Delta ABD)$

19. Show that BDEF is a parallelogram. If D, E, and F the mid- points of the side BC, CA, and AB of triangle ABC.

Ans: Given: A $\triangle \mathrm{ABC}$ in which $\mathrm{D}, \mathrm{E}, \mathrm{F}$ are the mid-point of the side $\mathrm{BC}$, CA and $\mathrm{AB}$ respectively.

Proof:

In $\triangle \mathrm{ABC}$,

$\therefore \mathrm{F}$ is the mid-point of side $\mathrm{AB}$ and $\mathrm{E}$ is the midpoint of side $\mathrm{AC}$.

$\therefore$ EF $\|$ BD : Line joining the mid-points of any two sides of a $\Delta$ is parallel to the third side.

Similarly,

ED $\| \mathrm{FB}$.

Hence, BDEF is a parallelogram.

Hence Proved.

20. Prove that $ar(\Delta O L P)=\operatorname{ar}(\Delta M N L) \text { if } \mathrm{MN}|| \mathrm{PO}$

Ans:  $a r(\Delta M P O)=\operatorname{ar}(\Delta M P N)$

$\operatorname{ar}(\Delta M P O)-\operatorname{ar}(\Delta M P L)=\operatorname{ar}(\Delta M P N)-\operatorname{ar}(\Delta M P L)$

$\operatorname{ar}(\Delta O L P)=\operatorname{ar}(\Delta M L N)$

21. Justify the line corresponding to side EF if $ar(\Delta ABC) = \operatorname{ar} (\Delta DEF)$ in $\Delta ABC,AB = 8$ and altitude ${\text{AB}}$ is $5\;{\text{cm}}$ and $\vartriangle DEF,EF = 10\;{\text{cm}}$

Ans: Given that $\operatorname{ar} (ABC) = \operatorname{ar} (\Delta DEF)$

$\dfrac{1}{2} \times AB \times AM = \dfrac{1}{2} \times EF \times DN$

$\dfrac{1}{2} \times 8 \times 5 = \dfrac{1}{2} \times 10 \times DN$

$20 = 5DN$

$DN = 4\;{\text{cm}}$

22. In a parallelogram PQRS, $PQ = 6\;{\text{cm}}$ and the corresponding altitude \[ST\]is $5\;{\text{cm}}$. find area of parallelogram.

Ans: Area of || gm PQRS

\[ = \]Base $ \times $ Altitude

$ = 6 \times 5$ (Square cm)

$ = 30$ square ${\text{cm}}$

23. Show that the median of a triangle divides it into two triangles of equal area.

Ans: Let $\mathrm{ABC}$ be a triangle and Let $\mathrm{AD}$ be one of its medians.

In $\triangle \mathrm{ABD}$ and $\triangle \mathrm{ADC}$ the vertex is common and these bases $\mathrm{BD}$ and $\mathrm{DC}$ are equal.

Draw $\mathrm{AE} \perp \mathrm{BC}$.

Now area $(\triangle \mathrm{ABD})=\dfrac{1}{2} \times$ base $\times$ altitude of $\triangle \mathrm{ADB}$

$=\dfrac{1}{2} \times \mathrm{BD} \times \mathrm{AE}$

$=\dfrac{1}{2} \times \mathrm{DC} \times \mathrm{AE}(\because \mathrm{BD}=\mathrm{DC})$

but DC and AE is the base and altitude of $\triangle \mathrm{ACD}$

$=\dfrac{1}{2} \times$ base DC $\times$ altitude of $\triangle \mathrm{ACD}$

$=$ area $\triangle \mathrm{ACD}$

$\Rightarrow \operatorname{area}(\triangle \mathrm{ABD})=\operatorname{area}(\triangle \mathrm{ACD})$

Hence the median of a triangle divides it into two triangles of equal areas.

24. The area of rectangle PQRS is 500 sq cm. if ${\text{T}}$ be any Point on PQ, find area of $\Delta TRS.$

Ans: As of $\Delta TRS = \dfrac{1}{2}$ as rectangle PQRS

$ = \dfrac{1}{2} \times 500$ Square ${\text{cm}}$ 

$ = 250$ square ${\text{cm}}$

25. Prove that as $(\Delta ROS) = $ ar $(\Delta PQO)$ if ${\mathbf{PS}}||{\mathbf{RQ}}$

Ans: $\operatorname{ar} (\Delta PSR) = \operatorname{ar} (\Delta PSQ)$

$\operatorname{ar} (\Delta PSR) - \operatorname{ar} (\Delta PSO) = \operatorname{ar} (\Delta PSQ) - \operatorname{ar} (\Delta PSO)$

$\operatorname{ar} (\Delta ROS) = \operatorname{ar} (\Delta PQO)$

26. Show that $ar(quad.{\text{ABCD}}) = \dfrac{1}{2}{\text{BD}}$ (AM+CN) ${\text{BD}}$ is one of the diagonals of a quadrilateral ABCD, AM and CN are the $ \bot $ from A and C

Ans: $ar\;quadABCD) = \operatorname{ar} (\Delta ABD) + \operatorname{ar} (\Delta BCD)$

$ = \dfrac{1}{2}(BD \times AM) + \dfrac{1}{2}(BD \times CN)$

$ = \dfrac{1}{2}BD(AM + CN)$

27. D, E, F are respectively the mid-points of the sides ${\text{BC}},{\text{CA}}$ and ${\text{AB}}$ of $\vartriangle ABC$ Prove that $\operatorname{ar} (\Delta {\mathbf{DEF}}) = \dfrac{1}{4}\operatorname{ar} (\Delta {\mathbf{ABC}})$

Ans: $\operatorname{ar} (\Delta BDF) = \operatorname{ar} (\Delta DEF)$

Now, 

$ = 2 \times \dfrac{1}{4}\operatorname{ar} (\Delta ABC)$

$ = \dfrac{1}{2}\operatorname{ar} (\Delta ABC)$

28. In a parallelogram PQRS, PS = 12. The altitude to side PS is equal to $12\;{\text{cm}}$. find area of parallelogram PQRS?

Ans: Area of parallelogram PQRS

\[ = \]Base $ \times $ Corresponding Altitude

$ = 12 \times 12$

$ = 144$ square cm

29. A line through D, Parallel to AC meets BC produced in P. prove that area $\Delta ABP = \operatorname{arABCD} $

Ans: $\operatorname{ar} (\Delta ACP) = \operatorname{ar} (\Delta ACD)$

$\operatorname{ar} (\Delta ACP) + \operatorname{ar} (\Delta ABC) = \operatorname{ar} (\Delta ACD) + \operatorname{ar} (\Delta ABC)$

$ar(\Delta ABP) = ar(quadABCD)$ 

30. In a parallelogram PQRS, ${\text{PQ}} = 13.$ The altitude corresponding to sides ${\text{PQ}}$ is equal to $5\;{\text{cm}}$. find the area of parallelogram.

Ans: Area of parallelogram = base $ \times $ Altitude

$ = 13 \times 5$

$ = 65\;{\text{cm}}$

31. Prove that \[ar\left( {AOC} \right) = ar\left( {BOD} \right).\]Diagonals AC and BD of a trapezium ABCD with ${\text{AB}}||{\text{DC}}$ intersect each other at ${\text{O}}$.

Ans: $\operatorname{Ar} (\Delta ADC) = \operatorname{ar} (\Delta BDC)$

$\operatorname{ar} (\Delta ADC) - \operatorname{ar} (\Delta ODC) = \operatorname{ar} (\Delta BDC) - \operatorname{ar} (\Delta ODC)$

$\operatorname{ar} (\Delta AOC) = \operatorname{ar} (\Delta BOD)$

32. Prove that $\operatorname{ar} ({\text{AQC}}) = \operatorname{ar} ({\mathbf{PBR}})$ if ${\mathbf{AP}}||{\text{BQ}}||{\mathbf{CR}}$.

Ans: $\operatorname{ar} (\Delta ABQ) = \operatorname{ar} (\Delta PBQ)$

$\operatorname{ar} (\Delta CBQ) = \operatorname{ar} (\Delta RBQ)$

$\operatorname{ar} (\Delta ABQ) + \operatorname{ar} (\Delta CBQ) = \operatorname{ar} (\Delta PBQ) + \operatorname{ar} (\Delta PBQ)$

$\operatorname{ar} (AQC) = \operatorname{ar} (PBR)$

3 Marks Questions

1. ${\text{P}}$ and ${\mathbf{Q}}$ are any two points lying on the sides ${\text{DC}}$ and ${\text{AD}}$ respectively of a parallelogram ABCD. Show that $ar({\text{APB}}) = \operatorname{ar} ({\text{BQC}})$.

Ans: Given: ${\text{ABCD}}$ is a parallelogram. ${\text{P}}$ is a point on ${\text{DC}}$ and ${\text{Q}}$ is a point on ${\text{AD}}$.

To prove: $ar{\text{ }}(\Delta {\text{APB}}) = \operatorname{ar} (\Delta {\text{BQC}})$

Construction: Draw PM  and .

Proof: Since ${\text{QC}}$ is the diagonal of parallelogram QNCD.

Again ${\text{BQ}}$ is the diagonal of parallelogram ABNQ.

AP is the diagonal of  gm AMPD.

And ${\text{PB}}$ is the diagonal of  PCBM.

$\operatorname{ar} (\Delta {\text{BQC}}) = \operatorname{ar} (\Delta {\text{APB}}){\text{ or }}\operatorname{ar} (\Delta {\text{APB}}) = \operatorname{ar} (\Delta {\text{BQC}})$

2. A farmer was having a field in the form of a parallelogram PQRS. She took any point A on RS and joined it to points P and Q. In how many parts the field is divided? What are the shapes of these parts? The farmer wants to sow wheat and pulses in equal portions of the field separately. How should she do it?

Ans: When A is joined with P and Q; the field is divided into three parts viz. $\Delta {\text{PAS}},\Delta {\text{APQ}}$ and $\Delta {\text{AQR}}$

$\vartriangle $ APQ and parallelogram PQRS are on the same base PQ and between same parallels PQ and SR.

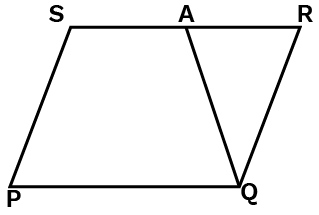
It implies that triangular region APQ covers a half portion of parallelogram-shaped field PQRS. So if a farmer sows wheat in triangular-shaped field APQ then she will definitely sow pulses in the other two triangular parts PAS and AQR.

Or

When she sows pulses in triangular-shaped field APQ then she will sow wheat in other two triangular parts PAS and AQR.

3. Show that the diagonals of a parallelogram divide it into four triangles of equal area.

Ans: Let parallelogram be ABCD and its diagonals AC and BD intersect each other at ${\text{O}}$.

In $\Delta {\text{ABC}}$ and $\vartriangle {\text{ADC}}$,

${\text{AB}} = {\text{DC}}$ (Opposite sides of a parallelogram)

${\text{BC}} = {\text{AD}}$ (Opposite sides of a parallelogram)

And $AC = $ AC (Common)

$\therefore \Delta {\text{ABC}} \cong \Delta {\text{CDA}}[{\text{ By SSS congruency }}]$

Since, diagonals of a parallelogram bisect each other.

$\therefore {\text{O}}$ is the mid-point of bisection.

Now in $\vartriangle $ ADC, DO is the median.

$\therefore \operatorname{ar} (\Delta {\text{AOD}}) = \operatorname{ar} (\Delta {\text{COD}})$

(Median divides a triangle into two equal areas)

Similarly, in $\vartriangle {\text{ABC}},{\text{OB}}$ is the median.

$\therefore \operatorname{ar} (\Delta {\text{AOB}}) = \operatorname{ar} (\Delta {\text{BOC}}) \ldots  \ldots  \ldots ..{\text{ (ii) }}$

And in $\Delta {\text{AOB}}$ and $\Delta {\text{AOD}}$, AO is the median.

$\therefore \operatorname{ar} (\Delta {\text{AOB}}) = \operatorname{ar} (\Delta {\text{AOD}}) \ldots  \ldots  \ldots .{\text{ (iii) }}$

${\text{From}}{\kern 1pt} \,{\kern 1pt} {\text{eq}}{\text{.(i),}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{(ii)}}{\kern 1pt} \,{\kern 1pt} {\text{and}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{(iii)}},$

$\operatorname{ar} (\Delta {\text{AOB}}) = \operatorname{ar} (\Delta {\text{AOD}}) = \operatorname{ar} (\Delta {\text{BOC}}) = \operatorname{ar} (\Delta {\text{COD}})$

Thus diagonals of parallelogram divide it into four triangles of equal area.

4.  In figure, ${\text{ABC}}$ and ${\text{ABD}}$ are two triangles on the same base ${\text{AB}}$. If line-segment ${\text{CD}}$ is bisected by ${\text{AB}}$ at ${\text{O}}$, show that $\operatorname{ar} ({\text{ABC}}) = {\text{ar}}$ (ABD).

Ans: Draw ${\text{CM}} \bot {\text{AB}}$ and ${\text{DN}} \bot {\text{AB}}$.

In $\Delta {\text{CMO}}$ and $\Delta {\text{DNO}}$

$\angle {\text{CMO}} = \angle {\text{DNO}} = {90^\circ }[$ (By construction)

 $\angle {\text{COM}} = \angle {\text{DON}}$ (Vertically opposite)

${\text{OC}} = {\text{OD}}$ (Given)

$\therefore \Delta {\text{CMO}} \cong \Delta {\text{DNO}}$ (By ASA congruency)

$\because \quad AM = DN[$ By C P C T]$......(i)$

${\text{Now}}{\kern 1pt} {\kern 1pt} \,{\kern 1pt} \,ar(\Delta {\text{ABC}}) = \dfrac{1}{2} \times AB \times CM$ ………(ii)

$\operatorname{ar} (\Delta {\text{ADB}}) = \dfrac{1}{2} \times AB \times DN.......{\text{ }}$(iii)

${\text{Using}}{\kern 1pt} {\kern 1pt} {\text{eq}}{\text{.(i)}}{\kern 1pt} {\kern 1pt} {\text{and}}{\kern 1pt} \,{\text{(iii),}}$

$\operatorname{ar} (\Delta {\text{ADB}}) = \dfrac{1}{2} \times AB \times CM$ (iv)

${\text{From}}{\kern 1pt} {\kern 1pt} {\kern 1pt} \,{\text{eq}}{\text{.(ii)}}{\kern 1pt} {\kern 1pt} {\text{and}}{\kern 1pt} \,{\text{(iv),}}$

$\operatorname{ar} (\Delta {\text{ABC}}) = \operatorname{ar} (\Delta {\text{ADB}})$

5. XY is a line parallel to side BC of triangle ABC. If BE  AC and CF  AB meet XY at E and F respectively, show that ${\mathbf{ar}}({\text{ABE}}) = \operatorname{ar} ({\text{ACF}})$

Ans: $\Delta {\text{ABE}}$ and parallelogram BCYE lie on the same base ${\text{BE}}$ and between the same parallels ${\text{BE}}$ and ${\text{AC}}$.

Also, $\Delta $ ACF and gm BCFX lie on the same base \[CF\]and between the same parallel BX and CF.

But || gm BCYE and || gm BCFX lie on the same base ${\text{BC}}$ and between the same parallels BC and EF.

…………(iii)

From eq. (i), (ii) and (iii), we get,

$\operatorname{ar} (\Delta {\text{ABE}}) = \operatorname{ar} (\Delta {\text{ACF}})$

6. The side AB of parallelogram ABCD is produced to any point P. A line through A and parallel to CP meets CB produced at Q and then parallelogram PBQR is completed. Show that $\operatorname{ar} ({\text{ABCD}}) = \operatorname{ar} ({\text{PBQR}})$

Ans: Given: ${\text{ABCD}}$ is a parallelogram, CP  and ${\text{PBQR}}$ is a parallelogram.

To prove: $ar({\text{ABCD}}) = \operatorname{ar} ({\text{PBQR}})$

Construction: Join AC and QP.

Proof: Since AQ  CP

$\therefore \operatorname{ar} (\Delta {\text{AQC}}) = \operatorname{ar} (\Delta {\text{AQP}})$

Subtracting  $ar(\vartriangle {\text{ABQ}})$ from both sides, we get

$\operatorname{ar} (\Delta {\text{AQC}} - \operatorname{ar} (\Delta {\text{ABQ}}) = \operatorname{ar} (\Delta {\text{AQP}}) - \operatorname{ar} (\Delta {\text{ABQ}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{ABC}}) = \operatorname{ar} (\Delta {\text{QBP}})$

Now we get,

$\operatorname{ar} ({\text{ABCD}}) = \operatorname{ar} ({\text{PBQR}})$

7. A villager Itwaari has a plot of land of the shape of quadrilateral. The Gram Panchayat of two villages decided to take over some portion of his plot from one of the corners to construct a health centre. Itwaari agrees to the above personal with the condition that he should be given equal amount of land in lieu of his land adjoining his plot so as to form a triangular plot. Explain how this proposal will be implemented.

Ans: Let Itwaari has land in shape of quadrilateral PQRS.

Draw a line through 5 parallel to PR, which meets QR produced at M.

Let diagonals PM and RS of new formed quadrilateral intersect each other at point N.

We have PR  SM (By construction)

$\therefore $ $ar(\Delta {\text{PRS}}) = \operatorname{ar} (\Delta {\text{PMR}})$

Subtracting  $ar(\Delta {\text{PNR}})$ from both sides,

$\operatorname{ar} (\Delta {\text{PRS}}) - \operatorname{ar} (\Delta {\text{PNR}}) = \operatorname{ar} (\Delta {\text{PMR}}) - \operatorname{ar} (\Delta {\text{PNR}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{PSN}}) = \operatorname{ar} (\Delta {\text{MNR}})$

It implies that Itwaari will give a corner triangular shaped plot PSN to the Gram panchayat for health centre and will take an equal amount of land (denoted by $\Delta $ MNR) adjoining his plot so as to form a triangular plot PQM. 

8. ABCD is a trapezium with AB  DC. A line parallel to AC intersects AB at X and BC at Y. Prove that $\operatorname{ar} ({\text{ADX}}) = \operatorname{ar} ({\text{ACY}})$

Ans: Join CX, $\Delta $ ADX and ACX lie on the same base XA and between the same parallels \[XA\]and \[DC.\]

$\therefore \operatorname{ar} (\Delta {\text{ADX}}) = \operatorname{ar} (\Delta {\text{ACX}})$ (i)

Also $\Delta {\text{ACX}}$ and $\Delta {\text{ACY}}$ lie on the same base

AC and between same parallels ${\text{CY}}$ and ${\text{XA}}$.

$\therefore \operatorname{ar} (\Delta {\text{ACX}}) = \operatorname{ar} (\Delta {\text{ACY}})$(ii)

${\text{From}}{\kern 1pt} \,\,{\text{(i)}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{and}}{\kern 1pt} {\kern 1pt} {\text{(ii),}}$

$\operatorname{ar} (\Delta {\text{ADX}}) = \operatorname{ar} (\Delta {\text{ACY}})$

9. Diagonals AC and BD of a quadrilateral ABCD intersect at 0 in such a way that\[ar\left( {AOD} \right) = ar\left( {BOC} \right)\]. Prove that ABCD is a trapezium.

Ans: Given that ${\mathbf{ar}}(\Delta {\text{AOD}}) = \operatorname{ar} (\Delta {\text{BOC}})$

Adding $\Delta {\text{AOB}}$ both sides,

$\operatorname{ar} (\Delta {\text{AOD}}) + \operatorname{ar} (\Delta {\text{AOB}}) = \operatorname{ar} (\Delta {\text{BOC}}) + \operatorname{ar} (\Delta {\text{AOB}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{ABD}}) = \operatorname{ar} (\Delta {\text{ABC}})$

Since if two triangles equal in area, lie on the same base then, they lie between same parallels. We have $\Delta {\text{ABD}}$ and $\Delta {\text{ABC}}$ lie on common base ${\text{AB}}$ and are equal in area.

$\therefore $ They lie in same parallels \[AB\]and \[DC.\]

Now in quadrilateral ABCD, we have AB || DC

Therefore ${\text{ABCD}}$ is trapezium.

10. In figure, $\operatorname{ar} ({\text{DRC}}) = \operatorname{ar} ({\text{DPC}})$ and ${\text{ar}}({\text{BDP}}) = {\text{ar}}\left( {{\mathbf{ARC}}} \right).$ Show that both the quadrilaterals ABCD and DCPR are trapeziums.

Ans: It is given that

Area $(\Delta D R C)=$ Area $(\Delta D P C)$

As $\triangle \mathrm{DRC}$ and $\triangle \mathrm{DPC}$ lie on the same base $\mathrm{DC}$ and have equal areas, therefore, they must lie between the same parallel lines.

$\therefore \mathrm{DC} \| \mathrm{RP}$

Therefore, DCPR is a trapezium.

It is also given that

Area $(\Delta B D P)=$ Area $(\triangle \mathrm{ARC})$

$\Rightarrow$ Area $(\Delta B D C)=$ Area $(\Delta A D C)$

Since $\triangle \mathrm{BDC}$ and $\triangle \mathrm{ADC}$ are on the same base $\mathrm{CD}$ and have equal areas, they must lie between the same parallel lines. $\therefore \mathrm{AB} \| \mathrm{CD}$

Therefore, $\mathrm{ABCD}$ is a trapezium.

11. Parallelogram ABCD and rectangle ABEF are on the same base AB and have equal areas. Show that the perimeter of the parallelogram is greater than that of the rectangle.

Ans: Given: Parallelogram ABCD and rectangle ABEF are on same base AB and between the same parallels ${\text{AB}}$ and ${\text{CF}}$. 

To prove: ${\text{AB}} + {\text{BC}} + {\text{CD}} + {\text{AD}} > {\text{AB}} + {\text{BE}} + {\text{EF}} + {\text{AF}}$

Proof: ${\text{AB}} = {\text{CD}}$

${\text{AB}} = {\text{EF}}$ 

$\therefore {\text{CD}} = {\text{EF}}$

Adding AB both sides,

${\text{AB}} + {\text{CD}} = {\text{AB}} + {\text{EF}}$

$\therefore $ Off all the segments that can be drawn to a given line from a point not lying on it, the perpendicular segment is the shortest.

$\therefore {\text{BE}} < {\text{BC}}$ and ${\text{AF}} < {\text{AD}}$

$ \Rightarrow {\text{BC}} > {\text{BE}}$ and ${\text{AD}} > {\text{AF}}$

12. In figure, $A B C D$ is a parallelogram and BC is produced to a point $Q$ such that $AD = $ CQ. If AQ intersects DC at P, show that $\operatorname{ar} (BPC) = \operatorname{ar} (DPQ)$.

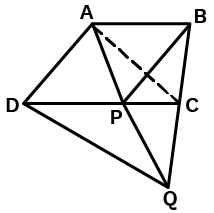
Ans: Join ${\text{A}}$ and ${\text{C}}$.

$\Delta {\text{APC}}$ and $\Delta {\text{BPC}}$ are on the same base ${\text{PC}}$ and between the same parallels ${\text{PC}}$ and ${\text{AB}}$.

$\therefore $ $ar(\Delta {\text{APC}}) = \operatorname{ar} (\Delta {\text{BPC}}) \ldots  \ldots  \ldots ..({\text{i}})$

Now ACBD is a parallelogram.

${\text{AD}} = {\text{BC}}$ (opposite sides of a parallelogram are always equal)

Also ${\text{BC}} = {\text{CQ}}$ (given)

$\therefore {\text{AD}} = {\text{CQ}}$

Now AD  [Since ${\text{CQ}}$ is the extension of ${\text{BC}}]$

And $AD = CQ$

$\therefore $ ADQC is a parallelogram.

Since diagonals of a parallelogram bisect each other.

$\therefore {\text{AP}} = {\text{PQ}}$ and ${\text{CP}} = {\text{DP}}$

Now in $\Delta $ APC and $\vartriangle DPQ$,

${\text{AP}} = {\text{PQ}}$ (Proved above)

$\angle $ APC $ = \angle $ DPQ (Vertically opposite angles)

${\text{PC}} = {\text{PD}}$ (Prove above)

$\therefore \Delta {\text{APC}} \cong \Delta {\text{DPQ}}$

$ \Rightarrow $ $ar(\Delta {\text{APC}}) = \operatorname{ar} (\Delta {\text{DPQ}})$ (area of congruent figures is always equal)

From eq. (i) and (ii),

$\operatorname{ar} (\Delta {\text{BPC}}) = \operatorname{ar} (\Delta {\text{DPQ}})$

13. PQRS is a quadrilateral and SQ is one of its diagonals. Show that PQRS is a Parallelogram and find its area too.

Ans: We know that, area of | |gram PQRS. In which

${\text{PQ}} = {\text{SR}} = 3,{\text{SQ}} = 4$

And $\angle S = \angle Q = {90^\circ }$

$\angle PQS = \angle QSR = {90^\circ }$

${\text{PQ}}||{\text{SR}}$

${\text{PQ}} = {\text{SR}} = 3$

${\text{ABCD}}$ is a || gram

Area of parallelogram

\[ = \]Base $ \times $ corresponding Altitude

$ = 3 \times 4$

$ = 12$ square units

14. In a parallelogram PQRS. The Altitude corresponding to sides ${\text{PQ}}$ and ${\text{PS}}$ are respectively. $7\;{\text{cm}}$ and $8\;{\text{cm}}$ find ${\text{PS}}$, if ${\text{PQ}} = 10\;{\text{cm}}$.

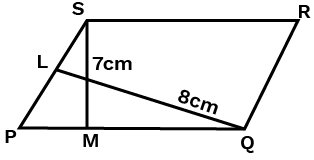
Ans: Area of | | gram PQRS

$ = {\text{PQ}} \times {\text{SM}}$

$ = 10 \times 7$

$ = 70$ square ${\text{cm}}$…….(i)

Area of Parallelogram PQRS

$ = {\text{PS}} \times {\text{QL}}$

$ = ({\text{AD}} \times 8)$ square ${\text{cm}}$

From (i) and (ii)

${\text{PS}} \times 8 = 70$

${\text{PS}} = \dfrac{{70}}{8}$

$ = 8.75\;{\text{cm}}$

15. Area, base and corresponding altitude are ${x^2},x - 3$ and $x + 4$ respectively. Find the

area of parallelogram.

Ans: Area of parallelogram

$ = {\text{ Base }} \times {\text{ Corresponding Altitude}}$

${x^2} = (x - 3)(x + 4)$

${x^2} = {x^2} + 4x - 3x - 12$

$x = 12$

$ = (12 - 3)(12 - 4)$

$ = (9)(16)$

$ = 144$ square units.

16. Find the altitude corresponding to side ${\text{EF}}$ if area of $\vartriangle ABC = \Delta DEF$. If $\vartriangle ABC{\text{AB}}$ $ = 8\;{\text{cm}}$ and altitude corresponding to ${\text{AB}}$ is $5\;{\text{cm}}$. In $\Delta DEF,EF = 10\;{\text{cm}}$

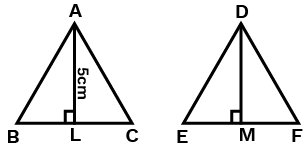
Ans: $\operatorname{ar} (\Delta ABC) = \operatorname{ar} (\Delta DEF)$

$\dfrac{1}{2} \times AB \times CM = \dfrac{1}{2} \times EF \times DN$

$\dfrac{1}{2} \times 8 \times 5 = \dfrac{1}{2} \times 10 \times DN$

$20 = 5DN$

$DN = 4\;{\text{cm}}$

Altitude corresponding to side ${\text{EF}}$ is $4\;{\text{cm}}$

17. Prove that the area of a trapezium is half of the product of its height and the sum of the parallel sides.

Ans: Join B and D. Draw BL $ \bot $ DC (Produced)

$\operatorname{ar} (ABCD) = \operatorname{ar} (\Delta ABD) + \operatorname{ar} (\Delta DCB)$

$ = \left( {\dfrac{1}{2}AB \times DK} \right) + \left( {\dfrac{1}{2}DC \times BL} \right)$

$ = \left( {\dfrac{1}{2}AB \times DK} \right) + \left( {\dfrac{1}{2}DC \times DK} \right)$

$ = \dfrac{1}{2}DK(AB + CD)$

18. Show that the area of a rhombus is half the product of the length of its diagonals.

Ans: $\operatorname{ar} (\Delta ABC) = \dfrac{1}{2} \times AC \times OB \ldots  \ldots .(i)$ 

$\operatorname{ar} (\Delta ACD) = \dfrac{1}{2} \times AC \times DO \ldots  \ldots ...(ii)$

Adding (i) and (ii)

$\operatorname{ar} (\Delta ABC + \Delta ACD)\dfrac{1}{2} \times AC \times (DO + OB)$

$ = \dfrac{1}{2} \times AC \times BD$

Hence, area of rhombus ${\text{ABCD}} = \dfrac{1}{2} \times AC \times BD$

19. In parallelogram P is any point inside it. Prove that $\operatorname{ar} (\Delta ABP) + \operatorname{ar} (\Delta DCP) = \dfrac{1}{2}\operatorname{ar} (\Delta ABCD)$

Ans: $\operatorname{ar} (\Delta ABP) = \dfrac{1}{2}AB \times PK$

$\operatorname{ar} (\Delta DCP) = \dfrac{1}{2}CD \times PM$

$ = \dfrac{1}{2}AB \times PM$

$\operatorname{ar} (\Delta ABP) + \operatorname{ar} (\Delta DCP) = \dfrac{1}{2}AB \times PK + \dfrac{1}{2}AB \times PM$

$ = \dfrac{1}{2}AB(PK + PM)$

$ = \dfrac{1}{2}AB \times MK$

20. Show that If $X$ is any point on side BR of PQRS and ABRS.

(i) $\operatorname{ar} ({\mathbf{PQRS}}) = \operatorname{ar} ({\text{ABRS}})$

Ans: | |gram PQRS and ABRS are on the same base SR and Between the same parallel PB and SR,

So, $\operatorname{ar} (PQRS) = \operatorname{ar} (ABRS)$

(ii) $\operatorname{ar} ({\text{AXS}}) = \dfrac{1}{2}\operatorname{ar} ({\text{PQRS}})$

Ans:  $\operatorname{ar} (AXS) = \dfrac{1}{2}\operatorname{ar} (ABRS)$

$\operatorname{ar} (ABRS) = \operatorname{ar} (PQRS)$

$\operatorname{ar} (AXS) = \dfrac{1}{2}\operatorname{ar} (PQRS)$

21. Show that the diagonals of a parallelogram divide if into four triangles of equal area.

Ans: Given: A parallelogram ${\text{ABCD}}$ and ${\text{AC}}$ and ${\text{BC}}$ are diagonals

To prove: $\operatorname{ar} ({\text{ABO}}) = \operatorname{ar} ({\text{COD}}) = \operatorname{ar} ({\text{BCO}}) = \operatorname{ar} ({\text{AOD}})$

Proof: $\operatorname{ar} ({\text{ADB}}) = \operatorname{ar} ({\text{ACB}})$

$ \Rightarrow \operatorname{ar} (ADB) - \operatorname{ar} (ABO) = \operatorname{ar} (ACB) - \operatorname{ar} (ABO)$

$ \Rightarrow \operatorname{sr} (ADO) = \operatorname{ar} (BCO) \ldots  \ldots .(i)$

$\operatorname{Ar} ({\text{ADC}}) = \operatorname{ar} ({\text{BCD}})$

$ \Rightarrow \operatorname{ar} (ADC) - \operatorname{ar} (CDO) = \operatorname{ar} (BCD) - \operatorname{ar} (CDO)$

$ \Rightarrow \operatorname{ar} (ADO) = \operatorname{ar} (AOB) \ldots  \ldots  \ldots .(ii)$

In triangle ${\text{ABC}}$, BO is median

$\therefore \operatorname{ar} (ABO) = \operatorname{ar} (BCO)$

In triangle ADC, OD is median

\[\therefore \operatorname{ar} (ADO) = \operatorname{ar} (CDO)\]

From (i), (ii), (iii) and (iv)

$\operatorname{Ar} ({\text{ABO}}) = \operatorname{ar} ({\text{CDO}}) = \operatorname{ar} ({\text{BCO}}) = \operatorname{ar} ({\text{ADO}})$

Hence proved.

22. Show that PQ divides the ||gram in two Parts of the equal area if diagonal of ||gram ABCD intersect Point \[0.\]through Point 0, a line is drawn to intersect AD at $P$ and \[BC\] at Q.

Ans: To prove: Are (quadrilateral APQB) $ = {\text{ }}ar\left( {{\text{quadrilateral PQCD}}} \right) = \dfrac{1}{2}\;\left( {ar{\text{ }}\left| {\text{ }} \right|{\text{ }}gram{\text{ }}ABCD} \right)$Proof: $\angle AOP = \angle COQ$ (Vertically opposite angles)

$OA = OC$

$\angle OAP = \angle OCQ$

$\therefore \vartriangle AOP \cong \vartriangle DOQ$

$ \Rightarrow \operatorname{ar} (\Delta AOP) = \operatorname{ar} (\Delta COQ).$

$ar(\Delta ABC) = ar(\Delta ACD)(\because $ Two triangles on the base and between same par allels $)$

$ \Rightarrow $ $ar{\text{ }}(quad.{\text{ABQO}}) + \operatorname{ar} (\Delta COQ) = ar({\text{quadrilateralOCDP}}) + ar(\Delta {\text{AOP}})$ 

$ \Rightarrow \operatorname{ar} (quad.{\text{APQB}}) = \operatorname{ar} (quad.\operatorname{PQCD} )$  

23. Show that $(\Delta ABE) = $ are of $(\Delta ACE)$ if ${\mathbf{E}}$ is any Point on its median AD.

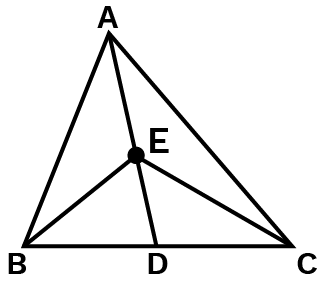
Ans: Join ${\text{BE}}$ and CE

$\operatorname{ar} (\Delta ABD) = \operatorname{ar} (\Delta ACD) \ldots  \ldots  \ldots ...({\text{i}})$

$\operatorname{ar} (\Delta EBD) = \operatorname{ar} (\Delta ECD) \ldots  \ldots  \ldots ..(ii)$

${\text{Subtracting}}{\kern 1pt} {\kern 1pt} {\text{(ii)}}{\kern 1pt} {\kern 1pt} {\text{from}}{\kern 1pt} {\kern 1pt} {\text{(}}{\kern 1pt} {\text{i)}}$

$\operatorname{ar} (\Delta ABD) - \operatorname{ar} (\Delta EBD) = \operatorname{ar} (\Delta ACD) - \operatorname{ar} (AECD)$

$ \Rightarrow \operatorname{ar} (\Delta ABE) = \operatorname{ar} (\Delta ACE)$

24. The triangle PQR and PSR are equal in area, if PR and QS bisect at O.

Ans: \[PO\]is median of $\vartriangle PQS$

$\therefore \operatorname{ar} (\Delta {\text{POQ}}) = \operatorname{ar} (\Delta {\text{POS}})$

${\text{RO}}$ is median of $\Delta {\text{QRS}}$

$\therefore \operatorname{ar} (\Delta QOR) = \operatorname{ar} (\Delta ROS)..(ii)$

${\text{Adding}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{(i)}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{and}}{\kern 1pt} \,{\text{(ii}})$

$\operatorname{ar} (\Delta POQ) + \operatorname{ar} (\Delta {\text{QOR}}) = \operatorname{ar} (\Delta POS) + \operatorname{ar} (\Delta ROS)$

$ \Rightarrow \operatorname{ar} (\Delta PQR) = \operatorname{ar} (\Delta PSR)$

25. Show that ${\mathbf{ar}}(\Delta ABG) = \dfrac{1}{3}\operatorname{ar} (\Delta ABC)$, if median of $\Delta $ intersect at ${\text{G}}$.

Ans: Given,

AM, BN and CL are medians.

To prove:

$\operatorname{ar}(\Delta \mathrm{AGB})=\operatorname{ar}(\Delta \mathrm{AGC})=\operatorname{ar}(\Delta \mathrm{BGC})$

$=\dfrac{1}{3} \cdot \operatorname{ar}(\Delta \mathrm{ABC})$

Proof:

To $\Delta \mathrm{AGB} \& \Delta \mathrm{AGC}$

AG is the median

$\therefore$ ar. $\Delta \mathrm{AGB}=\operatorname{ar} \cdot \Delta \mathrm{AGC}$

Similarly

BG is the median

$\therefore$ ar. $\Delta \mathrm{AGB}=\operatorname{ar} \cdot \Delta \mathrm{BGC}$

So we can say that

ar. $\Delta \mathrm{AGB}=\operatorname{ar} \cdot \Delta \mathrm{AGC}=\operatorname{ar} \cdot \Delta \mathrm{BGC}$

Now,

$\Delta \mathrm{AGB}+\Delta \mathrm{AGC}+\Delta \mathrm{BGC}=\mathrm{ar} \cdot \Delta \mathrm{ABC}$

$\dfrac{1}{3} \cdot \Delta \mathrm{AGB}+\dfrac{1}{3} \Delta \mathrm{AGC}+\dfrac{1}{3} \Delta \mathrm{BGC}=\operatorname{ar} . \Delta \mathrm{ABC}$

(they are in equal area)

$\Rightarrow \dfrac{1}{3}(\Delta \mathrm{AGB}+\Delta \mathrm{AGC}+\Delta \mathrm{BGC})=\operatorname{ar} . \Delta \mathrm{ABC}$

$\Rightarrow \Delta \mathrm{AGB}+\Delta \mathrm{AGC}+\Delta \mathrm{BGC}=\dfrac{1}{3} \cdot \operatorname{ar} \Delta \mathrm{ABC}$

Hence proved.

26. Show that $\operatorname{ar} ({\text{ABCD}}) = \;ar\left( {BQRP} \right),$ AQ is drawn Parallel to CP to intersect ${\text{CB}}$ produced to $Q$ and parallelogram BQRP is completed if ${\mathbf{P}}$ is any Point on AB produced.

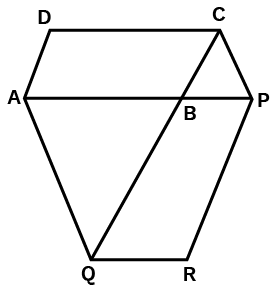
Ans: Let us join AC and P Q.

$\triangle \mathrm{ACQ}$ and $\triangle \mathrm{AQP}$ are on the same base $\mathrm{AQ}$ and between the same parallels $\mathrm{AQ}$ and $\mathrm{CP}$.

$\therefore$ Area $(\triangle \mathrm{ACQ})=$ Area

$(\triangle \mathrm{APQ})$

$\Rightarrow \text { Area }(\triangle \mathrm{ACQ})-\text { Area }(\triangle \mathrm{ABQ})=\operatorname{Area}(\triangle \mathrm{ACQ})-\operatorname{Area}(\triangle \mathrm{ABQ})$

$\Rightarrow \text { Area }(\triangle \mathrm{ABC})=\text { Area }(\triangle \mathrm{ABQ}) \ldots(1)$

Since $\mathrm{AC}$ and $\mathrm{P} \mathrm{Q}$ are diagonals of parallelograms $\mathrm{ABCD}$ and $\mathrm{PBQR}$ respectively,

$\therefore$ Area $(\triangle \mathrm{ABC})=\dfrac{1}{2}$ Area of parallelogram ABCD ... (2)

$\operatorname{Area}(\triangle \mathrm{QBP})=\dfrac{1}{2}$ Area of parallelogram BP RQ ... (3)

From equations $(1),(2)$, and (3), we obtain

$\dfrac{1}{2}$ Area of parallelogram $\mathrm{ABCD}=\dfrac{1}{2}$ Area of parallelogram $\mathrm{BP} \mathrm{RQ}$

$\therefore$ Area of parallelogram $\mathrm{ABCD}=$ Area of parallelogram BP RQ

27. Show that area of $\Delta BPQ = \dfrac{1}{2}$ area of $\vartriangle ABC.{\mathbf{D}}$ is mid-point of ${\text{AB}},{\mathbf{P}}$ is any point on BC. PQ is joined and line CQ is drawn parallel to PD to intersect ${\text{AB}}$ at ${\text{Q}}$.

Ans: Given: $\triangle A B C$ in which $D$ is the mid point of AB.PD Il CQ

Construction: Join $C$ to $D$ and $Q$ to $P$.

Proof: $C D$ is a median of the $\triangle A B C$

$\therefore$ ar $(B C D)=\operatorname{ar}(D A C)$

$\triangle P D C$ and $\triangle P D Q$ are on the same base PD and between the same parallels PD and CQ.

$\therefore$ ar $(P D C)=$ ar $(P D Q)$

ar $(B C D)=\dfrac{1}{2}$ ar $(A B C) \quad$ (median divides a triangle into two triangles of equal area)

$\operatorname{ar}(B P D)+\operatorname{ar}(P D C)=\dfrac{1}{2} \operatorname{ar}(A B C)$

$\operatorname{ar}(B P D)+\operatorname{ar}(P D Q)=\dfrac{1}{2} \operatorname{ar}(A B C)$

Hence ar $(B P Q)=\dfrac{1}{2}$ ar $(A B C)$

28. ${\mathbf{E}}$ is the mid-point of median AD, show that $ar(BED) = \dfrac{1}{4}\operatorname{ar} (ABC)$.

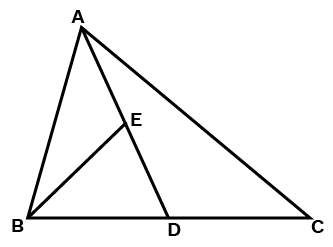
Ans: $\text { Since } A D \text { is the median of } \triangle A B C \text {, so it will divide } \triangle A B C \text { into two equal }$

$\text { triangles. }$

$\therefore \text { ar }(\triangle A B D)=\operatorname{ar}(\triangle A D C)$

$\text { Also, ar }(\triangle A B D)=1 / 2 \operatorname{ar}(A B C) \quad \ldots . \text { (i) }$

$\text { Now, In } \triangle A B D, B E \text { is the median, }$

$\text { Therefore, BE will divide } \triangle A B D \text { into two equal triangles }$

$\operatorname{ar}(\Delta B E D)=\operatorname{ar}(\Delta B A E) \text { and ar }(\triangle B E D)=1 / 2 \operatorname{ar}(\triangle A B D)$

$\operatorname{ar}(\Delta B E D)=1 / 2 \times[1 / 2 \operatorname{ar}(A B C)](\text { Using equation }(i))$

$\therefore \text { ar }(\triangle B E D)=1 / 4 \operatorname{ar}(\triangle A B C)$

29. Show that the line segments joining the mid-points of parallel sides of a trapezium divides it into two parts of equal area.

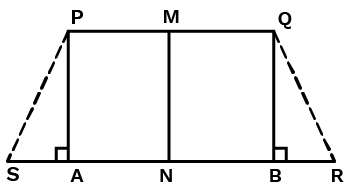
Ans: Given: PQRS is a trapezium and $\mathrm{M}, \mathrm{N}$ are the midpoints on $\mathrm{PQ}$ an SR respectively.

To prove: Line segment joining the midpoint of the parallel sides of a trapezium divides it in two equal parts.

Construction: PA and PB are heights of the trapezium.

Proof: Let us assume that PQRS is an isosceles triangle with $\mathrm{PS}=\mathrm{QR}$.

Now, PM=MQ, SN=NR

and $A N=P M, M Q=N B($ opposite angles of the rectangle)

Therefore, $\operatorname{ar}($ PMNA $)=\operatorname{ar}(\mathrm{MQBN})$

Now,in $\triangle \mathrm{PSA}$ and $\triangle \mathrm{QRB}$,

$\mathrm{PA}=\mathrm{QB}($ Heights of trapezium)

PS=QR (assumption)

$\angle \mathrm{PAS}=\angle \mathrm{QBR}\left(\right.$ each $\left.90^{\circ}\right)$

By RHS rule,

$\triangle \mathrm{PSA} \cong \Delta \mathrm{QRB}$

$\Rightarrow \operatorname{ar}(\mathrm{PAS})=\operatorname{ar}(\mathrm{QBR})$

Also, ar(PMNS)=ar(PAS)+ar(PMNA) and $\operatorname{ar}(\mathrm{MQRN})=\operatorname{ar}(\mathrm{QBR})+\operatorname{ar}(\mathrm{MQBN})$

$\Rightarrow \operatorname{ar}(\mathrm{PMNS})=\operatorname{ar}(\mathrm{MQRN})$

Hence proved.

30. Prove that $\operatorname{ar} (ADX) = \operatorname{ar} (ACY).$ if ${\mathbf{AB}}||{\mathbf{DC}}$ and line parallel to AC intersects ${\text{AB}}$ at $X$ and ${\text{BC}}$ at ${\mathbf{Y}}$

Ans: 

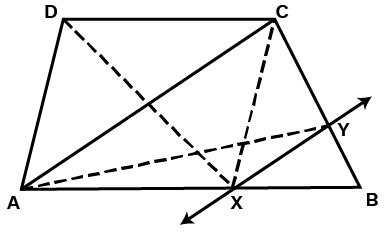
We observe that $\triangle \mathrm{ADX}$ and $\triangle \mathrm{ACX}$ are lying on the same base $\mathrm{AX}$ and are existing between the same parallels $A B$ and DC.

As we know that two triangles on the same base (or equal bases) and between the same parallels are equal in area.

Therefore, ar $(\triangle A D X)=\operatorname{ar}(\triangle A C X) \ldots$ (1)

Similarly, $\triangle \mathrm{ACY}$ and $\triangle \mathrm{ACX}$ are lying on the same base $\mathrm{AC}$ and are existing between the same parallels $A C$ and $X Y$.

Therefore, Area $(\Delta A C Y)=$ Area $(\Delta A C X) \ldots$ (2)

From Equations (1) and (2), we obtain

Area $(\triangle A D X)=$ Area $(\Delta A C Y)$

Hence proved.

31. Prove that area of $\Delta GBC = $ area of quadrilateral AFGE if BE and CF medians intersect at G.

Ans: $\mathrm{BE}$ is the median of $\Delta \mathrm{ABC}$

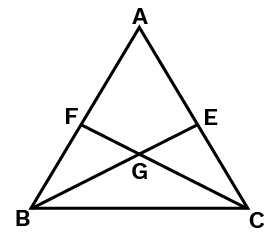
$\text { so, } \operatorname{ar}(\Delta \mathrm{BEC})=\dfrac{1}{2} \operatorname{ar}(\Delta \mathrm{ABC})$

Median of triangle divides into 2 triangles of equal area. Also CF is median of $\Delta \mathrm{ABC}$

$\Rightarrow \operatorname{ar}(\Delta \mathrm{ACF})=\dfrac{1}{2} \operatorname{ar}(\Delta \mathrm{ABC})$

from (1) and (2)

$\operatorname{ar}(\Delta \mathrm{ACF})=\operatorname{ar}(\Delta \mathrm{BEC})$

$\operatorname{ar}(\Delta \mathrm{GBC})+\operatorname{ar}(\Delta \mathrm{GEC})=\operatorname{ar}(\mathrm{AFGE})+\operatorname{ar}(\mathrm{GEC})$

$\therefore \operatorname{ar}(\Delta \mathrm{GBC})=\operatorname{ar}(\mathrm{AF} \mathrm{GE})$

32. Show that $\operatorname{ar} \Delta AED \times $ area $\Delta BEC = $ area $\Delta ABE \times $ area $\Delta CDE$ if diagonals of quadrilateral AC and BD intersect at a Point ${\text{E}}$. 

Ans: Draw $AM \bot BD$ and also $CN \bot BD$

$\operatorname{ar} (\Delta AED) \times \operatorname{ar} (\Delta BEC) = \left( {\dfrac{1}{2}ED \times AM} \right) \times \left( {\dfrac{1}{2}BE \times CN} \right)$

$ = \dfrac{1}{4}ED \times AM \times BE \times CN$

$ = \left( {\dfrac{1}{2}BE \times AM} \right) \times \left( {\dfrac{1}{2}ED \times CN} \right)$

$ = \operatorname{ar} (\Delta ABE) \times \operatorname{ar} (\Delta CDE)$

$\operatorname{ar} (\Delta AED) \times \operatorname{ar} (\Delta BEC) = \operatorname{ar} (\Delta ABE) \times \operatorname{ar} (\Delta CDE)$

4 Marks Questions 

1. Diagonals AC and BD of a quadrilateral ABCD intersect each other at P. Show that: ar $({\mathbf{APB}}) \times \operatorname{ar} ({\mathbf{CPD}}) = \operatorname{ar} ({\mathbf{APD}}) \times {\mathbf{ar}}({\text{BPC}})$

Ans: Given: A quadrilateral ABCD, in which diagonals

AC and BD intersect each other at point $E$.

To Prove: $ar(AED) \times ar(BEC)$

$ = \operatorname{cor} (ABE) \times \operatorname{ar} (CDE)$

Construction: From A, draw AM $ \bot {\text{BD}}$ and from ${\text{C}}$, draw ${\text{CN}} \bot {\text{BD}}$. 

Proof: $\operatorname{ar} (\Delta {\text{ABE}}) = \dfrac{1}{2} \times BE \times AM.........(i)$

And $ar(\Delta {\text{AED}}) = \dfrac{1}{2} \times DE \times AM.......(ii)$ 

Dividing eq. (ii) by (i), we get,

$\dfrac{{\operatorname{ar} (\Delta {\text{AED}})}}{{\operatorname{ar} (\Delta {\text{ABE}})}} = \dfrac{{\dfrac{1}{2} \times {\text{DE}} \times {\text{AM}}}}{{\dfrac{1}{2} \times {\text{BE}} \times {\text{AM}}}}$

$ \Rightarrow \dfrac{{\operatorname{ar} (\Delta {\text{AED}})}}{{\operatorname{ar} (\Delta {\text{ABE}})}} = \dfrac{{{\text{DE}}}}{{{\text{BE}}}}.......(iii)$

Similarly, $\dfrac{{\operatorname{ar} (\Delta {\text{CDE}})}}{{\operatorname{ar} (\Delta {\text{BEC}})}} = \dfrac{{{\text{DE}}}}{{{\text{BE}}}}........(iv)$

From eq. (iii) and (iv), we get

$\dfrac{{\operatorname{ar} (\Delta {\text{AED}})}}{{\operatorname{ar} (\Delta {\text{ABE}})}} = \dfrac{{\operatorname{ar} (\Delta {\text{CDE}})}}{{\operatorname{ar} (\Delta {\text{BEC}})}}$

$ar(AED) \times \operatorname{Car} (BEC)$

$ = ar(ABE) \times \operatorname{CO} (CDE)$

2. P and $Q$ are respectively the mid-points of sides \[AB\]and \[BC\]or a triangle \[ABC\]and $R$ is the mid-point of AP, show that:

(i) $\operatorname{ar} ({\mathbf{PRQ}}) = \dfrac{1}{2}\operatorname{ar} ({\text{ARC}})$

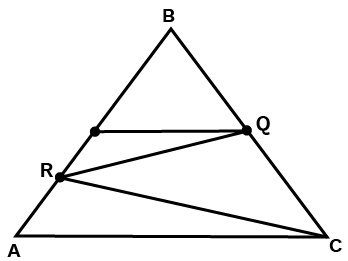
Ans:  ${\text{PC}}$ is the median of $\Delta {\text{ABC}}$. $\therefore \operatorname{ar} (\Delta {\text{BPC}}) = \operatorname{ar} (\Delta {\text{APC}})........(i)$

RC is the median of $\Delta {\text{APC}}$. 

$\therefore $ $\;ar(\Delta {\text{ARC}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{APC}}) \ldots  \ldots  \ldots .$ (ii)

${\text{PQ}}$ is the median of $\vartriangle {\text{BPC}}$.

$\therefore ar({\text{\Delta PQC}}) = \dfrac{1}{2}ar({\text{\Delta BPC}}) \ldots  \ldots  \ldots {\text{\;(iii)\;}}$ 

From eq. (i) and (iii), we get,

$\operatorname{ar} (\Delta {\text{PQC}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{APC}})........(iv)$

From eq. (ii) and (iv), we get,

$\operatorname{ar} (\Delta {\text{PQC}}) = \operatorname{ar} (\Delta {\text{ARC}}) \ldots  \ldots  \ldots ..({\text{v}})$

We are given that ${\text{P}}$ and ${\text{Q}}$ are the mid-points of ${\text{AB}}$ and ${\text{BC}}$ respectively.

 and ${\text{PA}} = \dfrac{1}{2}{\text{AC}}$

$ \Rightarrow \operatorname{ar} (\Delta {\text{APQ}}) = \operatorname{ar} (\Delta {\text{PQC}}) \ldots  \ldots  \ldots $ (vi) 

From eq. (v) and (vi), we get

$\operatorname{ar} (\Delta {\text{APQ}}) = \operatorname{ar} (\Delta {\text{ARC}}) \ldots  \ldots  \ldots .$ (vii)

${\text{R}}$ is the mid-point of AP. 

Therefore, RQ is the median of $\vartriangle {\text{APQ}}.$

$\therefore \operatorname{ar} (\Delta {\text{PRQ}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{APQ}}) \ldots  \ldots  \ldots $ (viii)

From (vii) and (viii), we get,

$\operatorname{ar} (\Delta {\text{PRQ}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ARC}})$

(ii) $\operatorname{ar} ({\text{RQC}}) = \dfrac{3}{8}\operatorname{ar} ({\text{ABC}})$

Ans: ${\text{PQ}}$ is the median of $\Delta {\text{BPC}}$

$\therefore \operatorname{ar} (\Delta {\text{PQC}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{BPC}}) = \dfrac{1}{2} \times \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}}) = \dfrac{1}{4}\operatorname{ar} (\Delta {\text{ABC}}) \ldots  \ldots  \ldots .{\text{ (ix) }}$

Also, $ar(\Delta {\text{PRC}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{APC}}){\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{[Using(iv)]}}$

$ \Rightarrow \operatorname{ar} (\Delta {\text{PRC}}) = \dfrac{1}{2} \times \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}}) = \dfrac{1}{4}\operatorname{ar} (\Delta {\text{ABC}}) \ldots  \ldots  \ldots ..({\text{x}})$

${\text{Adding}}{\kern 1pt} {\kern 1pt} \,{\kern 1pt} {\kern 1pt} {\text{eq}}{\text{.(ix)}}{\kern 1pt} \,{\kern 1pt} {\text{and}}{\kern 1pt} \,{\text{(x)}}$, we get,

$\operatorname{ar} (\Delta {\text{PQC}}) + \operatorname{ar} (\Delta {\text{PRC}}) = \left( {\dfrac{1}{4} + \dfrac{1}{4}} \right)\operatorname{ar} (\Delta {\text{ABC}})$

$ \Rightarrow $ $ar{\text{ }}(quad.{\text{PQCR}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}}) \ldots  \ldots  \ldots .({\text{xi}})$

Subtracting $ar{\text{ }}(\Delta {\text{PRQ}})$ from the both sides,

 $ar{\text{ }}(quad{\text{PQCR}}) - \operatorname{ar} (\Delta {\text{PRQ}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}}) - \operatorname{ar} (\Delta {\text{PRQ}})$

$\operatorname{ar} (\Delta {\text{RQC}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}}) - \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ARC}})[{\text{Using}}$ result (i)]

$\operatorname{ar} (\Delta {\text{ARC}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}}) - \dfrac{1}{2} \times \dfrac{1}{2}\operatorname{ar} (\Delta {\text{APC}})$

$\operatorname{ar} (\Delta {\text{RQC}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}}) - \dfrac{1}{4}\operatorname{ar} (\Delta {\text{APC}})$

$\operatorname{ar} (\Delta {\text{RQC}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}}) - \dfrac{1}{4} \times \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}})[{\text{PC}}$ is median of $\Delta {\text{ABC}}]$

$\operatorname{ar} (\Delta {\text{RQC}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}}) - \dfrac{1}{8}\operatorname{ar} (\Delta {\text{ABC}})$

$\operatorname{ar} (\Delta {\text{RQC}}) = \left( {\dfrac{1}{2} - \dfrac{1}{8}} \right){\text{x}}$ $ar(\Delta {\text{ABC}})$

$\operatorname{ar} (\Delta {\text{RQC}}) = \dfrac{3}{8}\operatorname{ar} (\Delta {\text{ABC}})$

(iii) $\operatorname{ar} ({\text{PBQ}}) = \operatorname{ar} ({\text{ARC}})$

Ans: $\operatorname{ar} (\Delta {\text{PRQ}}) = \dfrac{1}{2}{\text{ ar }}(\Delta {\text{ARC}})[{\text{Usingresult}}({\text{i}})]$

$ \Rightarrow 2\operatorname{ar} (\Delta {\text{PRQ}}) = \operatorname{ar} (\Delta {\text{ARC}})..({\text{xii}})$

$\operatorname{ar} (\Delta {\text{PRQ}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{APQ}})[{\text{RQ}}$ is the median of $\Delta {\text{APQ}}] \ldots  \ldots  \ldots ..$ (xiii)

But $ar(\Delta {\text{APQ}}) = \operatorname{ar} (\Delta {\text{PQC}})$ [Using reason of eq. (vi) $] \ldots  \ldots  \ldots $ (xiv)

From eq. (xiii) and (xiv), we get,

$\operatorname{ar} (\Delta {\text{PRQ}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{PQC}}) \ldots  \ldots  \ldots ({\text{xv}})$

But $ar(\Delta {\text{BPQ}}) = \operatorname{ar} (\Delta {\text{PQC}})[{\text{PQ}}$ is the median of $\Delta {\text{BPC}}] \ldots  \ldots  \ldots ..({\text{xvi}})$

From eq. (xv) and (xvi), we get,

$\operatorname{ar} (\Delta {\text{PRQ}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{BPQ}}) \ldots  \ldots  \ldots ..{\text{ (xvii) }}$

${\text{Now}}{\kern 1pt} \,{\kern 1pt} {\kern 1pt} {\text{from}}{\kern 1pt} \,{\kern 1pt} {\text{(xii)}}\,{\kern 1pt} \,{\text{and}}{\kern 1pt} {\kern 1pt} \,{\text{(xvii),we}}{\kern 1pt} {\kern 1pt} {\text{get,}}$

$2\left( {\dfrac{1}{2}{\text{ ar }}(\Delta {\text{BPQ}})} \right) = \operatorname{ar} (\Delta {\text{ARC}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{BPQ}}) = \operatorname{ar} (\Delta {\text{ARC}})$

3. In figure, \[ABC\]is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment AX $ \bot $ DE meets BC at Y. Show that: 

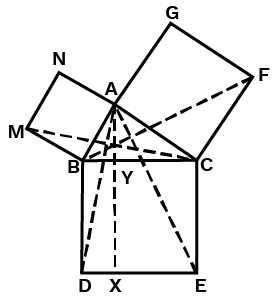
(i) $\Delta {\text{MBC}} \cong \Delta {\text{ABD}}$

Ans: $\angle {\text{ABM}} = \angle {\text{CBD}} = {90^\circ }$

Adding $\angle {\text{ABC}}$ both sides, we get,

$\angle {\text{ABM}} + \angle {\text{ABC}} = \angle {\text{CBD}} + \angle {\text{ABC}}$

$ \Rightarrow \angle {\text{MBC}} = {\text{ABD}}$

Now in $\Delta {\text{MBC}}$ and $\vartriangle {\text{ABD}}$,

${\text{MB}} = {\text{AB}}$ (equal sides of square ABMN)

${\text{BC}} = {\text{BD}}$ [sides of square ${\text{BCED}}]$

$\angle {\text{MBC}} = \angle {\text{ABD}}$ (proved above)

$\therefore \Delta {\text{MBC}} \cong \Delta {\text{ABD}}$ (By SAS congruency)

(ii) $\operatorname{ar} ({\text{BYXD}}) = 2\operatorname{ar} ({\text{MBC}})$

Ans: From above, $\Delta {\text{MBC}} \cong \Delta {\text{ABD}}$

$ \Rightarrow \operatorname{ar} (\Delta {\text{MBC}}) = \operatorname{ar} (\Delta {\text{ABD}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{MBC}}) = \operatorname{ar} ({\text{ trap}}{\text{. ABDX}}) - \operatorname{ar} (\Delta {\text{ADX}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{MBC}}) = \dfrac{1}{2}({\text{BD}} + {\text{AX}}){\text{BY}} - \dfrac{1}{2}{\text{DX}} \cdot {\text{AX}}$

$ \Rightarrow \operatorname{ar} (\Delta {\text{MBC}}) = \dfrac{1}{2}{\text{BD}} \cdot {\text{BY}} + \dfrac{1}{2}{\text{AX}} \cdot {\text{BY}} - \dfrac{1}{2}{\text{DX}}.{\text{AX}}$

$ \Rightarrow $ ${\mathbf{ar}}(\Delta {\text{MBC}}) = \dfrac{1}{2}{\text{BD}} \cdot {\text{BY}} + \dfrac{1}{2}{\text{AX}}({\text{BY}} - {\text{DX}})$

$ \Rightarrow $ ${\mathbf{ar}}(\Delta {\text{MBC}}) = \dfrac{1}{2}{\text{BD}} \cdot {\text{BY}} + \dfrac{1}{2}{\text{AX}}.0[{\text{BY}} = {\text{DX}}]$

$ \Rightarrow $ ${\mathbf{ar}}(\Delta {\text{MBC}}) = \dfrac{1}{2}{\text{BD}}.{\text{BY}}$

$ \Rightarrow 2\operatorname{ar} (\Delta {\text{MBC}}) = {\text{BD}} \cdot {\text{BY}} \Rightarrow 2\operatorname{ar} (\Delta {\text{MBC}}) = {\mathbf{ar}}\left( {{\mathbf{rect}}.{\text{ }}{\mathbf{BYXD}}} \right)$ 

Hence $ar({\text{BYXD}}) = 2{\text{ar}}(\Delta {\text{MBC}})$

(iii) $\operatorname{ar} ({\text{BYXD}}) = \operatorname{ar} ({\text{ABMN}})$

Ans: Join AM. ABMN is a square.

Therefore, NA 

Now $\Delta {\text{AMB}}$ and $\Delta {\text{MBC}}$ are on the same base and between the same parallels ${\text{MB}}$ and ${\text{AC}}$. $\therefore \operatorname{ar} (\Delta {\text{AMB}}) = \operatorname{ar} (\Delta {\text{MBC}}) \ldots  \ldots  \ldots .$ (ii)

From result (ii), we have $ar{\text{ }}\left( {BYXD} \right){\text{ }} = {\text{ }}2{\text{ }}ar(\Delta {\text{MBC}}) \ldots  \ldots  \ldots $ (iii)

Using eq. (ii) and (iii), we get, \[ar\left( {BYXD} \right) = 2{\text{ }}ar(\Delta {\text{AMB)}}\] 

$ \Rightarrow \operatorname{ar} ({\text{BYXD}}) = \operatorname{ar} (square{\text{ABMN}})$ 

(Diagonal AM of square ABMN divides it in two triangles of equal area)

(iv) $\Delta {\mathbf{FCB}} \cong \Delta {\text{ACE}}$

Ans:  In $\Delta {\text{FCB}}$ and $\Delta {\text{ACE}}$, ${\text{FC}} = {\text{AC}}$ (sides of square ACFG)

${\text{BC}} = {\text{CE}}$ (sides of square BCED)

$\angle {\text{BCF}} = \angle {\text{ACE}}$

Adding $\angle $ ACB both sides,

$\angle {\text{BCF}} + \angle {\text{ACB}} = \angle {\text{ACE}} + \angle {\text{ACB}} \Rightarrow \angle {\text{BCF}} = \angle {\text{ACE}}$

$\therefore \Delta {\text{FCB}} \cong \Delta $ ACE (By SAS congruency)

(v) $\operatorname{ar} ({\text{CYXE}}) = 2{\text{ar}}({\text{FCB}})$

Ans: From (iv), we have, $\Delta {\text{FCB}} \cong \Delta {\text{ACE}}$

$ \Rightarrow \operatorname{ar} (\Delta {\text{FCB}}) = \operatorname{ar} (\Delta {\text{ACE}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{FCB}}) = \operatorname{ar} ({\text{ trap}}{\text{. ACEX}}) - \operatorname{ar} (\Delta {\text{AEX}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{FCB}}) = \dfrac{1}{2}({\text{CE}} + {\text{AX}}){\text{CY}} - \dfrac{1}{2}{\text{XE}} \cdot {\text{AX}}$

$ \Rightarrow \operatorname{ar} (\Delta {\text{FCB}}) = \dfrac{1}{2}{\text{CE}} \cdot {\text{CY}} + \dfrac{1}{2}{\text{AX}}({\text{CY}} - {\text{XE}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{FCB}}) = \dfrac{1}{2}{\text{CE}}.{\text{CY}} + \dfrac{1}{2}{\text{AX}}.0[{\text{CY}} = {\text{XE}}]$

$ \Rightarrow \operatorname{ar} (\Delta {\text{FCB}}) = \dfrac{1}{2}{\text{CE}}.{\text{CY}}$

$ \Rightarrow 2\operatorname{ar} (\Delta {\text{FCB}}) = {\text{CE}}.{\text{CY}} \Rightarrow 2\operatorname{ar} (\Delta {\text{FCB}}) = \operatorname{ar} ({\mathbf{rect}}.{\text{CYXE}})$ 

Hence $ar({\text{BYXD}}) = 2\operatorname{ar} (\Delta {\text{FCB}})$

(vi) $\operatorname{ar} ({\text{CYXE}}) = \operatorname{ar} ({\text{ACFG}})$

Ans:  Join AF. ACFG is a square.

Now $\Delta {\text{ACF}}$ and $\Delta {\text{FCB}}$ are on the same base ${\text{FC}}$ and between the same parallels ${\text{FC}}$ and ${\text{AB}}$. 

$\therefore \operatorname{ar} (\Delta {\text{ACF}}) = \operatorname{ar} (\Delta {\text{FCB}})$ ………..(v)

From result (v), we get, 

$ar\left( {CYXE} \right) = 2\operatorname{ar} (\Delta {\text{FCB}}) \ldots  \ldots  \ldots .$ (v)

Using eq. (v) in (vi), we get,

$\operatorname{ar} ({\text{CYXE}}) = 2ar(\Delta {\text{ACF}})$ 

Diagonal AF of square ACFG divides it in two triangles of equal area.

$\therefore \operatorname{ar} ({\text{CYXE}}) = \operatorname{ar} ({\text{ sq}}{\text{. ACFG}}) \ldots  \ldots  \ldots ..{\text{ (vii) }}$

(vii) $\operatorname{ar} ({\text{BCED}}) = \operatorname{ar} ({\text{ABMN}}) + \operatorname{ar} ({\text{ACFG}})$

Ans:  Adding eq. (iv) and (vii), we get,

$\operatorname{ar} ({\text{BYXD}}) + \operatorname{ar} ({\text{CYXE}}) = \operatorname{ar} ({\text{ABMN}}) + \operatorname{ar} ({\text{ACFG}})$

$ \Rightarrow \operatorname{ar} ({\text{BCED}}) = \operatorname{ar} ({\text{ABMN}}) + \operatorname{ar} ({\text{ACFG}})$

4. Prove that the parallelogram which is a rectangle has the greatest area.

Ans: Let PQRS be a parallelogram in which ${\text{PQ}} = {\text{a}}$ and ${\text{PS}} = {\text{b}}$ and ${\text{h}}$ be the altitude corresponding to base PQ

Area of parallelogram ${\text{PQRS}} = $ Base $ \times $ corresponding Altitude $ = {\text{ah}}$

$\Delta PSK$ is a right angled triangle $b(PS)$ being its hypotenuse.

But hypotenuse is the greatest side of $\Delta $

Area of (ah) of | | gram PQRS will be greatest when $h$ is greatest

${\text{H}} = {\text{b}}$, then ${\text{PS}} \bot {\text{PQ}}$

The | | gram PQRS will be a rectangle.

Hence, the area of  is greatest when it is a rectangle.

5. Prove that If $\vartriangle {\text{ABC}}$ and $\Delta {\text{DBE}}$ are two equilateral triangles such that ${\text{D}}$ is the mid-point of ${\text{BC}}$ and AE intersects ${\text{BC}}$ at ${\text{F}}$

(i) $\operatorname{ar} (\Delta {\mathbf{BDE}}) = \dfrac{1}{4}\operatorname{ar} (\Delta {\mathbf{ABC}})$

Ans: Join EC

let a be the side of equilateral $\vartriangle ABC$

$\operatorname{ar} (\Delta ABC) = \dfrac{{\sqrt 3 }}{4}{a^2}$

$ar(\Delta BDE) = \dfrac{{\sqrt 3 }}{4}{\left( {\dfrac{a}{2}} \right)^2}$

$ = \dfrac{{\sqrt 3 }}{{16}}{a^2}$

$\operatorname{ar} (\Delta BDE) = \dfrac{1}{4}\operatorname{ar} (\Delta ABC)$

(ii) $\operatorname{ar} (\Delta {\mathbf{BDE}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\mathbf{BAE}})$

Ans:  $\operatorname{ar} (\Delta BDE) = \dfrac{1}{2}\operatorname{ar} (\Delta BEC)$

$\angle EBC = {60^\circ }$

$\angle BCA = {60^\circ }$

$\angle EBC = \angle BCA$

$\operatorname{ar} (\Delta BEC) = \operatorname{ar} (\Delta BAE)$

$\operatorname{ar} (BDE) = \dfrac{1}{2}\operatorname{ar} (\Delta BAE)$

6. Show that EFGH is a | | gram and its area is half of the area of | |gram ABCD. If E, F, G, ${\text{H}}$ are respectively the mid points of the sides AB, BC, CD and DA.

Ans: Join HF

${\text{E}}$ and ${\text{F}}$ are the mid-points of ${\text{AB}}$ and ${\text{BC}}$

$\therefore {\text{EF}} = \dfrac{1}{2}{\text{AC}}$ and ${\text{EF}}||{\text{AC}} \ldots  \ldots  \ldots ..$ (i)

Similarly, ${\text{GH}} = \dfrac{1}{2}{\text{AC}}$ and ${\text{GH}}||{\text{AC}} \ldots  \ldots  \ldots $ (ii)

From (i) and (ii)

${\text{GH}} = {\text{EF}}$ and ${\text{GH}}||{\text{EF}}$

$\therefore $ EFGH is a | | gram

$\operatorname{ar} (HGF) = \dfrac{1}{2}\operatorname{ar} (\Delta HDFC)$

(iii)

$\operatorname{ar} (HGF) = \dfrac{1}{2}\operatorname{ar} (\Delta HABF)$ (IV)

${\text{Adding}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{(iii)}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{and}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{(iv),}}$

$\operatorname{ar} (HGF) + \operatorname{ar} (HEF)= \dfrac{1}{2}\operatorname{ar} (\Delta ABCD)\operatorname{ar} (\Delta EFGH)$

7. Show that $\operatorname{ar} ({\text{BPC}}) = \operatorname{ar} ({\text{DPQ}})$ if ${\text{BC}}$ is produced to a point ${\text{Q}}$ such that ${\text{AD}} = {\text{CQ}}$ and ${\text{AQ}}$ intersect DC at P

Ans: Join AC

$\operatorname{ar} (\Delta BCP) = \operatorname{ar} (\Delta APC) \ldots (i)$

${\text{AD}} = {\text{CQ}}$

Hence, a pair of opposite side AD and CQ of the quadrilateral ADQC is equal and parallel. In $\vartriangle APC$ and $\Delta QPD$,

${\text{AP}} = {\text{QP}}$

${\text{CP}} = {\text{DP}}$

$\angle APC = \angle QPD$

$\vartriangle APC \cong \Delta QPD$

$\operatorname{ar} (\vartriangle APC) = \operatorname{ar} (\Delta QPD)......(ii)$

From (i) and (ii)

$\operatorname{ar} (\Delta BCP) = \operatorname{ar} (\Delta QPD)$

$\operatorname{ar} (BPC) = \operatorname{ar} (DPQ)$

8. If area of $\Delta PAB = K$ and two points ${\text{A}}$ and ${\mathbf{B}}$ are positive real number ${\text{K}}$. find the lows of a point ${\mathbf{p}}$

Ans: Let the perpendicular distance of ${\text{P}}$ from ${\text{AB}}$ be ${\text{h}}$

$\operatorname{ar} (\Delta PAB) = K$

$\dfrac{1}{2} \times (AB) \times h = K$

$h = \dfrac{{2K}}{{AB}}$

Since ${\text{AB}}$ and ${\text{K}}$ are given ${\text{h}}$ is a fixed Positive real number. This means that ${\text{P}}$ lies on a line Parallel to ${\text{AB}}$ at a distance ${\text{h}}$ from it.

Hence, the locus of ${\text{P}}$ is a pair of lines at a distance $h = \dfrac{{2K}}{{AB}}$, parallel to ${\text{AB}}$.

9. In figure, $P$ is a point in the interior of a parallelogram ABCD. Show that:

(i) $\operatorname{ar} ({\text{APB}}) + \operatorname{ar} ({\text{PCD}}) = \dfrac{1}{2}\operatorname{ar} ({\text{ABCD}})$

Ans:  Draw a line passing through point P and parallel to AB which intersects AD at Q and ${\text{BC}}$ at ${\text{R}}$ respectively.

Now $\Delta $ APB and parallelogram ABRQ are on the same base \[AB\]and between same parallels AB and QR.

Also $\Delta {\text{PCD}}$ and parallelogram DCRQ are on the same base ${\text{AB}}$ and between same parallels ${\text{AB}}$ and ${\text{QR}}$.

Adding eq. (i) and (ii),

(ii) $\operatorname{ar} ({\text{APD}}) + \operatorname{ar} ({\text{PBC}}) = \operatorname{ar} ({\text{APB}}) + \operatorname{ar} ({\text{PCD}})$

Ans: Draw a line through P and parallel to AD which intersects \[AB\]at $M$ and \[DC\]at $N$.

Now $\Delta $ APD and parallelogram AMND are on the same base AD and between same parallels AD and MN.

Also $\Delta {\text{PBC}}$ and parallelogram MNCB are on the same base ${\text{BC}}$ and between same parallels BC and MN.

Adding eq. (i) and (ii),

From eq. (iii) and (vi), we get,

$\operatorname{ar} (\Delta {\text{APB}}) + \operatorname{ar} (\Delta {\text{PCD}}) = \operatorname{ar} (\Delta {\text{APD}}) + \operatorname{ar} (\Delta {\text{PBC}})$

or $ar(\Delta {\text{APD}}) + \operatorname{ar} (\Delta {\text{PBC}}) = \operatorname{ar} (\Delta {\text{APB}}) + \operatorname{ar} (\Delta {\text{PCD}})$

Hence proved.

10. D, E and $F$ are respectively the mid-points of the sides \[BC,CA\]and \[AB\]of a $\Delta ABC$. Show that:

(i) BDEF is a parallelogram.

Ans: ${\text{F}}$ is the mid-point of ${\text{AB}}$ and ${\text{E}}$ is the mid-point of ${\text{AC}}$ is the part of ${\text{BC}}]$ And $FE = BD$

Also, D is the mid-point of BC.

$\therefore {\text{BD}} = \dfrac{1}{2}{\text{BC}}$

And  and ${\text{FE}} = {\text{BD}}$

Again ${\text{E}}$ is the mid-point of ${\text{AC}}$ and ${\text{D}}$ is the mid-point of ${\text{BC}}$.

 and ${\text{DE}} = \dfrac{1}{2}{\text{AB}}$

 is the part of ${\text{AB}}]$

And ${\text{DE}} = {\text{BF}}$

Again ${\text{F}}$ is the mid-point of ${\text{AB}}$.

$\therefore {\text{BF}} = \dfrac{1}{2}{\text{AB}}$

${\text{But DE }} = \dfrac{1}{2}{\text{AB}}$

$\therefore {\text{DE}} = {\text{BF}}$

Now we have  and  

And ${\text{FE}} = {\text{BD}}$ and ${\text{DE}} = {\text{BF}}$

Therefore, BDEF is a parallelogram.

(ii) $\operatorname{ar} ({\text{DEF}}) = \dfrac{1}{4}\operatorname{ar} ({\text{ABC}})$

Ans: ${\text{BDEF}}$ is a parallelogram.

$\therefore \operatorname{ar} (\Delta {\text{BDF}}) = \operatorname{ar} (\Delta {\text{DEF}}).$

DCEF is also parallelogram.

$\therefore \operatorname{ar} (\Delta {\text{DEF}}) = \operatorname{ar} (\Delta {\text{DEC}}).$

Also, AEDF is also parallelogram.

$\therefore \operatorname{ar} (\Delta {\text{AFE}}) = \operatorname{ar} (\Delta {\text{DEF}}).$

From eq. (i), (ii) and (iii),

$\operatorname{ar} (\Delta {\text{DEF}}) = \operatorname{ar} (\Delta {\text{BDF}}) = \operatorname{ar} (\Delta {\text{DEC}}) = \operatorname{ar} (\Delta {\text{AFE}}) \ldots  \ldots  \ldots ..$ (iv)

Now, $ar(\Delta {\text{ABC}}) = \operatorname{ar} (\Delta {\text{DEF}}) + \operatorname{ar} (\Delta {\text{BDF}}) + \operatorname{ar} (\Delta {\text{DEC}}) + \operatorname{ar} (\Delta {\text{AFE}}) \ldots  \ldots  \ldots .$ (v)

$ \Rightarrow \operatorname{ar} (\Delta {\text{ABC}}) = \operatorname{ar} (\Delta {\text{DEF}}) + \operatorname{ar} (\Delta {\text{DEF}}) + \operatorname{ar} (\Delta {\text{DEF}}) + \operatorname{ar} (\Delta {\text{DEF}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{ABC}}) = 4 \times \operatorname{ar} (\Delta {\text{DEF}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{DEF}}) = \dfrac{1}{4}\operatorname{ar} (\Delta {\text{ABC}})$

(iii) $\operatorname{ar} ({\text{BDEF}}) = \dfrac{1}{2}\operatorname{ar} ({\text{ABC}})$

Ans: Since, $\operatorname{ar}(\triangle \mathrm{BDF})=\operatorname{ar}(\triangle \mathrm{DEF})$

DE is a diagonal of parallelogram DCEF.

So, $\operatorname{ar}(\triangle \mathrm{DCE})=\operatorname{ar}(\triangle \mathrm{DEF}) \quad \ldots . .(2)$

FE is a diagonal of parallelogram AFDE.

$\operatorname{ar}(\triangle \mathrm{AF} \mathrm{E})=\operatorname{ar}(\triangle \mathrm{DEF})$

From $1,2 \& 3$, we have,

$\operatorname{ar}(\triangle \mathrm{BDF})=\operatorname{ar}(\triangle \mathrm{DCE})=\operatorname{ar}(\triangle \mathrm{AF} \mathrm{E})=\operatorname{ar}(\triangle \mathrm{DEF})$

But, $\operatorname{ar}(\triangle \mathrm{BDF})+\operatorname{ar}(\triangle \mathrm{DCE})+\operatorname{ar}(\triangle \mathrm{AF} \mathrm{E})+\operatorname{ar}(\triangle \mathrm{DEF})=\operatorname{ar}(\triangle \mathrm{ABC})$

So, $4 \operatorname{ar}(\triangle \mathrm{DEF})=\operatorname{ar}(\triangle \mathrm{ABC})$

$\operatorname{ar}(\triangle \mathrm{DEF})=\dfrac{1}{4} \operatorname{ar}(\triangle \mathrm{ABC})$

Now, $\operatorname{ar}\left(\|^{g m} \mathrm{BDEF}\right)=2 \operatorname{ar}(\triangle \mathrm{DEF})$

$\operatorname{ar}\left(\|^{\mathrm{gm}} \mathrm{BDEF}\right)=2 \times \dfrac{1}{4} \operatorname{ar}(\triangle \mathrm{ABC})=\dfrac{1}{2} \operatorname{ar}(\triangle \mathrm{ABC})$

11. In figure, diagonals AC and BD of quadrilateral ABCD intersect at 0 such that $OB = $ OD. If $AB = CD$, then show that:

(i) $\operatorname{ar} ({\mathbf{DOC}}) = \operatorname{ar} ({\text{AOB}})$

Ans: Draw ${\text{BM}} \bot {\text{AC}}$ and ${\text{DN}} \bot {\text{AC}}$

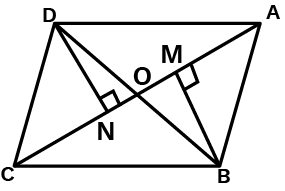
In $\Delta {\text{DON}}$ and $\Delta {\text{BOM}}$

${\text{OD}} = {\text{OB}}$

$\angle {\text{DNO}} = \angle {\text{BMO}} = {90^\circ }$ (By construction)

$\angle {\text{DON}} = \angle {\text{BOM}}$ (Vertically opposite)

$\therefore \Delta {\text{DON}} \cong \Delta {\text{BOM}}$ (By RHS congruency)

$ \Rightarrow {\text{DN}} = {\text{BM}}[{\text{ByCPCT}}]$

Also $ar(\Delta {\text{DON}}) = \operatorname{ar} (\Delta {\text{BOM}})$ …...(i)

Again, In $\Delta {\text{DCN}}$ and $\Delta {\text{ABM}}$,

${\text{CD}} = {\text{AB}}[$ Given]

$\angle {\text{DNC}} = \angle {\text{BMA}} = {90^\circ }$ (By construction)

DN = BM [Prove above]

$\therefore \Delta {\text{DCN}} \cong \Delta $ BAM (By RHS congruency)

$\therefore \operatorname{ar} (\Delta {\text{DCN}}) = \operatorname{ar} (\Delta {\text{BAM}})$

Adding eq. (i) and (ii),

$\operatorname{ar} (\Delta {\text{DON}}) + \operatorname{ar} (\Delta {\text{DCN}}) = \operatorname{ar} (\Delta {\text{BOM}}) + \operatorname{ar} (\Delta {\text{BAM}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{DOC}}) = \operatorname{ar} (\Delta {\text{AOB}})$

(ii) $\operatorname{ar} ({\mathbf{DCB}}) = \operatorname{ar} ({\text{ACB}})$

Ans:  Since $\operatorname{ar} (\Delta {\text{DOC}}) = \operatorname{ar} (\Delta {\text{AOB}})$

Adding $ar\Delta {\text{BOC}}$ both sides,

$\operatorname{ar} (\Delta {\text{DOC}}) + {\text{ar}}\Delta {\text{BOC}} = \operatorname{ar} (\Delta {\text{AOB}}) + {\text{ar}}\Delta {\text{BOC}}$

$ \Rightarrow \operatorname{ar} (\Delta {\text{DCB}}) = \operatorname{ar} (\Delta {\text{ACB}})$

 (iii) DA  CB or ABCD is a parallelogram.

Ans: Since $ar(\Delta {\text{DCB}}) = \operatorname{ar} (\Delta {\text{ACB}})$

Therefore, these two triangles in addition to be on the same base ${\text{CB}}$ lie between two same parallels ${\text{CB}}$ and DA.

Now ${\text{AB}} = {\text{CD}}$ and 

Therefore, ${\text{ABCD}}$ is a parallelogram.

12. In figure, ${\text{ABC}}$ and ${\text{BDF}}$ are two equilateral triangles such that ${\text{D}}$ is the mid-point of BC. If AE intersects BC at F, show that:

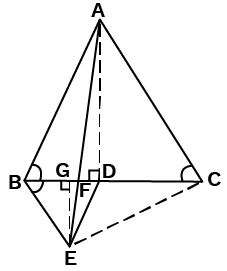
(i) $\operatorname{ar} ({\text{BDE}}) = \dfrac{1}{4}\operatorname{ar} ({\text{ABC}})$

Ans: Join EC and AD.

Since $\Delta {\text{ABC}}$ is an equilateral triangle.

$\therefore \angle {\text{A}} = \angle {\text{B}} = \angle {\text{C}} = {60^\circ }$

Also $\Delta {\text{BDE}}$ is an equilateral triangle.

$\therefore \angle {\text{B}} = \angle {\text{D}} = \angle {\text{E}} = {60^\circ }$

If we take two lines, ${\text{AC}}$ and ${\text{BE}}$ and ${\text{BC}}$ as a transversal.

Then $\angle {\text{B}} = \angle {\text{C}} = {60^\circ }$ [Alternate angles]

Similarly, for lines AB and DE and BF as transversal.

Then $\angle {\text{B}} = \angle {\text{C}} = {60^\circ }$ [Alternate angles]

Area of equilateral triangle ${\text{BDE}} = \dfrac{{\sqrt 3 }}{4}{({\text{BD}})^2} \ldots  \ldots  \ldots $(i)

Area of equilateral triangle ${\text{ABC}} = \dfrac{{\sqrt 3 }}{4}{({\text{BC}})^2}.$…….. (ii)

Dividing eq. (i) by (ii),

$\dfrac{{\operatorname{ar} (\Delta {\text{BDE}})}}{{\operatorname{ar} (\Delta {\text{ABC}})}} = \dfrac{{\dfrac{{\sqrt 3 }}{4}{{({\text{BD}})}^2}}}{{\dfrac{{\sqrt 3 }}{4}{{({\text{BC}})}^2}}}$

$ \Rightarrow \dfrac{{\operatorname{ar} (\Delta {\text{BDE}})}}{{\operatorname{ar} (\Delta {\text{ABC}})}} = \dfrac{{\dfrac{{\sqrt 3 }}{4}{{({\text{BD}})}^2}}}{{\dfrac{{\sqrt 3 }}{4}{{(2{\text{BD}})}^2}}}$

$ \Rightarrow \dfrac{{\operatorname{ar} (\Delta {\text{BDE}})}}{{\operatorname{ar} (\Delta {\text{ABC}})}} = \dfrac{{{{({\text{BD}})}^2}}}{{{{(2{\text{BD}})}^2}}}$

$ \Rightarrow \dfrac{{\operatorname{ar} (\Delta {\text{BDE}})}}{{{\text{ar}}(\Delta {\text{ABC}})}} = \dfrac{1}{4}$

$ \Rightarrow \operatorname{ar} (\Delta {\text{BDE}}) = \dfrac{1}{4}\operatorname{ar} (\Delta {\text{ABC}})$

(ii) $\operatorname{ar} ({\text{BDE}}) = \dfrac{1}{2}\operatorname{ar} ({\text{BAE}})$

Ans: In $\Delta $ BEC, ED is the median.

$\therefore \operatorname{ar} (\Delta {\text{BEC}}) = \operatorname{ar} (\Delta {\text{BAE}}) \ldots  \ldots  \ldots {\text{ (i) }}$

Now BE 

And $\Delta {\text{BEC}}$ and $\Delta {\text{BAE}}$ are on the same base ${\text{BE}}$ and between the same parallels ${\text{BE}}$ and ${\text{AC}}$.

$\therefore \operatorname{ar} (\Delta {\text{BEC}}) = \operatorname{ar} (\Delta {\text{BAE}}) \ldots  \ldots  \ldots ..$ (ii)

Using eq. (i) and (ii), we get

$\operatorname{Ar} (\Delta {\text{BDE}}) = \dfrac{1}{2}\operatorname{ar} (\Delta {\text{BAE}})$

(iii) $\operatorname{ar} ({\text{ABC}}) = 2\operatorname{ar} ({\text{BEC}})$

Ans: We have $ar(\Delta {\text{BDE}}) = \dfrac{1}{4}ar(\Delta {\text{ABC}})$  [Proved in part (i)] ..........(iii) 

$\operatorname{ar} (\Delta {\text{BDE}}) = \dfrac{1}{4}\operatorname{ar} (\Delta $ BAE) 

$\operatorname{ar} (\Delta {\text{BDE}}) = \dfrac{1}{4}\operatorname{ar} (\Delta {\text{BEC}})$ [Using eq. (iii)] ...........(iv)

From eq. (iii) and (iv), we het

$\dfrac{1}{4}\operatorname{ar} (\Delta {\text{ABC}}) = \dfrac{1}{4}\operatorname{ar} (\Delta {\text{BEC}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{ABC}}) = 2\operatorname{ar} (\Delta {\text{BEC}})$

(iv) $\operatorname{ar} ({\text{BFE}}) = \operatorname{ar} ({\text{AFD}})$

Ans: $\Delta {\text{BDE}}$ and $\Delta {\text{AED}}$ are on the same base ${\text{DE}}$ and between same parallels ${\text{AB}}$ and ${\text{DE}}$.

$\therefore \operatorname{ar} (\Delta {\text{BDE}}) = \operatorname{ar} (\Delta {\text{AED}})$

Subtracting $\Delta $ FED from both the sides,

$\operatorname{ar} (\Delta {\text{BDE}}) - \operatorname{ar} (\Delta {\text{FED}}) = \operatorname{ar} (\Delta {\text{AED}}) - \operatorname{ar} (\Delta {\text{FED}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{BFE}}) = \operatorname{ar} (\Delta {\text{AFD}}) \ldots  \ldots  \ldots ({\text{v}})$

(v) $\operatorname{ar} ({\text{BFE}}) = 2{\text{ar}}$ (FED)

Ans:  An in equilateral triangle, median drawn is also perpendicular to the side,

$\therefore {\text{AD}} \bot {\text{BC}}$

Now $ar(\Delta {\text{AFD}}) = \dfrac{1}{2} \times FD \times AD$ (vi)

Draw EG $ \bot BC$

$\therefore \operatorname{ar} (\Delta {\text{FED}}) = \dfrac{1}{2} \times FD \times EG \ldots  \ldots  \ldots .$ (vii)

Dividing eq. (vi) by (vii), we get

$\dfrac{{\operatorname{ar} (\Delta {\text{AFD}})}}{{\operatorname{ar} (\Delta {\text{FED}})}}\dfrac{{\dfrac{1}{2} \times {\text{FD}} \times {\text{AD}}}}{{\dfrac{1}{2} \times {\text{FD}} \times {\text{EG}}}}$

$ \Rightarrow \dfrac{{{\text{ ar }}(\Delta {\text{AFD}})}}{{\operatorname{ar} (\Delta {\text{FED}})}} = \dfrac{{{\text{AD}}}}{{{\text{EG}}}}$

$ \Rightarrow \dfrac{{\operatorname{ar} (\Delta {\text{AFD}})}}{{\operatorname{ar} (\Delta {\text{FED}})}} = \dfrac{{\dfrac{{\sqrt 3 }}{4}{\text{BC}}}}{{\dfrac{{\sqrt 3 }}{4}{\text{BD}}}}$

$ \Rightarrow \dfrac{{\operatorname{ar} (\Delta {\text{AFD}})}}{{\operatorname{ar} (\Delta {\text{FED}})}} = \dfrac{{2{\text{BD}}}}{{{\text{BD}}}}$

$ \Rightarrow \dfrac{{\operatorname{ar} (\Delta {\text{AFD}})}}{{\operatorname{ar} (\Delta {\text{FED}})}} = 2$

$ \Rightarrow \operatorname{ar} (\Delta {\text{AFD}}) = 2\operatorname{ar} (\Delta {\text{FED}}) \ldots  \ldots {\text{ (viii) }}$

${\text{Using}}{\kern 1pt} {\kern 1pt} {\kern 1pt} \,{\text{the}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{value}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{of}}{\kern 1pt} {\kern 1pt} {\text{eq}}{\text{.(viii)}}{\kern 1pt} \,{\text{in}}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\text{eq}}{\text{.(v)}},$

$\operatorname{Ar} (\Delta {\text{BFE}}) = 2\operatorname{ar} (\Delta {\text{FED}})$

(vi) $\operatorname{ar} ({\text{FED}}) = \dfrac{1}{8}\operatorname{ar} ({\text{AFC}})$

Ans:  $\operatorname{ar} (\Delta {\text{AFC}}) = \operatorname{ar} (\Delta {\text{AFD}}) + \operatorname{ar} (\Delta {\text{ADC}}) = 2\operatorname{ar} (\Delta {\text{FED}}) + \dfrac{1}{2}\operatorname{ar} (\Delta {\text{ABC}})$

$ = 2\operatorname{ar} (\Delta {\text{FED}}) + \dfrac{1}{2}[4{\text{x}}{\mathbf{ar}}(\Delta {\text{BDE}})]$  (Using result of part (i))

$ = 2\operatorname{ar} (\Delta {\text{FED}}) + 2\operatorname{ar} (\Delta {\text{BDE}}) = 2\operatorname{ar} (\Delta {\text{FED}}) + 2\operatorname{ar} (\Delta {\text{AED}})$

[ $\Delta {\text{BDE}}$ and $\Delta {\text{AED}}$ are on the same base and between same parallels]

$ = 2\operatorname{ar} (\Delta {\text{FED}}) + 2[\operatorname{ar} (\Delta {\text{AFD}}) + \operatorname{ar} (\Delta {\text{FED}})]$

$ = 2\operatorname{ar} (\Delta {\text{FED}}) + 2\operatorname{ar} (\Delta {\text{AFD}}) + 2\operatorname{ar} (\Delta {\text{FED}})$

$ = 4\operatorname{ar} (\Delta {\text{FED}}) + 4\operatorname{ar} (\Delta {\text{FED}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{AFC}}) = 8\operatorname{ar} (\Delta {\text{FED}})$

$ \Rightarrow \operatorname{ar} (\Delta {\text{FED}}) = \dfrac{1}{8}\operatorname{ar} (\Delta {\text{AFC}})$

Advantages of the Important Questions

Because the laws of Mathematics are all around us, arithmetic is vital to everyday life. It is a subject that requires a lot of practice and understanding of many formulae in order to answer various numerical issues. Students in Class 9 can now improve their arithmetic problem-solving abilities by practising the important problems for Class 9 Maths questions that are supplied here. The major advantages of solving the important questions include the following:

• The questions are curated to cover the complete syllabus of the topic.  

• In order for students to perform well on their exams, all of the questions have been chosen from the Class 9 Mathematics updated syllabus.

• The important questions will give you a competitive edge in your preparations.

• The solutions to the important questions were designed by our subject specialists in a step-wise manner, and we at Vedantu give you the answers as part of your preparation.

• It will help you understand the trends and weightage of the questions followed in the exam.

Important Related Links for CBSE Class 9