




Concepts of Rotational Motion for JEE Main Physics
The rotational motion of the objects plays an important role in understanding dynamics in 3D. In the past chapters, students have learned about the motion in one and two-dimensional planes, where an object will be in a straight line motion. Now the question arises will the same kinematics theory and equations be applicable if the object starts rolling? To answer this question students should study the chapter on rotational motion and understand what is rotational motion.
The rotational motion chapter deals with all the phenomena and theories related to rotation and rotational motion examples. In the rotational motion chapter, we will look at the kinematics and dynamics of a solid body in two different motions. Rotation around a stationary axis, often known as pure rotation, is the first type of motion for a solid body. The second type of solid motion is plane motion, in which the solid body's centre of mass moves in a fixed direction while the body's angular velocity remains perpendicular to that plane at all times.
Furthermore, in the rotational motion chapter, we study the rotational dynamics and the parameters associated with it. The chapter includes many important concepts such as angular velocity, angular acceleration, perpendicular axis theorem, parallel axis theorem, and torque, and we define the moment of inertia of various objects such as a ring, disc, cylinder, etc.. which are all important in exam perspective.
In this article, we will cover the concepts which are useful for the JEE exam and boost their exam preparation.
JEE Main Physics Chapters 2025
Important Topics of Rotational Motion Chapter
Rigid Bodies
Rotational Kinematics
Moment of Inertia
Parallel Axis Theorem
Perpendicular Axis Theorem
Torque
Angular Momentum
Angular Displacement
Conservation of Angular Momentum
Instantaneous Axis of Rotation
Rolling motion
Toppling
Rotational Motion Important Concept for JEE Main
Torque on a Rotating Rigid Object
Some coupled forces on a rigid body cause it to rotate along its axis. These forces are called torque or moment of force. The figure below depicts the torque on a rigid body.
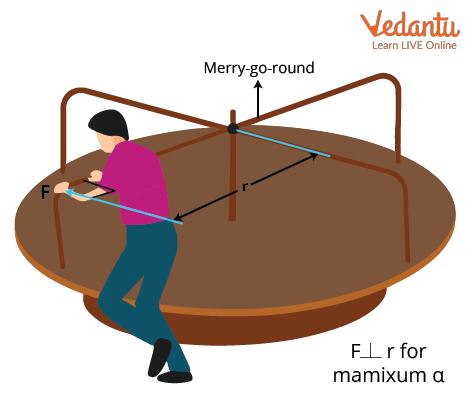
Torque on a Merry-go-round
The torque of a rotating rigid body is denoted as $\tau=r . f=r F \sin \theta$
Where, $\tau$ is the torque, F is the force applied, r is the distance between an applied force from its axis of rotation and $\theta$ is the angle between r and F.
In case the direction of F and r are parallel to one another the angle between them is zero, thus making the value of $\sin \theta$ as zero. It makes the net torque acting on a body to be equal to zero.
Work Done in Rotational Motion
According to the work-energy principle, the total work done on a body is the sum of all the forces acting on it which is equal to the change in kinetic energy of the body.
As we know that the work done on an object is equal to the change in its kinetic energy thus work is denoted as
$W=\Delta K E=K E_{2}-K E_{1}=\dfrac{1}{2} I\left(\omega_{2-}^{2} \omega_{1}^{2}\right)$
Power in Rotational Motion
Power for both linear and rotational motion is important. The power for a linear motion is denoted as $P=\vec{F}$ where the force is constant. In the section below the torque is considered to be constant. From the work and kinetic energy that has been derived above the power in rotational motion is defined as the rate of doing work.
$P=\dfrac{dW}{dt}$
If the net torque is constant, then work done become $W=\tau\theta$, the power then is calculated as $P=\dfrac{dW}{dt}$
On substituting the value of W we get,
$\begin{align} &\mathrm{P}=\dfrac{d(\tau \theta)}{d t}\\ &\mathrm{P} =\tau \dfrac{d \theta}{d t}\\ &\text { or } P=\tau \omega \end{align}$
Rotational Dynamics
Rotational Dynamics are completely analogous to linear motion. Depicted below is the comparison between the quantities in translation or linear and rotational dynamics.
From the table above it is clear that force in a linear motion is the torque for the rotational motion.
List of Important Formulas for Rotational Motion Chapter
Important Comparison of Rotational Kinematics and Linear Kinematics
When we start preparing for our exams its essential to know our understanding with the help of comparisions, differences between the concepts we have previously studied. We know the kinematics of linear motion, now let us have a look at the comparison of linear kinematics with rotational motion.
JEE Main Rotational Motion Solved Examples
The moment of inertia of a uniform cylinder of length and radius R about its perpendicular bisector is I. What is the ratio l/R such that the moment of inertia is minimum?
Sol:
Given,
We have a uniform cylinder of length l and radius R, such that the pendicular bisector is I. Now we are asked find the ratio $\dfrac {l}{R}$.
We know that, moment of inertia of a cylinder of length l is:
$I=\dfrac {M{R^2}}{4} +\dfrac {M {l^2}}{12}$
$I=\dfrac {M^2}{4{\rho}{\pi}l}+\dfrac {M{l^2}}{12}$
For maximum I, we have $\dfrac {dI}{dl}=0$
$\dfrac {dI}{dl}=\dfrac {-M^2}{4{\pi}{\rho}{l^2}}+\dfrac {M{l}}{6}=0$
$\dfrac {M^2}{4{\pi}{\rho}{l^2}}=\dfrac {M{l}}{6}$
$\dfrac {M}{{4{\pi}{\rho}}}=\dfrac {l^3}{6}$
$\dfrac {4 \pi \rho {R^2}{l}}{{4{\pi}{\rho}}}=\dfrac {l^3}{6}$
$\dfrac {l^2}{R^2}=\dfrac {3}{2}$
$\dfrac {l}{R}=\sqrt {\dfrac {3}{2}}$
Therefore, the ratio of $\dfrac {l}{R}$ is $\sqrt {\dfrac {3}{2}}$.
Key point: Here we should use the formula of volume mass density $\rho=\dfrac {m}{4\pi {R^2} l}$. While solving problems related to cylinder or any other geometrical objects we must remember that the mass density will vary from object to object.
Seven identical circular planar disks, each of mass M and radius R are welded symmetrically as shown. The moment of inertia of the arrangement about the axis normal to the plane and passing through the point P is?
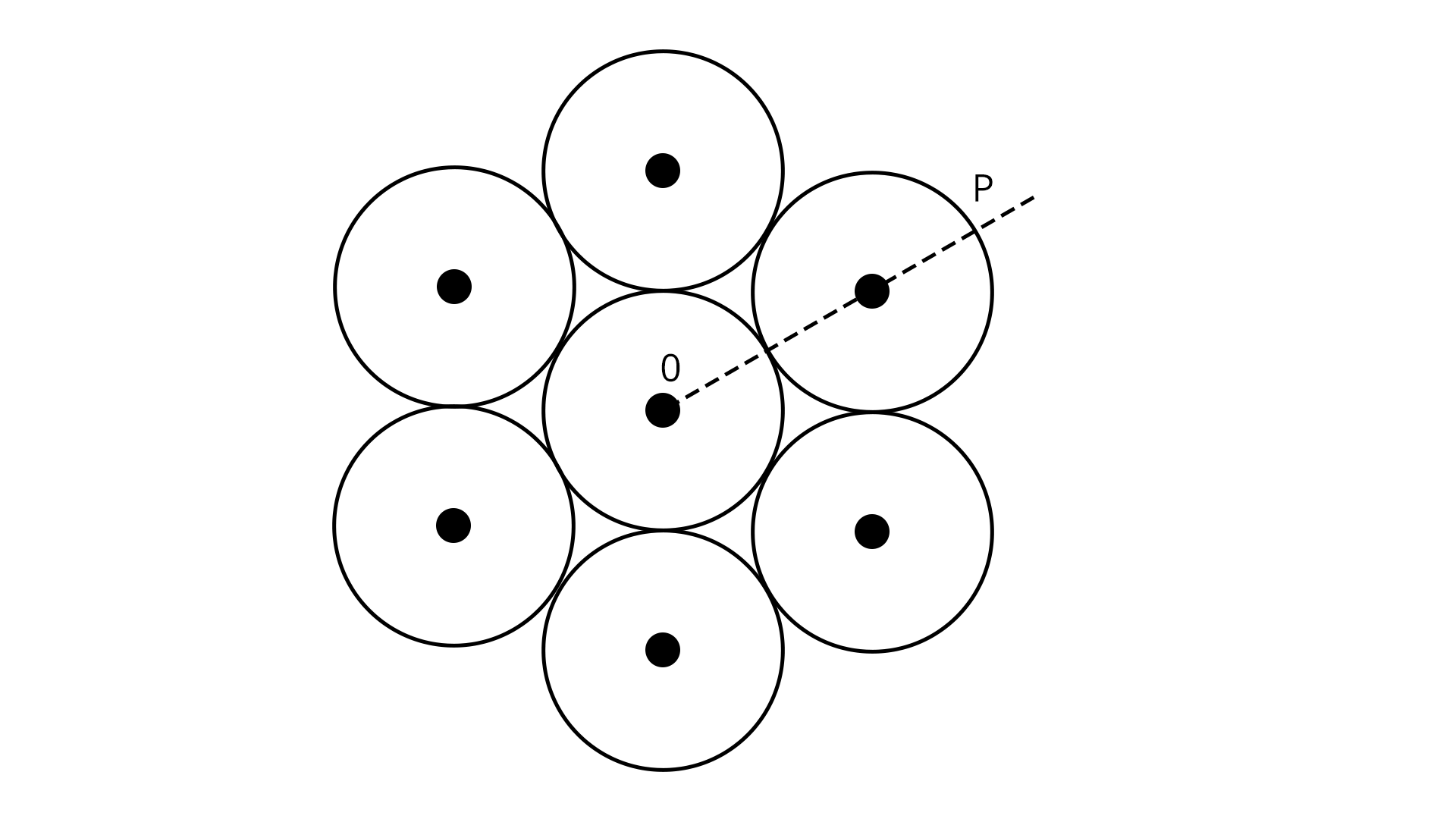
Sol:
Here, the arrangement of seven identical disks are welded symmetrically, thus all the discs will rotate about center of mass. We know that, according to the parallel axis theorem, The moment of inertia of a body about any axis is equal to the sum of the Moment of Inertia of the body about a parallel axis passing through its centre of mass and the product of its mass and the square of the distance between the two parallel axes.
I.e.,
$I={I_C}+M {d^2}$
Where,
d- The perpendicular distance between the two axes.
${I_o}=\dfrac {7M{R^2}}{2}+6M {{\left (2R\right)}^2}=\dfrac {55 M{R^2}}{2}$
Now, The moment of inertia of the arrangement about the axis normal to the plane and passing through the point P is:
${I_P}={I_o}+M{d^2}$
${I_P}=\dfrac {55 M{R^2}}{2}+7M{\left (3R\right )}^2=\dfrac {181}{2} M{R^2}$
Key point: While solving this question, we must first calculate the moment of inertia of the entire system of seven particles, and then we can calculate the moment of inertia at any point on the disk with the help of parallel axis theorem.
Previous Year Questions of Rotational Motion
A 2 kg steel rod of length 0.6 m is clamped on a table vertically at its lower end and is free to rotate in the vertical plane. The upper end is pushed so that the rod falls under gravity, ignoring the friction due to clamping at its lower end, the speed of the free end of the rod when it passes through its lowest position is ____________ ms-1. (Take g = 10 ms-2) (JEE 2021)
Sol:
Given,
Mass of the steel rod=M= 2 kg
Length of the steel rod=l=0.6 m
The given rod is clamped on a table vertically at its lower end and its free to rotate in a vertical plane. Now, we are asked to determine the speed of the free end of the rod when it passes through its lowest position.
Here, we can use the conservation of energy to obtain the speed. From conservation of energy we get:
P.E=K.E
$mgl=\dfrac {1}{2} I {\omega^2}$
$mgl=\dfrac {1}{2} \dfrac {m {l^2}{\omega^2}}{3}$
$\omega =\sqrt {\dfrac {6g}{l}}$
Speed $v=l\omega=l\times \sqrt {\dfrac {6g}{l}}=\sqrt {6gl}$
$v=6 m/s$
Therefore, the speed of the free end of rod when it passes through its lowest position 6 m/s.
Trick: Here, we can notice that the one end of the rod is fixed, thus we can use the moment of inertia formula when the axis of rotation is perpendicular to the rod at one end i.e., $I=\dfrac {M{l^2}}{3}$.
The centre of mass of the solid hemisphere of radius 8 cm is x from the centre of the flat surface. Then value of x is __________. (JEE 2020)
Sol:
We know that centre of mass of a solid hemisphere is,
$x=\dfrac {3R}{8}=\dfrac {3\times 8}{8}=3 cm$
Therefore, the value of x is 3 cm.
Trick: For this kind of problem we need to recall and practice COM of a few geometrical shapes, which will save our time calculation.
Practice Questions
1. A uniform thin bar of mass 6 kg and length of 2.4 meters is bent to make an equilateral hexagon. The moment of inertia about an axis passing through the centre of mass and perpendicular to the plane of a hexagon is _______ 10-1 kg m2.
(Ans: 8)
2. A small bob tied at one end of a thin string of length 1 m is describing a vertical circle so that the maximum and minimum tension in the string is in the ratio 5: 1. The velocity of the bob at the highest position is ________ m/s. (Take g = 10 m/s2)
(Ans: 5)
3. What is the physical significance of the moment of inertia?
JEE Main Physics Rotational Motion Study Materials
Here, you'll find a comprehensive collection of study resources for Rotational Motion designed to help you excel in your JEE Main preparation. These materials cover various topics, providing you with a range of valuable content to support your studies. Simply click on the links below to access the study materials of Rotational Motion and enhance your preparation for this challenging exam.
JEE Main Physics Study and Practice Materials
Explore an array of resources in the JEE Main Physics Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Main exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Physics preparation.
Conclusion
In this article, we discussed one of the important chapters for JEE, rotational motion. We have covered most of the important concepts, formulae, and solved examples along with recently asked questions in JEE exams. In this article, we have also studied how linear kinematics is different from the rotational kinematics and had an overview of the rotational kinematic equations and what rotary motion, translational motion, etc... This article will help students prepare for JEE exam and boost their preparation in one stance.
FAQs on Rotational Motion Chapter - Physics JEE Main
1. Is rotational motion important for JEE?
When it comes to the physics section of JEE advanced exams, mechanics make up a large portion of the problems, with rotational mechanics being the most crucial. Rotational motion includes the concepts that carry most of the important formulae for the calculation of JEE questions.
2. What is the weightage of rotational motion in JEE main?
The rotational motion chapter is having high importance because of its vast syllabus and concepts. The chapter plays an important role in the mechanics part with a weightage of 6% which makes 3-4 questions (2 from dynamics and 2 from centre of mass).
3. Is rotational motion hard?
Rotational Motion (Rotational Mechanics) is considered to be one of the tricky topics in Class 11 JEE Physics. With the help of continuous practice and understanding of the formulas, students can easily solve questions from the rotational motion chapter.

















