Kinematics Class 11 Chapter for JEE Main 2025 Preparation
Kinematics is an important topic for JEE exams. It mainly consists of one-dimensional and two-dimensional motion, and the questions asked in the examination are based on their applications. Compared to other concepts, this section will become easier with adequate practice and conceptual understanding.
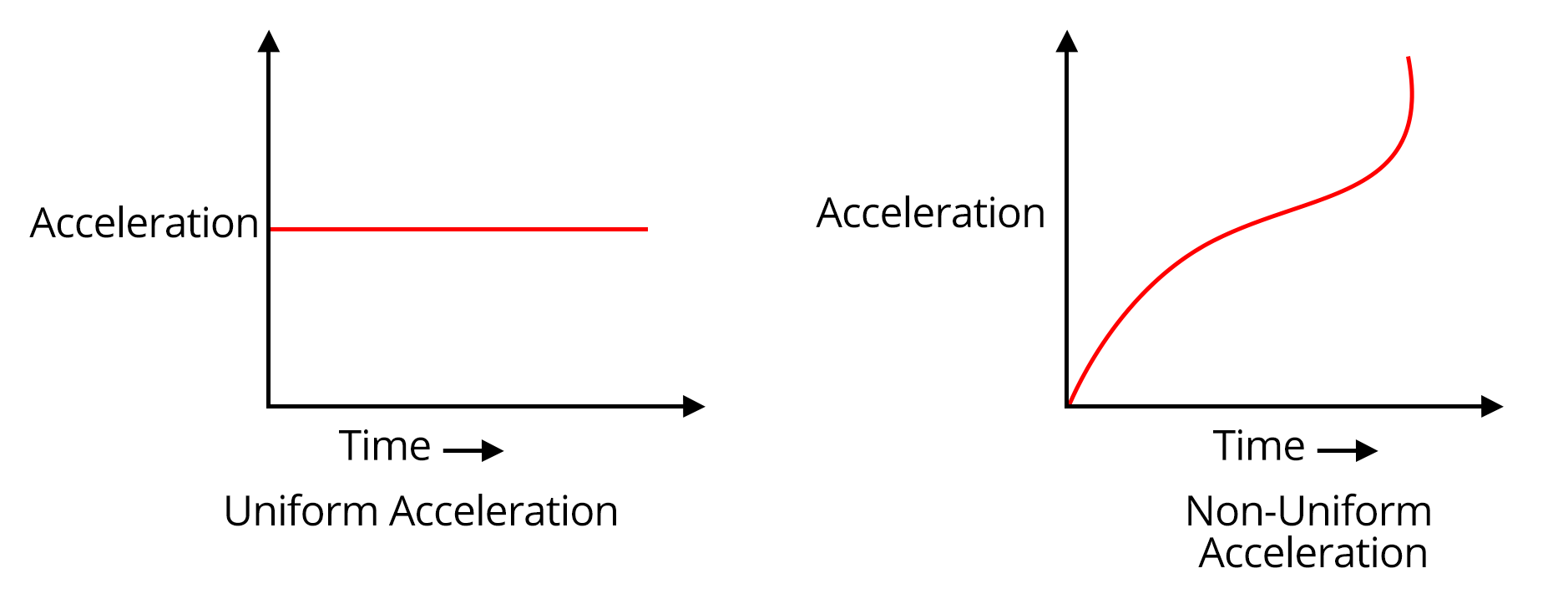
In kinematics, first, we will learn about motion along a straight line and discuss different physical quantities like distance, displacement, velocity, etc., and their formulas. Different types of motions are also discussed in this section. Problems related to the three kinematical equations of motion for uniformly accelerated motion are very important. We will also see how we can draw a conclusion about the motion by evaluating and finding required quantities from various graphs.
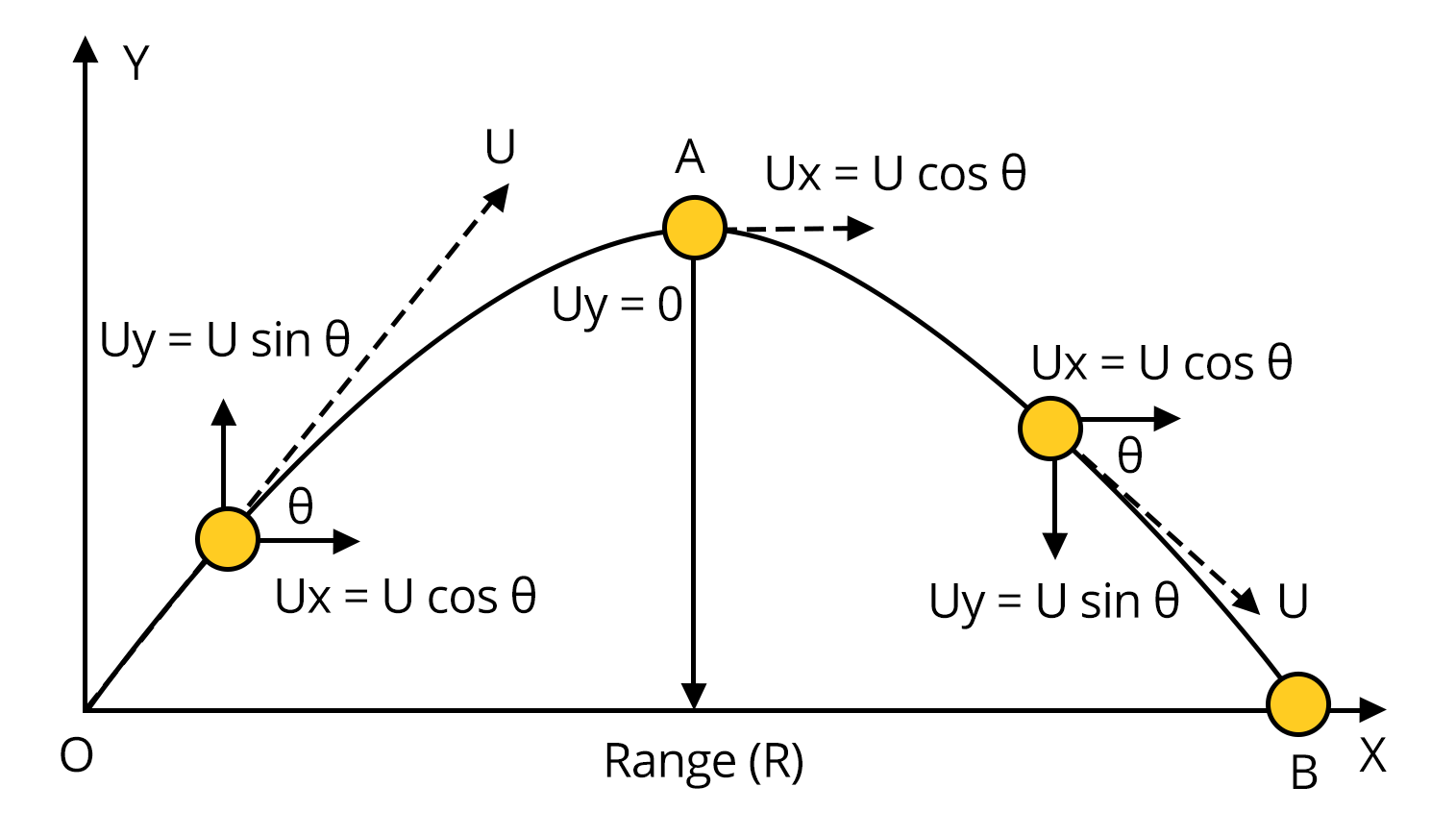
Coming to motion along a plane, properties of vectors and the law of vector addition like triangular law and parallelogram law of vector additions, scalar and vector product of vectors are important. The problems with projectile motion on a horizontal and inclined plane are frequently asked in JEE exam.
Let us see what is kinematics and the important concepts and formulas needed to score a full marks for this topic in JEE Main with the help of some solved kinematics examples.
Important Topics of Kinematics Chapters
Motion in a Straight Line
Kinematics Equations
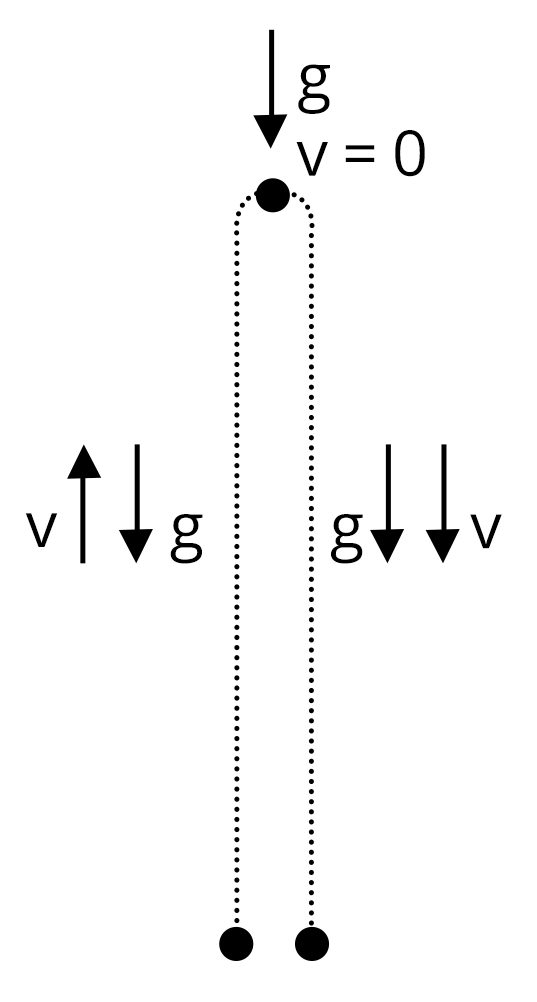
Motion under gravity
Graphs for all types of motion
Motion in a Plane
Projectile Motion
Resolution of Vector Rectangular Components
Vector Addition
Circular Motion
Kinematics Important Concept for JEE Main
Frame of Reference:
Frame of reference in kinematics is a coordinate system from which motion is measured. It is a conceptual framework that is used to describe and analyze the motion of objects.
There are two types of frames of reference: inertial and non-inertial.
Inertial frames of reference are at rest or moving at a constant velocity in a straight line. In an inertial frame of reference, Newton's laws of motion hold true.
Non-inertial frames of reference are accelerating or rotating. In a non-inertial frame of reference, Newton's laws of motion do not hold true without modification.
In addition to the analytical methods discussed above, you can also use graphical analysis to solve kinematics problems. Graphical Analysis of Kinematics is a powerful tool that can be used to visualize and understand motion.
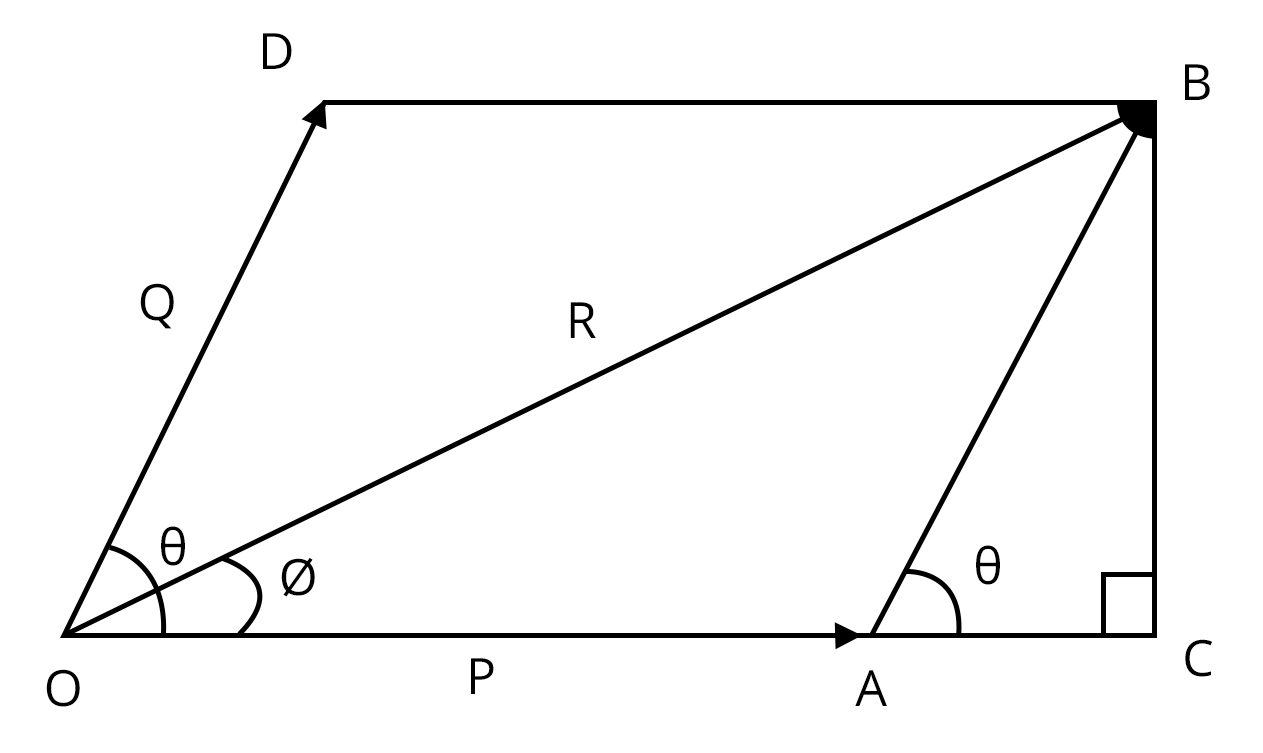
Addition and Subtraction of Vectors:
Vectors can be added or subtracted by the parallelogram law. To add two vectors, draw them from a common point. The resultant vector is the diagonal of the parallelogram that is formed by the two vectors. To subtract two vectors, draw them from the same point. The resultant vector is the vector that points from the head of the first vector to the head of the second vector.
Zero vector:
The zero vector is a vector that has a magnitude of zero. It is represented by the symbol 0 . The zero vector can be added to any vector without changing the direction or magnitude of the vector.
Scalar and Vector Products:
The scalar product of two vectors is a scalar quantity that is equal to the product of the magnitudes of the two vectors and the cosine of the angle between them. The vector product of two vectors is a vector quantity that is perpendicular to both of the given vectors.
Unit Vector:
A unit vector is a vector that has a magnitude of one. It is used to represent the direction of a vector. To find the unit vector of a vector v , divide v by its magnitude:
$\hat{v} = \frac{\vec{v}}{\|\vec{v}\|}$
Resolution of a Vector:
The resolution of a vector is the process of breaking it down into its components along two or more axes. For example, a vector in two dimensions can be resolved into its components along the x-axis and the y-axis.
Kinematics Examples for JEE Main
Kinematics examples for JEE Main cover various scenarios involving the motion of objects, focusing on displacement, velocity, acceleration, and equations of motion. Some examples include:
Free Fall: Calculating the time taken by an object to reach the ground from a certain height or determining its final velocity upon impact.
Projectile Motion: Analyzing the trajectory, range, maximum height, and time of flight of a projectile launched at an angle.
Uniform Circular Motion: Deriving expressions for centripetal acceleration, tangential velocity, and total acceleration in circular motion.
Relative Motion: Solving problems involving the motion of two objects in different frames of reference, calculating their relative velocity and displacement.
Motion in One Dimension: Using equations of motion to determine unknown quantities such as displacement, initial velocity, final velocity, time, and acceleration.
Motion in Two Dimensions: Solving problems involving motion along both x and y axes, considering independent horizontal and vertical components of motion.
Graphical Analysis: Interpreting and analyzing position-time, velocity-time, and acceleration-time graphs to extract information about an object's motion.
Elastic and Inelastic Collisions: Applying conservation laws to solve collision problems, determining velocities and directions of objects after collisions.
Velocity and Acceleration Calculations: Calculating instantaneous and average velocities, acceleration due to gravity, and angular acceleration.
Motion under Varying Acceleration: Solving problems where acceleration changes with time, applying calculus concepts to derive equations for varying acceleration.
List of Important Formulae
JEE Main Kinematics Solved Examples
1. A balloon carrying a stone rises from rest on the ground with an acceleration of 10 m/s2 in the upward direction. After 5 sec, the stone is released and ultimately reaches the ground. Find the maximum height attained by the height.
Ans: Here, we will take the upward direction as positive and the downward direction as negative. Given the balloon rises up from rest. Therefore,
The initial velocity of the balloon, u1 = 0 m/s
Acceleration of the ball, a = 10 m/s2
First of all, let us find the height reached by the balloon in 5 seconds before the stone is released using the equation of motion.
$s=ut+\dfrac{1}{2}at^{2}$
Substitute the value of each term in the equation to calculate the height reached by the balloon.
$S=0\times 5+\dfrac{1}{2}\times 10\times 5^{2}$
$S= 125 m$
Now, even after the stone is released, it will continue to move upward for some time because it has some velocity in the upward direction. Then, the initial velocity of the stone is equal to the final velocity of the balloon after 5 sec. So, let us calculate the final velocity of the balloon using the formula given below:
$v=u+at$
Substitute the values and calculate the final velocity.
$v=0+10\times 5$
$v= 50 m/s$
Now, calculate the distance covered by the stone to reach the maximum height where the final velocity is zero by using the formula given below. (Take acceleration due to gravity as -10 m/s2 since it is in the downward direction.)
$v^2=u^2+2as$
$0^2=50^2+2\times (-10)\times$
$20s=2500$
$s=125 m$
Therefore, the maximum height attained by the stone is the sum of the distance travelled by the balloon in 5 sec and the distance travelled by the stone after it is released, i.e, 125 m + 125 m = 250 m
Key Point: The velocity of any body thrown upward will be zero when it reaches its highest point but the acceleration will be equal to the acceleration due to gravity acting downwards.
2. An aeroplane moving at a speed of 180 km/hr drops a food packet while flying at a height of 500 m. Find the horizontal range?
Ans: We will consider the motion along with vertical and horizontal directions separately to apply equations of motion.
First, find the time taken by the packet to reach the ground considering motion along the vertical direction.

The vertical displacement of the packet, Sy = h = 500 m
Initial velocity along vertical direction, uy = 0 m/s
Acceleration of the packet along vertical direction = g = 10 m/s2
$s_y=u_yt+\dfrac{1}{2}a_yt^{2}$
$S_y=u_yt+\dfrac{1}{2}a_yt^{2}$
$500 =0\times t+\dfrac{1}{2}\times 10t^{2}$
$1000=10t^{2}$
$t^2=100$
$t=10s$
Now consider the motion along horizontal direction and calculate the horizontal range using the equation of motion.
Initial velocity along the horizontal direction, ux = 180 km/hr = $180\times \dfrac{5}{18}$ = 50 m/s
Acceleration along the horizontal direction, ax = 0 m/s2
Time taken to reach the ground, t = 10s
$S_x=u_xt+\dfrac{1}{2}a_xt^{2}$
$S=50\times10+\dfrac{1}{2}\times 0\times 10^{2}$
$S_x= 500 m$
Key Point: Whenever dealing with the problems related to the two-dimensional motion of a body under gravity, consider the motion along with vertical and horizontal directions separately to apply equations of motion.
Previous Year Questions from JEE Exam
1. Two guns A and B can fire bullets at speeds 1 km/s and 2 km/s respectively. From a point on horizontal ground, they are fired in all possible directions. The ratio of maximum areas covered by bullets fired by two guns on the ground is… (JEE 2019)
Ans: For a projectile motion, maximum range is attained at an angle of 45o. The area covered by the bullets will be equal to the area of the circle with a radius equal to the maximum range of each bullet.
Bullets fired by gun A:
Let RA be the maximum range of the bullets fired by gun A at an angle of 45o.
The velocity of the bullets fired by gun A, uA = 1 km/s
The maximum range of the bullet from gun A is given by
$R_A=\dfrac{u_A^2\sin(2\theta)}{g}$
$R_A=\dfrac{u_A^2\sin(2\times45)}{g}$
$R_A=\dfrac{u_A^2}{g}$
Maximum area covered by the bullets from gun A is
$Area_A=\pi R_A^2$
$Area_A=\pi\left( \dfrac{u_A^4}{g^2}\right) $
Similarly,
The velocity of the bullets fired by gun B, uB = 2 km/s
The maximum range of the bullet from gun B is given by
$R_B=\dfrac{u_B^2}{g}$
Maximum area covered by the bullets from gun B is
$Area_B=\pi R_B^2$
$Area_B=\pi\left( \dfrac{u_B^4}{g^2}\right) $
Then the ratio of two area covered by the bullets fired by gun A and gun B is,
$\dfrac{Area_A}{Area_B}=\dfrac{\pi\left( \dfrac{u_A^4}{g^2}\right)}{\pi\left( \dfrac{u_B^4}{g^2}\right)}$
$\dfrac{Area_A}{Area_B}=\dfrac{u_A^4}{u_B^4}=\dfrac{1^4}{2^4}=\dfrac{1}{16} $ Therefore, the ratio of area covered by the bullets A and B is 1:16.
Key Point: The maximum range is attained by a given projectile for a given velocity at an angle of 450.
2. A stone is dropped from the top of a building. When it crosses a point 5m below the top, another stone starts to fall from a point 25m below the top, both stones reach the bottom of the building simultaneously. The height of the building is: (Take g = 10 m/s2) (JEE 2021 Feb)
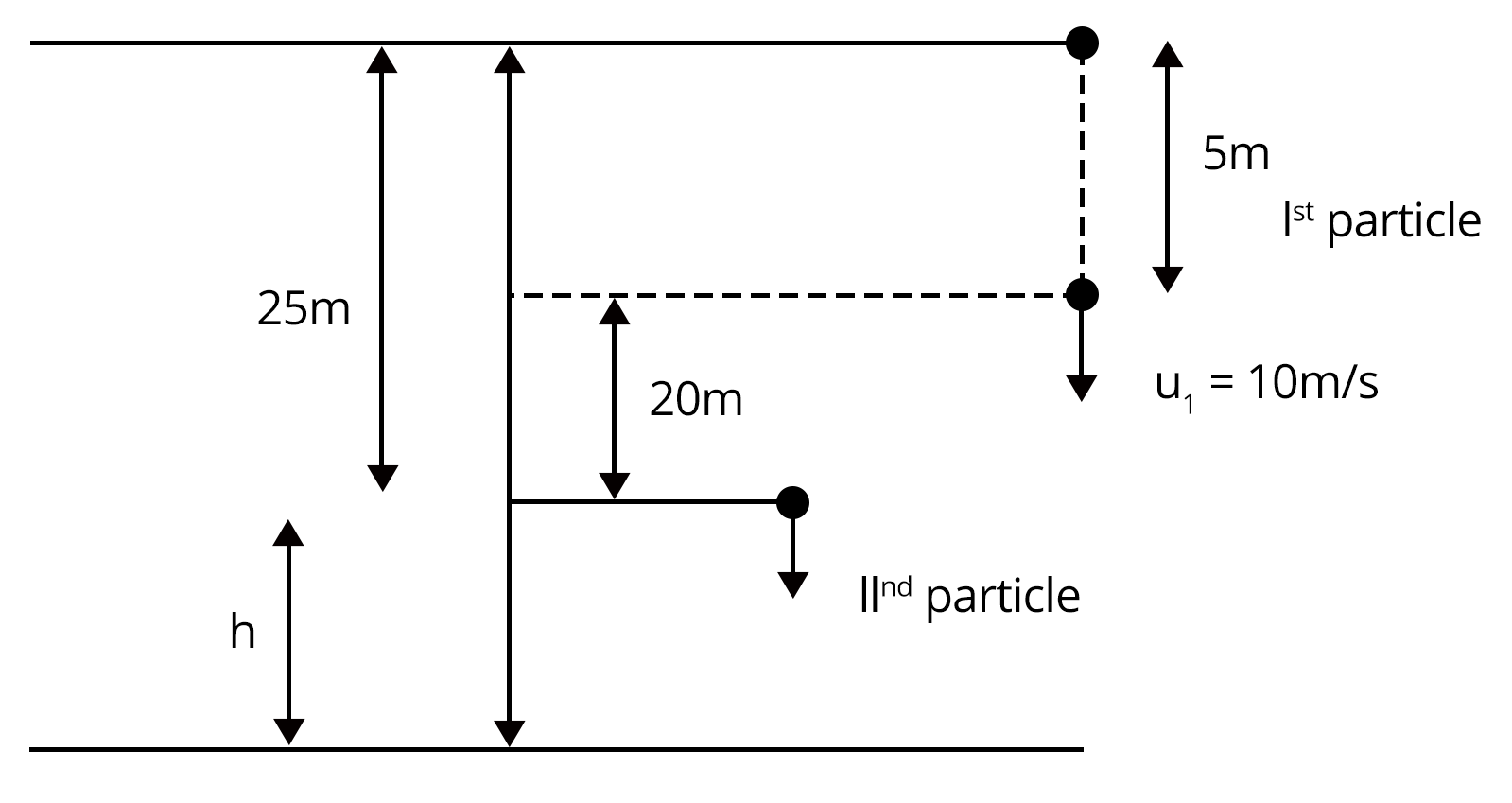
Ans: First, we have to calculate the velocity of the first stone (u1) at a height 5 m below the top of the building using the formula given below.
$u_1=\sqrt {2gh}$
$u_1=\sqrt {2\times 10\times 5}=10m/s$
Now, apply the 2nd equation of motion on the first stone at a point 5 m below from the top till it reaches the ground. Then the displacement covered by the first stone is 20+h.
$20+h=u_1t+\dfrac{1}{2}gt^{2}$
$20+h=10t+\dfrac{1}{2}gt^{2}$.....(1)
For the second stone, the displacement covered to reach the ground is h and the time taken is t. Using the 2nd equation of motion,
$20+h=10t+\dfrac{1}{2}gt^{2}$.....(2)
Put equation (2) in equation (1) to calculate the time t
$20+\dfrac{1}{2}gt^{2}=10t+\dfrac{1}{2}gt^{2}$
t=2s
Now, put t=2s in equation (2) to calculate h
$h=\dfrac{1}{2}\times 10\times 2^{2}=20 m$
The total height of the building = h + 25= 20 + 25 = 45 m
Key Point: Whenever an object is dropped or released, the initial velocity must be taken as zero.
Practice Questions
A body is projected at 600 with the ground. It covers a horizontal distance of 100 m. If the same body is projected at 600 with vertical with the same velocity, what will be the new range? (Ans: 100 m)
A car moving with a speed of 50 km/hr, can be stopped by brakes after at least 6m. If the same car is moving at a speed of 100 km/hr, what will be the minimum stopping distance? (Ans: 24 m)
JEE Main Physics Kinematics Study Materials
Here, you'll find a comprehensive collection of study resources for Kinematics designed to help you excel in your JEE Main preparation. These materials cover various topics, providing you with a range of valuable content to support your studies. Simply click on the links below to access the study materials of Kinematics and enhance your preparation for this challenging exam.
JEE Main Physics Chapters 2025
JEE Main Physics Study and Practice Materials
Explore an array of resources in the JEE Main Physics Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Main exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Physics preparation.
Conclusion
The chapter on Kinematics is a vital component of JEE Main, serving as the foundation for understanding motion and its intricacies. It equips students with the tools to analyse and interpret the dynamics of objects, whether in classical mechanics or in the realm of modern physics. A profound grasp of kinematic principles not only paves the way for excelling in JEE Main but also lays the groundwork for comprehending more advanced physics concepts. The ability to unravel the mysteries of motion and translate them into mathematical equations is not just beneficial for succeeding in exams, but it also underpins innovations in engineering, technology, and scientific research.
Important Links for JEE Main and Advanced 2025
Access a curated collection of study materials and tips to excel in JEE Main and Advanced 2025, helping you prepare effectively for these prestigious engineering entrance exams.
JEE Main Physics Chapter Kinematics for JEE Main 2025

 Share
ShareFAQs on JEE Main Physics Chapter Kinematics for JEE Main 2025
1. How many questions are asked from Kinematics in JEE Main?
Mostly, 1-2 questions from this chapter are asked in JEE Main every year which corresponds to around 8 marks in the JEE Main exam.
2. What is the importance of kinematics in the JEE exam?
Compared to other chapters, kinematics concepts will become easier for you once you start practicing more and more questions related to it. You can easily solve all the types of kinematics problems asked from this chapter once you learn all the topics covered in the chapter conceptually. Besides, the concepts discussed in this chapter are linked to problems from other chapters too.
3. What is Kinematics?
Kinematics is the branch of physics that studies the motion of objects without considering the forces causing the motion.
4. What is Kinematics Class 11 for JEE Main exam?
Kinematics Class 11 focuses on understanding the motion of objects, including concepts like displacement, velocity, acceleration, and equations of motion.
5. What is the Kinematics formula?
Some key Kinematics formulas include:
$v = u + at$
$s = ut + \frac{1}{2}at^2$
$v^2 = u^2 + 2as$
where u is initial velocity, v is final velocity, aaa is acceleration, t is time, and s is displacement.
6. Can you provide a Kinematics definition with example?
Kinematics is the study of motion in terms of position, velocity, and acceleration without considering forces. For example, calculating the time it takes for a car to stop when brakes are applied is a Kinematics problem.
7. What are the key topics in Kinematics Class 11 for JEE Main?
Key topics in Kinematics Class 11 include motion in a straight line, motion in a plane, displacement, velocity, acceleration, and the equations of motion.
8. Why is Kinematics important in physics?
Kinematics provides the foundation for understanding motion, which is essential for solving problems in mechanics and other areas of physics.
9. How do you apply Kinematics formulas?
Kinematics formulas are used to calculate unknown quantities like displacement, velocity, or time when given sufficient initial conditions.
8. What is the difference between Kinematics and Dynamics?
Kinematics deals with the description of motion, while Dynamics focuses on the forces causing the motion.
10. How does Kinematics relate to real-life scenarios?
Kinematics is used to analyse motions like a ball being thrown, a car accelerating, or a satellite orbiting a planet.
11. What is the best way to learn Kinematics Class 11 for JEE Main?
The best way to learn Kinematics Class 11 is by understanding the concepts, practising numerical problems, and applying formulas to real-world situations.














 Watch Video
Watch Video