Current Electricity Chapter - Physics JEE Main

Current Electricity is a crucial chapter in the JEE Main syllabus, and it's all about understanding how electric currents work and how they interact with materials. In simple terms, it's like learning how electricity flows in wires and devices. This chapter is fundamental as it forms the basis for a multitude of advanced electrical and electronic concepts.

In this article, explore various aspects of Current Electricity, including key concepts like Electric Current (the flow of electricity), Ohm's Law (which explains the relationship between voltage and current), Electrical Resistance (how materials slow down electric flow), and Drift Velocity (how fast electrons move). We will also discuss different materials' resistances, Ohmic and non-Ohmic conductors, and the basics of Electrical Energy and Power. Understanding electrical resistivity, the color code for resistors, and how resistors can be combined in series and parallel is also on our list. Plus, we will delve into the temperature effects on resistance.

JEE Main Physics Chapters 2024  

Current Electricity Important Concept for JEE Main

Concepts	Key Points of the Concepts
Electric current density and drift velocity	Electric current is the rate of flow of charge per unit time given by the current electricity formula, $\lim_{\Delta t \rightarrow 0}\dfrac {\Delta q}{\Delta t}$ Electric current density (J) is a vector quantity  and is equal to electric current per unit area. $J=\dfrac{I}{A}$ Drift velocity is the velocity acquired by the electron due to an applied electric field. The relation between current density (J) and drift velocity is  $J=nev_d$ Where n is the number of charge carriers per unit volume. Relaxation time (τ) is the time taken by electrons between two successive collisions.
Ohm’s law and temperature depend on resistivity.	Ohm’s law states that the current passing through a conductor is directly proportional to the potential difference across it at a constant temperature. The formula for resistance is given by ohm’s law. $R=\dfrac{V}{I}$ Resistivity (𝜌) is the reciprocal of conductivity (𝜎) and both depend on the materials and temperature only. The unit of resistivity is Ωm and the unit of conductivity is S/m (siemen per metre) $\rho=\dfrac{1}{\sigma}$  Resistivity increases with temperature. Note: Ohm’s Law and Resistance are used in a wide variety of applications, such as electronics, circuits, and power systems. Having a good understanding of it can help you to solve problems and design circuits more effectively.
Electrical Resistance	Resistance, 'R,' quantifies the opposition a material offers to the flow of electric current. It is determined by the material's properties and geometry. The formula for resistance is: R = ρ * (L/A) Where: 'R' is resistance. 'ρ' is the electrical resistivity of the material. 'L' is the length of the conductor. 'A' is the cross-sectional area.
Resistances of Different Materials	Different materials have varying electrical resistivities. For example, metals typically have low resistivity, while insulators have high resistivity. Conductors with low resistivity allow for efficient current flow.
V-I Characteristics	Ohmic Conductors: In Ohmic conductors, like most metals, the V-I graph is a straight line, indicating a linear relationship between voltage and current. Ohm's Law holds true for such materials. Non-Ohmic Conductors: Non-Ohmic conductors, like diodes and semiconductors, do not follow Ohm's Law. Their V-I characteristics are nonlinear, and the resistance varies with voltage and current.
Electrical Energy and Power	Electrical energy, 'E,' is calculated using the formula: E = VIt Where: 'V' is the voltage. 'I' is the current. 't' is the time. Electrical power, 'P,' is the rate at which electrical energy is consumed or produced and is calculated as: P = VI
Electrical Resistivity	Electrical resistivity ('ρ') is a material property that quantifies its resistance to the flow of electric current. It is measured in Ohm-meter (Ω·m) and varies from one material to another.
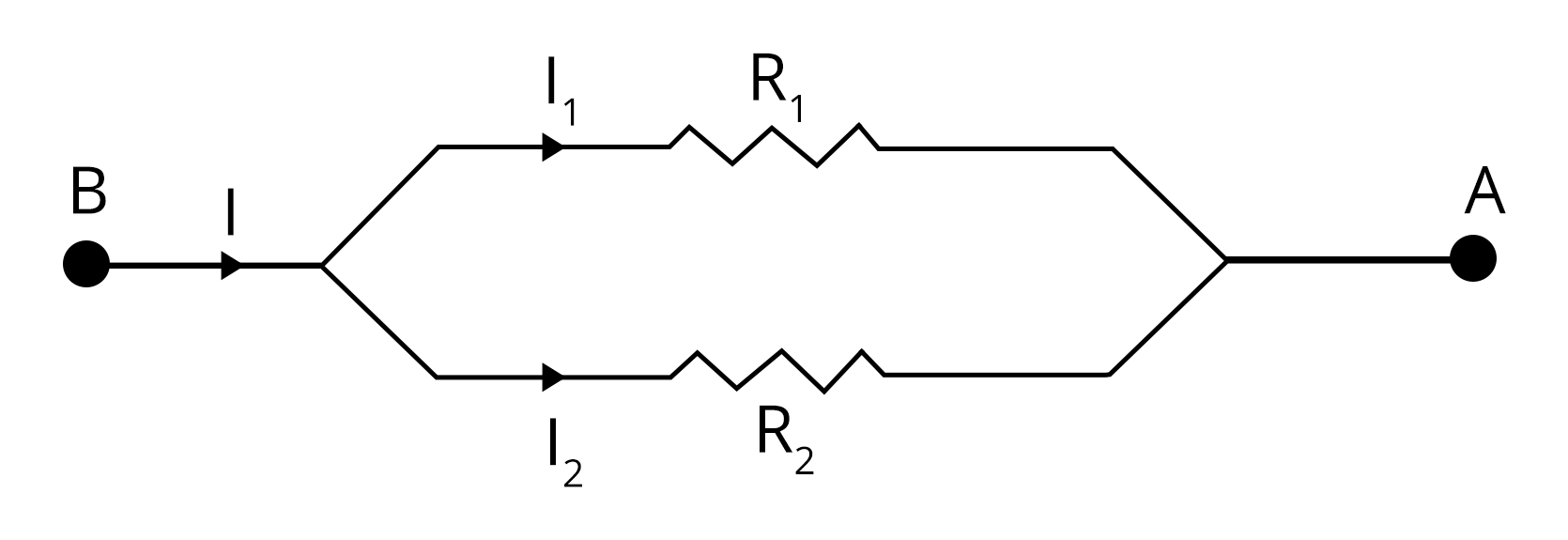
Grouping of Resistors	For resistors in series connection, the current through the resistors is the same. (Image Will Be Updated Soon) $R_{eq}=R_1+R_2+R_3$ (Image Will Be Updated Soon) For resistors in parallel combination, the potential difference across each resistor is the same. (Image Will Be Updated Soon) $\dfrac{1}{R_{eq}}=\dfrac{1}{R_1}+\dfrac{1}{R_2}+\dfrac{1}{R_3}$ (Image Will Be Updated Soon)
Colour code of carbon resistor	The resistance value of carbon resistors is marked on it using colour bands. Each colour denotes a digit from 0 to 9. The first two colour bands represent the first two digits and the third band represents the multiplier of 10. The fourth band represents the tolerance level. The numerical digit of each colour is given below Black - 0 Brown - 1 Red - 2 Orange - 3 Yellow - 4 Green - 5 Blue - 6 Violet - 7 Grey - 8 White - 9 Gold - 5% tolerance Silver - 10 %
Grouping of cells	Electric Cell: An electric cell is a device that converts chemical energy into electrical energy. It consists of two electrodes immersed in an electrolyte. The two main types of cells are the primary cell (non-rechargeable) and the secondary cell (rechargeable). Internal resistance(r) is the resistance offered within the cell or battery against the current. When cells are connected in a series combination in such a way that the positive terminal is connected to the negative terminal of another cell, the net emf of the combination of cells is given by  $\varepsilon_{eq}=\varepsilon_1+\varepsilon_2+\varepsilon_3$ The effective internal resistance has to be calculated based on the formula of equivalent resistance in series combination. $r_{eq}=r_1+r_2+r_3$ When cells are connected in parallel combination, the effective emf of the combination of cells and effective internal resistance is given by the formula $\dfrac{\varepsilon_{eq}}{r_{eq}}=\dfrac{\varepsilon_1}{r_1}+\dfrac{\varepsilon_2}{r_2}+\dfrac{\varepsilon_3}{r_3}$ $\dfrac{1}{r_{eq}}=\dfrac{1}{r_1}+\dfrac{1}{r_2}+\dfrac{1}{r_3}$
Kirchhoff’s Law	Kirchhoff’s current law is based on the law of conservation of charge. According to the law, the current entering and leaving a junction are equal (Image Will Be Updated Soon) $I_1+I_2+I_3=I_4+I_5$ Kirchhoff’s voltage law is based on the law of conservation of energy. It states that the algebraic sum of voltage within a loop is always equal to zero. (Image Will Be Updated Soon) $V_{AB}+V_{BC}+V_{CD}++V_{DA}=0$ Know more about Kirchhoff's Laws of Electric Circuits and how they can be used to analyze and design electrical circuits.
Wheatstone bridge	A galvanometer is connected across B and D and a battery is connected across A and C of a Wheatstone bridge. (Image Will Be Updated Soon) A Wheatstone bridge is said to be balanced if no current passes through the galvanometer and the condition for the Wheatstone bridge to get balanced is given by  $\dfrac{R_2}{R_{1}}=\dfrac{R_4}{R_3}$
Metre bridge	Metre bridge uses the principle of the Wheatstone bridge to calculate the unknown resistance. (Image Will Be Updated Soon) By knowing the balance length (l) when the current through galvanometer is used, we can find the value of the unknown resistance using the formula given by $\dfrac{R}{S}=\dfrac{l}{100-l}$
Potentiometer	Potentiometer is a device that measures the potential difference across two points or emf of a cell without drawing current from it. The potentiometer is more accurate than a voltmeter. The potentiometer is used to compare the emf of two cells and the circuit diagram is given below. The balancing length (l1) for the cell having emf E1 is obtained when switch s1 is only closed and the balancing length (l2) for the cell having emf E2 is obtained when switch s2 is only closed. The ratio of the emfs of the two cells is given by the formula $\dfrac{E_1}{E_2}=\dfrac{l_1}{l_2}$ The internal resistance of a cell can be calculated using potential. The experimental set-up is given below. The balancing length (l1) is obtained when the switch S is open and balancing length (l2) is obtained when the switch S is closed. The formula for calculating the internal resistance (r) is given by $r=\left (\dfrac{l_1}{l_2}-1\right )R$

Ohm’s Law Formula

Types of Electric Current

1.  Alternating current (AC) — In its systems, the movement of electric charge reverses direction periodically. AC is the form of electric power that is most commonly delivered to businesses and residences. Audio and radio signals that are carried on electrical wires are some examples of AC. 

2. Direct current (DC) — It is the unidirectional flow of electric charge and a system in which electric charge moves in one direction only. Sources of direct current are batteries, thermocouples, solar cells, and commutator-type electric machines of the dynamo type. DC flows in a conductor like wire, but can also flow through semiconductors, insulators, or a vacuum as in electron or ion beams. 

Relation Between Resistance and Resistivity

Resistance: To obstruct the flow of electric current is called the resistance.

Let’s say we have a conductor, and we apply a potential across its ends.

Then the resistance of this material is,

R = V/I..(1)

So on applying the potential difference, ΔV across the ends of the conductor, if the current I flow through it, then the ratio of V/I is the resistance.

This relationship isn’t for Ohm’s law because this law states that V ∝ I or V = IR 

Where R is constant, but resistance can be variable.

So for the devices having a variable resistance, we can consider the potential difference at an instant and use the above equation (1):

Therefore, Ohm’s law isn’t valid everywhere.

We know that:

1. R ∝ 1/L

2. R ∝ A

On removing the constant of proportionality sign, we get:

R = ρ L/A

The proportionality constant, ρ is called the specific resistance or the electrical resistivity of a material which is measured in Ohm-m or Ω m.

What is Conventions?

In a conductive object, the moving charged particles that constitute the electric current are known as charge carriers. In metals, the positively charged atomic nuclei of the atoms are held in a fixed position whereas the negatively charged electrons that are charge carriers that are free to move in the metal. In semiconductors, the charge carriers can be positive or negative, depending on the dopant used. A flow of positive charge gives the electric current and has an effect in a circuit the same as an equal flow of negative charges in the opposite direction. 

Measurement of Current 

Current can be measured using an ammeter. It’s also measured by detecting the magnetic field associated with the current. 

Different Techniques to Measure Current are:

• Shunt resistors

• Hall effect current sensor transducers

• Transformers

• Magnetoresistive field sensors

• Rogowski coils

• Current clamps

Resistive Heating

Joule heating or resistive heating is a process of power dissipation through which the passage of an electric current through a conductor increases the internal energy of the conductor, converting thermodynamic work into heat. James Prescott Joule, the inventor of this phenomenon, immersed a length of wire in a fixed mass of water and measured the temperature rise due to a known current through the wire for 30 minutes. By varying the current and length of the wire, he deduced that the heat produced was proportional to the square of the current multiplied by the electrical resistance of the wire.

P ∝ I²R

This relationship is known as Joule's Law. The SI unit of energy was named joule and given the symbol J. The SI unit of power, watt (W), is equivalent to one joule per second.

List of Important Formulas for Current Electricity Chapter

Sl. No	Name of the Concept	Formula
	Relation between current density(J) and conductivity(𝜎)	$\vec J=\sigma \vec E$ Where E is the electric field
	Relation between resistivity(𝜌) and relaxation time (𝜏)	$\rho=\dfrac{m}{ne^2\tau}$ Where m is the mass of an electron, e is charge of an electron and n is the charge carrier density
.	Resistance(R) and conductance (C)	$R=\dfrac{1}{C}t$
	Resistance of conductor having length (l) and area (A)	$R=\rho\dfrac{l}{A}$
	Temperature dependance of resistivity	$\rho_2 =\rho_1(1+\alpha\Delta T)$ Where ΔT is the change in temperature and  ⍺ is the temperature coefficient of resistivity
	Heat energy(H) when a current (I) is passing through a resistor (R)	$H=I^2Rt$ $H=\dfrac{V^2}{R}t$ $H=IVt$ Where t is the time of flow of current (I).
j	Power delivered (P) when a current (I) is passing through a resistor (R)	$P=I^2R$ $P=\dfrac{V^2}{R}$ $P=IV$
	Current through resistors in parallel	$I_1=I\left(\dfrac{R_2}{R_1+R_2}\right)$ $I_2=I\left(\dfrac{R_1}{R_1+R_2}\right)$
	Potential difference across resistors in series	(Image Will Be Updated Soon) $V_1=V\left(\dfrac{R_1}{R_1+R_2+R_3}\right)$ $V_2=V\left(\dfrac{R_2}{R_1+R_2+R_3}\right)$ $V_3=V\left(\dfrac{R_3}{R_1+R_2+R_3}\right)$

JEE Main Current Electricity Solved Examples 

1. The internal resistance of a cell of emf 2V is 0.1 Ω. It is connected to a resistance of 3.9 Ω. Find the voltage across the cell.

Sol:

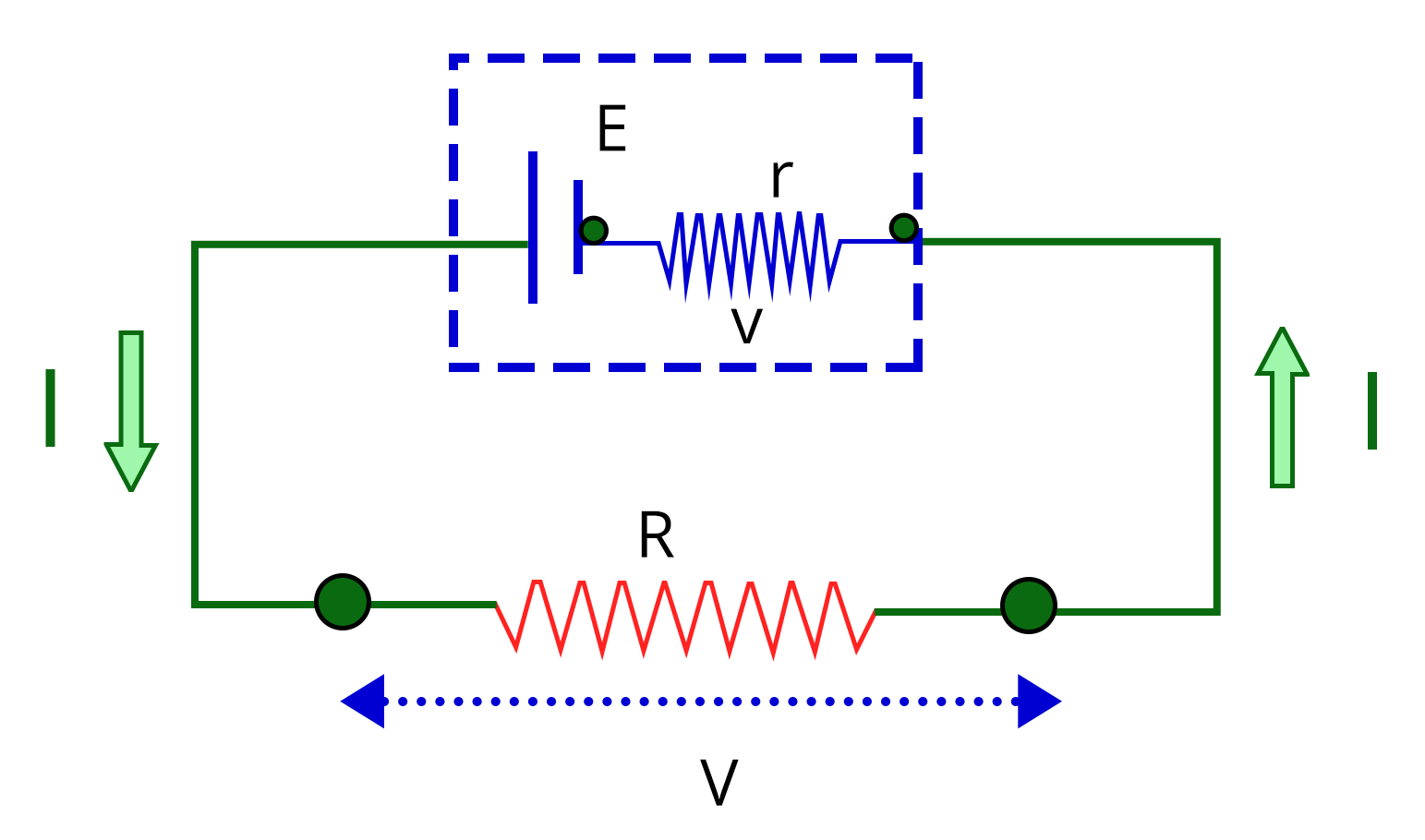
Given:

Emf of the cell, E = 2V

Internal resistance of the cell, r = 0.1 Ω

Resistance connected to the cell, R = 3.9 Ω

Let V be the terminal voltage across the cell.

We know that the voltage drop across the resistor, 

$V=IR$....(1)

Applying Kirchhoff's voltage law,

$E-V-Ir=0$

$E-IR-Ir=0$

$2V-3.9I-0.1I=0$

$I=\dfrac{2}{4}=0.5~A$

Substitute the value of current I in equation (1) to obtain the voltage across the cell V.

$V=0.5\times 3.9=1.95~V$

Therefore, the voltage across the cell is 1.95 V

Key point: Since resistor R and the cell are in parallel connection, the voltage across them are equal.

2. The resistance of a wire is R ohm. If it is melted and stretched to n times its original length, its new resistance will be_________.

Sol:

Given:

The resistance of the wire is R. 

Let the length and area of the cross-section of wire be l and A, respectively and the resistivity of the wire be 𝜌. 

If the length of the wire is stretched to n times, then the area of the cross-section of the wire is reduced by n times.

Let the length and area of the cross-section of the stretched wire be l’ and A’, respectively.

The resistance of the stretched wire is given by

$R'=\rho\dfrac{l'}{A'}$

$R'=\rho\dfrac{(nl)}{\left(\dfrac{A}{n}\right)}$

$R'=\rho\dfrac{n^2l}{A}$

$R'=n^2\times\left(\rho\dfrac{l}{A}\right)$

$R'=n^2R$

Therefore, the resistance of the stretched wire is n2 times the initial resistance.

Key point: When a wire is stretched, its length increases and the area of cross-section decreases while keeping the volume constant.

Previous Years’ Questions from JEE Paper

1. Five equal resistances are connected in a network as shown in the figure. The net resistance between points A and B is______. (Feb -2021)

a. 3R/2

b. R/2

c. R

d. 2R

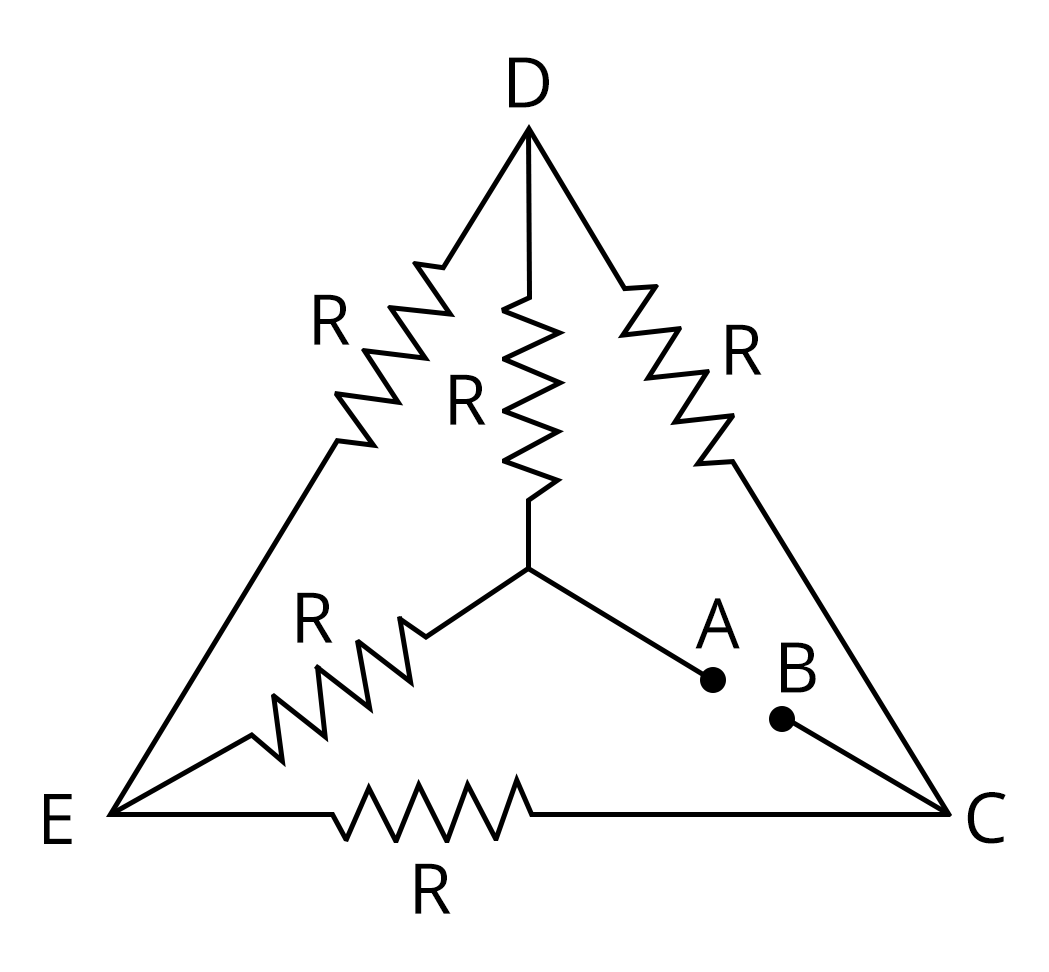
Sol:

If you observe the diagram closely, you can see that the above circuit diagram is a balanced Wheatstone bridge since all the resistances are the same. Therefore, it satisfies the condition

$\dfrac{R_2}{R_{1}}=\dfrac{R_4}{R_3}$

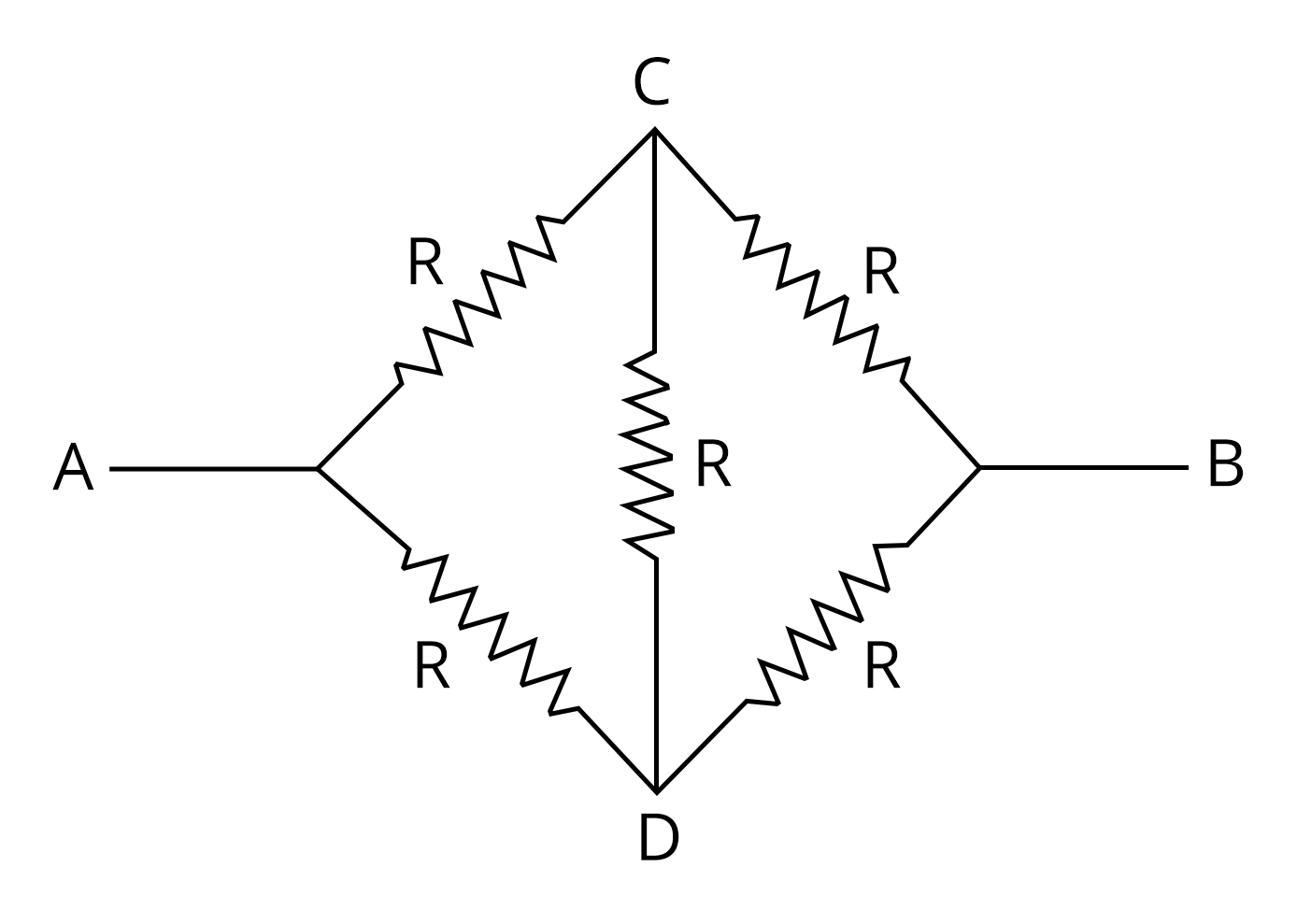
Since it is balanced, the current does not pass through the resistor in the bridge and can be removed while calculating the effective resistance.

The two 2R resistors are in parallel, so the equivalent resistance between A and B is

$R_{eq}=\dfrac{2R\times 2R}{2R+2R}$

$R_{eq}=\dfrac{4R^2}{4R}$

$R_{eq}=R$

Therefore, the equivalent resistance between A and B is R and the correct answer is option C.

Key point to remember: When a difficult circuit diagram having resistors are given, always look for the possibility of a balanced Wheatstone bridge.

2. The length of a potentiometer wire is 1200 𝑐𝑚 and it carries a current of 60 𝑚𝐴. For a cell of emf 5 𝑉 and internal resistance of 20Ω, the null point on it is found to be at 1000 𝑐𝑚. The resistance of the whole wire is_____. (JEE 2020)

a. 80Ω

b. 100Ω

c. 120Ω

d. 60Ω

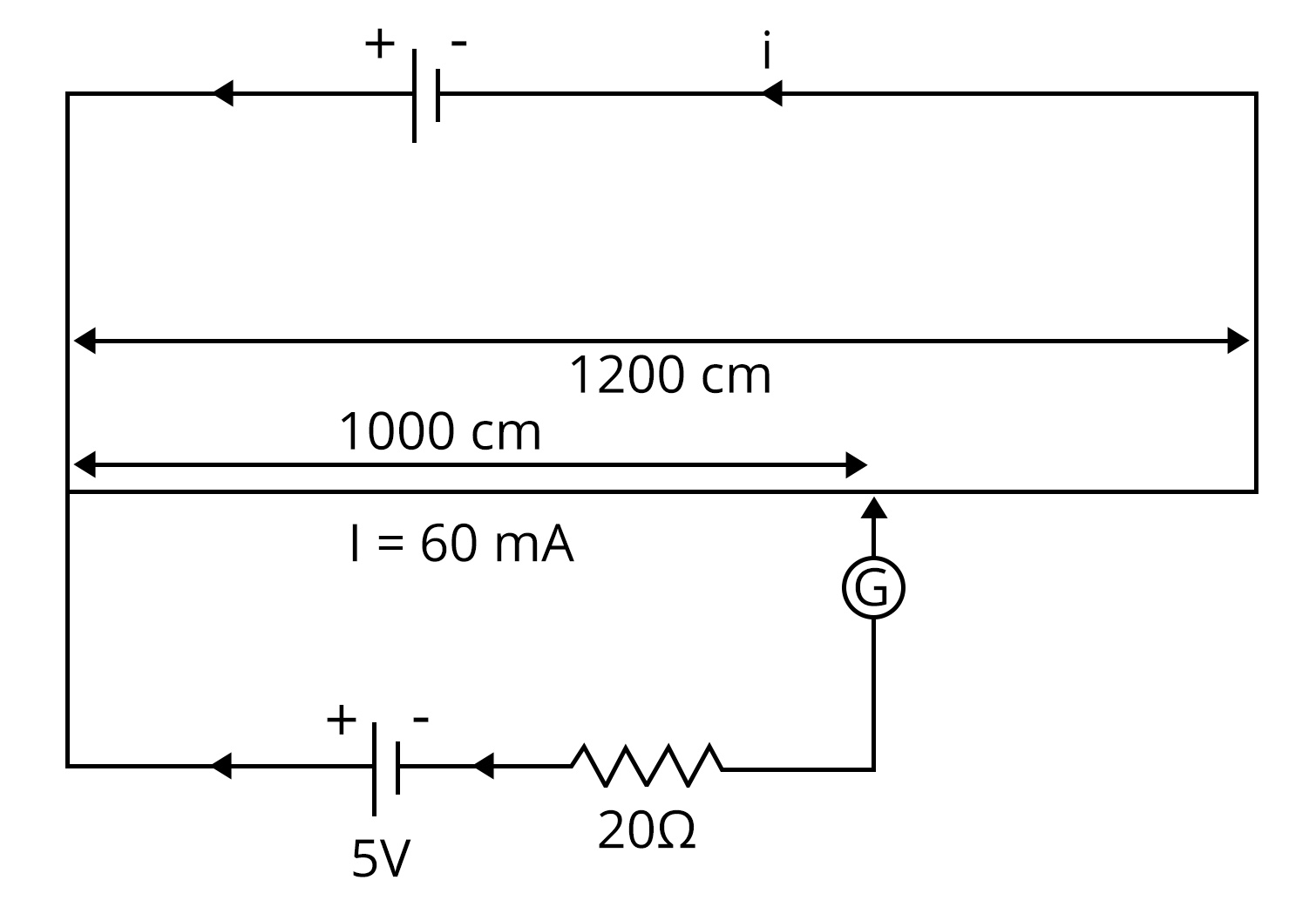
Sol: 

Given:

The null point of the potentiometer = 1000 cm

The current through the potentiometer wire, I = 60 mA

Emf of the cell in the secondary circuit, E = 5 V

At the null point, no current passes through the galvanometer and hence no current is drawn from the cell. Therefore, the emf of the cell is equal to the potential difference across the section of the wire having a length of 1000 cm.

So, the resistance of the wire of 1000 cm (R1) can be calculated.

$R_1=\dfrac{E}{I}$

$R_1=\dfrac{5~V}{60~\text{mA}}$

$R_1=83.33~ \Omega $

Let the resistance of the wire per unit length be r.

$r=\dfrac{83.33~ \Omega}{1000~\text{cm} }$

$r=83.33\times 10^{-3}~ \Omega/cm$

Now, the resistance of the 1200 cm potentiometer wire (R) is obtained by

$R=83.33\times 10^{-3}~ \Omega/cm~\times 1200~\text{cm}$

$R=100~\Omega$

Therefore, the correct answer is option B.

Key point to remember: At the balancing point, no current is drawn from the cell and hence the terminal voltage and emf are equal.

Practice Questions

1. A uniform wire of resistance 9 Ω is cut into three equal parts. They are connected in the form of an equilateral  triangle ABC. A cell of emf 2V and negligible internal  resistance is connected across B and C. Potential difference across AB is____. (Ans: 1 V)

2. A filament bulb (500 W, 100V) is to be used in a 230 V main supply. When a resistance R is connected in series, it works perfectly and consumes 500 W. The value of R is_______. (Ans: 26 Ω)

JEE Main Physics Current Electricity Study Materials

Here, you'll find a comprehensive collection of study resources for Current Electricity designed to help you excel in your JEE Main preparation. These materials cover various topics, providing you with a range of valuable content to support your studies. Simply click on the links below to access the study materials of Current Electricity and enhance your preparation for this challenging exam.

JEE Main Physics Study and Practice Materials

Explore an array of resources in the JEE Main Physics Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Main exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Physics preparation.

Benefits of Using Vedantu for JEE Main Physics 2024 - Current Electricity

Embark on a dynamic journey through the realm of Current Electricity for JEE Main 2024 with Vedantu. Experience interactive learning, expert guidance, and personalized study plans, ensuring a comprehensive understanding, effective problem-solving skills, and thorough preparation for the exam.

1. Interactive Learning with a Focus on Current Electricity: Vedantu's platform for JEE Main Physics ensures interactive and engaging learning, specifically focusing on the challenging concepts of Current Electricity.

2. Expert Educators Specializing in Current Electricity: Benefit from the expertise of seasoned educators who specialise in teaching Current Electricity, ensuring students gain a comprehensive understanding of this topic.

3. Live Classes for Real-time Doubt Resolution: Attend live classes dedicated to Current Electricity, providing real-time interaction with teachers for doubt resolution and clarifications, enhancing understanding through active engagement.

4. Tailored Learning Plans for Current Electricity: Vedantu's customised learning plans cater to individual needs, allowing students to focus on specific topics within Current Electricity, ensuring a targeted approach for effective learning.

5. Practice Material, Quizzes, and Assignments for Current Electricity: Access a variety of practice materials, mock tests, and study material tailored for Current Electricity, reinforcing understanding and enhancing problem-solving skills with real-life examples.

6. Regular Assessments to Gauge Progress: Engage in regular assessments and tests focused on Current Electricity to gauge progress, identify areas for improvement, and ensure thorough preparation for JEE Main Physics.

7. Flexible Timings Aligned with Current Electricity Learning: Enjoy the flexibility of learning at your own pace, with classes available at times that suit your schedule, making it convenient to delve into the intricacies of Current Electricity.

8. 24/7 Doubt Solving Support for Current Electricity: Vedantu offers continuous doubt-solving support for Current Electricity, ensuring that students receive assistance whenever they encounter challenges or have queries.

9. Comprehensive Coverage of Current Electricity: The JEE Main Physics course on Vedantu ensures thorough coverage of the Current Electricity topic, using relatable examples to prepare students effectively for the exam, making complex concepts more understandable and memorable.

Conclusion

In this guide, we'll dive into the world of Current Electricity, a key topic in JEE Main physics. We'll demystify the core concepts, providing simple explanations and problem-solving strategies. You'll learn about electric circuits, Ohm's law, and how to calculate current and voltage. We've compiled everything you need in one place, including free downloadable PDFs with detailed explanations and practice questions. This resource is a valuable tool to help you ace your exams.