




What is Wheatstone Bridge - Key Concept for JEE Main 2025 Preparation
The Wheatstone Bridge is a key topic in JEE Main 2025, helping students understand the behaviour of electrical circuits. This concept plays a vital role in solving problems related to current, voltage, and resistance. Its applications are widely seen in experiments and practical scenarios, making it an essential part of the syllabus. In this guide, you will explore its working principle, and how it’s applied to solve problems effectively.
What is Wheatstone Bridge?
The Wheatstone bridge is the setup that is used for measuring the unknown resistance. Wheatstone bridge consists of four arms known as resistors and the ratio of the two of these resistors is kept at a fixed value. The remaining two arms are balanced, one of them is an unknown resistor while the other resistance of the other arm can be varied.
The unknown resistance is then computed through the balancing or null condition. The circuit of the Wheatstone Bridge gives a precise measurement of the resistance. The numerous adaptations of the Wheatstone bridge are utilised for the AC circuits. Some of the instruments on the Wheatstone bridge principle are the Carey Foster Bridge, metre bridge, Wien bridge etc.
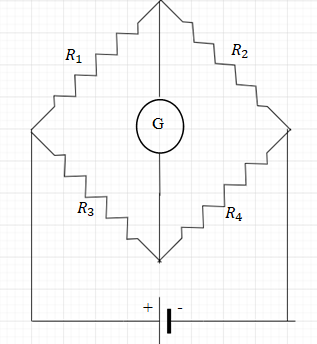
Working on Wheatstone Bridge
Components: The bridge consists of four resistances ($R_1, R_2, R_3, R_x$), a galvanometer, and a voltage source.
Balanced Condition: When the ratio of resistances in one branch equals the ratio in the other, the galvanometer shows zero deflection.
Calculation: Using the balance condition, the unknown resistance can be determined accurately.
Wheatstone Bridge Formula
The formula for a Wheatstone Bridge is derived when the bridge is in a balanced condition. In this state, no current flows through the galvanometer, and the ratio of the resistances in one branch equals the ratio in the other branch. The formula is:
$\dfrac{R_1}{R_2} = \dfrac{R_3}{R_4}$
Where:
$R_1$ and $R_2$ are resistances in one branch.
$R_3$ and $R_4$ are resistances in the other branch.
The Principle of Wheatstone Bridge
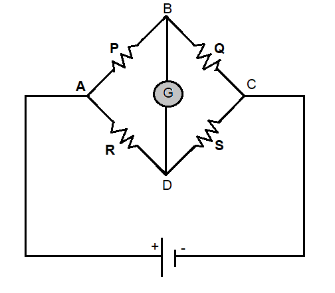
The circuit of the Wheatstone bridge consists of four resistors P, Q, R, and S and they are arranged as the quadrilateral ABCD. Points A and B are connected to battery E through K₁. The B and D points are connected to the galvanometer G via the key K₂. The resistances are selected such that the galvanometer needle doesn’t deflect or the current Ig through it is 0. This is essentially called the balanced condition of a bridge or null condition. For this condition
\[ \frac{P}{Q} = \frac{R}{S} \]
Instead of S, the unknown resistor is connected and resistor R can be varied. Resistors P and Q are also known as ratio arms. You keep a fixed value for the ratio \[ \frac{P}{Q} \] and then R is adjusted to the value where the null condition is met. Unknown resistance is given by the \[S = \frac{Q}{P} R \] .
Wheatstone Bridge Derivation
The current through the galvanometer, at the balanced condition of the bridge, is zero, which is IG = 0. Current through arms AB and BC is denoted by I1 Current through arms of AD as well as DC is I2 As per the circuital law of Kirchhoff, voltage drop through the closed-loop is 0. The application of this law in the ABDA loop results in the dropping of the sum of voltage through the individual arms of the loop which is 0.
\[ I_{1} P - I_{2} R = 0 \]
\[ \frac{I_{1}}{I_{2}} = \frac{R}{P} \]
Applying Kirchhoff’s law to the loop CBDC
\[ I_{1} Q - I_{2} S = 0 \]
\[ \frac{I_{1}}{I_{2}} = \frac{S}{Q}\]
Comparing conditions,
\[ \frac{R}{P} = \frac{S}{Q} \]
\[ \frac{P}{Q} = \frac{R}{S} \]
This is the formula for the Wheatstone Bridge.
Application of Wheatstone Bridge
Resistance measurement via direct application of Ohm’s law can’t be done precisely. In this setup, current and voltage through the unknown resistor must be measured using an ammeter and voltmeter respectively. The ideal ammeter must have zero resistance plus the ideal voltmeter must have infinite resistance. However infinite or zero resistance is impossible and therefore the circuit is unable to give precise measurements. Here the whitestone bridge circuit can be deployed for accurate measurements in such scenarios. For experimental purposes, two adaptations of the Wheatstone bridge circuit are the Metre bridge and the Post office box.
Resistance of some of the materials, such as semiconductors, tends to vary with the temperature. The variations are large in comparison to the ordinary resistors. These are known as thermistors. A slight change in temperatures can be measured using thermistors for the Wheatstone bridge setup.
Changes in the intensity of light are measured via the replacement of an unknown resistor, in the Wheatstone bridge circuit, with a photoresistor. The resistance of the photoresistor is the function of the incident light.
Wheatstone bridge can also be used for measuring strain and pressure.
Limitations of Wheatstone Bridge
Wheatstone bridge is a very sensitive device. The measurements may not be precise in an off-balance condition.
Wheatstone bridge is generally used for measuring resistances ranging from a few ohms to a few kilo-ohms.
The sensitivity of the circuit reduces if the four resistances are not comparable.
Uses of Wheatstone Bridge
Provides accurate and precise measurements.
Easy to set up and use in experiments.
Helps understand the fundamentals of electrical circuits.
Solved Examples
1. The ratio arms of a Wheatstone bridge has resistances equal to 100 \[ \Omega \] and 10\[ \Omega \]. An unknown resistor is connected to the fourth arm. What should be the value of the unknown resistance if the third arm has a resistance of 153 \[ \Omega \] in a balanced condition?
Solution: Resistance of the first arm P=100 \[ \Omega \]
Resistance of the second arm Q=10\[ \Omega \]
Resistance of the third arm R=153\[ \Omega \]
If the unknown resistance is X, the ratio of resistances in the balanced condition If the unknown resistance is X, the ratio of resistances in the balanced condition,
\[\frac{R}{X} = \frac{P}{Q} \]
\[ X = \frac{Q}{P} R\]
Substituting the values,
\[ X = \frac{10}{100} 153 \Omega\]
\[ X = 15.3 \Omega \]
The unknown resistance is 15.3 \[ \Omega \]
2. The four resistances of a Wheatstone bridge are 100\[ \Omega \], 10\[ \Omega \], 300\[ \Omega \], and 30\[ \Omega \]. If the bridge is connected to a 1.5 V battery, what are the currents through individual resistors?
Solution: Resistance of the first arm P=100\[ \Omega \]
Resistance of the second arm Q=10\[ \Omega \]
Resistance of the third arm R=300\[ \Omega \]
Resistance of the fourth arm S=30\[ \Omega \]
The image will be uploaded soon
Points A and C are connected to the battery such that, the potential difference is VAC =1.5V.
The ratios of the arms,
\[ \frac{P}{Q} = \frac{100}{10} = 10 \]
\[ \frac{R}{S} = \frac{300}{30} = 10\]
Therefore, the null condition is satisfied,
\[ \frac{P}{Q} = \frac{R}{S} \].
The current through the galvanometer is zero. The total resistance along the path ABC is R1=P+Q since these two resistances are connected in series. Similarly, total resistance along the path ADC is
\[ R_{2} = R + S \]. The resistance R1 and R2 are connected in a parallel combination between points A and C. Therefore,
Current through P= current through \[Q= I_{1}\]
\[I_{1} = \frac{V_{AB}}{R_{1}} \]
\[= \frac{V_{AB}}{P+Q} \]
\[= \frac{1.5V}{(100 + 10)\Omega} \]
= 0.0136A
Current through R = current through \[S = I_{2}\] where
\[I_{2} = \frac{V_{AB}}{R_{2}} \]
\[\frac{V_{AB}}{R+S}\]
\[\frac{1.5 V}{(300+30)}\Omega\]
= 0.0045A
The current through the 100\[ \Omega \] and 10\[ \Omega \] resistors is 0.0136 A whereas the current through the 300\[ \Omega \] and 30\[ \Omega \] resistors is 0.0045 A.
Did You know?
The Wheatstone bridge circuit was initially invented by Samuel Hunter Christie and later improved by Charles Wheatstone.
Various adaptations of the Wheatstone bridge can be used to measure impedance, inductance, and capacitance in AC circuits.
Maxwell improved the circuit to use AC circuits, which is known as the Maxwell bridge.
Conclusion
The Wheatstone Bridge is an essential concept in physics, helping students master the principles of circuit analysis and resistance calculation. By understanding its workings, formulas, and applications, JEE aspirants can solve related problems with ease. For a deeper understanding, download Vedantu’s FREE PDF on Wheatstone Bridge, which includes step-by-step explanations, solved examples, and practical insights to help you excel in your exams.
JEE Main 2025 Subject-Wise Important Chapters
The JEE Main 2025 subject-wise important chapters provide a focused strategy for Chemistry, Physics, and Maths. These chapters help students prioritise their preparation, ensuring they cover high-weightage topics for better performance in the exam.
Check Other Important Links for JEE Main 2025
JEE 2025 needs well-structured study materials. Here are some valuable resources to help you plan your preparation and practice efficiently.
FAQs on Wheatstone Bridge for JEE Main Physics 2025
1. What is the Wheatstone bridge used for?
Wheatstone bridge is used to measure resistances ranging from a few ohms to a few kilo-ohms. The measurements are very precise since the apparatus is very sensitive. Some arrangements, based on the same principle, are
Carey Foster bridge
Post office bridge
Meter bridge
Kelvin bridge
Maxwell bridge
Wien bridge
2. Why are Wheatstone bridge measurements accurate?
The common setups lack precision because practical ammeters and voltmeters do not have zero and infinite resistances respectively. The principle of the Wheatstone bridge is based on the null method (the arrangement is such that the current through the galvanometer is zero) that does not depend on the resistance of the galvanometer. This makes the measurements very precise.
3. What is the principle of the Wheatstone Bridge?
The Wheatstone Bridge works on the principle of balancing two legs of a bridge circuit. When the ratio of resistances in one branch equals the ratio in the other, no current flows through the galvanometer, indicating a balanced Wheatstone Bridge.
4. How is the Wheatstone Bridge formula derived?
The Wheatstone Bridge formula is derived using Kirchhoff’s Laws. For a balanced Wheatstone Bridge, the voltage drops across each branch are equal, leading to the relationship:
$\frac{R_1}{R_2} = \frac{R_3}{R_x}$
This is used to calculate the unknown resistance $R_x$.
5. What are the advantages of using a Wheatstone Bridge?
The Wheatstone Bridge provides highly accurate and precise measurements, especially for medium-range resistances. It is simple to set up and effective in practical applications like strain gauges and temperature sensors.
6. What is the role of the galvanometer in a Wheatstone Bridge circuit?
The galvanometer detects the flow of current through its branch. In a balanced Wheatstone Bridge, the galvanometer shows no deflection, indicating no current flow.
7. How is a Wheatstone Bridge diagram represented?
A typical Wheatstone Bridge diagram consists of four resistances arranged in a quadrilateral shape, a voltage source across one pair of opposite corners, and a galvanometer across the other pair.
8. What are some common applications of the Wheatstone Bridge?
Applications include:
Measuring unknown resistances.
Strain gauge circuits for detecting mechanical strain.
Thermistor-based temperature measurements.
Testing electrical components in laboratories.
9. How does the Wheatstone Bridge circuit behave in an unbalanced state?
In an unbalanced Wheatstone Bridge, there is a potential difference across the galvanometer, causing current to flow. This imbalance is used to identify resistance changes.
10. What are the limitations of the Wheatstone Bridge?
Limitations include:
Ineffectiveness for very high or low resistances.
Sensitivity to temperature and voltage fluctuations.
11. How does a balanced Wheatstone Bridge improve measurement accuracy?
A balanced Wheatstone Bridge eliminates the dependency on the resistance of the galvanometer and ensures that only the resistances in the bridge affect the measurement.
12. What is the use of the Wheatstone Bridge in modern electronics?
Modern uses include sensors like strain gauges and piezoresistive elements, where the bridge detects minute changes in resistance caused by external factors.
13. What factors affect the accuracy of a Wheatstone Bridge?
Factors include:
Stability of the voltage source.
Precision of the known resistances.
Temperature stability of the components.
14. How is the Wheatstone Bridge used to calculate unknown resistance?
By adjusting the known resistances and balancing the bridge, the unknown resistance is calculated using the formula:
$R_x = \frac{R_3 \cdot R_2}{R_1}$
15. Why is the Wheatstone Bridge preferred over simple series-parallel circuits?
It provides more accurate results due to the null method, where the measurement is independent of galvanometer resistance.















