NCERT Free PDF Download Class 12 Physics
FAQs on NCERT Exemplar for Class 12 Physics - Communication Systems - Free PDF Download
1. What are the definitions of terms like Amplitude, Frequency, etc?
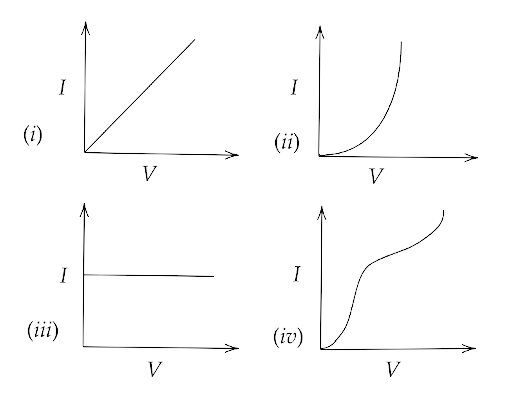
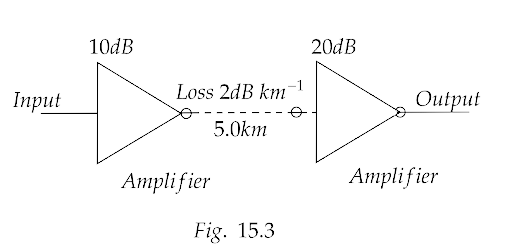
Amplitude is defined as the maximum amount of oscillation from the level of Equilibrium. Frequency is the number of waves that pass through a specific location in certain periods of time. Attenuation is when the signal’s strength decreases when it passes through a particular medium. Bandwidth is the Frequency range in which a particular equipment functions. Range is the farthest distance between the source and the destination till where a signal can be strong enough to be received effectively. Amplification is when an electronic circuit is used to increase the Amplitude of a signal. A phase shift happens when the percentage of a wave cycle has elapsed in relative position to the origin.
2. How can Revision Notes enhance my understanding of this topic?
The NCERT textbooks offer a syllabus that is perfectly in sync and completely comprehensive in nature. Vedantu’s Revision Notes helps you take your study and preparation a notch higher. They offer a bird’s eye view of every Chapter included in Class 12 Physics . When you sit to revise for your Exams a few days before they commence, you naturally do not have the time to go through your complete textbook. This is where having Vedantu’s Revision Notes for Class 12 Physics Chapter 14 can be quite handy. Moreover it is available for free download! All you need to do is sign in and register yourself.
3. What is meant by modulation? What are the different types of modulation?
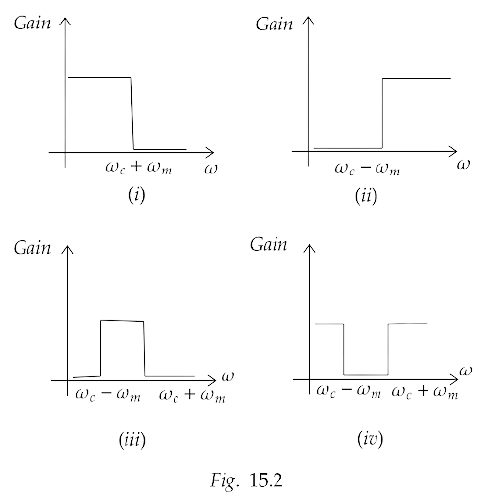
When low-Frequency information signals become incapable of covering long distance, they are superimposed on a high Frequency wave at the transmission point which then acts as a data carrier. Modulations are of three different types - 1) Amplitude modulation 2) Frequency modulation 3) Phase modulation. Amplitude modulation is when the carrier wave’s Amplitude varies in response to the information signal. Frequency modulation is when the carrier wave’s Frequency varies in response to the information signal. Phase modulation is when the phase changes in response to the information stream. There is also pulse-wave modulation which includes pulse-Amplitude modulation, pulse width modulation and pulse position modulation.
4. What are some terminologies related to bandwidth?
Bandwidth is the amount of data that can be transmitted in a certain length of time. All the systems of communication that we use such as pictures, music, computer data can be used as message signals. All of them have different Frequency ranges. For instance, video transmissions require a bandwidth of 4.2 MHz to transmit a picture. Mediums such as FM broadcasting, television, radio in our mobile phones have fixed Frequency bands. It is the International Telecommunication Union which manages Frequency allocation.
5. How can I benefit from the NCERT Exemplar Solutions?
The NCERT Exemplar Solutions PDF is the perfect companion to the exhaustive material covered in the NCERT Textbooks. Working out Physics problems and answering the in-text Exercises is as important as strengthening your conceptual understanding as that covers the major portion of the Physics question papers. The NCERT Exemplar Solutions are prepared by subject experts passionate about creating a difference in the way education reaches the students. It includes solutions to the in-text Exercises as per the guidelines of the CBSE that prepares the NCERT textbooks. Hence, the PDFs are error-free and your best friend as you prepare for the Exams! You can benefit by going through them regularly and comparing your own answers to the ones given in the NCERT Exemplar Solutions document.