Quadrilaterals Class 9 Notes CBSE Maths Chapter 8 (Free PDF Download)

Introduction:

• We're all familiar with planar figures with sides defined by straight line segments, which are known as Polygons.

• The word polygon comes from the Greek language. 

• It refers to a figure with a lot of angles, meaning a lot of sides. 

• Quadrilaterals are squares, rectangles, and other four-sided geometric shapes produced by the union of four line segments.

• A quadrilateral is a polygon with four sides.

Examples of Quadrilaterals:

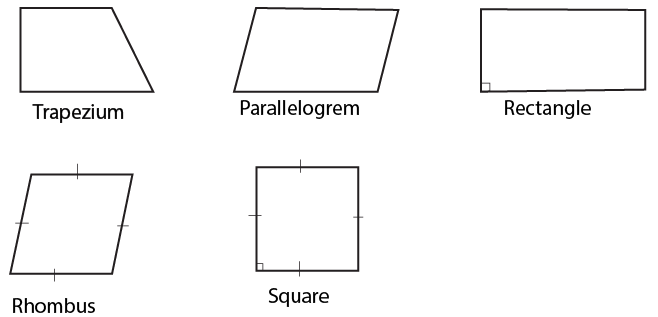
Parallelograms:

• A parallelogram is a quadrilateral with parallel and equal opposite sides.

• Parallelograms include a rectangle, a rhombus, and a square.

• A trapezium is a quadrilateral with one pair of opposite sides that are parallel to one other. As a result, it isn't a parallelogram.

• The opposite sides of each pair are equal and parallel.

In the diagram,

• Opposite Sides: 

$AB\parallel DC$ and $AD\parallel BC$ 

$AB=DC$ and $AD=BC$

• Opposite Angles are Equal.

From figure,

$\widehat{A}=\widehat{C}$ and $\widehat{B}=\widehat{D}$

• Diagonals of a Parallelogram Bisect Each Other.

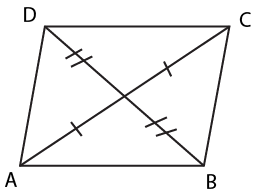
In the diagram, 

$OD=OB$ and $OA=OC$

• Each diagonal divides the parallelogram into two congruent triangles.

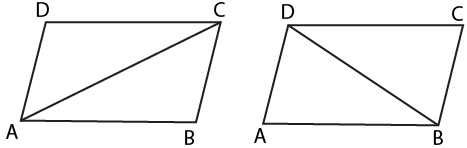
In the diagram, 

$\vartriangle ABC\cong \vartriangle CDA$

$\vartriangle ABD\cong \vartriangle CDB$

Opposite Sides of a Quadrilateral:

• Two sides of a quadrilateral, which have no common point, are called opposite sides.

• In the diagram, $AB$ and $DC$ is one pair of opposite sides.

• $DC$ and $BC$ is the other pair of opposite side

Consecutive Sides of a Quadrilateral:

• Two sides of a quadrilateral, which have a common end point, are called consecutive sides. 

• In the diagram,

$AB$ and $BC$ is one pair of consecutive sides.

$BC,CD;CD,DA$and $DA,AB$are the other three pairs of consecutive sides.

Opposite Angles of a Quadrilateral:

• Two angles, which do not include a side in their intersection, are called the opposite angles of a quadrilateral.

• In the diagram,

$\widehat{A}$ and $\widehat{C}$ is one pair of opposite angles, $\widehat{B}$ and $\widehat{D}$ are another pair of opposite angles.

Consecutive angles of a quadrilateral:

• Two angles of a quadrilateral, which include a side in their intersection, are called consecutive angles.

• In the diagram,

$\widehat{A}$ and $\widehat{B}$ is one pair of consecutive angles, $\widehat{B},\widehat{C};\widehat{C}\widehat{D}$ and $\widehat{D}\widehat{A}$ are other three pairs of consecutive angles.

Theorem $1$:

Statement:

• The diagonals of a parallelogram bisect each other.

• If two sides of a triangle are unequal, the longer side has the greater angle opposite to it.

• $ABCD$ is a parallelogram in which diagonals $AC$ and \[BD\] intersect each other at $O$.

To prove:

The diagonals $AC$ and \[BD\] bisect eac$\widehat{B}$ and $\widehat{D}$ areh other that is,

$AO=OC$ and

$BO=DO$.

Proof:

$AB\parallel CD$ (By definition of parallelogram) 

$AC$ is a transversal.

$\therefore O\widehat{A}B=O\widehat{C}D\text{ }.....\text{ }\left( i \right)$ (Alternate angles are equal in a parallelogram)

Also,

$AB=DC$ (Opposite sides are equal in a parallelogram) 

Now in $\Delta AOB$ and $\Delta COD

$AB=DC$ (Opposite sides of parallelogram are equal)

$O\widehat{A}B=O\widehat{C}D$ (Proved by $\left( i \right)$)

$A\widehat{O}B=C\widehat{O}D$ (Vertically opposite angles are equal)

Therefore,

\[\vartriangle AOB\text{ }\cong \text{ }\vartriangle COD\] ($AAS$ Congruency condition)

Therefore,

$AO=OC$ and $BO=OD$(corresponding parts of congruent triangles are congruent) that is the diagonals of a parallelogram bisect each other.

Sufficient Conditions for a Quadrilateral to be a Parallelogram:

We can state the defining property of a parallelogram as follows:

"If a quadrilateral is a parallelogram, then its opposite sides are equal".

Converse: 

"If both pairs of opposite sides of a quadrilateral are equal, then the quadrilateral is a parallelogram".

• For a quadrilateral to be a parallelogram, the converse assertion given above is a necessary condition.

• Similarly, for a quadrilateral to be a parallelogram, we can establish the following two requirements;

1. "If the diagonals of a quadrilateral bisect each other, the quadrilateral is a parallelogram".

2. "If either pair of opposite sides of a quadrilateral are equal and parallel, the quadrilateral is a parallelogram".

Theorem $2$ :

Statement:

If the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.

Given:

$ABCD$ is a quadrilateral in which diagonals $AC$ and $BD$  intersect at $O$ such that $AO=OC$ and $BO=OD$.

To prove:

$ABCD$ is a parallelogram.

Proof:

In triangles $AOB$and $COD$,

 $AO=CO$ (Given)

$BO=OD$ (Given)

$A\widehat{O}B=C\widehat{O}D$ (Vertically opposite angles are equal)

Therefore,

\[\Delta AOB\text{ }\cong \text{ }\Delta COD\] ($SAS$ Congruency condition)

Therefore,

$O\widehat{A}B=O\widehat{C}D$ ($cpct$)

Since these are alternate angles made by the transversal $AC$ intersecting $AB$ and $CD$

Therefore,

$AB\parallel CD$

Similarly,

$AD\parallel BC$

Hence, $ABCD$is a parallelogram.

Theorem $3$:

Statement:

A quadrilateral is a parallelogram if one pair of opposite sides are equal and parallel.

Given:

$ABCD$ is a quadrilateral in which $AB\parallel CD$and $AB=CD$.

To prove:

$ABCD$ is a parallelogram.

Construction:

Join $AC$.

Proof:

In triangles $ABC$ and  $ADC$, 

$AB=CD$ (Given)

$B\widehat{A}C=A\widehat{C}D$ (Alternate angles are equal)

$AC=AC$ (Common side)

Therefore,

\[\Delta ABC\text{ }\cong \text{ }\Delta CDA\] ($SAS$ Congruency condition)

$B\widehat{C}A=D\widehat{A}C$ (Corresponding parts of corresponding triangles) 

Since these are alternate angles, 

$AB\parallel CD$

Thus, in the quadrilateral $ABCD$, $AB\parallel CD$ and $AD\parallel BC$

Therefore, $ABCD$ is a parallelogram.

Special Parallelograms:

• The set of parallelograms includes rectangles, rhombuses, and squares. 

• The following is a possible definitions for each of these:

A is a parallelogram that is both equilateral and equiangular. As a result, a square can be both a rectangle and a rhombus.

• The relationships between the special parallelograms can be visualized in the diagram below:

• Because every rectangle and rhombus must be a parallelogram, they're depicted as subsets of a parallelogram, and because a square is both a rectangle and a rhombus, it's represented by the overlapping shaded part.

Rectangle:

A rectangle is a parallelogram with one of its angles as a right angle.

In the above figure,

Let, $\widehat{A}={{90}^{\circ }}$

Since,

$AD\parallel BC$,

$\widehat{A}+\widehat{B}={{180}^{\circ }}$

(Sum of interior angles on the same side of transversal $AB$)

Therefore,

$\widehat{B}={{90}^{\circ }}$

Here,

$AB\parallel CD$ and $\widehat{A}={{90}^{\circ }}$ (Given)

Therefore,

$\widehat{A}+\widehat{D}={{180}^{\circ }}$

   $\therefore \widehat{D}={{90}^{\circ }}$

   $\therefore \widehat{C}={{90}^{\circ }}$

Corollary: Each of the four angles of a rectangle is a right angle. 

Rhombus:

A rhombus is a parallelogram with a pair of its consecutive sides equal. 

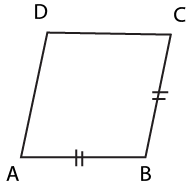
$ABCD$ is a rhombus in which $AB=BC$.

Since a rhombus is a parallelogram, 

$AB=DC$ and $BC=AD$

 Thus, $AB=BC=CD=AD$

Corollary: All the four sides of a rhombus are equal (congruent). 

Square:

A square is a rectangle with a pair of its consecutive sides equal.

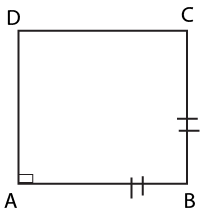
Since square is a rectangle, each angle of a rectangle is a right angle and $AB=DC$, $BC=CD$. 

Thus,

 $AB=BC=CD=AD$

Each of the four angles of a square is a right angle and each of the four sides is of the same length.

Theorem $4$:

Statement:

The diagonals of a rectangle are equal in length.

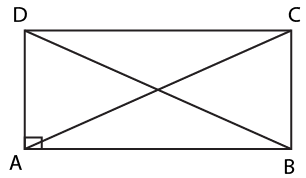
Given:

$ABCD$ is a rectangle.

$AC$ and $BD$are diagonals.

To prove:

$AC=BD$

Proof:

Let, $\widehat{A}={{90}^{\circ }}$ (By definition of rectangle)

$\widehat{A}+\widehat{B}={{180}^{\circ }}$ (Consecutive interior angle)

$\widehat{A}=\widehat{B}={{90}^{\circ }}$

Now in triangles, $ABD$ and $ABC$,

$AB=AB$ (Common side)

$\widehat{A}=\widehat{B}={{90}^{\circ }}$ (Each angle is a right angle) 

$AD=BC$ (Opposite sides of parallelogram)

Therefore,

\[\Delta ABD\text{ }\cong \text{ }\Delta BAC\]

Therefore,

$BD=AC$ (Corresponding parts of corresponding triangles) 

Hence the theorem is proved.

Converse of Theorem $4$:

Statement:

If two diagonals of a parallelogram are equal, it is a rectangle.

Given:

$ABCD$ is a parallelogram in which $AC=BD$.

To prove:

Parallelogram $ABCD$ is a rectangle.

Proof:

In triangles $ABC$ and $DBC$,

$AB=DC$ (Opposite sides of parallelogram) 

$BC=BC$ (Common side)

$AC=BD$ (Given)

Therefore,

\[\Delta ABC\text{ }\cong \text{ }\Delta DCB\] (\[SSS\] congruency condition)

Therefore,

$A\widehat{B}C=D\widehat{C}B$ (Corresponding parts of corresponding triangles)

But these angles are consecutive interior angles on the same side of transversal $BC$ and $AB\parallel DC$.

Therefore,

$A\widehat{B}C+D\widehat{C}B={{180}^{\circ }}$

But, 

$A\widehat{B}C=D\widehat{C}B$

Therefore,

$A\widehat{B}C=D\widehat{C}B={{90}^{\circ }}$

Therefore, by definition of rectangle, parallelogram $ABCD$ is a rectangle.

Hence the theorem is proved.

Theorem $5$:

Statement:

The diagonals of a rhombus are perpendicular to each other.

Given:

$ABCD$ is a rhombus. 

Diagonal $AC$ and $BD$ intersect at $O$.

To prove:

$AC$and $BD$ bisect each other at right angles.

Proof:

A rhombus is a parallelogram such that  

$\text{AB = DC = AD = BC    }......\left( \text{i} \right)$

Also the diagonals of a parallelogram bisect each other. 

Hence,

$BO=DO$ and $AO=\text{OC   }......\left( \text{ii} \right)$ 

Now, compare triangles $AOB$ and $AOD$,

$AB=AD$ (From $\left( \text{i} \right)$ above)

$BO=DO$ (From $\left( \text{ii} \right)$ above)

$\text{AO = AO}$ (Common side)

Therefore,

\[\Delta AOB\text{ }\cong \text{ }\Delta AOD\] (\[SSS\] congruency condition)

Therefore,

\[A\widehat{O}B=A\widehat{O}D\] (Corresponding parts of corresponding parts)

 \[BD\] is a straight line segment.

Therefore,

\[A\widehat{O}B+A\widehat{O}D={{180}^{\circ }}\]

But,

\[A\widehat{O}B=A\widehat{O}D\] (Proved)

Therefore,

\[A\widehat{O}B=A\widehat{O}D=\dfrac{{{180}^{\circ }}}{2}\]

\[A\widehat{O}B=A\widehat{O}D={{90}^{\circ }}\]

That is, the diagonals bisect at right angles. 

Hence the theorem is proved.

Converse of Theorem $5$: 

Statement:

If the diagonals of a parallelogram are perpendicular then it is a rhombus.

Given:

$ABCD$ is a parallelogram in which $AC$ and $BD$ are perpendicular to each other.

To prove:

$ABCD$ is a rhombus.

Proof:

Let $AC$ and $BD$ intersect at right angles at $O$.

\[A\widehat{O}B={{90}^{\circ }}\]

In triangles $AOD$ and $COD$,

$AO=OC$ (Diagonals bisect each other) 

$OD=OD$ (Common side)

\[A\widehat{O}D=C\widehat{O}D={{90}^{\circ }}\] (Given)

Therefore,

\[\Delta AOD\text{ }\cong \text{ }\Delta COD\] (\[SAS\] congruency condition)

$AD=DC$

That is, the adjacent sides are equal.

Therefore, by definition, $ABCD$ is a rhombus.

Hence the theorem is proved.

Theorem $6$:

Statement:

The diagonals of a square are equal and perpendicular to each other.

Given:

$ABCD$ is a square.

$AC$ and $BD$ are diagonals intersecting at $O$.

To prove:

$AC=BD$ and $AC\bot BD$

Proof:

$AB=AD$ (Sides of a square are equal)

$AB\parallel DC$ (Opposite sides of a square are parallel)

Therefore,

$ABCD$ is parallelogram with consecutive sides equal. 

Hence, $ABCD$ is a rhombus. (By definition)

Since, the diagonals of a rhombus are perpendicular to each other,

$AC\bot BD$

Therefore,

$ABCD$ is a parallelogram.

$AB=AD$ and \[\widehat{A}={{90}^{\circ }}\]

Therefore, $ABCD$ is a rectangle with a pair of its consecutive sides equal.

Since the diagonals of a rectangle are equal, $AC=BD$. 

Therefore,

$\text{Diagonal AC = Diagonal BD}$ and $AC\bot BD$

Hence, the theorem is proved.

Converse of Theorem $6$:

Statement:

If in a parallelogram, the diagonals are equal and perpendicular, then it is a square.

Given:

$ABCD$ is a parallelogram. 

$AC=BD$ and $AC\bot BD$

To prove:

$ABCD$ is a square.

Proof:

Since the diagonals $AC$ and $BD$ are equal,

$ABCD$ is a rectangle  - - - - $\left( \text{i} \right)$ 

(Diagonal property of rectangle)

Since the diagonals are perpendicular to each other. 

$ABCD$ is a rhombus.

Therefore,

\[AB=AD\] - - - - $\left( \text{ii} \right)$

$ABCD$ is a rectangle. (From $\left( \text{i} \right)$)

With consecutive sides equal. (From $\left( \text{ii} \right)$)

Therefore,

$ABCD$ is a square. (By definition of a square)

 Hence, the theorem is proved.

The Mid-point Theorem:

Parallel lines and Triangles

So far, we've proved a number of parallelogram theorems. Now let's use these theorems to prove a few interesting and helpful triangle facts.

Statement:

"The line segment joining the mid-points of any two sides of a triangle is parallel to the third side and equal to half of it".

Given:

In $\Delta ABC$, 

$AB=DB$and $AE=EC$

To prove:

1. $DE\parallel BC$

2. $DE=\dfrac{1}{2}BC$

Construction:

Analysis for construction shows that you need to draw $CF\parallel BD$.

A parallelogram with $DB$ and $BC$ as consecutive sides. 

Proof:

In triangles, $ADE$ and $CEF$,

$AE=EC$ (Given)

\[A\widehat{E}D=C\widehat{E}F\] (Vertically opposite angles)

\[D\widehat{A}E=E\widehat{C}F\] (Alternate angles, \[AD\parallel CF\] by construction)

Therefore,

\[\Delta ADE\text{ }\cong \text{ }\Delta CFE\] (\[ASA\] Congruency test)

Therefore, 

\[AD=CF\] and \[DE=EF\] (Corresponding parts of corresponding triangles) 

But

\[AD=DB\] (Given)

Therefore,

\[\text{DB = CF     }.....\left( \text{i} \right)\] 

(\[AD\] is equal to both \[DB\]and \[CF\]) 

In quadrilateral \[DBCF\],

\[DB=CF\] and \[DB\parallel CF\]

Therefore,

\[DBCF\] is a parallelogram. (By definition of parallelogram)

Therefore,

\[DF=BC\] (Opposite sides of a parallelogram are equal) 

and 

\[\text{DF }\parallel \text{ BC     }.....\left( \text{ii} \right)\]

But

\[DE=EF\] (Proved above)  

and

\[DF=DE+EF\]

\[DF=2DE\]

and \[DF=BC\] (From \[\left( \text{ii} \right)\])

Therefore,

 \[BC=2DE\] or

\[DE=\dfrac{1}{2}BC\]

Hence, the theorem is proved.

Converse of Mid-point Theorem:

Statement:

A straight line drawn through the mid-point of one side and parallel to another side of a triangle bisects the third side.

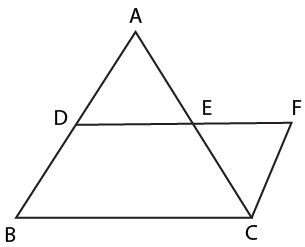
Given:

\[\Delta ABC\] in which \[D\] is the mid-point of \[AB\] and \[\text{DE }\parallel \text{ BC}\].

To prove:

\[\text{E}\] is the mid-point of \[\text{AC}\]. That is, to prove \[\text{AE=EC}\].

Construction:

Since \[\text{DE }\parallel \text{ BC}\], we can complete a parallelogram with \[\text{DB}\] and \[\text{BC}\] as consecutive sides. 

Hence draw \[\text{EF }\ parallel \text{ BD}\] to meet \[\text{DE}\] produced at \[\text{F}\].

Proof:

In quadrilateral \[\text{DBCF}\],

\[\text{DB }\parallel \text{ CF}\] (By construction) 

\[\text{DF }\parallel \text{ BC}\] (Given)

Therefore,

\[\text{DBCF}\] is a parallelogram.

\[\text{DB = CF     }.....\left( \text{i} \right)\] (Opposite sides of a parallelogram) 

But

\[\text{DB = AD     }.....\left( \text{ii} \right)\] (Given)

Now, from \[\left( \text{i} \right)\] and \[\left( \text{ii} \right)\], 

\[\text{AD = CF}\]

Now compare triangles, \[\text{AED}\] and \[\text{CEF}\],

\[\text{AD=CF}\]

\[A\widehat{E}D=E\widehat{C}F\] (Vertically opposite angles)

\[D\widehat{A}E=E\widehat{C}F\] (Alternate angles, \[AD\parallel CF\] by construction)

Therefore,

\[\Delta AED\text{ }\cong \text{ }\Delta CEF\] (\[ASA\] Congruency test)

\[ AE=EC\]( \[CPCT\])

That is, \[\text{E}\] is the midpoint of \[\text{AC}\]. 

Hence, the theorem is proved.

Recall the above theorem and apply it to the diagram given.

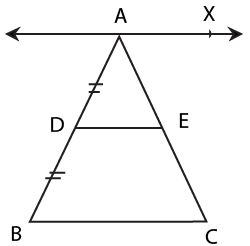
In the diagram if \[\text{D}\] is the mid-point of \[\text{AB}\] and \[\text{DE}\] is drawn parallel to \[\text{BC}\], then \[\text{E}\] will be the midpoint of \[\text{AC}\].

That is,

\[\text{AE = EC}\]

Now if \[\text{AX}\] is drawn parallel to \[\text{BC}\], then also \[\text{AE = EC}\] if \[\text{AD = DB}\].

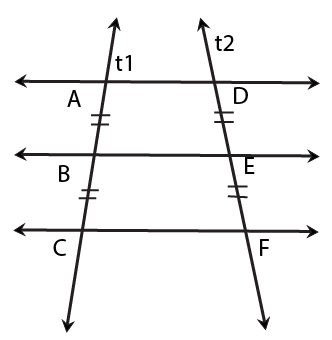
Now draw three parallel lines \[\text{AD, BE, CF}\] as shown in the figure. 

Draw a transversal \[{{\text{t}}_{1}}\] such that \[\text{AB = BC}\].

Now draw another transversal \[{{\text{t}}_{2}}\].

Measure \[DE\] and \[EF\]. 

We will get find that \[\text{DE = EF}\] and \[\text{AB = BC}\]. 

In the diagram, \[AD,BE\]and \[CF\] are three parallel lines.

\[AB\] and \[BC\] are equal intercepts made on \[{{\text{t}}_{1}}\].

If any transversal is drawn, the intercepts made on it will also be equal.

The Intercept Theorem:

Statement:

If there are three or more parallel lines and the intercepts made by them on one transversal are equal, the corresponding intercepts of any transversal are also equal.

Given:

\[l\parallel m\parallel n\]

\[P\] is a transversal intersecting \[l,m\] and \[n\] at \[A,B\] and \[C\] respectively such that \[\text{AB = BC}\].

\[Q\] is another transversal drawn to cut \[l,m\] and \[n\] at \[D,E\] and \[F\] respectively. 

\[DE\] and \[EF\] are the intercepts made on \[q\].

To prove:

\[\text{DE = EF}\]

Construction:

Draw a line through \[E\] parallel to \[p\] intersecting \[l\] in \[G,n\] in \[H\] respectively.

Proof:

\[AG\parallel BE\] (Given)

\[GE\parallel AB\] (By construction) 

Therefore,

\[AGEB\] is a parallelogram.

Therefore,

\[\text{GE = AB     }.....\left( \text{i} \right)\] (Opposite sides of parallelogram)

Similarly, \[BEHC\] is a parallelogram.

Therefore,

\[\text{EH = BC     }.....\left( \text{ii} \right)\] (Opposite sides of parallelogram)

But 

\[\text{AB = BC}\] (Given)

From \[\left( \text{i} \right)\] and \[\left( \text{ii} \right)\], 

\[\text{GE = EH}\]

Now, compare triangles \[\text{GED}\] and \[\text{EFH}\], 

\[\text{GE = EH}\] (Proved)

\[G\widehat{E}D=F\widehat{E}H\] (Vertically opposite angles)

\[D\widehat{G}E=F\widehat{H}E\] (Alternate angles, \[GD\parallel FH\] by construction)

Therefore,

\[\Delta GED\text{ }\cong \text{ }\Delta HEF\] (\[ASA\] Congruency test)

Therefore,

\[\text{DE = EF}\]  (Corresponding parts of corresponding triangles)

Hence, the theorem is proved. 

Class 9 Maths Quadrilaterals Notes Free PDF

Quadrilaterals Class 9 Notes is available in a free PDF format on the vedantu official website Download this PDF helps the students to read and understand during their convenient times without bothering about the internet connection. Also, the notes are available in both English and Hindi to avoid any inconvenience for the students to understand the concepts thoroughly. 9th Class Maths Notes pdf is available for students to take the hard copy and store for future use. Also, the notes are available for all chapters of all Subjects class-wise. 

Class 9 Maths Quadrilaterals Notes

Introduction

The chapter has been introduced with the revision of triangles, which consists of three angles. Similarly, a quadrilateral is a figure which consists of four angles, four sides with four different lengths. These figures have certain properties, which can be explained in the rest of the chapter. Certain things like a blackboard, book, table, etc., can be taken as examples for these quadrilaterals to get a clear idea about these figures for the students.

Angle Sum Property of The Quadrilateral

In this section, one of the basic properties of the quadrilateral is explained. It is known as additive property. According to this property, the sum of all angles of a quadrilateral is equal to 360°. It means a complete angle of a circle. 

Types of Quadrilaterals

As of now, it is clear that what is a quadrilateral and what is its angle sum property. Then it is important to explore more about the quadrilaterals and their types. According to 9th Class, Maths Notes Chapter 8, we have five major types of quadrilaterals. They are,

The trapezium is a figure of quadrilateral where a single pair of sites are parallel to each other.

A parallelogram is another type of quadrilateral where both the pairs are parallel to each other.

The rectangle is the third classification of quadrilaterals. It has a right angle, which is equal to 90°.

The rhombus is a quadrilateral figure in which all the sides are equal.

Square is also a type of quadrilateral that combines the features of both rectangle and rhombus. It means that the Square has all equal sides along with an angle of 90°.

Properties of Parallelogram 

Class 9 Quadrilaterals Notes has explained various properties of the most parallelogram by using theorems along with the proofs. Those properties of a parallelogram are,

• A diagonal of a parallelogram can divide it into two congruent triangles.

• In a parallelogram, the opposite sides are equal.

• It is similar to the above property. If the opposite sides are equal to each other in a quadrilateral, then the quadrilateral is said to be a parallelogram.

• Along with the sides, the parallelogram has other properties called opposite angles equal.

• If two diagonals are drawn in a parallelogram, then those two will bisect each other.

• If the above condition applies in a quadrilateral, then that is also known as a parallelogram, and the diagonals also bisect each other.

• If the diagonals are drawn in a rhombus, then the diagonals are perpendicular to each other.

Another Condition For A Quadrilateral To Be A Parallelogram

Quadrilaterals Notes Class 9 specifies another condition for a quadrilateral to be a parallelogram. According to this condition, if a figure of Quadrilaterals has opposite sides equal and parallel with each other, then also it is called a parallelogram. So far it is understood that any of the conditions may get satisfied, from now an additional condition which satisfies both criteria.

Midpoint Theorem 

Quadrilaterals Class 9 Notes has brought a new theorem called midpoint theorem in the last section of the chapter before summary. According to this theorem, the students realized that if a line segment joins two sides of the triangle, it is parallel to the third side of the triangle. It is proved easily with the help of a diagram. Class 9 Maths Chapter 8 Notes also proves the converse of the theorem. This theorem is known as the exact midpoint theorem. According to this theorem, the line drawn to the midpoint of one side of the triangle is parallel to the other side and bisects the third side.

Benefits of Referring Vedantu’s Revision Notes for Class 6 Science Chapter 9

• The notes offer a condensed version of the chapter, making it easy to review important concepts, definitions, and key points in a short amount of time.

• These notes simplify complex topics, ensuring that students have a clear understanding of the chapter's content, making it easier to grasp the subject matter.

• They serve as an excellent resource for last-minute exam preparations, helping students revise the entire chapter efficiently.

• Vedantu provides well-structured notes help in better retention of information, ensuring that students remember crucial details during exams.

• The notes are geared towards helping students prepare for their exams. They include summaries of important points, potential exam questions, and tips for answering them effectively.

• They save time by providing a consolidated source of information, reducing the need to go through the entire textbook again.

• Revision notes often highlight the most important topics and questions, allowing students to prioritize their study efforts.

• These notes may include practical examples and applications, helping students relate the theoretical knowledge to real-life situations.

• By having a clear and concise set of revision notes, students can approach exams with greater confidence, knowing they have covered the essential aspects of the chapter.

Conclusion

The Class 9 CBSE Maths Chapter 8, "Quadrilaterals," is a vital part of your mathematics education. Our free PDF download provides you with comprehensive notes and solutions for a deeper understanding of the topic. You've learned about different types of quadrilaterals, their properties, and how to solve problems related to them. These notes are a valuable resource to help you prepare for your exams and gain confidence in your math skills. Understanding quadrilaterals is not only a fundamental concept in geometry but also a building block for more advanced math topics. So, make the most of these notes to enhance your mathematical knowledge and perform well in your exams.