Answer
399.3k+ views
Hint: We will first find the value of f (x) by generalizing the given relation, $ \int\limits_{1}^{b}{f\left( x \right)dx}=\sqrt{{{b}^{2}}+1}-\sqrt{2} $ and then we will differentiate the obtained general term to get the value of f (x).
Complete step-by-step answer:
It is given in the question that the area bounded by the x-axis, curve y = f (x) and the lines x = 1, x = b is equal to $ \sqrt{{{b}^{2}}+1}-\sqrt{2} $ for all b > 1 and we have been asked to find the value of f (x).
We can represent the given data as follows.
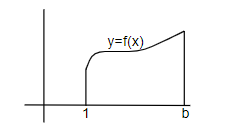
We know that the area under the curve y = (x) and x-axis is given by the integration of y = f (x) from 1 to b, as we have the lines x = 1 and x = b. So, we get,
Area = $ \int\limits_{1}^{b}{f\left( x \right)} $
Now, we have been given that $ \int\limits_{1}^{b}{f\left( x \right)dx} $ is equal to $ \sqrt{{{b}^{2}}+1}-\sqrt{2} $ , so we can write,
$ \int\limits_{1}^{b}{f\left( x \right)dx}=\sqrt{{{b}^{2}}+1}-\sqrt{2} $
We can also write $ \sqrt{2} $ as $ \sqrt{1+1} $ . So, we get,
$ \int\limits_{1}^{b}{f\left( x \right)dx}=\sqrt{{{b}^{2}}+1}-\sqrt{1+1} $
Now, if we observe $ \sqrt{{{b}^{2}}+1}-\sqrt{1+1} $ , we get to know that we can generalize it using the general function $ \left[ \sqrt{{{x}^{2}}+1} \right]_{1}^{b} $ , so we get,
$ \int\limits_{1}^{b}{f\left( x \right)dx}=\left[ \sqrt{{{x}^{2}}+1} \right]_{1}^{b} $
We know that integration is the reverse of differentiation, so we can write it as,
$ f\left( x \right)=\dfrac{d\left( \sqrt{{{x}^{2}}+1} \right)}{dx} $
Now, we will differentiate $ \left( \sqrt{{{x}^{2}}+1} \right) $ with respect to x. We know that the derivative of $ \sqrt{x} $ is $ \dfrac{1}{2\sqrt{x}} $ . So, we can write the derivative of $ \left( \sqrt{{{x}^{2}}+1} \right) $ as,
$ \begin{align}
& f\left( x \right)=\dfrac{1}{2\sqrt{{{x}^{2}}+1}}\times 2x \\
& f\left( x \right)=\dfrac{x}{\sqrt{{{x}^{2}}+1}} \\
\end{align} $
So, the correct answer is “Option D”.
Note: Many time, the students take the opposite limit after generalizing the function, f (x), they may take the function, $ \int{f\left( x \right)dx}=\left[ \sqrt{{{x}^{2}}+1} \right]_{b}^{1} $ which gives the opposite result. They might get a negative sign in the area of the region found out, but it is also correct as we will neglect the negative sign because the area cannot be negative.
Complete step-by-step answer:
It is given in the question that the area bounded by the x-axis, curve y = f (x) and the lines x = 1, x = b is equal to $ \sqrt{{{b}^{2}}+1}-\sqrt{2} $ for all b > 1 and we have been asked to find the value of f (x).
We can represent the given data as follows.
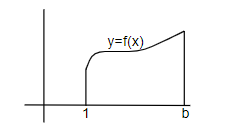
We know that the area under the curve y = (x) and x-axis is given by the integration of y = f (x) from 1 to b, as we have the lines x = 1 and x = b. So, we get,
Area = $ \int\limits_{1}^{b}{f\left( x \right)} $
Now, we have been given that $ \int\limits_{1}^{b}{f\left( x \right)dx} $ is equal to $ \sqrt{{{b}^{2}}+1}-\sqrt{2} $ , so we can write,
$ \int\limits_{1}^{b}{f\left( x \right)dx}=\sqrt{{{b}^{2}}+1}-\sqrt{2} $
We can also write $ \sqrt{2} $ as $ \sqrt{1+1} $ . So, we get,
$ \int\limits_{1}^{b}{f\left( x \right)dx}=\sqrt{{{b}^{2}}+1}-\sqrt{1+1} $
Now, if we observe $ \sqrt{{{b}^{2}}+1}-\sqrt{1+1} $ , we get to know that we can generalize it using the general function $ \left[ \sqrt{{{x}^{2}}+1} \right]_{1}^{b} $ , so we get,
$ \int\limits_{1}^{b}{f\left( x \right)dx}=\left[ \sqrt{{{x}^{2}}+1} \right]_{1}^{b} $
We know that integration is the reverse of differentiation, so we can write it as,
$ f\left( x \right)=\dfrac{d\left( \sqrt{{{x}^{2}}+1} \right)}{dx} $
Now, we will differentiate $ \left( \sqrt{{{x}^{2}}+1} \right) $ with respect to x. We know that the derivative of $ \sqrt{x} $ is $ \dfrac{1}{2\sqrt{x}} $ . So, we can write the derivative of $ \left( \sqrt{{{x}^{2}}+1} \right) $ as,
$ \begin{align}
& f\left( x \right)=\dfrac{1}{2\sqrt{{{x}^{2}}+1}}\times 2x \\
& f\left( x \right)=\dfrac{x}{\sqrt{{{x}^{2}}+1}} \\
\end{align} $
So, the correct answer is “Option D”.
Note: Many time, the students take the opposite limit after generalizing the function, f (x), they may take the function, $ \int{f\left( x \right)dx}=\left[ \sqrt{{{x}^{2}}+1} \right]_{b}^{1} $ which gives the opposite result. They might get a negative sign in the area of the region found out, but it is also correct as we will neglect the negative sign because the area cannot be negative.
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Assertion The resistivity of a semiconductor increases class 13 physics CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the stopping potential when the metal with class 12 physics JEE_Main

The momentum of a photon is 2 times 10 16gm cmsec Its class 12 physics JEE_Main

Using the following information to help you answer class 12 chemistry CBSE

Trending doubts
Difference Between Plant Cell and Animal Cell

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

Write an application to the principal requesting five class 10 english CBSE

What organs are located on the left side of your body class 11 biology CBSE

What is the z value for a 90 95 and 99 percent confidence class 11 maths CBSE



