
What is the z value for a 90, 95, and 99 percent confidence interval?
Answer
455.1k+ views
1 likes
Hint: We need to know how to calculate the area under the curve for the given z value using the formula
Complete step-by-step solution:
Let us consider the first case for which the given confidence level is 90 percent. In this case, we need to calculate the area under the curve and it can be given as shown in the figure below.
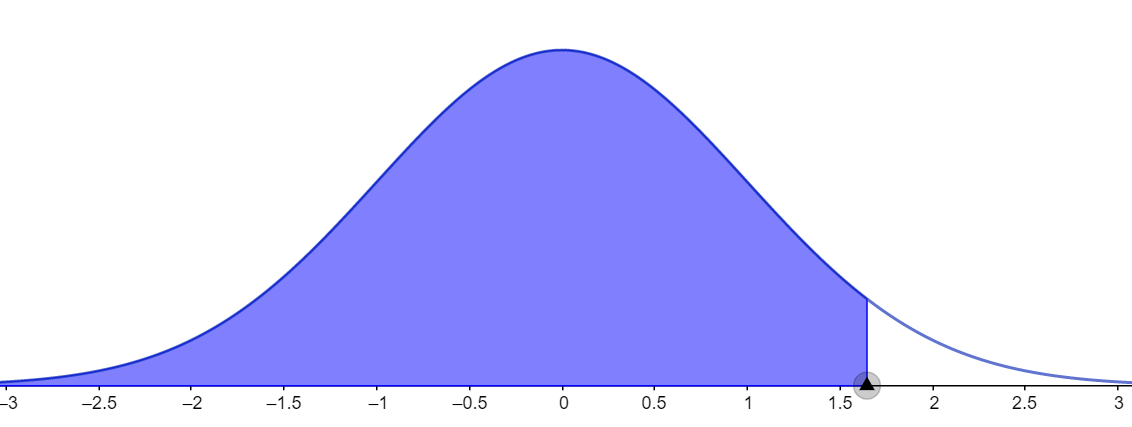
It can be calculated by using the formula
Adding and dividing by 2,
Looking for this value in the normal distribution table given below, we can see that this value lies close to the row containing 1.6 and column containing 0.05. It also lies close to the row containing 1.6 and column containing 0.04. So, we take a mean of these values to obtain the z value at this point.
Hence, the z value at the 90 percent confidence interval is 1.645.
Let us consider the second case for which the given confidence level is 95 percent. In this case, we need to calculate the area under the curve and it can be given as shown in the figure below.
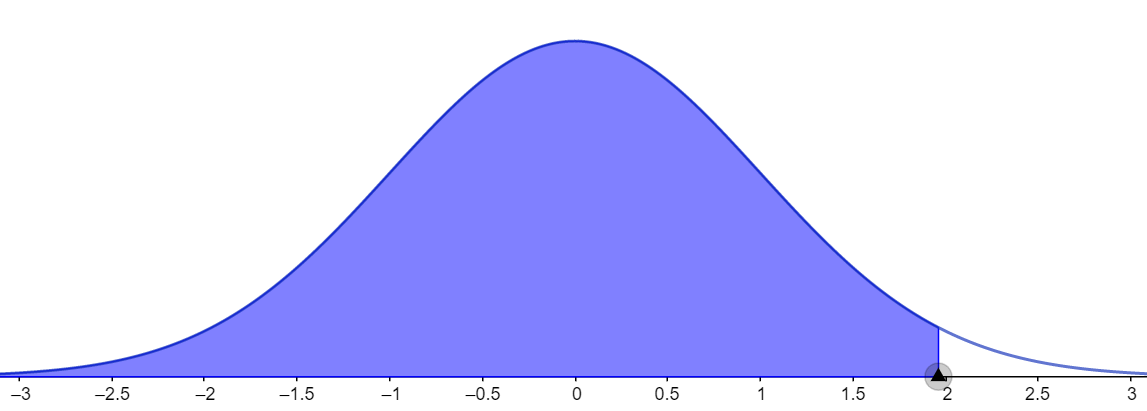
It is calculated by using the formula
Adding and dividing by 2,
Looking for this value in the normal distribution table given above, we can see that this value lies on the row containing 1.9 and column containing 0.06. Adding the two values,
Hence, the z value at the 95 percent confidence interval is 1.96.
Let us consider the third case for which the given confidence level is 99 percent. In this case too, we need to calculate the area under the curve and it can be given as shown in the figure below.
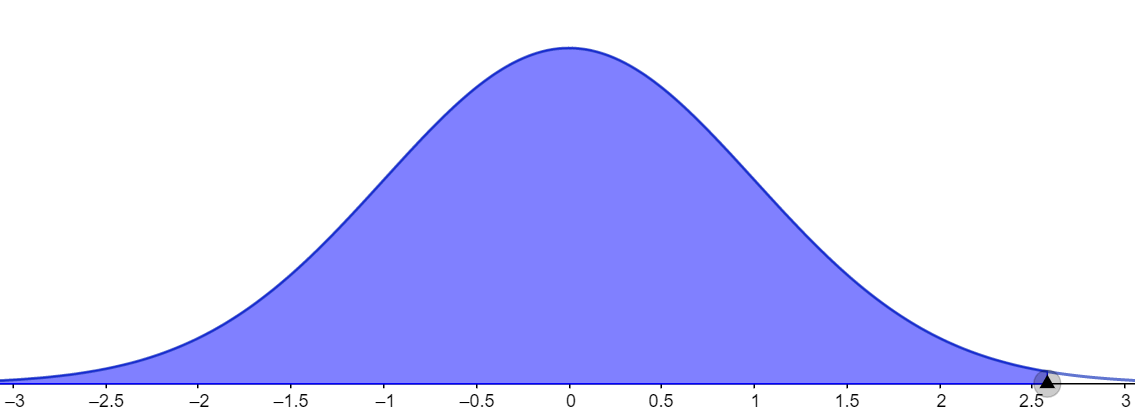
It is calculated by using the formula
Adding and dividing by 2,
Looking for this value in the normal distribution table given above, we can see that this value lies on the row containing 2.5 and column containing 0.08. Adding the two values,
Hence, the z value at the 99 percent confidence interval is 2.58.
Note: : It is important to take care while noting down the z value from the table, since it can be confusing and it is common to make errors while reading data from a table usually. It is important to know the concept of probability and statistics to solve this question.
Complete step-by-step solution:
Let us consider the first case for which the given confidence level is 90 percent. In this case, we need to calculate the area under the curve and it can be given as shown in the figure below.

It can be calculated by using the formula
Adding and dividing by 2,
Looking for this value in the normal distribution table given below, we can see that this value lies close to the row containing 1.6 and column containing 0.05. It also lies close to the row containing 1.6 and column containing 0.04. So, we take a mean of these values to obtain the z value at this point.
Hence, the z value at the 90 percent confidence interval is 1.645.

Let us consider the second case for which the given confidence level is 95 percent. In this case, we need to calculate the area under the curve and it can be given as shown in the figure below.

It is calculated by using the formula
Adding and dividing by 2,
Looking for this value in the normal distribution table given above, we can see that this value lies on the row containing 1.9 and column containing 0.06. Adding the two values,
Hence, the z value at the 95 percent confidence interval is 1.96.
Let us consider the third case for which the given confidence level is 99 percent. In this case too, we need to calculate the area under the curve and it can be given as shown in the figure below.

It is calculated by using the formula
Adding and dividing by 2,
Looking for this value in the normal distribution table given above, we can see that this value lies on the row containing 2.5 and column containing 0.08. Adding the two values,
Hence, the z value at the 99 percent confidence interval is 2.58.
Note: : It is important to take care while noting down the z value from the table, since it can be confusing and it is common to make errors while reading data from a table usually. It is important to know the concept of probability and statistics to solve this question.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE




