NCERT Solutions for Class 11 Maths Chapter 10 Miscellaneous Exercise - Free PDF Download
FAQs on NCERT Solutions for Class 11 Maths Chapter 10 Conic Sections Miscellaneous Exercise
1. What is the focus of the NCERT Solutions of Miscellaneous Exercise Class 11 Chapter 10?
In NCERT Solutions of Miscellaneous Exercise Class 11 Chapter 10 reviews conic sections, including circles, parabolas, ellipses, and hyperbolas. It integrates various concepts learned throughout the Chapter. The exercise ensures a thorough understanding and application of these concepts.
2. Why are conic sections important in NCERT Solutions of Miscellaneous Exercise Class 11 Chapter 10?
In NCERT Solutions of Miscellaneous Exercise Class 11 Chapter 10, conic sections are crucial in mathematics due to their presence in real-life applications like satellite orbits, architectural designs, and physics. Understanding these shapes aids in solving complex problems related to these areas, making them essential for students to master.
3. How can one identify the type of conic section from its equation in NCERT Solutions of Conic Sections Class 11 Miscellaneous Exercise?
Identifying conic sections involves analysing the general equation $Ax^{2}+Byx+Cy^{2}+Dx+Ey+F=0$. The coefficients and discriminant determine if it is a circle, ellipse, parabola, or hyperbola. Understanding these criteria is essential for solving related problems.
4. What are key strategies for solving problems in NCERT Solutions of Conic Sections Class 11 Miscellaneous Exercise?
Key strategies include understanding the standard forms of conic sections and practising completing the square for equations. Applying geometric properties and drawing diagrams helps visualize and solve the problems effectively, making these strategies crucial for success.
5. Are there any real-life applications included in NCERT Solutions of Conic Sections Class 11 Miscellaneous Exercise?
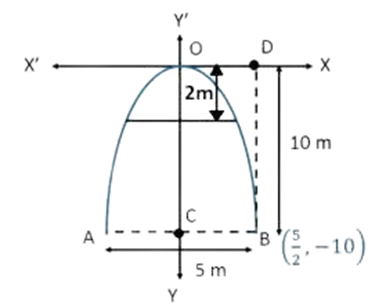
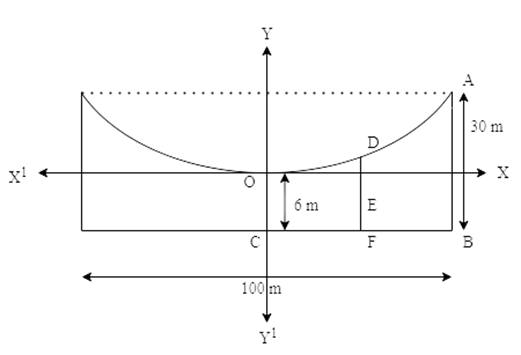
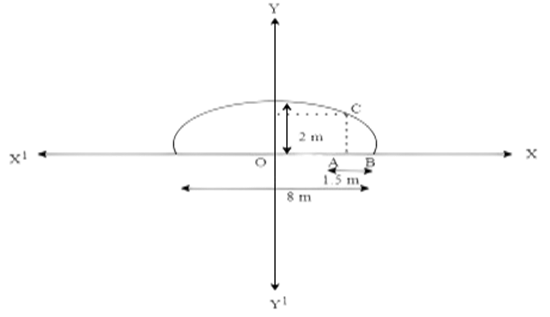
Yes, Conic Sections Class 11 Miscellaneous Exercise some problems involve real-life scenarios such as planetary paths, reflective surface designs, and trajectories in physics. These applications illustrate the practical significance of conic sections, helping students understand their relevance beyond textbook problems.
6. What should students focus on while preparing for this NCERT Solution of Class 11 Maths Conic Sections Miscellaneous Exercise?
Students should focus on mastering the derivation and properties of conic sections. Practising a variety of problems and understanding how to transition between different forms of equations are essential. Consistent practice and reviewing solved examples are crucial for success.
7. What topics are covered in the Class 11 Maths Conic Sections Miscellaneous Exercise?
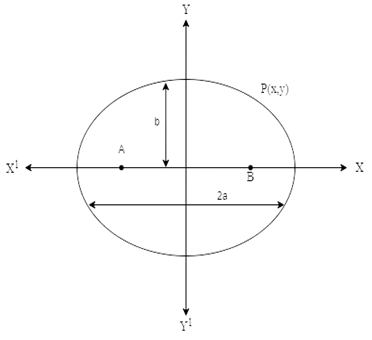
The Miscellaneous Exercise covers various topics related to conic sections, including the equations and properties of circles, ellipses, parabolas, and hyperbolas.
8. How do I solve problems involving the equation of a circle in Class 11 Maths Conic Sections Miscellaneous Exercise?
To solve these problems from Class 11 Maths Conic Sections Miscellaneous Exercise, you need to understand how to derive the equation of a circle given its centre and radius, and how to apply this equation to different scenarios.
9. What kind of questions involve ellipses in the Class 11 Maths Chapter 10 Miscellaneous Solutions?
Questions involving ellipses may require you to find the standard form of the ellipse equation, identify its major and minor axes, and solve problems related to its geometric properties.
10. How are parabolas addressed in the Class 11 Maths Chapter 10 Miscellaneous Solutions?
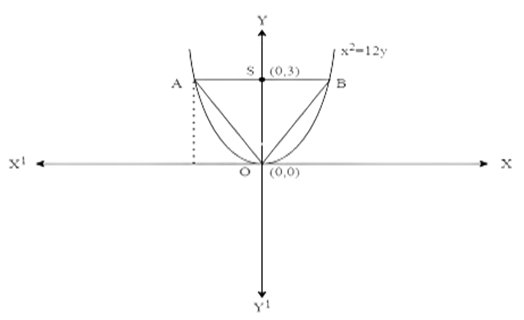
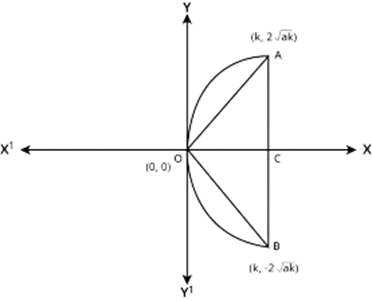
Problems related to parabolas might include finding their equations, determining the focus and directrix, and solving real-world applications using these properties.
11. What is the approach to solving hyperbola-related questions in Class 11 Maths Chapter 10 Miscellaneous Solutions?
Class 11 Maths Chapter 10 Miscellaneous Solutions for hyperbolas, you need to know how to derive their equations, understand their asymptotes, and solve problems involving their geometric features.
12. Why is practising the Class 11 Maths Chapter 10 Miscellaneous Solutions important for exams?
Practising Class 11 Maths Chapter 10 Miscellaneous Solutions helps you thoroughly understand the concepts of conic sections, improves your problem-solving skills, and ensures you are well-prepared for board exams and competitive tests.