Question

Man A sitting in a car moving with a speed of $54 km/hr$ observes a man B in front of the car crossing perpendicularly a road of width $15 m$ in three seconds. Then the velocity of man B (in m/s) will be:
A) $5\sqrt {10} $ $\text{towards the car at some angle.}$
B) $5\sqrt {10} $ $\text{away from the car at some angle.}$
C) $\text{5 perpendicular to the road.}$
D) $\text{15 along the road.}$

Answer 1

Hint: The relative velocity is the velocity of a body with respect to another body. Generally the velocity is represented with respect to the ground but sometimes the point of reference changes and we need to then solve and get the relative velocity of the body.

Complete step by step solution:
The speed of man A in $m/s$ is equal to,
$ \Rightarrow 54 \times \dfrac{5}{{18}} = 15m/s$
The speed of man B is equal to,
$ \Rightarrow {V_B} = \dfrac{{15}}{3} m/s$
$ \Rightarrow {V_B} = 5m/s$.
Since the man A observes the man B moving in the perpendicular direction then the vector representation can be used to solve this further.

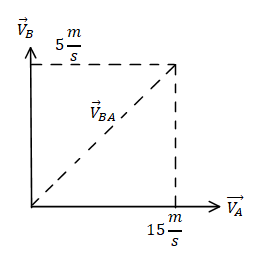
Let resultant velocity of the velocity of man B with respect to man A is given by ${V_{BA}}$.
The magnitude of the speed of the man B observed from man A is given by.
Applying Pythagoras we get,
$ \Rightarrow {V_{BA}} = \sqrt {{5^2} + {{15}^2}} $
$ \Rightarrow {V_{BA}} = \sqrt {25 + 225} $
$ \Rightarrow {V_{BA}} = \sqrt {250} $
$ \Rightarrow {V_{BA}} = 5\sqrt {10} m/s$.
The speed of man B with respect to man A is equal to ${V_{BA}} = 5\sqrt {10} m/s$ and is away from the car at some angle.

The correct answer for this problem is option B.

Note: The vector addition and subtraction is very useful in solving problems like these and therefore it is advised for students to understand and remember the formula of the vector addition and vector substation and also the magnitude calculation of the same.