
A point charge is placed at the corner of a cube. The electric flux through the shaded surface is

(A)
(B)
(C)
(D)
Answer
154.2k+ views
1 likes
Hint: To solve this question, we need to use the Gauss theorem. For that we need to place the charge symmetrically within a closed surface, which must be generated by using the similar cubes as given in the question. Then, using the unitary method, we can determine the required flux.
Complete step by step solution:
We know from the gauss theorem that the total flux passing through a closed surface, within which a charge is enclosed, is given by
In the figure given in the question, the charge is placed at the corner of the cube, not within the cube. So we need to enclose it within a closed surface, so that the Gauss theorem can be applied.
So we place three more cubes, similar to the one given in the question around the given charge, as shown in the figure below.
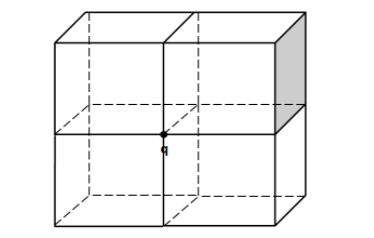
As we can clearly see in the above figure, that now also the charge is not enclosed. This is because the charge is kept at the outer surface of the cuboid, not within it. So we have to take a similar bigger cuboid and keep it on the outer surface of the cuboid, so that the charge is enclosed within the bigger cube thus formed.
As the charge is enclosed in the bigger cube, so the total flux passing through it is given by
Since the bigger cube is composed of two cuboids, and the charge is symmetrically placed with respect to both of them, so the flux passing through each of the cuboids is
From (1)
So the flux passing through the cuboid shown in the above figure is equal to
Now, the cuboid is composed of eight cubes, and the charge is placed symmetrically with respect to all of them, so the flux passing through each of these four cubes is given by
From (2)
So the flux passing through the given cube is equal to
We know that a cube has a total of six faces. But the flux will not pass through each of these faces.
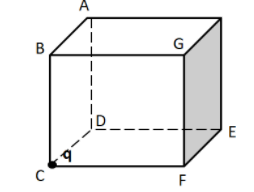
As can be seen in the above figure, the point charge placed at the corner C is touching the three faces of the cube, namely ABCD, CDEF, and BGFC. So the electric field through the charge will not be able to cut through these three faces. Therefore, the flux through all of these three faces will be equal to zero. Hence, the flux will pass through the rest of the three faces of the cube. Therefore, the flux passing through each of these three faces is given by
From (3)
The shaded region given in the question is nothing but a face of the cube. So the flux passing through the shaded region is equal to
Hence, the correct answer is option (C).
Note: We should not forget the fact that the flux passing through the surfaces, which the charge is touching, will be equal to zero. The electric field through the point charge will be parallel to these faces.
Complete step by step solution:
We know from the gauss theorem that the total flux passing through a closed surface, within which a charge is enclosed, is given by
In the figure given in the question, the charge is placed at the corner of the cube, not within the cube. So we need to enclose it within a closed surface, so that the Gauss theorem can be applied.
So we place three more cubes, similar to the one given in the question around the given charge, as shown in the figure below.

As we can clearly see in the above figure, that now also the charge is not enclosed. This is because the charge is kept at the outer surface of the cuboid, not within it. So we have to take a similar bigger cuboid and keep it on the outer surface of the cuboid, so that the charge is enclosed within the bigger cube thus formed.
As the charge is enclosed in the bigger cube, so the total flux passing through it is given by
Since the bigger cube is composed of two cuboids, and the charge is symmetrically placed with respect to both of them, so the flux passing through each of the cuboids is
From (1)
So the flux passing through the cuboid shown in the above figure is equal to
Now, the cuboid is composed of eight cubes, and the charge is placed symmetrically with respect to all of them, so the flux passing through each of these four cubes is given by
From (2)
So the flux passing through the given cube is equal to
We know that a cube has a total of six faces. But the flux will not pass through each of these faces.

As can be seen in the above figure, the point charge placed at the corner C is touching the three faces of the cube, namely ABCD, CDEF, and BGFC. So the electric field through the charge will not be able to cut through these three faces. Therefore, the flux through all of these three faces will be equal to zero. Hence, the flux will pass through the rest of the three faces of the cube. Therefore, the flux passing through each of these three faces is given by
From (3)
The shaded region given in the question is nothing but a face of the cube. So the flux passing through the shaded region is equal to
Hence, the correct answer is option (C).
Note: We should not forget the fact that the flux passing through the surfaces, which the charge is touching, will be equal to zero. The electric field through the point charge will be parallel to these faces.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Young's Double Slit Experiment Step by Step Derivation

JEE Atomic Structure and Chemical Bonding important Concepts and Tips

JEE Amino Acids and Peptides Important Concepts and Tips for Exam Preparation

JEE Electricity and Magnetism Important Concepts and Tips for Exam Preparation

Chemical Properties of Hydrogen - Important Concepts for JEE Exam Preparation

JEE Energetics Important Concepts and Tips for Exam Preparation

Trending doubts
If a wire of resistance R is stretched to double of class 12 physics JEE_Main

Explain the construction and working of a GeigerMuller class 12 physics JEE_Main

Wheatstone Bridge for JEE Main Physics 2025

The force of interaction of two dipoles if the two class 12 physics JEE_Main

Three charges sqrt 2 mu C2sqrt 2 mu Cand sqrt 2 mu class 12 physics JEE_Main

The potential of A is 10V then the potential of B is class 12 physics JEE_Main

Other Pages
JEE Advanced 2025 Revision Notes for Mechanics

Ideal and Non-Ideal Solutions Raoult's Law - JEE

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

An uncharged sphere of metal is placed inside a charged class 12 physics JEE_Main

Three mediums of refractive indices mu 1mu 0 and mu class 12 physics JEE_Main

A signal of 5kHz frequency is amplitude modulated on class 12 physics JEE_Main




