Class 9 Maths Revision Notes for Statistics of Chapter 12 - Free PDF Download
FAQs on Statistics Class 9 Notes CBSE Maths Chapter 12 (Free PDF Download)
1. What is the meaning of Statistics according to NCERT Solutions for Chapter 12 of Class 9 Maths?
Statistics is a branch of Mathematics that deals with a large number of given raw data and is associated with the collection, arrangement, tabulation, calculation, interpretation, and finally, the analysis of that data. These processes are carried out by certain pre-developed statistical tools and methods that help the users efficiently conduct their analysis. In Class 9, you will be introduced to this concept and must concentrate on it properly to solve the problems accurately.
2. What is raw data in Chapter 12 of Statistics of Class 9?
When it comes to statistics, you will come across the term raw or numerical data. This is the original or primary data that the researcher collects. Raw data is unarranged scores of a group or an individual. Raw data must be arranged and interpreted for detailed analysis and present a conclusion easily understood by the general population. You can refer to the Vedantu app or website for more information on Chapter 12 of Class 9 Maths.
3. According to Chapter 12 of Class 9 Maths, what is the class boundary?
In Statistics, when you have a set of data, you also have lower limits and upper limits to the class from that data set. Now these upper and lower limits are nothing but the two ends of an entire set of scores or values given for analysis. The average is calculated for these two limits then what you will get is the class boundary. This important aspect of statistical analysis helps you interpret and draw results.
4. What is the range of data according to Chapter 12 of Class 9 Maths?
You will learn about the data range when you study the Statistics chapter from Class 9 Maths Chapter 12 regions notes provided by Vedantu. This data is nothing but the scores and values that are primarily collected for interpretation. Now, this data is not endless. They have a starting and ending point. When you are given raw data, you need first to arrange that data properly, and then you will notice the two extreme limits of that data set. The entire data between these limits is called the range of data in statistics.
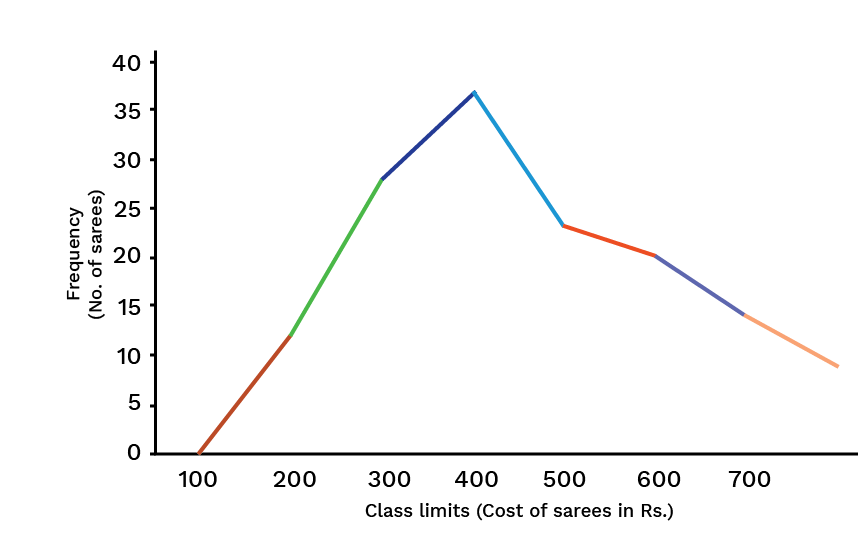
5. How are frequency polygons drawn in Statistics?
In Statistics, frequency polygons can be drawn independently without drawing histograms. For this, you just need the midpoints of the class intervals used in the data. The midpoints of the class intervals are also known as class marks. To find the class marks, you just need to calculate the sum of the upper and the lower limit of a class- interval and divide it by 2.