Question

Two bulbs rated 220 V, 100 W and 220 V, 60 W are connected in series across a voltage supply V. The bulbs can be assumed as purely resistive and they cannot withstand a voltage more than their voltage rating. Select the incorrect alternative:

A. When V=220 V, the total power output is 37.5W
B. When V=220 V, $P_{60}$>$P_{100}$ (P is power)
C. When V=440 V, 60 W bulb will fuse.
D. The maximum safe value of V is 400V

Answer 1

Hint: We have to verify each option and check for its correctness. For the first option, determine the individual resistances of the bulb, and find the total power using the effective resistance of the circuit by taking P proportional to square of V and inversely proportional to R. As for the second option, you can heuristically determine the relation between resistance and power and arrive at the appropriate conclusion.
The same goes for the third option, by keeping in mind that in a series circuit, more voltage is borne by that component with the higher resistance. And as for the final option, ensuring that the maximum voltage drop across the 60W bulb must not exceed 220V, calculate the safe supply voltage. To this end, the option that does not yield a result consistent with its claim will be the appropriate choice.
Formula used:
Power $P=\dfrac{V^2}{R} = I^2 R$

Complete answer:
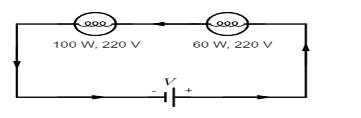
Let us verify the claim of each option and determine which one is the incorrect alternative.

A. When V=220 V, the total power output is 37.5W
Let us begin by first finding the individual resistances of the two bulbs.
We know that power $P = \dfrac{V^2}{R} \Rightarrow R = \dfrac{V^2}{P}$, where V = 220 V
$R_{100} = \dfrac{220^2}{100} = 484\Omega$
$R_{60} = \dfrac{220^2}{60} = 806.667\Omega$
The effective resistance of the two bulbs in series will be:
$R_{eff} = R_{100} +R_{60} = 484 + 806.667 = 1290.667\Omega$.
Therefore, the total power output of the circuit is given by:
$P_{total} = \dfrac{V^2}{R_{eff}} = \dfrac{220^2}{1290.667} = \dfrac{48400}{1290.667} = 37.5\;W$
Therefore, the first option is correct.

B. When V=220 V, $P_{60}$>$P_{100}$ (P is power)
From the previous evaluation, we have $R_{100}$<$R_{60}$.
Now, in a series circuit, the current flowing through all circuit components will be the same.
From previous evaluation, we have, power $P = \dfrac{V^2}{R} = \dfrac{(IR)^2}{R} = I^2 R$
Following this we have:
$P_{100} = I^2 R_{100} = 484I^2$
$P_{60} = I^2 R_{60} = 806.667I^2$
This means that $P_{60}$>$P_{100}$.
Therefore, the second option is also correct.

C. When V=440 V, 60 W bulb will fuse
In a series combination, the bulb with a higher resistance will handle more voltage and hence will be the first to fuse.
Thus, the 60W will fuse first. Let us determine the voltage at which the bulb fuses:
$V_{60} = \dfrac{R_{60}}{R_{100}+R_{60}}\times 440 = \dfrac{806.667}{1290.667} \times 440 = 275\;V$
Therefore, the third option is also correct.
Now, this leaves us with the fourth alternative to be the incorrect alternative, so let us see why.

D. The maximum safe value of V is 400V
For the 60W bulb to not fuse, the voltage drop across it should not exceed 220V, i.e. it should be $\leq$ 220V:
$\dfrac{R_{60}}{R_{100}+R_{60}}\times V \leq 220$
$\Rightarrow \dfrac{806.667}{1290.667} \times V \leq 220$
$\Rightarrow V \leq \dfrac{220 \times 1290.667}{806.667} = 351.99 \approx 352\;V$
Therefore, the fourth option is incorrect.

So, the correct answer is “Option A”.

Note:
Remember that a series circuit is a voltage divider whereas a parallel circuit is a current divider. We add up all the resistances in a series circuit because the resistances are arranged in such a way that the current has only one path to take. However, when resistances are connected in parallel, there are multiple paths for the current to pass through but have the same potential difference across them. This results in a net resistance that is lower than any of the individual resistances.