Answer
35.4k+ views
Hint:- In this question first we have to find the value of the point of intersection of the family of straight lines then apply Pythagoras theorem to get the required answer.
Complete step-by-step solution -
The equation of the family of lines is,
\[(4a+3)x-(a+1)y-(2a+1)=0\]
Expanding this, we get
\[4ax+3x-ay-y-2a-1=0\]
Now regrouping them, we get
\[3x-y-1+a(4x-y-2)=0\]
Now this is of the form \[{{L}_{1}}+\lambda {{L}_{2}}=0\].
Let the point of intersection of these two lines, i.e., \[3x-y-1=0\]and \[4x-y-2=0\]be P.
So, the given family of lines will pass through this fixed point P, as shown below.
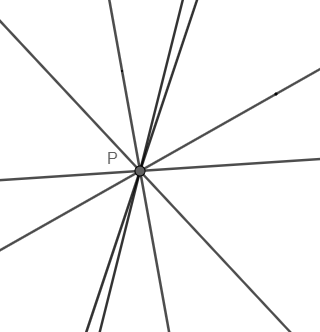
Now we will find the coordinates of point P.
Consider,
\[3x-y-1=0\]
\[3x=y+1\]
\[x=\dfrac{y+1}{3}........(i)\]
Substitute this value in other equation of line, we get
\[4x-y-2=0\]
\[4\left( \dfrac{y+1}{3} \right)-y-2=0\]
\[\dfrac{4y+4-3y-(3\times 2)}{3}=0\]
\[y+4-6=0\]
\[y=6-4=2\]
Substituting the value of ‘y’ in equation (i), we get
\[x=\dfrac{2+1}{3}=\dfrac{3}{3}=1\]
So, the point of intersection of the family of lines is \[P(1,2)\].
Now, there is a perpendicular drawn from the origin onto each line. Let the foot of the perpendicular on each line be \[A(x,y)\], as shown below.
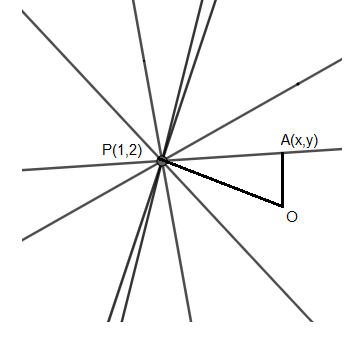
Now the points OPA will form a right-angled triangle in all the lines.
Applying Pythagoras theorem on \[\Delta OPA\], we get
\[O{{P}^{2}}=O{{A}^{2}}+A{{P}^{2}}.........(ii)\]
We know the distance between points \[({{x}_{1}},{{y}_{1}})\]and \[({{x}_{2}},{{y}_{2}})\]is given by the distance formula, i.e.,
\[\sqrt{{{({{x}_{2}}-{{x}_{1}})}^{2}}+{{({{y}_{2}}-{{y}_{1}})}^{2}}}\]
Applying the distance formula in equation (ii), we get
\[{{\left( \sqrt{{{(1-0)}^{2}}+{{(2-0)}^{2}}} \right)}^{2}}={{\left( \sqrt{{{(x-0)}^{2}}+{{(y-0)}^{2}}} \right)}^{2}}+{{\left( \sqrt{{{(x-1)}^{2}}+{{(y-2)}^{2}}} \right)}^{2}}\]
\[{{1}^{2}}+{{2}^{2}}={{x}^{2}}+{{y}^{2}}+{{(x-1)}^{2}}+{{(y-2)}^{2}}\]
Expanding, we get
\[1+4={{x}^{2}}+{{y}^{2}}+({{x}^{2}}+1-2x)+({{y}^{2}}+4-4y)\]
\[5=2{{x}^{2}}+2{{y}^{2}}+1-2x+4-4y\]
As this is not matching with the options, we will multiply the above equation with \[2\], we get
\[10=4{{x}^{2}}+4{{y}^{2}}+2-4x+8-8y\]
Grouping them, we get
\[4{{x}^{2}}-4x+2+4{{y}^{2}}-8y+8=10\]
Now we will try to convert this into \[{{(a+b)}^{2}}\]form, as in options.
\[\left( 4{{x}^{2}}-4x+1 \right)+1+4({{y}^{2}}-2y+1)+4=10\]
We know, \[\left( {{a}^{2}}-2ab+{{b}^{2}} \right)={{(a+b)}^{2}}\], so above equation can be re-written as,
\[{{\left( 2x-1 \right)}^{2}}+4{{(y-1)}^{2}}=10-4-1\]
\[{{\left( 2x-1 \right)}^{2}}+4{{(y-1)}^{2}}=5\]
So, the locus of the foot of the perpendicular from the origin on each member of the family \[(4a+3)x-(a+1)y-(2a+1)=0\] is \[{{\left( 2x-1 \right)}^{2}}+4{{(y-1)}^{2}}=5\].
Hence, the correct answer is option (d).
Note: Another method to find the answer is, instead of using Pythagoras theorem; we can find the slope of the equation of perpendicular line from the origin to a point. Then applying the formula, $y=mx+c$. But this would be a lengthy process.
Complete step-by-step solution -
The equation of the family of lines is,
\[(4a+3)x-(a+1)y-(2a+1)=0\]
Expanding this, we get
\[4ax+3x-ay-y-2a-1=0\]
Now regrouping them, we get
\[3x-y-1+a(4x-y-2)=0\]
Now this is of the form \[{{L}_{1}}+\lambda {{L}_{2}}=0\].
Let the point of intersection of these two lines, i.e., \[3x-y-1=0\]and \[4x-y-2=0\]be P.
So, the given family of lines will pass through this fixed point P, as shown below.

Now we will find the coordinates of point P.
Consider,
\[3x-y-1=0\]
\[3x=y+1\]
\[x=\dfrac{y+1}{3}........(i)\]
Substitute this value in other equation of line, we get
\[4x-y-2=0\]
\[4\left( \dfrac{y+1}{3} \right)-y-2=0\]
\[\dfrac{4y+4-3y-(3\times 2)}{3}=0\]
\[y+4-6=0\]
\[y=6-4=2\]
Substituting the value of ‘y’ in equation (i), we get
\[x=\dfrac{2+1}{3}=\dfrac{3}{3}=1\]
So, the point of intersection of the family of lines is \[P(1,2)\].
Now, there is a perpendicular drawn from the origin onto each line. Let the foot of the perpendicular on each line be \[A(x,y)\], as shown below.

Now the points OPA will form a right-angled triangle in all the lines.
Applying Pythagoras theorem on \[\Delta OPA\], we get
\[O{{P}^{2}}=O{{A}^{2}}+A{{P}^{2}}.........(ii)\]
We know the distance between points \[({{x}_{1}},{{y}_{1}})\]and \[({{x}_{2}},{{y}_{2}})\]is given by the distance formula, i.e.,
\[\sqrt{{{({{x}_{2}}-{{x}_{1}})}^{2}}+{{({{y}_{2}}-{{y}_{1}})}^{2}}}\]
Applying the distance formula in equation (ii), we get
\[{{\left( \sqrt{{{(1-0)}^{2}}+{{(2-0)}^{2}}} \right)}^{2}}={{\left( \sqrt{{{(x-0)}^{2}}+{{(y-0)}^{2}}} \right)}^{2}}+{{\left( \sqrt{{{(x-1)}^{2}}+{{(y-2)}^{2}}} \right)}^{2}}\]
\[{{1}^{2}}+{{2}^{2}}={{x}^{2}}+{{y}^{2}}+{{(x-1)}^{2}}+{{(y-2)}^{2}}\]
Expanding, we get
\[1+4={{x}^{2}}+{{y}^{2}}+({{x}^{2}}+1-2x)+({{y}^{2}}+4-4y)\]
\[5=2{{x}^{2}}+2{{y}^{2}}+1-2x+4-4y\]
As this is not matching with the options, we will multiply the above equation with \[2\], we get
\[10=4{{x}^{2}}+4{{y}^{2}}+2-4x+8-8y\]
Grouping them, we get
\[4{{x}^{2}}-4x+2+4{{y}^{2}}-8y+8=10\]
Now we will try to convert this into \[{{(a+b)}^{2}}\]form, as in options.
\[\left( 4{{x}^{2}}-4x+1 \right)+1+4({{y}^{2}}-2y+1)+4=10\]
We know, \[\left( {{a}^{2}}-2ab+{{b}^{2}} \right)={{(a+b)}^{2}}\], so above equation can be re-written as,
\[{{\left( 2x-1 \right)}^{2}}+4{{(y-1)}^{2}}=10-4-1\]
\[{{\left( 2x-1 \right)}^{2}}+4{{(y-1)}^{2}}=5\]
So, the locus of the foot of the perpendicular from the origin on each member of the family \[(4a+3)x-(a+1)y-(2a+1)=0\] is \[{{\left( 2x-1 \right)}^{2}}+4{{(y-1)}^{2}}=5\].
Hence, the correct answer is option (d).
Note: Another method to find the answer is, instead of using Pythagoras theorem; we can find the slope of the equation of perpendicular line from the origin to a point. Then applying the formula, $y=mx+c$. But this would be a lengthy process.
Recently Updated Pages
To get a maximum current in an external resistance class 1 physics JEE_Main

f a body travels with constant acceleration which of class 1 physics JEE_Main

If the beams of electrons and protons move parallel class 1 physics JEE_Main

Let f be a twice differentiable such that fleft x rightfleft class 11 maths JEE_Main

Find the points of intersection of the tangents at class 11 maths JEE_Main

For the two circles x2+y216 and x2+y22y0 there isare class 11 maths JEE_Main

Other Pages
Oxidation state of S in H2S2O8 is A 6 B 7 C +8 D 0 class 12 chemistry JEE_Main

Explain the construction and working of a GeigerMuller class 12 physics JEE_Main

The strongest oxidising agent among the following is class 11 chemistry JEE_Main

A closed organ pipe and an open organ pipe are tuned class 11 physics JEE_Main

Differentiate between homogeneous and heterogeneous class 12 chemistry JEE_Main

Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main



