




Angle of Deviation in Prism Important Concepts for JEE Main 2025
The angle of deviation in a prism is the angle between the direction of incident light and the emergent ray as it passes through the prism. It depends on factors such as the refractive index of the prism material, the angle of incidence, and the prism's angle. This concept is essential in understanding refraction, dispersion, and the behaviour of light in optical systems.
For JEE Main 2025, mastering the angle of deviation is crucial for solving questions related to ray optics and refraction through prisms. You can visit Vedantu website and get other useful study materials. These materials were made by our teachers and experts and are updated according to the JEE Main syllabus.
JEE Main Physics Syllabus JEE Main Physics Revision Notes JEE Main Physics Important Questions JEE Main Physics Difference Between JEE Main Physics Question Papers
What is The Angle of Deviation in Prism?
A prism can be defined as a wedge-shaped body that is made from a refracting medium. It is bound by two plane faces which are placed at an incline with each other at some angle. The two plane faces of the prism are called the refracting faces. The angle between the two faces is known as the angle of the prism. It is also known as the refracting angle. The faces of a prism can be parallelograms or rectangles without their bases. There are a variety of prisms and depending on the shape, the base of a prism could be a triangle, square, rectangle, or any n-sided polygon in general.
Key Facts About Prisms
Inside a prism, the refracted ray bends towards the base.
When the prism is in the position of minimum deviation, the refracted ray inside runs parallel to the base, and the light passes symmetrically through the prism.
For minimum deviation, the angle of incidence should be $90^\circ$.
In an equilateral prism $(A = 60^\circ)$, during minimum deviation, the refracted angles ($r_1$ and $r_2$) are $30^\circ$ each.
If the prism angle A exceeds twice the critical angle (2C) of the glass, no emergent ray occurs. This critical value of A is called the limiting angle of the prism.
Dispersion of Light
When white light passes through a prism, it separates into its constituent colours or wavelengths. This process is known as dispersion.
Dispersion occurs because the refractive index of a material changes slightly with different wavelengths of light.
Angular Dispersion ($\theta$)
Angular dispersion is the difference between the deviations of the extreme colours, red and violet, after passing through the prism.
Dispersive Power ($\nu$)
Dispersive power is the ratio of angular dispersion between two colours to the deviation of the mean ray (typically yellow) caused by the prism: $\nu = \dfrac{\Delta \theta}{\delta_m}$ where $\Delta \theta$ is the angular dispersion and $\delta_m$ is the deviation of the mean ray.
For small prism angles (A), dispersive power depends only on the material of the prism, not on its angle.
Dispersive power ($\nu$) is always zero or greater. It is zero in a vacuum, nearly zero in air, and greater than zero in other refractive materials.
Before deriving the expression of the angle of deviation in the prism, it is important to understand what happens to a beam of light that is incident on one of the faces of the prism. A light that is incident on a surface of the prism undergoes refraction at both the surfaces of the prism and is deviated from the original path that was supposed to follow. There is a deviation in the path of the beam of light due to refraction from the faces of the prism. The angle of deviation is the angle between the original path that the beam should have followed and the deviated beam. It can also be defined as the angle through which the incident ray gets deviated when passing through a prism.
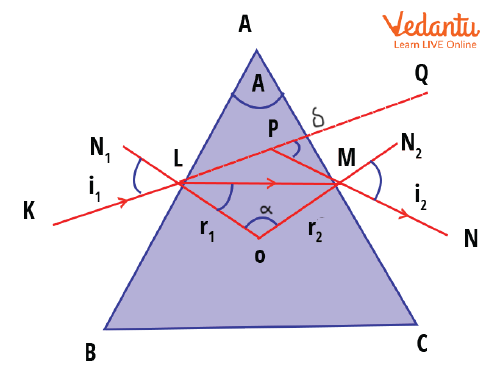
Image: Angle of deviation in a prism
From the above diagram, we can see that a ray KL is incident on the face AB of the prism that we have. KL is incident on point L from where N1LO is the normal and i1 can be termed as the angle of incidence. Now the light will be refracted from this surface and since the refraction takes place from air to glass, the ray LM will bend towards the normal such that r1 will be the angle of refraction. Considering µ to be the refractive index of the material of the prism, we can write using Snell’s law,
$\mu=\dfrac {\sin {i_1}}{\sin {r_1}}$
Now the refracted ray LM will be incident on another face AC of the prism at point M. At this point, N2MO is normal, so r2 will be the angle of incidence. Now the refraction is taking place from the material to air, that is from a denser medium to a rarer medium. So the emergent ray MN will bend away from the normal and i2 will be the angle of emergence.
As discussed above, without the prism, the incident ray KL would have followed a straight path but due to the presence of the prism, it changes its path to PMN. So the angle QPN, or δ is the angle of deviation. It is the angle through which the incident ray deviates when passing through a prism.
From the elementary analysis of the angles, we can write,
$\begin{align} &\delta=i_{1}-r_{1}+i_{2}-r_{2} \\ &\delta=i_{1}+i_{2}-\left(r_{1}+r_{2}\right) \end{align}$
We can also write from triangle LOM,
$\alpha=180^{\circ}-\left(r_{1}+r_{2}\right)$
Also, we know that
$\angle A L O=\angle A M O=90^{\circ}$
In quadrilateral ALOM we can write,
$\begin{align} &\angle A+\angle A L O+\alpha+\angle A M O=360^{\circ} \\ &\angle A+90^{\circ}+180^{\circ}-\left(r_{1}+r_{2}\right)+90^{\circ}=360^{\circ} \\ &\angle A=360^{\circ}-360^{\circ}+\left(r_{1}+r_{2}\right) \\ &\angle A=\left(r_{1}+r_{2}\right) \end{align}$
Using this value of angle A we can write,
$\begin{align} &\delta=i_{1}+i_{2}-A \\ &\delta+A=i_{1}+i_{2} \end{align}$
The above equation shows the relationship between the angle of prism and the angle of deviation. This equation shows that the angle of deviation is dependent on the nature of the material as well as the angle of incidence.
Minimum Angle of Deviation and Refractive Index
From experimental data, the curve given below has been plotted. The curve has been plotted between the angle of deviation and angle of incidence. One thing to be noted from the plot is that the angle of deviation has a minimum value $\delta_m$ only for one value of angle of incidence. This minimum value is known as the angle of minimum deviation. It is the angle of deviation when a ray of light passes through the prism with minimum deviation.
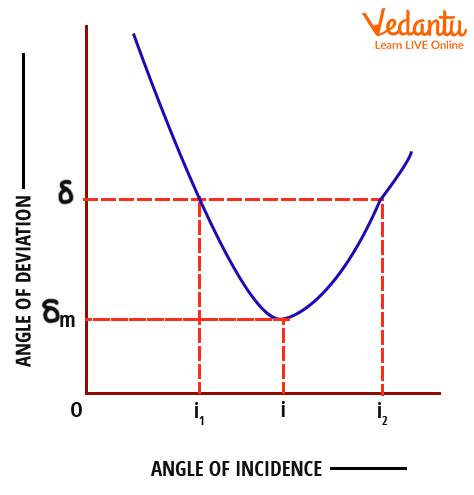
Image: Plot between Angle of Deviation and Angle of incidence
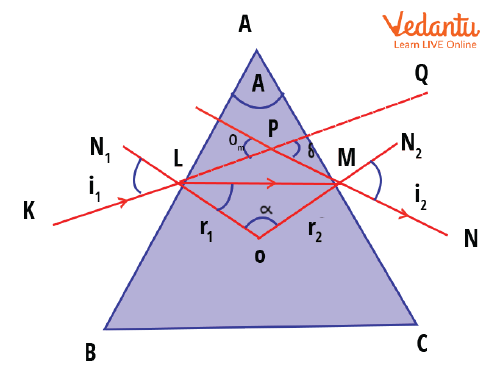
Image: Angle of minimum deviation in prism
The above figure shows the prism in a minimum deviation position. Now if any plane mirror is placed in the path of the emergent ray, in this case, MN then the ray will retrace its original path. It will suffer a minimum deviation of $\delta_m$.
For the minimum deviation position, we have from the above graph,
$\angle i_{1}=\angle i_{2}$
We can also say,
$\angle r_{1}=\angle r_{2}=\angle r$
We can also write,
$\angle A L M=\angle L M A=90^{\circ}-\angle r$
This means that AL=LM and so, LM is parallel to BC.
From all this, we can infer that the ray which suffers minimum deviation passes symmetrically through the prism and is parallel to the base.
Now we can write,
$\angle A=\angle r_{1}+\angle r_{2}$
Since $\angle r_{1}=\angle r_{2}=\angle r$,
A = 2r or
$r=\dfrac{A}{2}$
And since $\angle i_{1}=\angle i_{2}$, we let them be i1. We can write,
$\begin{align} &2 i_{1}=A+\delta m \\ &i_{1}=\dfrac{A+\delta m}{2} \end{align}$
Now we know that,
$\mu=\dfrac {\sin {i_1}}{\sin {i_2}}$
From the above equations, we can write,
$\mu=\dfrac{\sin \left(\dfrac{(A+\delta m)}{2}\right)}{\sin \left(\dfrac{A}{2}\right)}$
This is the relationship between the angle of minimum deviation and the refractive index of the material of the prism.
Numerical Examples on Angle of Deviation
Example 1: If the angle of minimum deviation of a light ray passing through a prism of refracting angle 60° is 30°, then find the refractive index of the prism.
Solution:
We know that,
$\mu=\dfrac{\sin \left(\dfrac{(A+\delta m)}{2}\right)}{\sin \left(\dfrac{A}{2}\right)}$
We have,
A=60° and $\delta_m$ = 30°
Substituting these values in the equation gives,
$\begin{align} &\mu=\dfrac{\sin \left(\dfrac{60^{\circ}+30^{\circ}}{2}\right)}{\sin \left(\dfrac{60^{\circ}}{2}\right)} \\ &\mu=\dfrac{\sin 45^{\circ}}{\sin 30^{\circ}} \\ &\mu=1.414 \end{align}$
So the refractive index of the material is 1.414.
Example 2: The refractive index of the material of a prism is 1.5. When the prism is placed in a minimum deviation position, the angle of incidence is 45º. Calculate the angle of prism and the angle of minimum deviation.
Solution:
We have $\mu=1.5,~i=45^{\circ},~A=?,~\delta m=?$
We know that for minimum deviation,
$\begin{align} &2 i=A+\delta_{m} \\ &2 \times 45^{\circ}=A+\delta_{m} \\ &90^{\circ}=A+\delta_{m} \end{align}$
Also, we know that
$\mu=\dfrac{\sin \left(\dfrac{(A+\delta m)}{2}\right)}{\sin \left(\dfrac{A}{2}\right)}$
Substituting for 90° = A + $\delta_m$ we get,
$\begin{align} &\sin \left(\dfrac{A}{2}\right)=\dfrac{\sin \left(45^{\circ}\right)}{1.5} \\ &A=2 \cdot \sin ^{-1}(0.471) \\ &A \approx 2 \cdot 28^{\circ} \\ &A \approx 56^{\circ} \end{align}$
Substituting this value of A in 90° = A + $\delta_m$, we get,
$\begin{align} &90^{\circ}-56^{\circ}=\delta_m \\ &34^{\circ}=\delta_m \end{align}$
So the angle of the prism is 56° and the angle of minimum deviation is 34°.
Significance of Angle of Deviation in Prism for JEE Main 2025
The angle of deviation is a critical concept in ray optics, explaining how light bends and disperses when passing through a prism.
It forms the basis for understanding dispersion, minimum deviation, and the relationship between refractive index and wavelength.
This topic is widely used in analysing optical instruments and light behaviour in materials.
Questions on the angle of deviation in a prism are common in the Ray Optics and Wave Optics section, appearing in 1-2 questions.
These questions often involve calculating deviation, understanding its dependence on the angle of incidence, and solving numerical problems, making it an important topic for securing marks.
Tips to Prepare Angle of Deviation in Prism for JEE Main 2025
Understand the Basics: Learn how light refracts through a prism and how the angle of deviation is defined.
Memorise Key Formulas: Familiarise yourself with formulas like δ=i1+i2−A\delta = i_1 + i_2 - Aδ=i1+i2−A and conditions for minimum deviation.
Practice Numerical Problems: Solve problems involving different angles of incidence and refractive indices to strengthen your application skills.
Study the Relation to Wavelength: Understand how dispersion affects deviation and how it varies for different wavelengths of light.
Draw Ray Diagrams: Practice ray diagrams for prisms to visualise the path of light and understand deviation geometrically.
Revise Minimum Deviation Concept: Focus on the condition for minimum deviation and its importance in determining the refractive index of the prism material.
Use Reliable Resources: Refer to the FREE PDF or standard study materials for detailed explanations and solved examples.
Solve Previous Year Questions: Practice JEE Main questions to understand the type of problems frequently asked.
Take Mock Tests: Simulate exam conditions to test your understanding and improve accuracy in solving questions on this topic.
Conclusion
A prism is a wedge-shaped body constructed of a refracting medium with two plane faces that are at an angle to each other. The angle between the faces of the prism is known as the angle of the prism. Any ray of light that is incident on the prism deviates from its path and the angle between the original light ray and the deviated ray is known as the angle of deviation. The formula for the angle of deviation is given as, $\delta=i_{1}+i_{2}-A$
A prism's minimum angle of deviation is the angle of deviation at which a ray of light passes through it with the least amount of deviation. The relationship between the minimum angle of deviation and the refractive index of the prism is given as,
$\mu=\dfrac{\sin \left(\dfrac{(A+\delta m)}{2}\right)}{\sin \left(\dfrac{A}{2}\right)}$
JEE Main 2025 Subject-Wise Important Chapters
The JEE Main 2025 subject-wise important chapters provide a focused strategy for Chemistry, Physics, and Maths. These chapters help students prioritise their preparation, ensuring they cover high-weightage topics for better performance in the exam.
Check Other Important Links for JEE Main and JEE Advanced 2025
JEE 2025 needs well-structured study materials. Here are some valuable resources to help you plan your preparation and practice efficiently.
FAQs on Learn About Angle Of Deviation In Prism: JEE Main Physics 2025
1. What is angle of deviation in prism?
The angle of deviation in a prism is the angle between the direction of the incident light ray and the emergent light ray after refraction through the prism.
2. What is the angle of deviation in prism formula?
The formula for angle of deviation is δ=i1+i2−A\delta = i_1 + i_2 - Aδ=i1+i2−A, where i1i_1i1 and i2i_2i2 are the angles of incidence and emergence, and AAA is the prism angle.
3. What is the minimum angle of deviation in prism?
The minimum angle of deviation occurs when the light ray passes symmetrically through the prism, and it is used to determine the refractive index of the prism material.
4. Can you explain the derivation of angle of deviation in prism?
The derivation involves applying Snell’s Law at both refracting surfaces of the prism and using geometric relationships to express δ\deltaδ in terms of i2, and A. Learn what is angle of deviation in prism better explained by our experts so download the PDF version for the better understanding of chapter.
5. What does the angle of deviation in prism graph represent?
The graph of angle of deviation versus angle of incidence shows that deviation initially decreases, reaches a minimum, and then increases as the angle of incidence increases.
6. How is the minimum angle of deviation in prism formula derived?
The minimum angle of deviation in prism formula is μ=sin[(A+δm)/2]sin(A/2)\mu = \frac{\sin[(A + \delta_m)/2]}{\sin(A/2)}μ=sin(A/2)sin[(A+δm)/2], where μ\muμ is the refractive index, A is the prism angle, and δm\delta_mδm is the minimum deviation.
7. Does Vedantu provide a detailed derivation of angle of deviation in prism for JEE Main 2025?
Yes, Vedantu offers a FREE PDF with a step-by-step derivation of the angle of deviation in prism, along with solved examples and diagrams for JEE Main 2025 preparation.
8. How is the angle of deviation in prism derivation useful for JEE Main?
The angle of deviation in prism derivation helps in solving numerical problems related to minimum deviation, refractive index calculation, and understanding the behaviour of light through a prism.
9. Why is the minimum angle of deviation in prism important in ray optics?
It is significant because it provides a way to calculate the refractive index of the prism material and explains the conditions for symmetric refraction.
10. What are common questions on angle of deviation in prism for JEE Main 2025?
Questions typically involve calculating deviation, finding minimum deviation, deriving formulas, and interpreting deviation graphs, making it an essential topic in ray optics.

































