Question

Let $\overrightarrow a ,\overrightarrow b ,\overrightarrow c $be three unit vectors such that $\overrightarrow a \times \left( {\overrightarrow b \times \overrightarrow c } \right) = \dfrac{{\sqrt 3 }}{2}\left( {\overrightarrow b + \overrightarrow c } \right)$. If $\overrightarrow b $ is not parallel to $\overrightarrow c $, then the angle between $\overrightarrow a $ and $\overrightarrow b $ is:
A) $\dfrac{{3\pi }}{4}$
B) $\dfrac{\pi }{2}$
C) $\dfrac{{2\pi }}{3}$
D) $\dfrac{{5\pi }}{6}$

Answer 1

Hint:We are given that $\overrightarrow a ,\overrightarrow b ,\overrightarrow c $be three unit vectors, so, $|\overrightarrow a | = |\overrightarrow b | = |\overrightarrow c | = 1$. And we know that $\overrightarrow a \times \left( {\overrightarrow b \times \overrightarrow c } \right) = \left( {\overrightarrow a .\overrightarrow c } \right)\overrightarrow b - \left( {\overrightarrow a .\overrightarrow b } \right)\overrightarrow c $. This is the theorem. So on further comparing this equation, you will get the answer.

Complete step-by-step answer:
So, according to the question, we are given that $\overrightarrow a ,\overrightarrow b ,\overrightarrow c $be three unit vectors such that $\overrightarrow a \times \left( {\overrightarrow b \times \overrightarrow c } \right) = \dfrac{{\sqrt 3 }}{2}\left( {\overrightarrow b + \overrightarrow c } \right)$.
So from this statement, it is clear that the magnitude of $\overrightarrow a ,\overrightarrow b ,\overrightarrow c $ are equal to $1$.
$|\overrightarrow a | = |\overrightarrow b | = |\overrightarrow c | = 1$
Or $a = b = c = 1$
A simple magnitude of $\overrightarrow a $ is written as $a$.
As we know that
$\overrightarrow a \times \left( {\overrightarrow b \times \overrightarrow c } \right) = \left( {\overrightarrow a .\overrightarrow c } \right)\overrightarrow b - \left( {\overrightarrow a .\overrightarrow b } \right)\overrightarrow c $
And it is given that $\overrightarrow a \times \left( {\overrightarrow b \times \overrightarrow c } \right) = \dfrac{{\sqrt 3 }}{2}\left( {\overrightarrow b + \overrightarrow c } \right)$
Let $\theta $ be the angle between $\overrightarrow a $ and $\overrightarrow c $.
Let $\beta $ be the angle between $\overrightarrow a $ and $\overrightarrow b $.

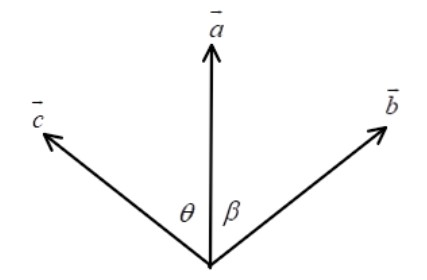
So, $\overrightarrow a .\overrightarrow c = ac\cos \theta $
Where $a = c = 1$
So, $\overrightarrow a .\overrightarrow c = \cos \theta $
Similarly, $\overrightarrow a .\overrightarrow b = ab\cos \beta $
Where $a = b = 1$
So, $\overrightarrow a .\overrightarrow b = \cos \beta $
So we got that
$
  \left( {\overrightarrow a .\overrightarrow c } \right)\overrightarrow b - \left( {\overrightarrow a .\overrightarrow b } \right)\overrightarrow c = \dfrac{{\sqrt 3 }}{2}\overrightarrow b + \dfrac{{\sqrt 3 }}{2}\overrightarrow c \\
  \left( {\cos \theta } \right)\overrightarrow b - \left( {\cos \beta } \right)\overrightarrow c = \dfrac{{\sqrt 3 }}{2}\overrightarrow b + \dfrac{{\sqrt 3 }}{2}\overrightarrow c \\
 $
Upon comparing both sides, we get
$\cos \theta = \dfrac{{\sqrt 3 }}{2} \Rightarrow \theta = {30^ \circ } \Rightarrow \theta = \dfrac{\pi }{6}$
And
$\cos \beta = - \dfrac{{\sqrt 3 }}{2} \Rightarrow \beta = \left( {\pi - \dfrac{\pi }{6}} \right) \Rightarrow \beta = \dfrac{{5\pi }}{6}$
So here we assume angle between $\overrightarrow a $ and $\overrightarrow b $ is $\beta $ that is $\dfrac{{5\pi }}{6}$

So, the correct answer is “Option D”.

Note:If $\overrightarrow b $ becomes parallel to $\overrightarrow c $, then, $\overrightarrow b \times \overrightarrow c = 0$. As $bc\sin \theta = \overrightarrow b \times \overrightarrow c $ and $\overrightarrow b ||\overrightarrow c $, so $\theta = 0$. Therefore, $\overrightarrow b \times \overrightarrow c = 0$. And $\left( {\overrightarrow b \times \overrightarrow c } \right)$ vector will be perpendicular to both $\overrightarrow b $ and $\overrightarrow c $. So, if $\overrightarrow a ,\overrightarrow b ,\overrightarrow c $ are in same plane then $\overrightarrow a .\left( {\overrightarrow b \times \overrightarrow c } \right) = \overrightarrow b \left( {\overrightarrow a \times \overrightarrow c } \right) = \overrightarrow c \left( {\overrightarrow b \times \overrightarrow a } \right) = 0$. As if $\overrightarrow a \bot \overrightarrow b $, then $\overrightarrow a .\overrightarrow b = 0$.