Question

A ray of light is incident on a thin film as shown in the figure. A and B are first two order reflected rays from first and second surface, and C & D are first two order final transmitted rays. Ray B and D undergo a phase change $\pi $. Choose the correct relation among the refractive indices.
(A) ${\mu _2} > {\mu _3} > {\mu _1}$
(B) ${\mu _3} > {\mu _2} > {\mu _1}$
(C) ${\mu _3} > {\mu _1} > {\mu _2}$
(D) None of these

Answer 1

Hint There are no phase changes during transmission. Also, a reflected ray has a phase change of $\pi $ when it is reflected from a medium that is denser than the travelling medium i.e. the medium the light ray travelled before it was reflected by the denser medium.
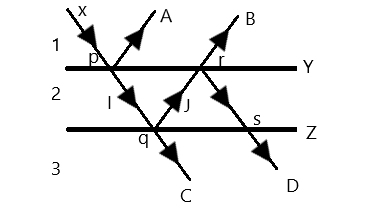
Use the above information to analyze the rays at the points p, q, r, s.

Complete step by step answer
We will move ray by ray from the incident ray X to the final ray D. A ray X is incident on surface Y at point P. It splits apart and is transmitted and another reflected. No information was given about the ray A, so no conclusion can be deduced from this alone. Now, the transmitted ray I, in medium 2, splits again at point q into a transmitted ray C and a reflected ray J. There can be no phase change in ray C since it is a transmitted ray. The reflected ray from point q (ray J) splits into a transmitted ray B and a reflected ray D. According to the question, ray B and D undergo a phase change of $\pi $. According to the concept, no phase change can occur for ray B (because it’s a transmitted ray) at point R, thus ray J must have a phase change of $\pi $. Since ray I has no phase change (because it’s a transmitted ray), ray J must have undergone phase change at point q. From the concept, medium 3 must therefore be denser than medium 2 which implies that ${\mu _3} > {\mu _2}$. Now, part of the phase-changed ray J was reflected as ray D. However, ray D also has a phase change of $\pi $ (with respect to the first incident ray X) which means that no other phase change occurred at point r. This happens if medium 1 is less dense than medium 2. Therefore, ${\mu _2} > {\mu _1}$.
Nothing occurs at point s
$\therefore {\mu _3} > {\mu _2} > {\mu _1}$
Therefore, the correct option is (B).

Note
The possible tripping point in the analysis is at point r. When ray D is reflected, you may think that a phase change of $\pi $ should occur because it was said that D has a phase change of $\pi $. Fortunately, a simple work around is to retain the fact that ray J already has a phase change of $\pi $ with respect to the initial incident ray X. If at point r, another phase change of $\pi $ where to occur, ray D would have a phase change of $2\pi $ or zero (no phase change) with respect to the incident ray X.