Question

When a pin is moved along the principal axis of a small concave mirror, the image position coincides with the object at a point 0.5 m the mirror. If the mirror is placed at a depth of 0.2 m in a transparent liquid, the same phenomenon occurs when the pin is placed 0.4 m from the mirror. Find the refractive index of the liquid.

Answer 1

Hint: When light rays travel from one medium to the other medium they will bend. If the observer is in one medium and if the object is in the other medium then the apparent object seen by the observer will be at a different position while the actual object will be at a different position. Same happens in the given question too.

Formula used:
${d_{app}} = \mu {d_{act}}$

Complete answer:
 Various positions of placing an object in front of a concave mirror gives us various positions of images.
If an object is placed between the pole and focus of the concave mirror then image will be formed on the other side of the mirror. That image formed can be enlarged or diminished and virtual.
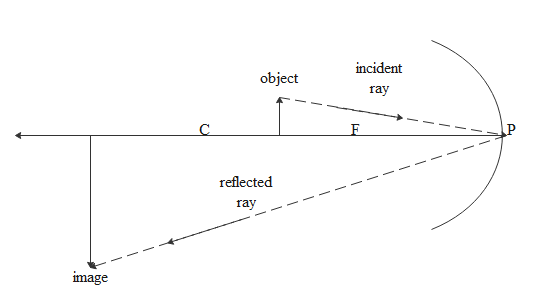
Now if we place an object between focus and center then the image is formed away from the center and the image will be real and inverted. The size of the image will be bigger than an object as shown in the diagram.

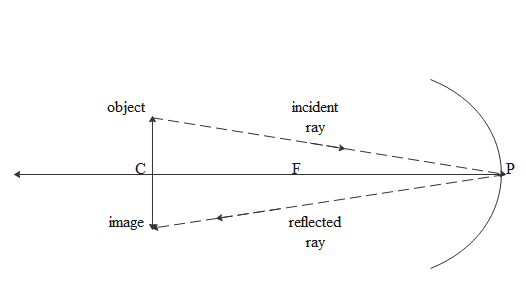
If the object is placed exactly at center the image also will be formed exactly at the center just as shown in the diagram below.

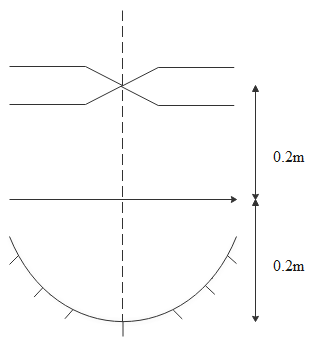
P denotes the pole and F denotes the focus and C denotes the center. Distance between pole and center is radius.
That means the radius of the mirror given is 0.5m as the image and object are at the same point when object distance is 0.5m.
Let the refractive index of liquid be $\mu $
Distance in the liquid from mirror to liquid surface is 0.2m(${d_1}$) which will remain same and actual distance from the liquid surface to the object is 0.2m and the apparent distance for this will be
${d_{app}} = \mu {d_{act}}$
$ \Rightarrow {d_{app}} = \mu (0.2)$
Now the sum of these distances must be equal to the radius.
${d_1} + {d_{app}} = 0.5$
$\eqalign{
  & \Rightarrow 0.2 + \mu (0.2) = 0.5 \cr
  & \Rightarrow \mu (0.2) = 0.5 - 0.2 \cr
  & \Rightarrow \mu (0.2) = 0.3 \cr
  & \Rightarrow \mu = \dfrac{3}{2} \cr} $
Hence the refractive index of liquid will be 1.5

Note:
When object is in rarer medium and observer is in denser medium then object seems farther than actual distance, when object is in denser medium and observer is in rarer medium then object seems nearer than the actual distance.