NCERT Solutions for Class 7 Maths Chapter 14 Symmetry - PDF Download
FAQs on NCERT Solutions for Class 7 Maths Chapter 12 Symmetry
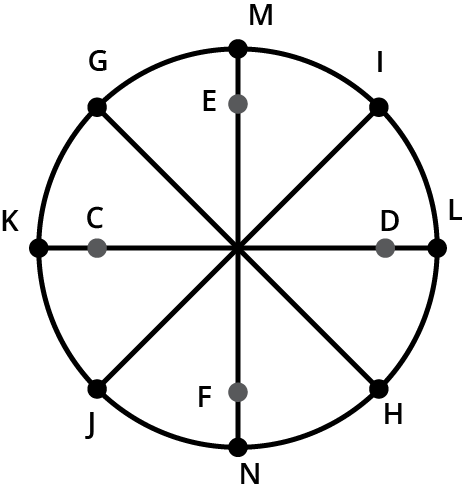
Q1. What is Rotational Symmetry and What is the Order of Rotational Symmetry?
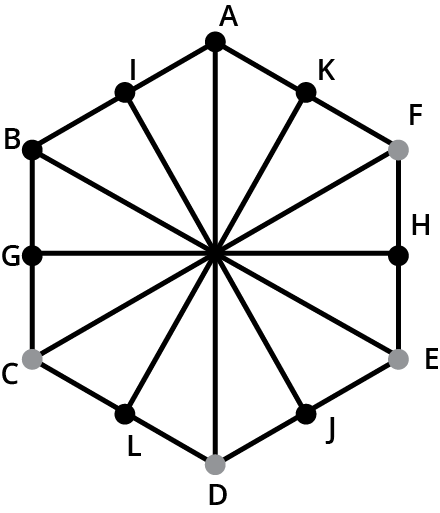
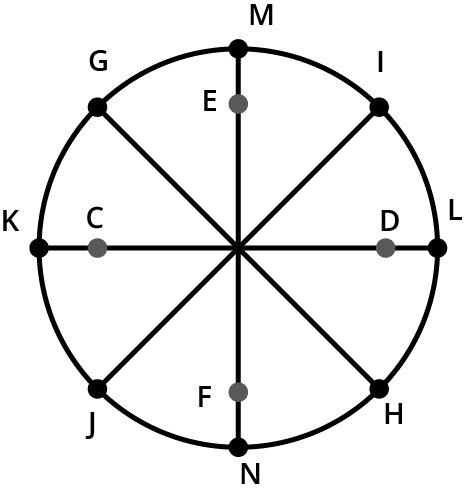
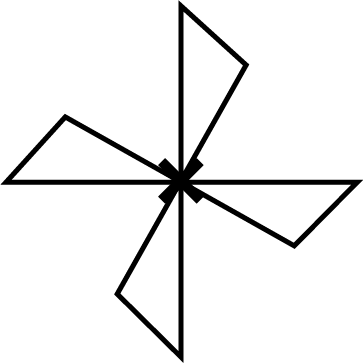
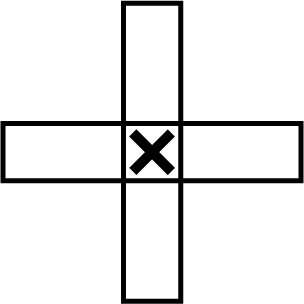
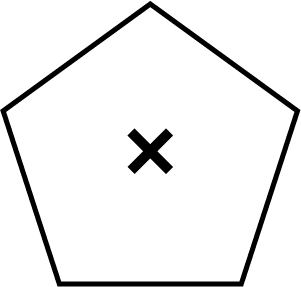
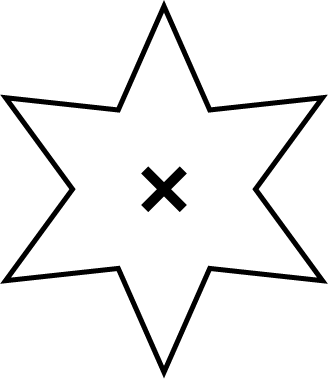
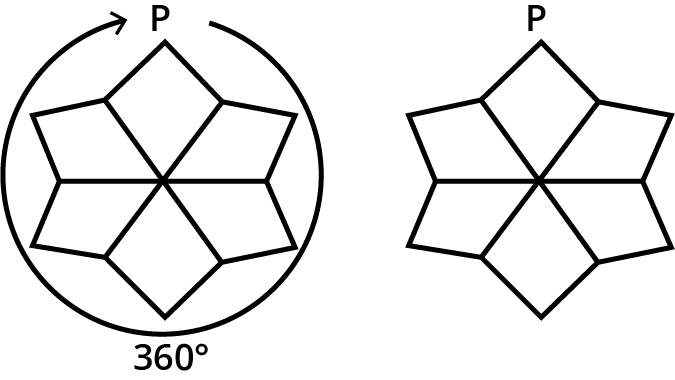
Ans: A figure is said to have the rotational symmetry if it fits into itself more than once during a complete rotation.
The number of times a figure fits onto itself in one complete rotation is called the order of rotational symmetry.
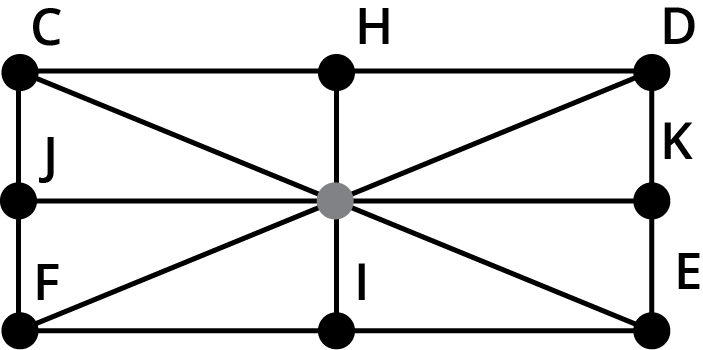
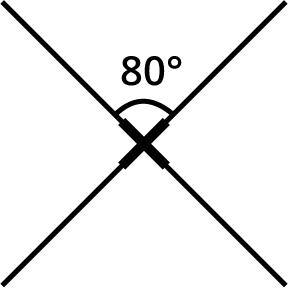
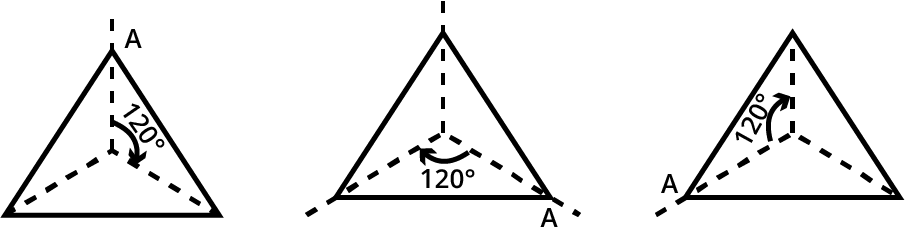
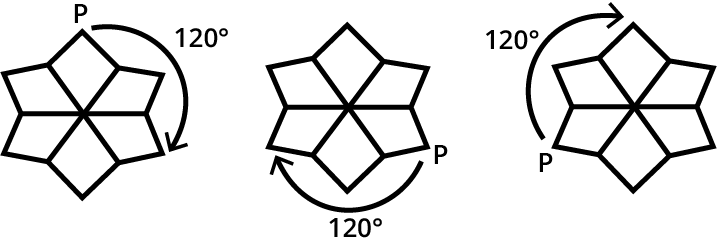
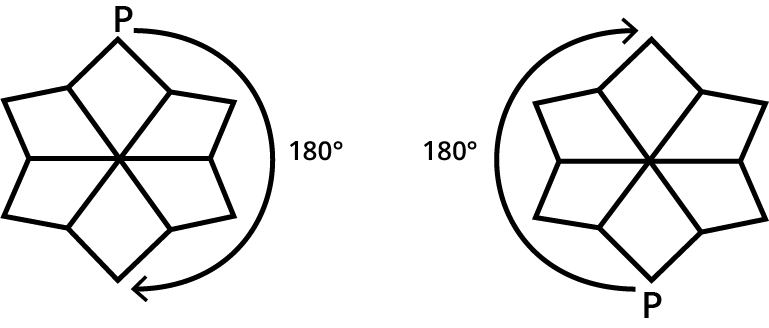
Q2. After Rotating by 600 about a Center, a Figure Looks Exactly the Same as its Original Position. At What Other Angles will this Happen for the Figure?
Ans: The figure will look exactly the same as its original position at 1200, 1800, 2400, 3000, and 3600.
Q3. Fill in the Blanks
The number of times a shape will fit onto itself in one complete turn is called ________________ of the rotational symmetry.
An equilateral triangle has _____________ symmetry of order three.
A square has a rotational symmetry of order ________________.
A rectangle has a rotational symmetry of order _________________.
Ans:
Order
Rotational
Four
Two
Q4. What are the Benefits of Learning from Vedantu?
Ans: Vedantu has a panel of expert teachers. In Vedantu, it is their commitment and responsibility to bring the best out of a student. It is a matter of pride for each and everyone in Vedantu when a student scores well in the exams. All the teachers are qualified and experienced and they understand the value of hard work. All the study materials on Vedantu are available for free download. They are also well versed in the psychology of a student and focus on them accordingly. Vedantu has a network of passionate individuals and strives to contribute towards the happiness and joy of each child through learning. So you can rest assured that your child is in the safest hands.
Q5. Where can you find symmetry in your daily life?
Ans: Chapter 14 of the NCERT Solutions for Class 7 Maths Symmetry is a term that describes the concept of symmetry, which can be seen practically everywhere in our surroundings, in nature, and real-life situations. Symmetric patterns can be found in many natural events, including the growth and development of living beings, flowers, trees, and leaves, among others. Visit Vedantu’s official website to get the solutions so that you can get all the things around you before you start practicing.
Q6. What all things can be used to create symmetries?
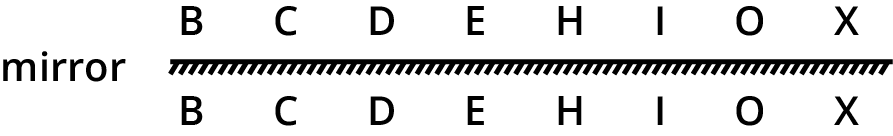
Ans: Architecture, as well as art and music, can all be used to create symmetry. In the same way, Mathematics is regulated by symmetries or patterns that repeat themselves over and over. Vedantu has all the solved questions which you can find on the website. Start by recalling the idea of line symmetry, which refers to a line along which the parts of a figure will meet when folded. The axis is another name for this symmetry line.
Q7. What is symmetry in nature?
Ans: One of the distinctive characteristics of life is symmetry, which is one of the fundamental principles that give rise to a wide range of phenomena, from fractals to crystals and hurricanes. Symmetries can be found in almost any subject, from the mechanics of waves and turbulence to the economic theories that underpin monetary systems. Vedantu has all the basic concepts related to this chapter, there you will find all the solutions and the whole chapter. You can even download books from its website.
Q8. How can students solve symmetry questions of Chapter 14 of Class 7 Maths easily?
Ans: Certain requirements must be met for a figure to be considered symmetrical. To assess and identify the given figure as symmetrical while utilizing the understanding of these criteria, students must have committed observational skills and attentiveness. As a result, these requirements or logic must be remembered. The following are a few of them.
Regular Polygon Lines of Symmetry: The number of lines of symmetry in a regular polygon equals the number of sides.
Rotational Symmetry Order: The number of times an object appears the same after a complete 360-degree turn is known as the order of rotational symmetry. It will be 4, for example, for a square.
Q9. How can CBSE students properly use NCERT Solutions for Chapter 14 of Class 7 Maths?
Ans: Since the CBSE board suggests studying from the NCERT Solutions for Chapter 14 of Class 7 Maths students must ensure that they practice all of the solved examples as well as the exercise questions in the book. They should pay close attention to how the assertions are structured in the solved instances, as this will give them an idea of how to answer a problem in the tests by demonstrating proper logic step by step. As a result, continuous practice will aid pupils in making better use of this crucial learning resource.